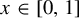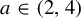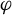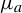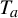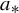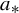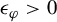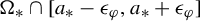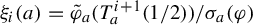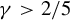1 Introduction
1.1 Background and motivation
Let
![]() $(\Omega _{*},m_{*},\mathcal {F}_{*})$
be a probability space. We say that a sequence of measurable functions
$(\Omega _{*},m_{*},\mathcal {F}_{*})$
be a probability space. We say that a sequence of measurable functions
![]() $\xi _i \colon \Omega _{*} \to {\mathbb R}$
,
$\xi _i \colon \Omega _{*} \to {\mathbb R}$
,
![]() $i\ge 1$
satisfies the almost sure invariance principle (ASIP) with error exponent
$i\ge 1$
satisfies the almost sure invariance principle (ASIP) with error exponent
![]() $\gamma <1/2$
if there exist a probability space
$\gamma <1/2$
if there exist a probability space
![]() $(\Omega _W, m_W,\mathcal {F}_W)$
supporting a (centred) one-dimensional Brownian motion W and a sequence of measurable functions
$(\Omega _W, m_W,\mathcal {F}_W)$
supporting a (centred) one-dimensional Brownian motion W and a sequence of measurable functions
![]() $\eta _i \colon \Omega _W\to {\mathbb R}$
,
$\eta _i \colon \Omega _W\to {\mathbb R}$
,
![]() $i\ge 1$
, such that:
$i\ge 1$
, such that:
-
(i) the random variables
 $\{\xi _i\}_{i\ge 1}$
and
$\{\xi _i\}_{i\ge 1}$
and
 $\{\eta _i\}_{i\ge 1}$
have the same distribution. (By definition of the distribution of discrete-time real-valued stochastic processes, this means that for any
$\{\eta _i\}_{i\ge 1}$
have the same distribution. (By definition of the distribution of discrete-time real-valued stochastic processes, this means that for any
 $n\ge 1$
and any
$n\ge 1$
and any
 $\{y_i\in {\mathbb R}\mid 1\le i \le n\}$
, the joint probability that
$\{y_i\in {\mathbb R}\mid 1\le i \le n\}$
, the joint probability that
 $\xi _i\le y_i$
for all
$\xi _i\le y_i$
for all
 $1\le i \le n$
coincides with the joint probability that
$1\le i \le n$
coincides with the joint probability that
 $\eta _i\le y_i$
for all
$\eta _i\le y_i$
for all
 $1\le i \le n$
.)
$1\le i \le n$
.) -
(ii) Almost surely,
 $ |W(n)-\sum _{i=1}^{n}\eta _i|=O(n^\gamma ) $
as
$ |W(n)-\sum _{i=1}^{n}\eta _i|=O(n^\gamma ) $
as
 $n\to \infty $
.
$n\to \infty $
.
Since a Brownian motion at integer times coincides with a sum of independent identically distributed (i.i.d.) Gaussian variables, the above definition can also be formulated as an almost sure approximation, with error
![]() $o(n^{\gamma })$
, by a sum of i.i.d. Gaussian variables.
$o(n^{\gamma })$
, by a sum of i.i.d. Gaussian variables.
It is a classical result (see [Reference Philipp and StoutPS]) that if the
![]() $\{\xi _i\}$
satisfies the ASIP, then it satisfies the law of the iterated logarithm (LIL), the central limit theorem (CLT) and the functional CLT: Letting
$\{\xi _i\}$
satisfies the ASIP, then it satisfies the law of the iterated logarithm (LIL), the central limit theorem (CLT) and the functional CLT: Letting
![]() $\sigma ^2>0$
be the variance of the Brownian motion W (the expectation is zero by assumption) and denoting Lebesgue measure by m, the LIL says that
$\sigma ^2>0$
be the variance of the Brownian motion W (the expectation is zero by assumption) and denoting Lebesgue measure by m, the LIL says that
 $$ \begin{align*} \limsup_{n\to\infty}\frac1{\sqrt{2n\log\log n}}\sum_{i=1}^n \xi_i(a) =\sigma\quad\text{for } m_{*}\text{-almost every (a.e.) } a\in \Omega_{*}, \end{align*} $$
$$ \begin{align*} \limsup_{n\to\infty}\frac1{\sqrt{2n\log\log n}}\sum_{i=1}^n \xi_i(a) =\sigma\quad\text{for } m_{*}\text{-almost every (a.e.) } a\in \Omega_{*}, \end{align*} $$
and the CLT (for the functional CLT, see [Reference de Lima and SmaniaDLS, Lemma 5.1]) says that
 $$ \begin{align*} \lim_{n\to\infty}m_{*} \bigg( \bigg\{\, a \in \Omega_{*} \mid \frac1{\sigma\sqrt{n}} \sum_{i=1}^n\xi_i(a)\le y \,\bigg\} \bigg) =\frac1{\sqrt{2\pi}}\int_{-\infty}^ye^{-s^2/2}\, ds \quad \text{for all } y \in {\mathbb R}. \end{align*} $$
$$ \begin{align*} \lim_{n\to\infty}m_{*} \bigg( \bigg\{\, a \in \Omega_{*} \mid \frac1{\sigma\sqrt{n}} \sum_{i=1}^n\xi_i(a)\le y \,\bigg\} \bigg) =\frac1{\sqrt{2\pi}}\int_{-\infty}^ye^{-s^2/2}\, ds \quad \text{for all } y \in {\mathbb R}. \end{align*} $$
We consider
![]() $I=[0,1]$
and the quadratic family
$I=[0,1]$
and the quadratic family
Denote by
![]() $c=1/2$
the critical point of
$c=1/2$
the critical point of
![]() $T_a$
and set
$T_a$
and set
![]() $ c_{j}(a)=T_a^{j}(c)$
for
$ c_{j}(a)=T_a^{j}(c)$
for
![]() $j\ge 1$
.
$j\ge 1$
.
If
![]() $\liminf _{n \to \infty } n^{-1} \log \partial _x(T_a^n) (T_a(c))> 0$
, we say that a is a Collet–Eckmann (CE) parameter. If a is CE, then
$\liminf _{n \to \infty } n^{-1} \log \partial _x(T_a^n) (T_a(c))> 0$
, we say that a is a Collet–Eckmann (CE) parameter. If a is CE, then
![]() $T_a$
admits a unique absolutely continuous invariant probability measure (acim)
$T_a$
admits a unique absolutely continuous invariant probability measure (acim)
![]() $\mu _a=h_a\,dm$
. Our goal is to find a positive Lebesgue measure set
$\mu _a=h_a\,dm$
. Our goal is to find a positive Lebesgue measure set
![]() $\Omega _{*}$
of CE parameters with a Lebesgue density point
$\Omega _{*}$
of CE parameters with a Lebesgue density point
![]() $a_{*}\in \Omega _{*}$
such that for any Hölder continuous function
$a_{*}\in \Omega _{*}$
such that for any Hölder continuous function
![]() $\varphi \colon I\to {\mathbb R}$
with
$\varphi \colon I\to {\mathbb R}$
with
![]() $\sigma _{a_{*}}(\varphi )\ne 0$
(see equation (1.2)), there exists
$\sigma _{a_{*}}(\varphi )\ne 0$
(see equation (1.2)), there exists
![]() $\epsilon _\varphi>0$
such that the ASIP holds for
$\epsilon _\varphi>0$
such that the ASIP holds for
![]() $m_{*}$
the normalized Lebesgue measure on
$m_{*}$
the normalized Lebesgue measure on
![]() $\Omega _{*}\cap [a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
and
$\Omega _{*}\cap [a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
and
where
![]() $\varphi _a$
is a suitable normalization of
$\varphi _a$
is a suitable normalization of
![]() $\varphi $
(see equation (1.6)). We follow the approach of Schnellmann [Reference SchnellmannSch], who developed this program for transversal families of piecewise expanding maps
$\varphi $
(see equation (1.6)). We follow the approach of Schnellmann [Reference SchnellmannSch], who developed this program for transversal families of piecewise expanding maps
![]() $T_a$
, for which
$T_a$
, for which
![]() $\Omega _{*}$
can be taken to be an interval.
$\Omega _{*}$
can be taken to be an interval.
Our main motivation is to extend to the quadratic family the method developed by de Lima and Smania [Reference de Lima and SmaniaDLS] in the setting of piecewise expanding maps, to study linear and fractional response. (This method requires a functional central limit theorem, see [Reference de Lima and SmaniaDLS, Lemma 5.1].)
We say that
![]() $T_a$
is mixing if it is topologically mixing on
$T_a$
is mixing if it is topologically mixing on
It will be convenient below to restrict to mixing maps
![]() $T_a$
. Tiozzo recently showed [Reference TiozzoTi, Corollary 3.15] (his result holds in fact for more general unimodal maps) that
$T_a$
. Tiozzo recently showed [Reference TiozzoTi, Corollary 3.15] (his result holds in fact for more general unimodal maps) that
![]() $T_a$
is (strongly) mixing for its unique measure of maximal entropy (MME) if its topological entropy is greater than
$T_a$
is (strongly) mixing for its unique measure of maximal entropy (MME) if its topological entropy is greater than
![]() $\log (2)/2$
. If a is a CE parameter with strongly mixing MME, then
$\log (2)/2$
. If a is a CE parameter with strongly mixing MME, then
![]() $T_a$
is topologically mixing on
$T_a$
is topologically mixing on
![]() $K(a)$
since the measure of maximal entropy has full support there. (Indeed, since
$K(a)$
since the measure of maximal entropy has full support there. (Indeed, since
![]() $T_a$
has no homtervals if
$T_a$
has no homtervals if
![]() $a\in CE$
, it is conjugated to its piecewise linear model
$a\in CE$
, it is conjugated to its piecewise linear model
![]() $F_a$
by a homeomorphism which maps the MME of
$F_a$
by a homeomorphism which maps the MME of
![]() $F_a$
to the MME of
$F_a$
to the MME of
![]() $T_a$
, and the MME of
$T_a$
, and the MME of
![]() $F_a$
is absolutely continuous with a positive density on
$F_a$
is absolutely continuous with a positive density on
![]() $[F^2_a(c), F_a(c)]$
.) Since the topological entropy of
$[F^2_a(c), F_a(c)]$
.) Since the topological entropy of
![]() $T_4$
is equal to
$T_4$
is equal to
![]() $\log 2$
, and the topological entropy of
$\log 2$
, and the topological entropy of
![]() $T_a$
is non-decreasing and continuous (in fact Hölder continuous [Reference Guckenheimer, Nitecki and RobinsonGu]) in a, there exists
$T_a$
is non-decreasing and continuous (in fact Hölder continuous [Reference Guckenheimer, Nitecki and RobinsonGu]) in a, there exists
![]() $a_{\mathrm {mix}}<4$
such that for all
$a_{\mathrm {mix}}<4$
such that for all
![]() $a \in (a_{\mathrm {mix}}, 4]\cap CE$
, the map
$a \in (a_{\mathrm {mix}}, 4]\cap CE$
, the map
![]() $T_a$
is topologically mixing on
$T_a$
is topologically mixing on
![]() $K(a)$
, and
$K(a)$
, and
![]() $\mu _a$
is strongly mixing, with support
$\mu _a$
is strongly mixing, with support
![]() $K(a)$
.
$K(a)$
.
Melbourne and Nicol [Reference Melbourne and NicolMN] showed the ASIP in the phase space
![]() $x\in K(a)$
, setting
$x\in K(a)$
, setting
![]() $\xi _i=T^i_a(x)$
for a fixed CE map
$\xi _i=T^i_a(x)$
for a fixed CE map
![]() $T_a$
, using an induced uniformly expanding system (then [Reference Philipp and StoutPS, §7] provides an ASIP which projects to the ASIP for the original CE map). However, to the best of our knowledge, the ASIP in the parameter a is still open.
$T_a$
, using an induced uniformly expanding system (then [Reference Philipp and StoutPS, §7] provides an ASIP which projects to the ASIP for the original CE map). However, to the best of our knowledge, the ASIP in the parameter a is still open.
In the parameter space, typicality (the law of large numbers, LLN) and the LIL are known: Avila and Moreira [Reference Avila and MoreiraAM2] showed that for Lebesgue almost every CE map
![]() $T_a$
, the critical point is typical for its unique absolutely continuous invariant measure
$T_a$
, the critical point is typical for its unique absolutely continuous invariant measure
![]() $\mu _a=h_a dm$
:
$\mu _a=h_a dm$
:
 $$ \begin{align} \lim_{n\to\infty} \frac1n \sum_{i=1}^n \varphi (c_i(a)) = \int_0^1 \varphi \, d\mu_a \quad\text{for all } \varphi\in C^0. \end{align} $$
$$ \begin{align} \lim_{n\to\infty} \frac1n \sum_{i=1}^n \varphi (c_i(a)) = \int_0^1 \varphi \, d\mu_a \quad\text{for all } \varphi\in C^0. \end{align} $$
(Benedicks and Carleson established typicality in [Reference Benedicks and CarlesonBC1] for the Cantor set of CE parameters considered there.) For Hölder continuous
![]() $\varphi \colon I\to {\mathbb R}$
and a topological mixing CE parameter a, define
$\varphi \colon I\to {\mathbb R}$
and a topological mixing CE parameter a, define
![]() $\sigma _a( \varphi )\ge 0$
by
$\sigma _a( \varphi )\ge 0$
by
 $$ \begin{align} ( \sigma_a(\varphi))^2 &:= \int_0^1 \bigg( \varphi-\int\varphi \,d\mu_a \bigg)^2 \, d\mu_a \end{align} $$
$$ \begin{align} ( \sigma_a(\varphi))^2 &:= \int_0^1 \bigg( \varphi-\int\varphi \,d\mu_a \bigg)^2 \, d\mu_a \end{align} $$
 $$ \begin{align} &\quad + 2\sum_{i>0} \int_0^1 \bigg( \varphi - \int\varphi \, d\mu_a \bigg) \bigg( \varphi - \int \varphi \, d\mu_a \bigg) \circ T^i_a \, d\mu_a, \end{align} $$
$$ \begin{align} &\quad + 2\sum_{i>0} \int_0^1 \bigg( \varphi - \int\varphi \, d\mu_a \bigg) \bigg( \varphi - \int \varphi \, d\mu_a \bigg) \circ T^i_a \, d\mu_a, \end{align} $$
where the sum in equation (1.3) is finite because topological mixing (that is, the fact that the map is non-renormalizable) implies [Reference Keller and NowickiKN] exponential mixing for the acim and Hölder continuous observables.
In a work in progress, Gao and Shen [Reference Gao and ShenGS2] show that, for Lebesgue-a.e. a in the set of mixing CE parameters, for every Hölder observable
![]() $\varphi $
, either
$\varphi $
, either
![]() $\sigma _a(\varphi )=0$
or the critical point c of
$\sigma _a(\varphi )=0$
or the critical point c of
![]() $T_a$
satisfies the LIL for
$T_a$
satisfies the LIL for
![]() $\varphi $
, that is,
$\varphi $
, that is,
 $$ \begin{align*} \limsup_{n\to\infty} \frac1{\sqrt{2n\log\log n}}\sum_{i=1}^n \bigg( \varphi(T_a^i(c))-\int\varphi \, d\mu_a \bigg) = \sigma_a(\varphi). \end{align*} $$
$$ \begin{align*} \limsup_{n\to\infty} \frac1{\sqrt{2n\log\log n}}\sum_{i=1}^n \bigg( \varphi(T_a^i(c))-\int\varphi \, d\mu_a \bigg) = \sigma_a(\varphi). \end{align*} $$
1.2 Statement of the ASIP (Theorem 1.1)
To state our main result, we need more notation and definitions. For
![]() $j\ge 0$
and
$j\ge 0$
and
![]() $a \in (a_{\mathrm {mix}},4]$
, set
$a \in (a_{\mathrm {mix}},4]$
, set
The family
![]() $T_a$
is transversal at
$T_a$
is transversal at
![]() ${a_{*}}$
if (see [Reference TsujiiTs1]) there exists
${a_{*}}$
if (see [Reference TsujiiTs1]) there exists
![]() $C\ge 1$
such that
$C\ge 1$
such that
 $$ \begin{align} \frac{1}{C}\le \bigg| \frac{x_j^{\prime}(a_{*})}{(T_{a_{*}}^j)^{\prime} (c_1(a_{*}))} \bigg| \le C\quad\text{for all } j\ge1. \end{align} $$
$$ \begin{align} \frac{1}{C}\le \bigg| \frac{x_j^{\prime}(a_{*})}{(T_{a_{*}}^j)^{\prime} (c_1(a_{*}))} \bigg| \le C\quad\text{for all } j\ge1. \end{align} $$
By [Reference TsujiiTs2, Theorem 3], all CE parameters are transversal. We refer to [Reference TsujiiTs1, (
![]() $N{V}_t$
)] for an equivalent condition expressed in terms of the postcritical orbit.
$N{V}_t$
)] for an equivalent condition expressed in terms of the postcritical orbit.
The map
![]() $T_a$
is
$T_a$
is
![]() $(H_a, \kappa _a)$
-polynomially recurrent, for
$(H_a, \kappa _a)$
-polynomially recurrent, for
![]() $\kappa _a\ge 1$
and
$\kappa _a\ge 1$
and
![]() $H_a\ge 1$
, if
$H_a\ge 1$
, if
If
![]() $\inf _{j\ge 1} |T_a^j(c)-c|>0$
, then a is called a Misiurewicz parameter. Misiurewicz parameters are CE and thus transversal. Avila and Moreira [Reference Avila and MoreiraAM1] showed that, for any
$\inf _{j\ge 1} |T_a^j(c)-c|>0$
, then a is called a Misiurewicz parameter. Misiurewicz parameters are CE and thus transversal. Avila and Moreira [Reference Avila and MoreiraAM1] showed that, for any
![]() $\kappa _0>1$
, the set of parameters a which are
$\kappa _0>1$
, the set of parameters a which are
![]() $(H_a, \kappa _0)$
-polynomially recurrent for some
$(H_a, \kappa _0)$
-polynomially recurrent for some
![]() $H_a$
has full measure in the set of CE parameters. The set of Misiurewicz parameters a is uncountable (it has full Hausdorff dimension [Reference ZakeriZa, Theorem 1.4] but zero Lebesgue measure).
$H_a$
has full measure in the set of CE parameters. The set of Misiurewicz parameters a is uncountable (it has full Hausdorff dimension [Reference ZakeriZa, Theorem 1.4] but zero Lebesgue measure).
Finally, we introduce the normalization
![]() $\varphi _a$
: let
$\varphi _a$
: let
![]() $\varphi $
be bounded such that
$\varphi $
be bounded such that
![]() $\sigma _{a}(\varphi )\ne 0$
for a mixing CE parameter a. Then the function
$\sigma _{a}(\varphi )\ne 0$
for a mixing CE parameter a. Then the function
 $$ \begin{align} \varphi_a(x):=\frac1{\sigma_a(\varphi)} \bigg(\varphi(x)-\int_0^1\varphi \, d\mu_a \bigg) \, \end{align} $$
$$ \begin{align} \varphi_a(x):=\frac1{\sigma_a(\varphi)} \bigg(\varphi(x)-\int_0^1\varphi \, d\mu_a \bigg) \, \end{align} $$
is well defined and satisfies
Theorem 1.1. (Main Theorem: ASIP)
For any Misiurewicz parameter
![]() $a_{*}\in (a_{\mathrm {mix}},4)$
, there exists a positive Lebesgue measure set
$a_{*}\in (a_{\mathrm {mix}},4)$
, there exists a positive Lebesgue measure set
![]() $\Omega _{*}$
of mixing polynomially recurrent parameters, containing
$\Omega _{*}$
of mixing polynomially recurrent parameters, containing
![]() $a_{*}$
as a Lebesgue density point, such that for any Hölder continuous function
$a_{*}$
as a Lebesgue density point, such that for any Hölder continuous function
![]() $\varphi $
with
$\varphi $
with
![]() $\sigma _{a_{*}}(\varphi )\ne 0$
, there exists
$\sigma _{a_{*}}(\varphi )\ne 0$
, there exists
![]() $\epsilon _\varphi>0$
such that the functions
$\epsilon _\varphi>0$
such that the functions
satisfy the ASIP for normalized Lebesgue measure
![]() $m_{*}$
on
$m_{*}$
on
![]() $\Omega _{*} \cap [a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
and all error exponents
$\Omega _{*} \cap [a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
and all error exponents
![]() $\gamma>2/5$
. (The choice of
$\gamma>2/5$
. (The choice of
![]() $\epsilon _\varphi $
ensures in particular that
$\epsilon _\varphi $
ensures in particular that
![]() $\sigma _a(\varphi )\ne 0$
if
$\sigma _a(\varphi )\ne 0$
if
![]() $\sigma _{a_{*}}(\varphi )\ne 0$
.)
$\sigma _{a_{*}}(\varphi )\ne 0$
.)
The value
![]() $a_{*}=4$
is not covered by our arguments for technical reasons, since
$a_{*}=4$
is not covered by our arguments for technical reasons, since
![]() $c_1$
and
$c_1$
and
![]() $c_2$
then lie on the boundary of I. (For example, the Banach space of [Reference Baladi, Benedicks and SchnellmannBBS] requires that the function on level zero of the tower be supported in
$c_2$
then lie on the boundary of I. (For example, the Banach space of [Reference Baladi, Benedicks and SchnellmannBBS] requires that the function on level zero of the tower be supported in
![]() $(0,1)$
, so this proof cannot cover the case
$(0,1)$
, so this proof cannot cover the case
![]() $a=4$
.) It is possible (but a bit cumbersome) to handle (a one-sided neighbourhood of) this value by a change of coordinates as in [Reference TsujiiTs1, Lemma 2.1].
$a=4$
.) It is possible (but a bit cumbersome) to handle (a one-sided neighbourhood of) this value by a change of coordinates as in [Reference TsujiiTs1, Lemma 2.1].
We expect that the methods of this paper can be extended to the case when the ‘root’
![]() $a_{*}$
is mixing, but only Collet–Eckmann and polynomially recurrent (for large enough
$a_{*}$
is mixing, but only Collet–Eckmann and polynomially recurrent (for large enough
![]() $\kappa _0>1$
), instead of Misiurewicz. (For example, [Reference AspenbergA, Lemma 8.1] would replace [Reference de Melo and van StrienDMS, Lemma V.6.5] in the proof of Proposition 2.2.) We restrict here to Misiurewicz parameters
$\kappa _0>1$
), instead of Misiurewicz. (For example, [Reference AspenbergA, Lemma 8.1] would replace [Reference de Melo and van StrienDMS, Lemma V.6.5] in the proof of Proposition 2.2.) We restrict here to Misiurewicz parameters
![]() $a_{*}$
for the sake of simplicity. What is most desirable, in view of our original motivation to extend the analysis of [Reference de Lima and SmaniaDLS], is to obtain a ‘fatter’ Cantor set
$a_{*}$
for the sake of simplicity. What is most desirable, in view of our original motivation to extend the analysis of [Reference de Lima and SmaniaDLS], is to obtain a ‘fatter’ Cantor set
![]() $\Omega _{*}$
(as opposed to a fatter set of root points
$\Omega _{*}$
(as opposed to a fatter set of root points
![]() $a_{*}$
). Indeed, this extension will probably require the ASIP on a set
$a_{*}$
). Indeed, this extension will probably require the ASIP on a set
![]() $\widetilde \Omega $
for which there exist
$\widetilde \Omega $
for which there exist
![]() $\beta>1$
and a full measure subset
$\beta>1$
and a full measure subset
![]() $\widetilde \Omega _1\subset \widetilde \Omega $
such that
$\widetilde \Omega _1\subset \widetilde \Omega $
such that
 $$ \begin{align} \lim_{\epsilon \to 0} \frac{m([a-\epsilon, a+ \epsilon] \setminus \widetilde \Omega)}{\epsilon^\beta} = 0 \quad\text{for all } a \in \widetilde \Omega_1. \end{align} $$
$$ \begin{align} \lim_{\epsilon \to 0} \frac{m([a-\epsilon, a+ \epsilon] \setminus \widetilde \Omega)}{\epsilon^\beta} = 0 \quad\text{for all } a \in \widetilde \Omega_1. \end{align} $$
(See [Reference Baladi and SmaniaBS2, equation (5), Proposition F], note that [Reference Baladi and SmaniaBS2, Lemma E] even uses
![]() $\beta <2$
close to
$\beta <2$
close to
![]() $2$
.) Equation (1.9) is known for all
$2$
.) Equation (1.9) is known for all
![]() $\beta <2$
for the sets
$\beta <2$
for the sets
![]() $\widetilde \Omega _1\subset \widetilde \Omega $
studied by Tsujii [Reference TsujiiTs1]. (Beware that Tsujii’s result cannot be used immediately. In particular, the main argument in the construction of the parameter set in Theorem 1 of ‘Pre-threshold fractional susceptibility function: holomorphy and response formula’, arXiv:2203.07942, is flawed.) For our Cantor set
$\widetilde \Omega _1\subset \widetilde \Omega $
studied by Tsujii [Reference TsujiiTs1]. (Beware that Tsujii’s result cannot be used immediately. In particular, the main argument in the construction of the parameter set in Theorem 1 of ‘Pre-threshold fractional susceptibility function: holomorphy and response formula’, arXiv:2203.07942, is flawed.) For our Cantor set
![]() $\Omega _{*}\subset \Omega _\mathrm{BC}$
, we expect that for any
$\Omega _{*}\subset \Omega _\mathrm{BC}$
, we expect that for any
![]() $\kappa>1$
, taking
$\kappa>1$
, taking
![]() $\kappa _0$
large enough in Proposition 2.2, the factor
$\kappa _0$
large enough in Proposition 2.2, the factor
![]() $\epsilon ^\beta $
in equation (1.9) must be replaced by
$\epsilon ^\beta $
in equation (1.9) must be replaced by
![]() $\epsilon |\log \epsilon |^{-\kappa }$
(see equation (2.20)), which does not seem good enough. Attaining the goal of our original motivation may thus require establishing the ASIP on a Cantor set having larger density, and thus weakening the polynomial lower recurrence in the construction (see comments in the next paragraph). We view this as the most desirable improvement of our main theorem.
$\epsilon |\log \epsilon |^{-\kappa }$
(see equation (2.20)), which does not seem good enough. Attaining the goal of our original motivation may thus require establishing the ASIP on a Cantor set having larger density, and thus weakening the polynomial lower recurrence in the construction (see comments in the next paragraph). We view this as the most desirable improvement of our main theorem.
To clarify the role of
![]() $\Omega _{*}$
, it is useful to compare Schnellmann’s proof with ours. In [Reference SchnellmannSch], Schnellmann studies suitable transversal one-parameter families of piecewise expanding interval maps and obtains a parameter ASIP on a set
$\Omega _{*}$
, it is useful to compare Schnellmann’s proof with ours. In [Reference SchnellmannSch], Schnellmann studies suitable transversal one-parameter families of piecewise expanding interval maps and obtains a parameter ASIP on a set
![]() $\Omega _{*}$
which is just an interval
$\Omega _{*}$
which is just an interval
![]() $[0,\epsilon ^\varphi ]$
of parameters. Indeed, existence of an exponentially mixing acim enjoying fractional response (with uniform bounds) holds in an entire interval
$[0,\epsilon ^\varphi ]$
of parameters. Indeed, existence of an exponentially mixing acim enjoying fractional response (with uniform bounds) holds in an entire interval
![]() $[0,\epsilon ^\varphi ]$
in his setting [Reference SchnellmannSch, Proposition 4.3, Lemma 4.5]. So,
$[0,\epsilon ^\varphi ]$
in his setting [Reference SchnellmannSch, Proposition 4.3, Lemma 4.5]. So,
![]() $[0,\epsilon ^\varphi ]$
is the baseline parameter space for his analysis. Some parameters in this baseline cause difficulties (‘exceptionally small sets’), but Schnellmann can get away with just ignoring them (taking advantage of the fact that their total measure is controlled [Reference SchnellmannSch, part (III), Theorem 3.2, Lemma 4.1, proof of Lemmas 6.1 and 6.2]) instead of excluding them from the baseline. Our situation is different, since we need to exclude parameters which do not have an acim or for which exponential mixing or fractional response (with uniform bounds) does not hold. Our baseline set is a Cantor set, and the best we can do is to make it as fat as possible.
$[0,\epsilon ^\varphi ]$
is the baseline parameter space for his analysis. Some parameters in this baseline cause difficulties (‘exceptionally small sets’), but Schnellmann can get away with just ignoring them (taking advantage of the fact that their total measure is controlled [Reference SchnellmannSch, part (III), Theorem 3.2, Lemma 4.1, proof of Lemmas 6.1 and 6.2]) instead of excluding them from the baseline. Our situation is different, since we need to exclude parameters which do not have an acim or for which exponential mixing or fractional response (with uniform bounds) does not hold. Our baseline set is a Cantor set, and the best we can do is to make it as fat as possible.
The polynomial recurrence in equation (1.5) in our parameter exclusion (Proposition 2.2), which causes the ‘thinness’ of
![]() $\Omega _{*}$
, is needed to apply the results of [Reference Baladi, Benedicks and SchnellmannBBS] in §§2.4 and 2.5 (Propositions 2.5 and 2.6 on uniform decorrelation and fractional response, and its consequence, Lemma 2.8). (See also (4.7) and (4.22), which may cause a different error exponent.) Due to this, we already exclude the parameters which could have exceptionally small image and we do not need to ignore them (Lemma 2.3, compare also [Reference SchnellmannSch, proof of Lemma 6.1] with equation (4.13) below). In addition, we get an easy proof of the local distortion estimate in equation (2.31). If the required consequences of [Reference Baladi, Benedicks and SchnellmannBBS] could be extended to sets of parameters which enjoy only exponential recurrence bounds, then we could use the (fatter) Benedicks–Carleson Cantor set
$\Omega _{*}$
, is needed to apply the results of [Reference Baladi, Benedicks and SchnellmannBBS] in §§2.4 and 2.5 (Propositions 2.5 and 2.6 on uniform decorrelation and fractional response, and its consequence, Lemma 2.8). (See also (4.7) and (4.22), which may cause a different error exponent.) Due to this, we already exclude the parameters which could have exceptionally small image and we do not need to ignore them (Lemma 2.3, compare also [Reference SchnellmannSch, proof of Lemma 6.1] with equation (4.13) below). In addition, we get an easy proof of the local distortion estimate in equation (2.31). If the required consequences of [Reference Baladi, Benedicks and SchnellmannBBS] could be extended to sets of parameters which enjoy only exponential recurrence bounds, then we could use the (fatter) Benedicks–Carleson Cantor set
![]() $\Omega ^\varphi _\mathrm{BC}$
as a baseline instead of
$\Omega ^\varphi _\mathrm{BC}$
as a baseline instead of
![]() $\Omega _{*}$
(if necessary, the Benedicks–Carleson technique could be replaced by ideas from Tsujii [Reference TsujiiTs1], Avila and Moreira [Reference Avila and MoreiraAM1] or Gao and Shen [Reference Gao and ShenGS1]). Next, one could try to ignore the parameters with exceptionally small images in Lemma 2.3. For equation (2.31), our proof is inspired from that of [Reference de Melo and van StrienDMS, Theorem V.6.2]. This is suboptimal but enough for our purposes. Adapting instead [Reference de Melo and van StrienDMS, Lemma V.6.4] could enhance equation (2.31).
$\Omega _{*}$
(if necessary, the Benedicks–Carleson technique could be replaced by ideas from Tsujii [Reference TsujiiTs1], Avila and Moreira [Reference Avila and MoreiraAM1] or Gao and Shen [Reference Gao and ShenGS1]). Next, one could try to ignore the parameters with exceptionally small images in Lemma 2.3. For equation (2.31), our proof is inspired from that of [Reference de Melo and van StrienDMS, Theorem V.6.2]. This is suboptimal but enough for our purposes. Adapting instead [Reference de Melo and van StrienDMS, Lemma V.6.4] could enhance equation (2.31).
We also note for the record here that the characteristic function
![]() $1_{\widetilde \Omega }$
of a fat enough Cantor set
$1_{\widetilde \Omega }$
of a fat enough Cantor set
![]() $\widetilde \Omega $
belongs to a Sobolev space
$\widetilde \Omega $
belongs to a Sobolev space
![]() $H^s_q(I)$
with
$H^s_q(I)$
with
![]() $s>0$
(see [Reference Hewett and MoiolaHM, Propositions 4.9 and 4.10]). Thus, working with a Cantor set of larger density may simplify some of our arguments (in the proof of Proposition 3.2, for example).
$s>0$
(see [Reference Hewett and MoiolaHM, Propositions 4.9 and 4.10]). Thus, working with a Cantor set of larger density may simplify some of our arguments (in the proof of Proposition 3.2, for example).
Finally, the results of this paper probably extend to more general families of smooth unimodal maps. In the present ‘proof of concept’ work, we choose to restrict to the quadratic family.
1.3 Structure of the text
Schnellmann pointed out [Reference SchnellmannSch, p. 370] that the ‘Markov partitions’ given by the intervals in the celebrated Benedicks–Carleson [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2] parameter exclusion construction would be the key to extend his result to non-uniformly expanding interval maps.
Our paper carries out this plan and is organized as follows. After recalling basic facts in §2.1, we adapt in §2.2 the Benedicks–Carleson procedure to construct, in a neighbourhood of a topologically mixing Misiurewicz point
![]() $a_{*}$
, a sequence
$a_{*}$
, a sequence
![]() $\Omega _n\subset \Omega _{n-1}$
where
$\Omega _n\subset \Omega _{n-1}$
where
![]() $\Omega _n$
is a finite union of intervals in
$\Omega _n$
is a finite union of intervals in
![]() $\mathcal {P}_n$
. At each step, some intervals in
$\mathcal {P}_n$
. At each step, some intervals in
![]() $\mathcal {P}_n$
are partitioned and the intervals which do not satisfy a time-n polynomial recurrence assumption are excluded. The remaining Cantor set
$\mathcal {P}_n$
are partitioned and the intervals which do not satisfy a time-n polynomial recurrence assumption are excluded. The remaining Cantor set
![]() $\Omega _{*}(a_{*})=\bigcap _n \Omega _n$
is a positive Lebesgue measure set of parameters satisfying the Collet–Eckmann property, polynomial returns and distortion control, with uniform constants. (Our distortion bound in equation (2.31) is new.) In addition, the construction ensures that there are no ‘exceptionally small’ sets (Lemma 2.3). Applying results from [Reference Baladi, Benedicks and SchnellmannBBS], this ensures uniform exponential decay of correlations (Proposition 2.5) and fractional response (Proposition 2.6), from which we obtain regularity of the map
$\Omega _{*}(a_{*})=\bigcap _n \Omega _n$
is a positive Lebesgue measure set of parameters satisfying the Collet–Eckmann property, polynomial returns and distortion control, with uniform constants. (Our distortion bound in equation (2.31) is new.) In addition, the construction ensures that there are no ‘exceptionally small’ sets (Lemma 2.3). Applying results from [Reference Baladi, Benedicks and SchnellmannBBS], this ensures uniform exponential decay of correlations (Proposition 2.5) and fractional response (Proposition 2.6), from which we obtain regularity of the map
![]() $a\mapsto \sigma _a$
(Lemma 2.8).
$a\mapsto \sigma _a$
(Lemma 2.8).
Sections 3 and 4 contain the proof of the ASIP along the lines of [Reference SchnellmannSch]: first approximate the Birkhoff sum by a sum of blocks of polynomial size (§§4.1 and 4.2), then (§4.3) approximate these blocks by a martingale difference sequence
![]() $Y_j$
and apply Skorokhod’s representation theorem linking a martingale with a Brownian motion (see [Reference Philipp and StoutPS, §3]). The usual application of the approach of [Reference Philipp and StoutPS, Ch. 7] in dynamics uses a strong independence condition (see [Reference Philipp and StoutPS, 7.1.2]) which we do not have (the
$Y_j$
and apply Skorokhod’s representation theorem linking a martingale with a Brownian motion (see [Reference Philipp and StoutPS, §3]). The usual application of the approach of [Reference Philipp and StoutPS, Ch. 7] in dynamics uses a strong independence condition (see [Reference Philipp and StoutPS, 7.1.2]) which we do not have (the
![]() $\xi _i$
terms are not iterations of a fixed map and there is no underlying invariant measure). (See the example (see [Reference KacKa, p. 646]) discussed in [Reference SchnellmannSch]. Also, as pointed out in [Reference SchnellmannSch], it is not clear how to apply the spectral techniques of [Reference GouëzelGo] in our setting.) We replace this strong independence condition by uniformity of constants in the exponential decay of correlations (given by [Reference Baladi, Benedicks and SchnellmannBBS]) which we translate into properties for the
$\xi _i$
terms are not iterations of a fixed map and there is no underlying invariant measure). (See the example (see [Reference KacKa, p. 646]) discussed in [Reference SchnellmannSch]. Also, as pointed out in [Reference SchnellmannSch], it is not clear how to apply the spectral techniques of [Reference GouëzelGo] in our setting.) We replace this strong independence condition by uniformity of constants in the exponential decay of correlations (given by [Reference Baladi, Benedicks and SchnellmannBBS]) which we translate into properties for the
![]() $\xi _i$
by switching from parameter to phase space (see Proposition 3.2), giving estimates similar to those in [Reference Philipp and StoutPS, §3].
$\xi _i$
by switching from parameter to phase space (see Proposition 3.2), giving estimates similar to those in [Reference Philipp and StoutPS, §3].
For
![]() $\varpi \in (0,1)$
, we shall denote by
$\varpi \in (0,1)$
, we shall denote by
![]() $C^\varpi $
the set of
$C^\varpi $
the set of
![]() $\varpi $
-Hölder continuous functions
$\varpi $
-Hölder continuous functions
![]() $\varphi \colon I\to {\mathbb R}$
, putting
$\varphi \colon I\to {\mathbb R}$
, putting
![]() $\|\varphi \|_\varpi =\sup |\varphi |+H_\varpi (\varphi )$
, with
$\|\varphi \|_\varpi =\sup |\varphi |+H_\varpi (\varphi )$
, with
![]() $H_\varpi (\varphi )$
the smallest
$H_\varpi (\varphi )$
the smallest
![]() $H_\varpi $
such that
$H_\varpi $
such that
![]() $|\varphi (x)-\varphi (y)|\le H_\varpi |x-y|^\varpi $
for all x, y in I. The letter C is used throughout to represent a (large) uniform constant, which may vary from place to place.
$|\varphi (x)-\varphi (y)|\le H_\varpi |x-y|^\varpi $
for all x, y in I. The letter C is used throughout to represent a (large) uniform constant, which may vary from place to place.
2 Bounds for the quadratic family. The Cantor set
 $\Omega _{*}(a_{*})$
$\Omega _{*}(a_{*})$
2.1 Basic properties
Clearly, the maps
are Lipschitz continuous uniformly in
![]() $x\in I$
and
$x\in I$
and
![]() $a\in (a_{\mathrm {mix}},4]$
, and, in addition,
$a\in (a_{\mathrm {mix}},4]$
, and, in addition,
Each
![]() $T_a$
has two monotonicity intervals, with partition points
$T_a$
has two monotonicity intervals, with partition points
![]() $0$
,
$0$
,
![]() $c=1/2$
and
$c=1/2$
and
![]() $1$
. The following easy lemma replaces [Reference SchnellmannSch], (30)]. (We do not need as in [Reference SchnellmannSch, (30)] that x has the same combinatorics under
$1$
. The following easy lemma replaces [Reference SchnellmannSch], (30)]. (We do not need as in [Reference SchnellmannSch, (30)] that x has the same combinatorics under
![]() $T_{a_1}$
and
$T_{a_1}$
and
![]() $T_{a_2}$
up to the
$T_{a_2}$
up to the
![]() $(n-1)$
th iteration. We thus do not need any analogue of [Reference SchnellmannSch, Sublemma 5.4].)
$(n-1)$
th iteration. We thus do not need any analogue of [Reference SchnellmannSch, Sublemma 5.4].)
Lemma 2.1. There exists
![]() $C<\infty $
such that, for any
$C<\infty $
such that, for any
![]() $a_1,a_2\in (2,4]$
, we have
$a_1,a_2\in (2,4]$
, we have
Proof. Clearly,
![]() $|T_{a_1}(x)-T_{a_2}(x)|\le |a_1-a_2|$
. For
$|T_{a_1}(x)-T_{a_2}(x)|\le |a_1-a_2|$
. For
![]() $n\ge 2$
, using the definition in equation (2.1) of
$n\ge 2$
, using the definition in equation (2.1) of
![]() $\Lambda $
and setting
$\Lambda $
and setting
![]() $C=\sum _{j=0}^\infty \Lambda ^{-j}$
, we get
$C=\sum _{j=0}^\infty \Lambda ^{-j}$
, we get
 $$ \begin{align*} |T_{a_1}^n(x)-T_{a_2}^n(x)| &\leq |T_{a_1} (T_{a_1}^{n-1} (x)) - T_{a_2} (T_{a_1}^{n-1} (x))| +|T_{a_2} (T_{a_1}^{n-1} (x)) - T_{a_2} (T_{a_2}^{n-1} (x))| \\ &\leq |a_1-a_2| + \Lambda |T_{a_1}^{n-1} (x) - T_{a_2}^{n-1} (x)|\\ &\leq |a_1-a_2| (1 + \Lambda) + \Lambda^2 |T_{a_1}^{n-2} (x) - T_{a_2}^{n-2} (x)| \leq \cdots \\ & \leq |a_1-a_2| \sum_{j=0}^{n-1} \Lambda^j \leq C \Lambda^n |a_1-a_2|.\\[-41pt] \end{align*} $$
$$ \begin{align*} |T_{a_1}^n(x)-T_{a_2}^n(x)| &\leq |T_{a_1} (T_{a_1}^{n-1} (x)) - T_{a_2} (T_{a_1}^{n-1} (x))| +|T_{a_2} (T_{a_1}^{n-1} (x)) - T_{a_2} (T_{a_2}^{n-1} (x))| \\ &\leq |a_1-a_2| + \Lambda |T_{a_1}^{n-1} (x) - T_{a_2}^{n-1} (x)|\\ &\leq |a_1-a_2| (1 + \Lambda) + \Lambda^2 |T_{a_1}^{n-2} (x) - T_{a_2}^{n-2} (x)| \leq \cdots \\ & \leq |a_1-a_2| \sum_{j=0}^{n-1} \Lambda^j \leq C \Lambda^n |a_1-a_2|.\\[-41pt] \end{align*} $$
2.2 A polynomial Benedicks–Carleson construction (
 $\Omega _{*}(a_{*})$
,
$\Omega _{*}(a_{*})$
,
 $\mathcal {P}_n$
)
$\mathcal {P}_n$
)
For each
![]() $j\ge 0$
, the function
$j\ge 0$
, the function
![]() $x_j(a)=T_a^{j+1}(c)$
is a map from the parameter space
$x_j(a)=T_a^{j+1}(c)$
is a map from the parameter space
![]() $(a_{\mathrm {mix}},4]$
to the phase space
$(a_{\mathrm {mix}},4]$
to the phase space
![]() $I=[0,1]$
, with
$I=[0,1]$
, with
![]() $x_j(a)\in K(a)$
for all a. The transversality condition in equation (1.4) says that the derivatives of
$x_j(a)\in K(a)$
for all a. The transversality condition in equation (1.4) says that the derivatives of
![]() $x_j$
and
$x_j$
and
![]() $T_a^j$
are comparable at
$T_a^j$
are comparable at
![]() $a_{*}$
, so that statistical properties (such as the ASIP) can be transferred from the maps
$a_{*}$
, so that statistical properties (such as the ASIP) can be transferred from the maps
![]() $x\mapsto T_a^j(x)$
to the maps
$x\mapsto T_a^j(x)$
to the maps
![]() $a\mapsto x_j(a)$
. To make this precise, we next construct a sequence of partitions in the parameter space. Our starting point is the following variant of the Benedicks–Carleson Cantor set
$a\mapsto x_j(a)$
. To make this precise, we next construct a sequence of partitions in the parameter space. Our starting point is the following variant of the Benedicks–Carleson Cantor set
![]() $\Omega _\mathrm{BC}=\Omega _\mathrm{BC}(a_{*})$
(see [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2]) associated to a Misiurewicz parameter
$\Omega _\mathrm{BC}=\Omega _\mathrm{BC}(a_{*})$
(see [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2]) associated to a Misiurewicz parameter
![]() $a_{*}$
(which is automatically transversal). (See equation (2.17) for the construction of
$a_{*}$
(which is automatically transversal). (See equation (2.17) for the construction of
![]() $\Omega _\mathrm{BC}$
.)
$\Omega _\mathrm{BC}$
.)
Proposition 2.2. (The Cantor set
 $\Omega _{*}=\Omega _{*}(a_{*},\kappa _0)$
)
$\Omega _{*}=\Omega _{*}(a_{*},\kappa _0)$
)
Let
![]() $a_{*}\in (a_{\mathrm {mix}},4]$
be a Misiurewicz parameter. There exist
$a_{*}\in (a_{\mathrm {mix}},4]$
be a Misiurewicz parameter. There exist
![]() $\unicode{x3bb} _\mathrm{CE}\in (1, \Lambda )$
and
$\unicode{x3bb} _\mathrm{CE}\in (1, \Lambda )$
and
![]() $C_0\in (0,1)$
such that, for any
$C_0\in (0,1)$
such that, for any
![]() $d_1\in (0,C_0\log \unicode{x3bb} _\mathrm{CE}/4)$
and
$d_1\in (0,C_0\log \unicode{x3bb} _\mathrm{CE}/4)$
and
![]() $d_0>0$
, there exists
$d_0>0$
, there exists
![]() $\epsilon>0$
such that for any
$\epsilon>0$
such that for any
![]() $\kappa _0>1/d_1$
, for all large enough
$\kappa _0>1/d_1$
, for all large enough
![]() $N_0\ge 1$
, there exists a sequence
$N_0\ge 1$
, there exists a sequence
![]() $\mathcal {P}_j$
of finite sets of pairwise disjoint subintervals of
$\mathcal {P}_j$
of finite sets of pairwise disjoint subintervals of
such that
![]() $\mathcal {P}_1=\mathcal {P}_2=\cdots =\mathcal {P}_{N_0}$
and, setting
$\mathcal {P}_1=\mathcal {P}_2=\cdots =\mathcal {P}_{N_0}$
and, setting
 $$ \begin{align*} \Omega_{*} = \Omega_{*}(a_{*},\kappa_0):=\bigcap_{j \geq N_0} \Omega_j \quad \text{with }\, \Omega_j:=\bigcup_{ \omega \in \mathcal{P}_j} \omega, \end{align*} $$
$$ \begin{align*} \Omega_{*} = \Omega_{*}(a_{*},\kappa_0):=\bigcap_{j \geq N_0} \Omega_j \quad \text{with }\, \Omega_j:=\bigcup_{ \omega \in \mathcal{P}_j} \omega, \end{align*} $$
we have
![]() $\Omega _{j+1}\subset \Omega _j$
for
$\Omega _{j+1}\subset \Omega _j$
for
![]() $j\ge N_0$
, and (the first bound of equation (2.4) implies that
$j\ge N_0$
, and (the first bound of equation (2.4) implies that
![]() $a\mapsto x_j(a)=T_a^{j+1}(c)$
is monotone on
$a\mapsto x_j(a)=T_a^{j+1}(c)$
is monotone on
![]() $\omega \in \mathcal {P}_j$
)
$\omega \in \mathcal {P}_j$
)
and there exists (note that equation (2.6) replaces [Reference SchnellmannSch, Lemma 2.4])
![]() $C<\infty $
such that for all
$C<\infty $
such that for all
![]() $j\geq N_0$
and
$j\geq N_0$
and
![]() $\omega \in \mathcal {P}_j$
,
$\omega \in \mathcal {P}_j$
,
 $$ \begin{align} &\frac{1}{C}\leq \bigg|\frac{x_n^{\prime}(a)}{(T_a^{n})^{\prime}(T_a(c))} \bigg| \leq C\quad \text{for all } N_0 \leq n \leq j, \text{ for all } a \in \omega, \end{align} $$
$$ \begin{align} &\frac{1}{C}\leq \bigg|\frac{x_n^{\prime}(a)}{(T_a^{n})^{\prime}(T_a(c))} \bigg| \leq C\quad \text{for all } N_0 \leq n \leq j, \text{ for all } a \in \omega, \end{align} $$
and, moreover,
Finally, we have that
![]() $a_{*}\in \Omega _{*}$
is a Lebesgue density point of
$a_{*}\in \Omega _{*}$
is a Lebesgue density point of
![]() $\Omega _{*}$
, with
$\Omega _{*}$
, with
 $$ \begin{align} |\Omega_{*}|\ge (1-d_0 \cdot e_j)|\Omega_{j-1}| \quad \text{for all } j \geq N_0, \text{ where } e_j := \sum_{n = j}^\infty n^{-d_1 \cdot \kappa_0} , \end{align} $$
$$ \begin{align} |\Omega_{*}|\ge (1-d_0 \cdot e_j)|\Omega_{j-1}| \quad \text{for all } j \geq N_0, \text{ where } e_j := \sum_{n = j}^\infty n^{-d_1 \cdot \kappa_0} , \end{align} $$
and we have the more precise (semi-local) bound
 $$ \begin{align} \sum_{\substack{\omega\in \mathcal{P}_{\ell}\\ \omega\subset \omega^{\prime}}} |\omega \setminus (\omega\cap \Omega_{*})| \le d_0 \cdot e_{\ell-\ell^{\prime}} | \omega^{\prime}| \quad \text{for all } \omega^{\prime} \in\mathcal{P}_{\ell^{\prime}}, \text{ for all } \ell \ge \ell^{\prime}\ge N_0. \end{align} $$
$$ \begin{align} \sum_{\substack{\omega\in \mathcal{P}_{\ell}\\ \omega\subset \omega^{\prime}}} |\omega \setminus (\omega\cap \Omega_{*})| \le d_0 \cdot e_{\ell-\ell^{\prime}} | \omega^{\prime}| \quad \text{for all } \omega^{\prime} \in\mathcal{P}_{\ell^{\prime}}, \text{ for all } \ell \ge \ell^{\prime}\ge N_0. \end{align} $$
See Lemma 2.3 below regarding the absence of exceptionally small sets and §2.3 for a Hölder distortion property refining equation (2.16).
Clearly, equation (2.8) means that any
![]() $a\in \Omega _{*}$
is
$a\in \Omega _{*}$
is
![]() $(N_0,\kappa _0)$
-polynomially recurrent.
$(N_0,\kappa _0)$
-polynomially recurrent.
The bound in equation (2.9) implies that the Cantor set
![]() $\Omega _{*}$
has positive Lebesgue measure as soon as
$\Omega _{*}$
has positive Lebesgue measure as soon as
![]() $d_1\cdot \kappa _0>1$
(and
$d_1\cdot \kappa _0>1$
(and
![]() $N_0$
is large enough). Proposition 2.2 holds for such
$N_0$
is large enough). Proposition 2.2 holds for such
![]() $\kappa _0$
, but we will need the stronger condition
$\kappa _0$
, but we will need the stronger condition
![]() $d_1 \cdot \kappa _0 \geq 11/3$
to use equation (2.9) in the proof of Proposition 3.2 (and
$d_1 \cdot \kappa _0 \geq 11/3$
to use equation (2.9) in the proof of Proposition 3.2 (and
![]() $d_1\cdot \kappa _0>9/5$
for Lemma 4.2).
$d_1\cdot \kappa _0>9/5$
for Lemma 4.2).
The local bound in equation (2.10) is used in the proof of Lemma 4.1.
Proof of Proposition 2.2
Let
![]() $r_0 \ge 2$
be a large integer (to be chosen later, with
$r_0 \ge 2$
be a large integer (to be chosen later, with
![]() $\epsilon \to 0$
as
$\epsilon \to 0$
as
![]() $r_0$
increases). For
$r_0$
increases). For
![]() $r\ge r_0$
, set
$r\ge r_0$
, set
![]() $I_r=I_r^{-}\cup I_r^{+}$
, where
$I_r=I_r^{-}\cup I_r^{+}$
, where
and cover each
![]() $I_r^{\pm }$
by
$I_r^{\pm }$
by
![]() $r^2$
pairwise disjoint intervals
$r^2$
pairwise disjoint intervals
![]() $I_{r,\ell }^{\pm }$
of equal size, each
$I_{r,\ell }^{\pm }$
of equal size, each
![]() $I_{r,\ell }^{\pm }$
containing its boundary point closest to c. Let
$I_{r,\ell }^{\pm }$
containing its boundary point closest to c. Let
![]() $\beta _\mathrm{BC}> \alpha _\mathrm{BC} > 0$
, where
$\beta _\mathrm{BC}> \alpha _\mathrm{BC} > 0$
, where
for
![]() $N_{0}$
a large integer to be chosen later. (The constant
$N_{0}$
a large integer to be chosen later. (The constant
![]() $\alpha _\mathrm{BC}$
is usually called
$\alpha _\mathrm{BC}$
is usually called
![]() $\alpha $
, but we shall need the letter
$\alpha $
, but we shall need the letter
![]() $\alpha $
for another purpose in equation (2.30).)
$\alpha $
for another purpose in equation (2.30).)
For
![]() $a\in (a_{\mathrm {mix}},4]$
,
$a\in (a_{\mathrm {mix}},4]$
,
![]() $\nu \ge 1$
, and
$\nu \ge 1$
, and
![]() $r\ge r_0$
such that
$r\ge r_0$
such that
![]() $T^\nu _a(c)\in I_r$
, the binding time
$T^\nu _a(c)\in I_r$
, the binding time
![]() $p(a)=p(r,a, \nu )$
of
$p(a)=p(r,a, \nu )$
of
![]() $U_r$
with
$U_r$
with
![]() $T^\nu _a(c)$
is the maximal
$T^\nu _a(c)$
is the maximal
![]() $p\in {\mathbb Z}_+ \cup \{\infty \}$
such that
$p\in {\mathbb Z}_+ \cup \{\infty \}$
such that
The first free return time
![]() $\nu _1(a)$
of
$\nu _1(a)$
of
![]() $a\in (a_{\mathrm {mix}},4]$
is the smallest integer
$a\in (a_{\mathrm {mix}},4]$
is the smallest integer
![]() $j \geq 1$
for which
$j \geq 1$
for which
![]() $T_a^j(c) \in U_{r_0}$
. For an interval
$T_a^j(c) \in U_{r_0}$
. For an interval
![]() $\omega \subset (a_{\mathrm {mix}},4]$
, the first free return time
$\omega \subset (a_{\mathrm {mix}},4]$
, the first free return time
![]() $\nu _1(\omega )$
is the smallest integer
$\nu _1(\omega )$
is the smallest integer
![]() $j \geq 1$
for which there exists
$j \geq 1$
for which there exists
![]() $a\in \omega $
with
$a\in \omega $
with
![]() $T_a^j(c) \in U_{r_0}$
. If there exists
$T_a^j(c) \in U_{r_0}$
. If there exists
![]() $r=r(\omega )$
such that
$r=r(\omega )$
such that
![]() $x_{\nu _1-1}(\omega )\subset I_r$
(recall that
$x_{\nu _1-1}(\omega )\subset I_r$
(recall that
![]() $T^{\nu _1}_a(c)=x_{\nu _1-1}(a)$
), we define the first binding time of
$T^{\nu _1}_a(c)=x_{\nu _1-1}(a)$
), we define the first binding time of
![]() $\omega $
by
$\omega $
by
![]() $p_1(\omega ) = \operatorname \min _{a \in \omega }p(r,a,\nu _1(\omega ))$
. For
$p_1(\omega ) = \operatorname \min _{a \in \omega }p(r,a,\nu _1(\omega ))$
. For
![]() $i\ge 2$
, define inductively the ith free return time of (suitable)
$i\ge 2$
, define inductively the ith free return time of (suitable)
![]() $\omega $
to be the largest integer
$\omega $
to be the largest integer
![]() $\nu _{i}(\omega )>\nu _{i-1}(\omega )+p_{i-1}(\omega )+1$
such that
$\nu _{i}(\omega )>\nu _{i-1}(\omega )+p_{i-1}(\omega )+1$
such that
and, for
![]() $r(\omega )$
such that
$r(\omega )$
such that
![]() $x_{\nu _{i-1}-1}(\omega )\subset I_r$
, set the ith binding time of
$x_{\nu _{i-1}-1}(\omega )\subset I_r$
, set the ith binding time of
![]() $\omega $
to be
$\omega $
to be
(Similarly, define inductively for
![]() $i\ge 2$
and a such that
$i\ge 2$
and a such that
![]() $ T^{\nu _{i-1}}_{a}(c)\in I_r$
, the pointwise binding times
$ T^{\nu _{i-1}}_{a}(c)\in I_r$
, the pointwise binding times
![]() $p_i(a)$
and free returns
$p_i(a)$
and free returns
![]() $\nu _i( a)$
.) The iterates between
$\nu _i( a)$
.) The iterates between
![]() $\nu _i(\omega )$
and
$\nu _i(\omega )$
and
![]() $\nu _i(\omega )+p_i(\omega )$
form the ith bound period of
$\nu _i(\omega )+p_i(\omega )$
form the ith bound period of
![]() $\omega $
, those between
$\omega $
, those between
![]() $\nu _{i-1}(\omega )+p_{i-1}(\omega )+1$
and
$\nu _{i-1}(\omega )+p_{i-1}(\omega )+1$
and
![]() $\nu _{i}(\omega )-1$
form its ith free period. Finally, if there exist
$\nu _{i}(\omega )-1$
form its ith free period. Finally, if there exist
![]() $a\in \omega $
and
$a\in \omega $
and
![]() $j\ge \nu _1(\omega )$
such that
$j\ge \nu _1(\omega )$
such that
![]() $T_a^j(c)\in U_{r_0}$
, we say that j is a return time of
$T_a^j(c)\in U_{r_0}$
, we say that j is a return time of
![]() $\omega $
. (Return times either are free returns
$\omega $
. (Return times either are free returns
![]() $\nu _i(\omega )$
or they occur during the bound period.)
$\nu _i(\omega )$
or they occur during the bound period.)
Note that for any fixed
![]() $\epsilon $
, setting
$\epsilon $
, setting
![]() $\omega _0=[a_{*}-\epsilon ,a_{*}+\epsilon ]$
, there exists
$\omega _0=[a_{*}-\epsilon ,a_{*}+\epsilon ]$
, there exists
![]() $N_\epsilon $
such that
$N_\epsilon $
such that
![]() $x_{N_\epsilon }(\omega _0)$
contains a neighbourhood of c (indeed, by transversality, for any
$x_{N_\epsilon }(\omega _0)$
contains a neighbourhood of c (indeed, by transversality, for any
![]() $a\in \omega _0\setminus \{a_{*}\}$
, there exists
$a\in \omega _0\setminus \{a_{*}\}$
, there exists
![]() $N(a)$
such that
$N(a)$
such that
![]() $T_{a_{*}}^{N(a)+1}(c)$
and
$T_{a_{*}}^{N(a)+1}(c)$
and
![]() $T_{a}^{N(a)+1}(c)$
lie on different sides of c). In particular,
$T_{a}^{N(a)+1}(c)$
lie on different sides of c). In particular,
![]() $\nu _1(\omega _0)<\infty $
. Similarly, all
$\nu _1(\omega _0)<\infty $
. Similarly, all
![]() $\nu _i(\omega _0)$
and
$\nu _i(\omega _0)$
and
![]() $p_i(\omega _0)$
are finite.
$p_i(\omega _0)$
are finite.
Let
![]() $W_{a_{*}}$
be a neighbourhood of c disjoint from
$W_{a_{*}}$
be a neighbourhood of c disjoint from
![]() $\{T^n_{a_{*}}(c)\mid n\ge 1\}$
. From now on, we only consider
$\{T^n_{a_{*}}(c)\mid n\ge 1\}$
. From now on, we only consider
![]() $r_0$
large enough such that
$r_0$
large enough such that
![]() $\overline U_{r_0-1}\subset W_{a_{*}}$
. Set
$\overline U_{r_0-1}\subset W_{a_{*}}$
. Set
![]() $W_{a_{*},r_0}^{+}=W_{a_{*}}\cap [c+e^{-r_0},1]$
and
$W_{a_{*},r_0}^{+}=W_{a_{*}}\cap [c+e^{-r_0},1]$
and
![]() $W_{a_{*},r_0}^{-}=W_{a_{*}}\cap [0,c-e^{-r_0}]$
. We claim that for any fixed large
$W_{a_{*},r_0}^{-}=W_{a_{*}}\cap [0,c-e^{-r_0}]$
. We claim that for any fixed large
![]() $r_0$
, we have that
$r_0$
, we have that
![]() $x_{\nu _1(\omega _0)-1}(\omega _0)$
contains
$x_{\nu _1(\omega _0)-1}(\omega _0)$
contains
![]() $W_{a_{*},r_0}^{+}$
or
$W_{a_{*},r_0}^{+}$
or
![]() $W_{a_{*},r_0}^{-}$
for all small enough
$W_{a_{*},r_0}^{-}$
for all small enough
![]() $\epsilon $
. (This fact is used in [Reference de Melo and van StrienDMS, Lemma V.6.8]. There,
$\epsilon $
. (This fact is used in [Reference de Melo and van StrienDMS, Lemma V.6.8]. There,
![]() $W_{a_{*}}$
is mistakenly mentioned instead of
$W_{a_{*}}$
is mistakenly mentioned instead of
![]() $W^{\pm }_{a_{*},r_0}$
. Our
$W^{\pm }_{a_{*},r_0}$
. Our
![]() $r_0$
is denoted by
$r_0$
is denoted by
![]() $\Delta $
and our
$\Delta $
and our
![]() $x_n(a)$
is denoted
$x_n(a)$
is denoted
![]() $\xi _{n+1}(a)$
in [Reference de Melo and van StrienDMS].) Indeed,
$\xi _{n+1}(a)$
in [Reference de Melo and van StrienDMS].) Indeed,
![]() $x_{\nu _1(\omega _0)-1}(\omega _0)$
is an interval intersecting
$x_{\nu _1(\omega _0)-1}(\omega _0)$
is an interval intersecting
![]() $U_{r_0}$
, and
$U_{r_0}$
, and
![]() $x_{\nu _1(\omega _0)-1}(\omega _0)$
contains
$x_{\nu _1(\omega _0)-1}(\omega _0)$
contains
![]() $T_{a_{*}}^{\nu _1(\omega )}(c)\notin W_{a_{*}}$
.
$T_{a_{*}}^{\nu _1(\omega )}(c)\notin W_{a_{*}}$
.
For small
![]() $\epsilon>0$
(to be chosen depending on
$\epsilon>0$
(to be chosen depending on
![]() $r_0$
), the sequence
$r_0$
), the sequence
![]() $ \mathcal {P}_j$
can now be defined inductively: start with the single interval
$ \mathcal {P}_j$
can now be defined inductively: start with the single interval
![]() $ \mathcal {P}_0= \mathcal {P}_1=\cdots = \mathcal {P}_{N_0} =\{\omega _0 \}$
for
$ \mathcal {P}_0= \mathcal {P}_1=\cdots = \mathcal {P}_{N_0} =\{\omega _0 \}$
for
![]() $\epsilon $
small enough such that
$\epsilon $
small enough such that
![]() $\nu _1( \omega _0)\ge N_0$
(note that
$\nu _1( \omega _0)\ge N_0$
(note that
![]() $\nu _1(\omega _0)$
increases if
$\nu _1(\omega _0)$
increases if
![]() $r_0$
increases or
$r_0$
increases or
![]() $\epsilon $
decreases). (We refer throughout to [Reference de Melo and van StrienDMS, §V.6]. The original ideas and key estimates appeared previously in the work of Benedicks and Carleson [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2]. The original construction in [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2] is for
$\epsilon $
decreases). (We refer throughout to [Reference de Melo and van StrienDMS, §V.6]. The original ideas and key estimates appeared previously in the work of Benedicks and Carleson [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2]. The original construction in [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2] is for
![]() $a_{*}=4$
, see [Reference MoreiraMo] for a self-contained account. It extends to Misiurewicz parameters: for CE parameters, the condition in [Reference de Melo and van StrienDMS, Theorem 6.1] is equivalent to equation (1.4), taking large enough k in the last line of [Reference de Melo and van StrienDMS, p. 406, Step 2].)
$a_{*}=4$
, see [Reference MoreiraMo] for a self-contained account. It extends to Misiurewicz parameters: for CE parameters, the condition in [Reference de Melo and van StrienDMS, Theorem 6.1] is equivalent to equation (1.4), taking large enough k in the last line of [Reference de Melo and van StrienDMS, p. 406, Step 2].)
For
![]() $j> N_0$
, each
$j> N_0$
, each
![]() $ \omega \in \mathcal {P}_{j-1}$
is partitioned into finitely many (possibly just one) intervals, at least one of which will be included into an auxiliary partition
$ \omega \in \mathcal {P}_{j-1}$
is partitioned into finitely many (possibly just one) intervals, at least one of which will be included into an auxiliary partition
![]() $\mathcal {P}^{\prime }_{j}$
, as follows.
$\mathcal {P}^{\prime }_{j}$
, as follows.
If j is not a free return time of
![]() $ \omega $
, we include
$ \omega $
, we include
![]() $\omega $
in
$\omega $
in
![]() $\mathcal {P}^{\prime }_{j}$
. (That is, either j is not a return, or it is a return within the bound period.) If j is a free return time of
$\mathcal {P}^{\prime }_{j}$
. (That is, either j is not a return, or it is a return within the bound period.) If j is a free return time of
![]() $\omega $
but
$\omega $
but
![]() $x_{j-1}( \omega )$
does not contain an interval
$x_{j-1}( \omega )$
does not contain an interval
![]() $I^{\pm }_{r,\ell }$
(we call this an inessential (free) return), we also include
$I^{\pm }_{r,\ell }$
(we call this an inessential (free) return), we also include
![]() $\omega $
in
$\omega $
in
![]() $\mathcal {P}^{\prime }_{j}$
.
$\mathcal {P}^{\prime }_{j}$
.
Otherwise, j is a free return time of
![]() $ \omega $
such that
$ \omega $
such that
![]() $x_{j-1}( \omega )$
contains at least one interval
$x_{j-1}( \omega )$
contains at least one interval
![]() $I_{r,\ell }^{\pm }$
. We call this an essential (free) return. In that case, we decompose
$I_{r,\ell }^{\pm }$
. We call this an essential (free) return. In that case, we decompose
![]() $x_{j-1}( \omega )$
into the following intervals:
$x_{j-1}( \omega )$
into the following intervals:
If
![]() $x_{j-1}( \omega )\setminus U_{r_0}\ne \emptyset $
, but any of the (at most two) connected components of
$x_{j-1}( \omega )\setminus U_{r_0}\ne \emptyset $
, but any of the (at most two) connected components of
![]() $x_{j-1}( \omega )\setminus U_{r_0}$
has size less than
$x_{j-1}( \omega )\setminus U_{r_0}$
has size less than
![]() $e^{-r_0}(1-1/e) r_0^{-2}=|I^{\pm }_{r_0,\ell }|$
, we join it to its neighbour
$e^{-r_0}(1-1/e) r_0^{-2}=|I^{\pm }_{r_0,\ell }|$
, we join it to its neighbour
![]() $x_{j-1}(\omega )\cap I^{\pm }_{r_0,\ell }=I^{\pm }_{r_0,\ell }$
. If a connected component of
$x_{j-1}(\omega )\cap I^{\pm }_{r_0,\ell }=I^{\pm }_{r_0,\ell }$
. If a connected component of
![]() $x_{j-1}( \omega )\setminus U_{r_0}$
has size larger than
$x_{j-1}( \omega )\setminus U_{r_0}$
has size larger than
![]() $S:=\sqrt {|U_{r_0}|}$
, we subdivide it into pairwise disjoint intervals of lengths between
$S:=\sqrt {|U_{r_0}|}$
, we subdivide it into pairwise disjoint intervals of lengths between
![]() $S/2$
and S. If
$S/2$
and S. If
![]() $x_{j-1}(\omega )\cap I_{r,\ell }^{\pm }\ne \emptyset $
, but
$x_{j-1}(\omega )\cap I_{r,\ell }^{\pm }\ne \emptyset $
, but
![]() $I_{r,\ell }^{\pm }$
is not contained in
$I_{r,\ell }^{\pm }$
is not contained in
![]() $x_{j-1}(\omega )$
(this can happen for at most two intervals
$x_{j-1}(\omega )$
(this can happen for at most two intervals
![]() $I_{r,\ell }^{\pm }$
), we join
$I_{r,\ell }^{\pm }$
), we join
![]() $x_{j-1}(\omega )\cap I_{r,\ell }^{\pm }$
to its neighbour
$x_{j-1}(\omega )\cap I_{r,\ell }^{\pm }$
to its neighbour
![]() $x_{j-1}( \omega )\cap I^{\pm }_{r^{\prime },\ell ^{\prime }}=I^{\pm }_{r^{\prime },\ell ^{\prime }}$
. Denote by
$x_{j-1}( \omega )\cap I^{\pm }_{r^{\prime },\ell ^{\prime }}=I^{\pm }_{r^{\prime },\ell ^{\prime }}$
. Denote by
![]() $\{\hat \omega _{r,\ell }\mid r\ge r_0-1\}$
the partition of
$\{\hat \omega _{r,\ell }\mid r\ge r_0-1\}$
the partition of
![]() $x_{j-1}(\omega )$
thus obtained, where the index
$x_{j-1}(\omega )$
thus obtained, where the index
![]() $(r,\ell )$
refers to the ‘host’ interval
$(r,\ell )$
refers to the ‘host’ interval
![]() $I_{r,\ell }$
contained in
$I_{r,\ell }$
contained in
![]() $\hat \omega _{r,\ell }$
if
$\hat \omega _{r,\ell }$
if
![]() $r\ge r_0$
, while
$r\ge r_0$
, while
![]() $\hat \omega _{r_0-1,\ell }\subset I\setminus U_{r_0}$
. Then we discard all intervals
$\hat \omega _{r_0-1,\ell }\subset I\setminus U_{r_0}$
. Then we discard all intervals
![]() $\hat \omega _{r,\ell }$
for which
$\hat \omega _{r,\ell }$
for which
Mapping the remaining intervals via the inverse of the diffeomorphism (see [Reference de Melo and van StrienDMS, Proposition V.6.2])
![]() $x_{j-1}$
gives finitely many subintervals of
$x_{j-1}$
gives finitely many subintervals of
![]() $\omega $
which we include in
$\omega $
which we include in
![]() $\mathcal {P}^{\prime }_j$
. Further intervals
$\mathcal {P}^{\prime }_j$
. Further intervals
![]() $\hat \omega _{r,\ell }$
need to be discarded from
$\hat \omega _{r,\ell }$
need to be discarded from
![]() $\mathcal {P}^{\prime }_j$
, using a requirement denoted
$\mathcal {P}^{\prime }_j$
, using a requirement denoted
![]() $(FA_{j})$
or
$(FA_{j})$
or
![]() $(FA^{\prime }_j)$
in [Reference de Melo and van StrienDMS, §V.6], [Reference MoreiraMo], which finally defines
$(FA^{\prime }_j)$
in [Reference de Melo and van StrienDMS, §V.6], [Reference MoreiraMo], which finally defines
![]() $\mathcal {P}_j$
. For further use, we denote these remaining intervals by
$\mathcal {P}_j$
. For further use, we denote these remaining intervals by
It is well known (the original construction in [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2] is for
![]() $a_{*}=4$
, see [Reference MoreiraMo] for a self-contained account. It extends to Misiurewicz parameters: for CE parameters, the condition in [Reference de Melo and van StrienDMS, Theorem 6.1] is equivalent to equation (1.4), taking large enough k in the last line of [Reference de Melo and van StrienDMS, p. 406, Step 2]) [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2, Reference de Melo and van StrienDMS] that, if we replace the condition in equation (2.11) (used to discard intervals) by the exponential condition
$a_{*}=4$
, see [Reference MoreiraMo] for a self-contained account. It extends to Misiurewicz parameters: for CE parameters, the condition in [Reference de Melo and van StrienDMS, Theorem 6.1] is equivalent to equation (1.4), taking large enough k in the last line of [Reference de Melo and van StrienDMS, p. 406, Step 2]) [Reference Benedicks and CarlesonBC1, Reference Benedicks and CarlesonBC2, Reference de Melo and van StrienDMS] that, if we replace the condition in equation (2.11) (used to discard intervals) by the exponential condition
to construct sequences
![]() $\mathcal {P}_j^{\prime ,\mathrm{BC}}$
and
$\mathcal {P}_j^{\prime ,\mathrm{BC}}$
and
![]() $\mathcal {P}_j^\mathrm{BC}$
, then there exists
$\mathcal {P}_j^\mathrm{BC}$
, then there exists
![]() $\unicode{x3bb} _\mathrm{CE}>1$
(called
$\unicode{x3bb} _\mathrm{CE}>1$
(called
![]() $e^\gamma $
in [Reference de Melo and van StrienDMS, (V.6.4), Theorem V.6.2]) such that for any small enough
$e^\gamma $
in [Reference de Melo and van StrienDMS, (V.6.4), Theorem V.6.2]) such that for any small enough
![]() $\beta _\mathrm{BC}>\alpha _\mathrm{BC}>0$
, there exist
$\beta _\mathrm{BC}>\alpha _\mathrm{BC}>0$
, there exist
![]() $N_0^{\prime }$
such that if
$N_0^{\prime }$
such that if
![]() $r_0$
is large enough and
$r_0$
is large enough and
![]() $\epsilon>0$
small enough, then the
$\epsilon>0$
small enough, then the
![]() $\mathcal {P}_j^\mathrm{BC}$
satisfy equations (2.3)–(2.7) (equation (2.5) is called
$\mathcal {P}_j^\mathrm{BC}$
satisfy equations (2.3)–(2.7) (equation (2.5) is called
![]() $(EX_j)$
in [Reference de Melo and van StrienDMS, §V.6]) for some
$(EX_j)$
in [Reference de Melo and van StrienDMS, §V.6]) for some
![]() $C<\infty $
, and the following condition (noted
$C<\infty $
, and the following condition (noted
![]() $(BA_j)$
in the literature) holds for all
$(BA_j)$
in the literature) holds for all
![]() $j\ge N_0^{\prime }$
:
$j\ge N_0^{\prime }$
:
(Strictly speaking, the condition
![]() $(BA_j)$
does not involve the factor
$(BA_j)$
does not involve the factor
![]() $2$
, and a condition
$2$
, and a condition
![]() $(BA^{\prime }_j)$
requiring that for each
$(BA^{\prime }_j)$
requiring that for each
![]() $\omega \in \mathcal {P}_j^{\prime \mathrm{BC}}$
, there exists
$\omega \in \mathcal {P}_j^{\prime \mathrm{BC}}$
, there exists
![]() $a\in \omega $
with
$a\in \omega $
with
![]() $|T_a^{n+1}(c)-c|> e^{-n \alpha _\mathrm{BC}}$
for
$|T_a^{n+1}(c)-c|> e^{-n \alpha _\mathrm{BC}}$
for
![]() $N_0^{\prime }\le n\le j$
is used in some lemmas. See [Reference de Melo and van StrienDMS, §V.6, Step 5].) Since
$N_0^{\prime }\le n\le j$
is used in some lemmas. See [Reference de Melo and van StrienDMS, §V.6, Step 5].) Since
![]() $\unicode{x3bb} _\mathrm{CE}$
does not depend on
$\unicode{x3bb} _\mathrm{CE}$
does not depend on
![]() $\alpha _\mathrm{BC}$
,
$\alpha _\mathrm{BC}$
,
![]() $N_0$
or
$N_0$
or
![]() $N_0^{\prime }$
, we may assume that
$N_0^{\prime }$
, we may assume that
and we may replace
![]() $N_0$
by
$N_0$
by
![]() $\operatorname \max \{N_0, N_0^{\prime }\}$
.
$\operatorname \max \{N_0, N_0^{\prime }\}$
.
In particular [Reference de Melo and van StrienDMS, Proposition V.6.1, Lemma V.6.1(b), (c)] give
![]() $\gamma _0>0$
,
$\gamma _0>0$
,
![]() $\unicode{x3bb} _\mathrm{CE}=e^\gamma \in (1, e^{\gamma _0})$
and
$\unicode{x3bb} _\mathrm{CE}=e^\gamma \in (1, e^{\gamma _0})$
and
![]() $C_0>0$
(independent of
$C_0>0$
(independent of
![]() $r_0$
and
$r_0$
and
![]() $\epsilon $
) such that if
$\epsilon $
) such that if
![]() $a\in \Omega _n$
and
$a\in \Omega _n$
and
![]() $\nu _{\ell +1}(a)\le n$
, writing
$\nu _{\ell +1}(a)\le n$
, writing
![]() $p_\ell $
,
$p_\ell $
,
![]() $\nu _\ell $
for
$\nu _\ell $
for
![]() $p_\ell (a)$
,
$p_\ell (a)$
,
![]() $\nu _\ell (a)$
, we have
$\nu _\ell (a)$
, we have

To establish equation (2.5) (the bound below will also be used for equation (2.34)), one takes
![]() $r_0$
such that
$r_0$
such that
The key distortion bound [Reference de Melo and van StrienDMS, Proposition V.6.3] gives C such that
 $$ \begin{align} \bigg| \frac{x_j^{\prime}(a_1)}{x_j^{\prime}(a_2)} \bigg| \leq C \quad \text{for all } N_0\le j \le n , \text{ for all } a_1, a_2\in\omega , \end{align} $$
$$ \begin{align} \bigg| \frac{x_j^{\prime}(a_1)}{x_j^{\prime}(a_2)} \bigg| \leq C \quad \text{for all } N_0\le j \le n , \text{ for all } a_1, a_2\in\omega , \end{align} $$
whenever
![]() $n+1$
is a free return time of
$n+1$
is a free return time of
![]() $\omega \in \mathcal {P}_n$
with
$\omega \in \mathcal {P}_n$
with
![]() $x_{n+1}(\omega )\subset U_{r_0/2}$
. The bound in equation (2.6) follows from [Reference de Melo and van StrienDMS, Proposition V.6.2 and Theorem V.6.2].
$x_{n+1}(\omega )\subset U_{r_0/2}$
. The bound in equation (2.6) follows from [Reference de Melo and van StrienDMS, Proposition V.6.2 and Theorem V.6.2].
Let
![]() $\Omega ^{\prime }_j:=\bigcup _{ \omega \in \mathcal {P}^{\prime }_j} \omega $
, recall
$\Omega ^{\prime }_j:=\bigcup _{ \omega \in \mathcal {P}^{\prime }_j} \omega $
, recall
![]() $\Omega _j$
, and define
$\Omega _j$
, and define
![]() $\Omega _j^\mathrm{BC}$
and
$\Omega _j^\mathrm{BC}$
and
![]() $\Omega _j^{\prime ,\mathrm{BC}}$
accordingly, setting
$\Omega _j^{\prime ,\mathrm{BC}}$
accordingly, setting
 $$ \begin{align} \Omega_\mathrm{BC}=\Omega_\mathrm{BC}(a_{*},\alpha_\mathrm{BC})=\bigcap_j \Omega^\mathrm{BC}_j \quad \mbox{so that } \Omega_{*}(a_{*})\subset\Omega_\mathrm{BC}(a_{*}). \end{align} $$
$$ \begin{align} \Omega_\mathrm{BC}=\Omega_\mathrm{BC}(a_{*},\alpha_\mathrm{BC})=\bigcap_j \Omega^\mathrm{BC}_j \quad \mbox{so that } \Omega_{*}(a_{*})\subset\Omega_\mathrm{BC}(a_{*}). \end{align} $$
It is easy to check that equation (2.11) implies equation (2.8) (for returns during a bound period, use that
![]() $\ell ^{-\kappa _0}-e^{-\ell \beta _\mathrm{BC }}\ge j^{-\kappa _0}$
for all
$\ell ^{-\kappa _0}-e^{-\ell \beta _\mathrm{BC }}\ge j^{-\kappa _0}$
for all
![]() $N_0\le \ell \le j-1$
, up to increasing
$N_0\le \ell \le j-1$
, up to increasing
![]() $N_0$
again). Our choice of
$N_0$
again). Our choice of
![]() $N_0$
implies
$N_0$
implies
![]() $\Omega _j\subset \Omega _j^\mathrm{BC}$
. Also, equation (2.6) with equation (2.4) imply that all points in
$\Omega _j\subset \Omega _j^\mathrm{BC}$
. Also, equation (2.6) with equation (2.4) imply that all points in
![]() $\Omega _{*}$
are transversal. Since equation (2.7) is an immediate consequence of equations (2.5) and (2.6), it only remains to establish that
$\Omega _{*}$
are transversal. Since equation (2.7) is an immediate consequence of equations (2.5) and (2.6), it only remains to establish that
![]() $a_{*}$
is a Lebesgue density point in
$a_{*}$
is a Lebesgue density point in
![]() $\Omega _{*}$
(clearly,
$\Omega _{*}$
(clearly,
![]() $a_{*}\in \Omega _{*}$
) and that equations (2.9) and (2.10) hold.
$a_{*}\in \Omega _{*}$
) and that equations (2.9) and (2.10) hold.
To show that
![]() $a_{*}$
is a Lebesgue density point of
$a_{*}$
is a Lebesgue density point of
![]() $\Omega _{*}$
, we may follow [Reference de Melo and van StrienDMS, Step 7 of the proof of Theorem V.6.1], replacing
$\Omega _{*}$
, we may follow [Reference de Melo and van StrienDMS, Step 7 of the proof of Theorem V.6.1], replacing
![]() $C e^{-i C_0}$
there by
$C e^{-i C_0}$
there by
![]() $C^{\prime } i^{-\kappa _0}$
. (We mention a typo there: although the constant
$C^{\prime } i^{-\kappa _0}$
. (We mention a typo there: although the constant
![]() $C=C(\epsilon )$
in the unnumbered equation on [Reference de Melo and van StrienDMS, p. 433] tends to zero as
$C=C(\epsilon )$
in the unnumbered equation on [Reference de Melo and van StrienDMS, p. 433] tends to zero as
![]() $\epsilon =|\omega _0|/2\to 0$
, the constant
$\epsilon =|\omega _0|/2\to 0$
, the constant
![]() $C_0$
is (fortunately) uniformly bounded away from zero. See the proof of [Reference de Melo and van StrienDMS, Lemma V.6.5].)
$C_0$
is (fortunately) uniformly bounded away from zero. See the proof of [Reference de Melo and van StrienDMS, Lemma V.6.5].)
We next establish equations (2.9) and (2.10). For suitably small
![]() $\bar \eta>0$
, and for
$\bar \eta>0$
, and for
![]() $J_0\ge 1$
such that
$J_0\ge 1$
such that
![]() $\prod _{j=J_0}^\infty (1-e^{-\bar \eta j})>3/4$
(since
$\prod _{j=J_0}^\infty (1-e^{-\bar \eta j})>3/4$
(since
![]() $\bar \eta $
is independent of
$\bar \eta $
is independent of
![]() $\epsilon $
,
$\epsilon $
,
![]() $r_0$
,
$r_0$
,
![]() $N_0$
, we may take
$N_0$
, we may take
![]() $N_0\ge J_0$
), the parameter exclusion rule in equation (2.13) gives
$N_0\ge J_0$
), the parameter exclusion rule in equation (2.13) gives
![]() $d^{\prime }_0>0$
(tending to zero with
$d^{\prime }_0>0$
(tending to zero with
![]() $\epsilon $
) such that ([Reference de Melo and van StrienDMS, §V.6, Step 7], [Reference MoreiraMo, §6])
$\epsilon $
) such that ([Reference de Melo and van StrienDMS, §V.6, Step 7], [Reference MoreiraMo, §6])
 $$ \begin{align} \begin{cases} |\omega \cap \Omega^{\prime,\mathrm{BC}}_j|\ge (1- d^{\prime}_0 e^{-j \bar \eta})|\omega| &\text{for all } \omega \in \mathcal{P}^\mathrm{BC}_{j-1}, \text{ for all } j\ge J_0,\\ |\Omega^\mathrm{BC}_j|\ge |\Omega^{\prime,\mathrm{BC}}_j|-e^{-j\bar \eta}|\omega_0| & \text{for all } j\ge J_0. \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} |\omega \cap \Omega^{\prime,\mathrm{BC}}_j|\ge (1- d^{\prime}_0 e^{-j \bar \eta})|\omega| &\text{for all } \omega \in \mathcal{P}^\mathrm{BC}_{j-1}, \text{ for all } j\ge J_0,\\ |\Omega^\mathrm{BC}_j|\ge |\Omega^{\prime,\mathrm{BC}}_j|-e^{-j\bar \eta}|\omega_0| & \text{for all } j\ge J_0. \end{cases} \end{align} $$
The above implies
![]() $|\Omega ^\mathrm{BC}_j|\ge (1- d^{\prime }_0 e^{-\bar \eta j})|\Omega ^\mathrm{BC}_{j-1}|-e^{-\bar \eta j}|\omega _0|$
for
$|\Omega ^\mathrm{BC}_j|\ge (1- d^{\prime }_0 e^{-\bar \eta j})|\Omega ^\mathrm{BC}_{j-1}|-e^{-\bar \eta j}|\omega _0|$
for
![]() $j\ge J_0$
, and, exploiting that
$j\ge J_0$
, and, exploiting that
![]() $|\omega _0|=|\Omega ^\mathrm{BC}_n|$
for all
$|\omega _0|=|\Omega ^\mathrm{BC}_n|$
for all
![]() $n\le N_0$
with
$n\le N_0$
with
![]() $N_0\ge J_0$
, and using the definition of
$N_0\ge J_0$
, and using the definition of
![]() $J_0$
, also that
$J_0$
, also that
 $$ \begin{align*} |\Omega^\mathrm{BC}_j| \geq \bigg( \prod_{n=J_0}^j (1- d^{\prime}_0 e^{-\bar \eta n}) - \sum_{n=J_0}^j e^{-\tilde \eta n} \bigg ) |\omega_0| \geq \frac 1 2 |\omega_0| \quad \text{for all } j \geq J_0. \end{align*} $$
$$ \begin{align*} |\Omega^\mathrm{BC}_j| \geq \bigg( \prod_{n=J_0}^j (1- d^{\prime}_0 e^{-\bar \eta n}) - \sum_{n=J_0}^j e^{-\tilde \eta n} \bigg ) |\omega_0| \geq \frac 1 2 |\omega_0| \quad \text{for all } j \geq J_0. \end{align*} $$
(By taking larger
![]() $J_0$
, that is, smaller
$J_0$
, that is, smaller
![]() $\epsilon $
, we could replace
$\epsilon $
, we could replace
![]() $1/2$
by a number close to
$1/2$
by a number close to
![]() $1$
.) Thus, applying inductively
$1$
.) Thus, applying inductively
we find
![]() $\tilde \eta>0$
such that for any
$\tilde \eta>0$
such that for any
![]() $j\ge J_0$
,
$j\ge J_0$
,
 $$ \begin{align} |\Omega_\mathrm{BC}|\geq \prod_{n=j}^\infty (1 - ( d^{\prime}_0+2) e^{-\bar \eta n}) |\Omega_{j-1}^\mathrm{BC}| \geq (1 - (\tilde d_0 + 2) e^{-\tilde \eta j} ) |\Omega_{j-1}^\mathrm{BC}|. \end{align} $$
$$ \begin{align} |\Omega_\mathrm{BC}|\geq \prod_{n=j}^\infty (1 - ( d^{\prime}_0+2) e^{-\bar \eta n}) |\Omega_{j-1}^\mathrm{BC}| \geq (1 - (\tilde d_0 + 2) e^{-\tilde \eta j} ) |\Omega_{j-1}^\mathrm{BC}|. \end{align} $$
Recall that we fixed
![]() $d_1 \in (0, {C_0}/{4} \log \unicode{x3bb} _\mathrm{CE})$
(independently of
$d_1 \in (0, {C_0}/{4} \log \unicode{x3bb} _\mathrm{CE})$
(independently of
![]() $\kappa _0$
). Let
$\kappa _0$
). Let
![]() $J_1$
be such that
$J_1$
be such that
![]() $\prod _{j=J_1}^\infty (1-e^{-\bar \eta j}-j^{-2})>3/4$
and return to the sets
$\prod _{j=J_1}^\infty (1-e^{-\bar \eta j}-j^{-2})>3/4$
and return to the sets
![]() $\Omega _j$
,
$\Omega _j$
,
![]() $\Omega _j^{\prime }$
constructed using the (polynomial) exclusion rule in equation (2.11) for
$\Omega _j^{\prime }$
constructed using the (polynomial) exclusion rule in equation (2.11) for
![]() $\kappa _0>1/d_1$
. We claim that for any
$\kappa _0>1/d_1$
. We claim that for any
![]() $d_0>0$
, if
$d_0>0$
, if
![]() $\epsilon $
is small enough,
$\epsilon $
is small enough,
 $$ \begin{align} \begin{cases} |\omega \cap \Omega^{\prime}_j|\ge (1-d_0 \cdot j^{-d_1 \kappa_0}) |\omega| &\text{for all } \omega \in \mathcal{P}_{j-1}, \text{ for all } j\ge J_1,\\ | \Omega_j|\ge |\Omega^{\prime}_j|- e^{-j\bar \eta}|\omega_0| &\text{for all } j\ge J_1. \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} |\omega \cap \Omega^{\prime}_j|\ge (1-d_0 \cdot j^{-d_1 \kappa_0}) |\omega| &\text{for all } \omega \in \mathcal{P}_{j-1}, \text{ for all } j\ge J_1,\\ | \Omega_j|\ge |\Omega^{\prime}_j|- e^{-j\bar \eta}|\omega_0| &\text{for all } j\ge J_1. \end{cases} \end{align} $$
Before establishing this claim, we note that, mutatis mutandis, equation (2.20) combined with the arguments leading to equation (2.19) implies equation (2.9), while the more precise claim in equation (2.10) follows from the refinement of equation (2.20) coming from the second statement of [Reference de Melo and van StrienDMS, Lemma V.6.9] (see the use of [Reference MoreiraMo, Lemma 6.3] in [Reference MoreiraMo, Lemma 6.4 and Proposition 6.5]).
To show equation (2.20), we proceed in three steps, performing the necessary changes in the proof in [Reference de Melo and van StrienDMS, §V.6]. Recall equation (2.12).
First, up to taking larger
![]() $N_0$
, the conclusion of [Reference de Melo and van StrienDMS, Lemma V.6.5] (which deals with
$N_0$
, the conclusion of [Reference de Melo and van StrienDMS, Lemma V.6.5] (which deals with
![]() $(BA^{\prime }_{j})$
for
$(BA^{\prime }_{j})$
for
![]() $\omega \in \mathcal {P}_{j-1}$
satisfying
$\omega \in \mathcal {P}_{j-1}$
satisfying
![]() $(BA^{\prime }_{j-1})$
and
$(BA^{\prime }_{j-1})$
and
![]() $(EX_{j-1})$
and having a return at time j), if we replace the exponential rate
$(EX_{j-1})$
and having a return at time j), if we replace the exponential rate
![]() $(BA^{\prime }_{j-1})$
there by our polynomial rate in equation (2.8), becomes
$(BA^{\prime }_{j-1})$
there by our polynomial rate in equation (2.8), becomes
 $$ \begin{align} \frac{|\omega \setminus \bigcup_{r \geq \kappa_0\log j}\, \omega_{r,\ell}|}{|\omega|}\ge 1- C j ^{-d_1 \kappa_0}\quad \text{for all } j \geq N_0. \end{align} $$
$$ \begin{align} \frac{|\omega \setminus \bigcup_{r \geq \kappa_0\log j}\, \omega_{r,\ell}|}{|\omega|}\ge 1- C j ^{-d_1 \kappa_0}\quad \text{for all } j \geq N_0. \end{align} $$
To show this first claim, use that the constant
![]() $C_0 \in (0,1)$
(introduced above) is independent of
$C_0 \in (0,1)$
(introduced above) is independent of
![]() $\kappa _0$
(because
$\kappa _0$
(because
![]() $\unicode{x3bb} _\mathrm{CE}$
does not depend on
$\unicode{x3bb} _\mathrm{CE}$
does not depend on
![]() $\kappa _0$
) and that [Reference de Melo and van StrienDMS, Lemma V.6.1] gives that the bound period p of a free return
$\kappa _0$
) and that [Reference de Melo and van StrienDMS, Lemma V.6.1] gives that the bound period p of a free return
![]() $\nu <j$
with
$\nu <j$
with
satisfies
![]() $p\ge C_0 r^{\prime }$
. Then, up to taking larger
$p\ge C_0 r^{\prime }$
. Then, up to taking larger
![]() $N_0$
, we can replace [Reference de Melo and van StrienDMS, V.(6.20)] in the proof of [Reference de Melo and van StrienDMS, Lemma V.6.5] by
$N_0$
, we can replace [Reference de Melo and van StrienDMS, V.(6.20)] in the proof of [Reference de Melo and van StrienDMS, Lemma V.6.5] by

where we used
![]() $d_1 \leq ({C_0}/{4}) \log \unicode{x3bb} _\mathrm{CE}$
in the second inequality. We can thus replace the chain of inequalities after [Reference de Melo and van StrienDMS, V.(6.20)] (using the distortion bound in equation (2.16) for
$d_1 \leq ({C_0}/{4}) \log \unicode{x3bb} _\mathrm{CE}$
in the second inequality. We can thus replace the chain of inequalities after [Reference de Melo and van StrienDMS, V.(6.20)] (using the distortion bound in equation (2.16) for
![]() $\tilde \omega \subset \omega $
the largest interval with
$\tilde \omega \subset \omega $
the largest interval with
![]() $x_n(\tilde \omega )\subset U_{r_0/2}$
, taking
$x_n(\tilde \omega )\subset U_{r_0/2}$
, taking
![]() $\epsilon $
small enough and
$\epsilon $
small enough and
![]() $N_0$
large enough such that equation (2.23) also holds for
$N_0$
large enough such that equation (2.23) also holds for
![]() $\tilde \omega $
) by
$\tilde \omega $
) by
 $$ \begin{align*} \frac{|\bigcup_{r \ge \kappa_0\log j}\, \omega_{r,\ell}|}{|\omega|} \le \frac{|\bigcup_{r \ge \kappa_0\log j}\, \omega_{r,\ell}|}{|\tilde \omega|} \le C\frac 1{j^{\kappa_0}}\frac{1}{|x_j(\tilde \omega)|} \le C j^{-d_1 \cdot \kappa_0}. \end{align*} $$
$$ \begin{align*} \frac{|\bigcup_{r \ge \kappa_0\log j}\, \omega_{r,\ell}|}{|\omega|} \le \frac{|\bigcup_{r \ge \kappa_0\log j}\, \omega_{r,\ell}|}{|\tilde \omega|} \le C\frac 1{j^{\kappa_0}}\frac{1}{|x_j(\tilde \omega)|} \le C j^{-d_1 \cdot \kappa_0}. \end{align*} $$
Second, [Reference de Melo and van StrienDMS, Lemma V.6.6] (which deals with
![]() $(FA_j)$
) uses equation (2.14) only via [Reference de Melo and van StrienDMS, Lemma V.6.3], while [Reference de Melo and van StrienDMS, Lemma V.6.3] still holds (with the same proof) if we replace equation (2.14) by our stronger assumption in equation (2.8). (We mention here a typo: [Reference de Melo and van StrienDMS, V.(6.24)] follows from [Reference de Melo and van StrienDMS, V.(6.22)] (and not [Reference de Melo and van StrienDMS, V.(6.20)] as stated there).)
$(FA_j)$
) uses equation (2.14) only via [Reference de Melo and van StrienDMS, Lemma V.6.3], while [Reference de Melo and van StrienDMS, Lemma V.6.3] still holds (with the same proof) if we replace equation (2.14) by our stronger assumption in equation (2.8). (We mention here a typo: [Reference de Melo and van StrienDMS, V.(6.24)] follows from [Reference de Melo and van StrienDMS, V.(6.22)] (and not [Reference de Melo and van StrienDMS, V.(6.20)] as stated there).)
Third, [Reference de Melo and van StrienDMS, Lemmas V.6.7–6.9] are unchanged, establishing equation (2.20).
Lemma 2.3 is the analogue of [Reference SchnellmannSch, (III)′]).
Lemma 2.3. (No exceptionally small sets)
For any
![]() $\kappa _1>\kappa _0$
, there exists
$\kappa _1>\kappa _0$
, there exists
![]() $N_1\ge N_0$
such that
$N_1\ge N_0$
such that
![]() $|x_j(\omega )|> j^{-\kappa _1}$
for all
$|x_j(\omega )|> j^{-\kappa _1}$
for all
![]() $j\ge N_1$
and
$j\ge N_1$
and
![]() $\omega \in \mathcal {P}_j=\mathcal {P}_j(a_{*},\kappa _0)$
.
$\omega \in \mathcal {P}_j=\mathcal {P}_j(a_{*},\kappa _0)$
.
Proof. We first show the lemma assuming that there exists
![]() $d_2\in (0,1)$
such that for any
$d_2\in (0,1)$
such that for any
![]() $j\ge N_0$
and any
$j\ge N_0$
and any
![]() $ \omega \in \mathcal {P}_{j}$
, we have
$ \omega \in \mathcal {P}_{j}$
, we have
 $$ \begin{align} |x_j(\omega)| \geq \frac{d_2 e^{-r_0}(1-1/e)}{( \kappa_0\log j)^2 j^{\kappa_0}} , \end{align} $$
$$ \begin{align} |x_j(\omega)| \geq \frac{d_2 e^{-r_0}(1-1/e)}{( \kappa_0\log j)^2 j^{\kappa_0}} , \end{align} $$
with
![]() $r_0$
as in the proof of Proposition 2.2. Indeed, equation (2.24) implies that
$r_0$
as in the proof of Proposition 2.2. Indeed, equation (2.24) implies that
 $$ \begin{align*} |x_{j}(\omega)|\geq \frac{d_2 e^{-r_0}(1 - e^{-1})}{\kappa_0^2} \frac{1}{j^{\kappa_0} (\log j)^2} \quad \text{for all } \omega\in\mathcal{P}_j, \text{ for all } j\ge N_0. \end{align*} $$
$$ \begin{align*} |x_{j}(\omega)|\geq \frac{d_2 e^{-r_0}(1 - e^{-1})}{\kappa_0^2} \frac{1}{j^{\kappa_0} (\log j)^2} \quad \text{for all } \omega\in\mathcal{P}_j, \text{ for all } j\ge N_0. \end{align*} $$
Clearly, there exists
![]() $N_1(\kappa _1)\ge N_0$
such that the right-hand side is larger than
$N_1(\kappa _1)\ge N_0$
such that the right-hand side is larger than
![]() $j^{-\kappa _1}$
for all
$j^{-\kappa _1}$
for all
![]() $j\ge N_1$
.
$j\ge N_1$
.
To establish equation (2.24), we shall use equation (2.8). If
![]() $j+1$
is an essential free return time of
$j+1$
is an essential free return time of
![]() $\omega $
, then taking r minimal such that
$\omega $
, then taking r minimal such that
![]() $x_{j}(\omega )$
contains an interval
$x_{j}(\omega )$
contains an interval
![]() $I_{r,\ell }^{\pm }$
,
$I_{r,\ell }^{\pm }$
,
 $$ \begin{align} |x_{j}(\omega)|\ge |I_{r,\ell}^{\pm}|=e^{-r}\frac {1-1/e}{r^2}> \frac{j^{-\kappa_0}(1-1/e)}{( \kappa_0\log j)^2}. \end{align} $$
$$ \begin{align} |x_{j}(\omega)|\ge |I_{r,\ell}^{\pm}|=e^{-r}\frac {1-1/e}{r^2}> \frac{j^{-\kappa_0}(1-1/e)}{( \kappa_0\log j)^2}. \end{align} $$
Otherwise, letting
![]() $j^{\prime }+1=\nu _{i^{\prime }}(\omega )\ge \nu _1(\omega )$
be the largest essential free return time of
$j^{\prime }+1=\nu _{i^{\prime }}(\omega )\ge \nu _1(\omega )$
be the largest essential free return time of
![]() $\omega $
such that
$\omega $
such that
![]() $ j^{\prime }+1<j+1$
, we have
$ j^{\prime }+1<j+1$
, we have
![]() $\omega \in \mathcal {P}_{j^{\prime }}$
(since if
$\omega \in \mathcal {P}_{j^{\prime }}$
(since if
![]() $\tilde {\omega } \supset \omega $
,
$\tilde {\omega } \supset \omega $
,
![]() $\tilde {\omega } \in \mathcal {P}_{j^{\prime }}$
, then
$\tilde {\omega } \in \mathcal {P}_{j^{\prime }}$
, then
![]() $\tilde {\omega }$
is never cut between time
$\tilde {\omega }$
is never cut between time
![]() $j^{\prime }$
and j), so that equation (2.25) implies
$j^{\prime }$
and j), so that equation (2.25) implies
We shall combine the above bound with [Reference de Melo and van StrienDMS, Lemma V.6.3 and Propositions V.6.1 and V.6.2] to handle the three cases left, namely: the time
![]() $j+1$
is an inessential free return of
$j+1$
is an inessential free return of
![]() $\omega $
; the time
$\omega $
; the time
![]() $j+1$
is a return within a bound period of
$j+1$
is a return within a bound period of
![]() $\omega $
; and the intersection of
$\omega $
; and the intersection of
![]() $x_j(\omega )$
and
$x_j(\omega )$
and
![]() $U_{r_0}$
is empty.
$U_{r_0}$
is empty.
If
![]() $j+1=\nu _i( \omega )$
is an inessential free return, then [Reference de Melo and van StrienDMS, V.(6.15) in Lemma V.6.3] gives, for
$j+1=\nu _i( \omega )$
is an inessential free return, then [Reference de Melo and van StrienDMS, V.(6.15) in Lemma V.6.3] gives, for
![]() $i^{\prime }\le i$
as defined above,
$i^{\prime }\le i$
as defined above,
If
![]() $j+1$
is a return within the bound period of a previous free return
$j+1$
is a return within the bound period of a previous free return
![]() $j^{\prime \prime }+1$
of
$j^{\prime \prime }+1$
of
![]() $\omega $
, then using equation (2.25) for the bound period of an essential return, respectively equation (2.26) for the bound period of a non-essential return, and applying the first claim of [Reference de Melo and van StrienDMS, Lemma V.6.3], we find
$\omega $
, then using equation (2.25) for the bound period of an essential return, respectively equation (2.26) for the bound period of a non-essential return, and applying the first claim of [Reference de Melo and van StrienDMS, Lemma V.6.3], we find
![]() $d_2\in (0,1)$
such that
$d_2\in (0,1)$
such that
If
![]() $x_j(\omega )\cap U_{r_0}=\emptyset $
, then [Reference de Melo and van StrienDMS, V.(6.2) in Propositions V.6.1 and V.6.2] and equation (2.25) give
$x_j(\omega )\cap U_{r_0}=\emptyset $
, then [Reference de Melo and van StrienDMS, V.(6.2) in Propositions V.6.1 and V.6.2] and equation (2.25) give
 $$ \begin{align} |x_{j}( \omega)|\ge d_2 e^{-r_0} |x_{j^{\prime}}( \omega)|> \frac{d_2 e^{-r_0}(1-1/e)}{( \kappa_0\log j)^2j^{\kappa_0}}. \end{align} $$
$$ \begin{align} |x_{j}( \omega)|\ge d_2 e^{-r_0} |x_{j^{\prime}}( \omega)|> \frac{d_2 e^{-r_0}(1-1/e)}{( \kappa_0\log j)^2j^{\kappa_0}}. \end{align} $$
2.3 A Hölder local distortion estimate
From now on, let
![]() $a_{*}\in (a_{\mathrm {mix}}, 4)$
be a Misiurewicz parameter, fix
$a_{*}\in (a_{\mathrm {mix}}, 4)$
be a Misiurewicz parameter, fix
![]() $\kappa _0\ge 11 / 3 d_1$
and let
$\kappa _0\ge 11 / 3 d_1$
and let
![]() $\Omega _{*}=\Omega _{*}(a_{*},\kappa _0)\subset \Omega _\mathrm{BC}= \Omega _\mathrm{BC}(a_{*})$
be the positive measure Cantor set constructed in §2.2 via families
$\Omega _{*}=\Omega _{*}(a_{*},\kappa _0)\subset \Omega _\mathrm{BC}= \Omega _\mathrm{BC}(a_{*})$
be the positive measure Cantor set constructed in §2.2 via families
![]() $\mathcal {P}_j=\mathcal {P}_j(a_{*},\kappa _0)$
. The following replaces [Reference SchnellmannSch, equations (33) and (31)]. (For [Reference SchnellmannSch, equation (30)], see equation (2.2). We do not need [Reference SchnellmannSch, equation (32)].) The bound in equation (2.31) is new.
$\mathcal {P}_j=\mathcal {P}_j(a_{*},\kappa _0)$
. The following replaces [Reference SchnellmannSch, equations (33) and (31)]. (For [Reference SchnellmannSch, equation (30)], see equation (2.2). We do not need [Reference SchnellmannSch, equation (32)].) The bound in equation (2.31) is new.
Lemma 2.4. (Hölder distortion bounds)
There exists
![]() $C<\infty $
such that for all
$C<\infty $
such that for all
![]() $n\ge N_0$
(with
$n\ge N_0$
(with
![]() $N_0$
as in Proposition 2.2) and any
$N_0$
as in Proposition 2.2) and any
![]() $\omega \in \mathcal {P}_n=\mathcal {P}_n(a_{*},\kappa _0)$
,
$\omega \in \mathcal {P}_n=\mathcal {P}_n(a_{*},\kappa _0)$
,
 $$ \begin{align} \frac 1 C \leq \bigg|\frac{x_n^{\prime}(a)/x_j^{\prime}(a)}{(T_a^{n-j})^{\prime}(x_j(a))} \bigg| \leq C \quad \text{for all } 1 \leq j \leq n , \text{ for all } a \in \omega. \end{align} $$
$$ \begin{align} \frac 1 C \leq \bigg|\frac{x_n^{\prime}(a)/x_j^{\prime}(a)}{(T_a^{n-j})^{\prime}(x_j(a))} \bigg| \leq C \quad \text{for all } 1 \leq j \leq n , \text{ for all } a \in \omega. \end{align} $$
In addition, there exist
![]() $C<\infty $
and
$C<\infty $
and
![]() $M_0>\kappa _0$
such that, for all
$M_0>\kappa _0$
such that, for all
![]() $n\ge N_0$
, each
$n\ge N_0$
, each
![]() $\tilde \omega \in \mathcal {P}_n=\mathcal {P}_n(a_{*},\kappa _0)$
, and every
$\tilde \omega \in \mathcal {P}_n=\mathcal {P}_n(a_{*},\kappa _0)$
, and every
![]() $\omega \subset \tilde \omega $
and
$\omega \subset \tilde \omega $
and
![]() $\alpha \in [0,1)$
satisfying
$\alpha \in [0,1)$
satisfying
we have
 $$ \begin{align} \bigg| \frac{x_n^{\prime}(a_1)}{x_n^{\prime}(a_2)} \bigg| \leq 1 + C|x_{n}([a_1,a_2])|^\alpha \quad \text{for all } a_1, a_2\in\omega. \end{align} $$
$$ \begin{align} \bigg| \frac{x_n^{\prime}(a_1)}{x_n^{\prime}(a_2)} \bigg| \leq 1 + C|x_{n}([a_1,a_2])|^\alpha \quad \text{for all } a_1, a_2\in\omega. \end{align} $$
If
![]() $\alpha =0$
, and
$\alpha =0$
, and
![]() $n+1$
is a free return of
$n+1$
is a free return of
![]() $\omega \in \mathcal {P}_n$
, the bound in equation (2.31) is just equation (2.16). We shall require equation (2.31) for some
$\omega \in \mathcal {P}_n$
, the bound in equation (2.31) is just equation (2.16). We shall require equation (2.31) for some
![]() $\alpha>0$
in Corollary 3.4.
$\alpha>0$
in Corollary 3.4.
Proof. The bound in equation (2.29) is an immediate consequence of equation (2.6).
We first claim that there exist
![]() $C^{\prime }$
and
$C^{\prime }$
and
![]() $\kappa _2>0$
such that for any n,
$\kappa _2>0$
such that for any n,
 $$ \begin{align} \sum_{i=0}^{j-1} |x_i(\omega)|\le C^{\prime} j^{\kappa_0+1+\kappa_2 } |x_{j}(\omega)| \quad \text{for all } 1\le j\le n ,\,\text{ for all } \omega\subset \tilde \omega \in \mathcal{P}_n. \end{align} $$
$$ \begin{align} \sum_{i=0}^{j-1} |x_i(\omega)|\le C^{\prime} j^{\kappa_0+1+\kappa_2 } |x_{j}(\omega)| \quad \text{for all } 1\le j\le n ,\,\text{ for all } \omega\subset \tilde \omega \in \mathcal{P}_n. \end{align} $$
(Our proof is inspired from that of [Reference de Melo and van StrienDMS, Theorem V.6.2]. This is suboptimal but enough for our purposes. Adapting instead [Reference de Melo and van StrienDMS, Lemma V.6.4] could enhance equation (2.31).) To start, there is C such that for any
![]() $0\le i\le j\le n$
, using equations (2.3) and (2.29), there exists
$0\le i\le j\le n$
, using equations (2.3) and (2.29), there exists
![]() $a=a(i,j,\omega )\in \omega $
such that, setting
$a=a(i,j,\omega )\in \omega $
such that, setting
![]() $X_{i,j}= x_j \circ x_i^{-1}$
,
$X_{i,j}= x_j \circ x_i^{-1}$
,
 $$ \begin{align} \frac{|x_i(\omega)|}{ |x_{j}(\omega)| } &= \frac{|x_i(\omega)|}{ |X_{i,j} (x_{i}(\omega))|}= \frac{|x^{\prime}_i(a)|}{ |x^{\prime}_{j}(a)|} \le \frac{C}{ |(T^{j-i}_a)^{\prime}(T^{i+1}_a(c))| }. \end{align} $$
$$ \begin{align} \frac{|x_i(\omega)|}{ |x_{j}(\omega)| } &= \frac{|x_i(\omega)|}{ |X_{i,j} (x_{i}(\omega))|}= \frac{|x^{\prime}_i(a)|}{ |x^{\prime}_{j}(a)|} \le \frac{C}{ |(T^{j-i}_a)^{\prime}(T^{i+1}_a(c))| }. \end{align} $$
(We used
![]() $X^{\prime }_{i,j}=x^{\prime }_j/x^{\prime }_i$
and the mean value theorem in the second equality.) Next, let
$X^{\prime }_{i,j}=x^{\prime }_j/x^{\prime }_i$
and the mean value theorem in the second equality.) Next, let
![]() $s_j(a)$
be the largest
$s_j(a)$
be the largest
![]() $\ell $
with
$\ell $
with
![]() $\nu _{\ell }(a)\le j$
, and put
$\nu _{\ell }(a)\le j$
, and put
 $$ \begin{align*} &q_\ell(a)=\nu_{\ell+1}(a)-(\nu_\ell(a)+p_\ell(a)+1), \quad \ell=0, \ldots, s_j(a)-1, \\[-2pt] &q_{s_j(a)}(a)=\operatorname\max\{0, j-(\nu_{s_j(a)}(a)+p_{s_j(a)}(a)+1)\} , \quad F_j(a)= \sum_{\ell=0}^{s_j(a)} q_\ell(a). \end{align*} $$
$$ \begin{align*} &q_\ell(a)=\nu_{\ell+1}(a)-(\nu_\ell(a)+p_\ell(a)+1), \quad \ell=0, \ldots, s_j(a)-1, \\[-2pt] &q_{s_j(a)}(a)=\operatorname\max\{0, j-(\nu_{s_j(a)}(a)+p_{s_j(a)}(a)+1)\} , \quad F_j(a)= \sum_{\ell=0}^{s_j(a)} q_\ell(a). \end{align*} $$
(The condition
![]() $(FA)_n$
implicitly used in Proposition 2.2 says that for some fixed arbitrarily small
$(FA)_n$
implicitly used in Proposition 2.2 says that for some fixed arbitrarily small
![]() $\tau>0$
,
$\tau>0$
,
![]() $ F_\ell (a)\ge \ell (1-\tau )$
for
$ F_\ell (a)\ge \ell (1-\tau )$
for
![]() $N_0\le \ell \le n$
. We shall not need this here.) Set
$N_0\le \ell \le n$
. We shall not need this here.) Set
![]() $p_\ell =p_\ell (a)$
,
$p_\ell =p_\ell (a)$
,
![]() $\nu _\ell =\nu _\ell (a)$
,
$\nu _\ell =\nu _\ell (a)$
,
![]() $q_\ell =q_\ell (a)$
, and
$q_\ell =q_\ell (a)$
, and
![]() $s_j=s_j(a)$
. Assume first that
$s_j=s_j(a)$
. Assume first that
![]() $i=0$
. Then, we have (see e.g. [Reference de Melo and van StrienDMS, V.(6.11)])
$i=0$
. Then, we have (see e.g. [Reference de Melo and van StrienDMS, V.(6.11)])
 $$ \begin{align*} \nonumber|(T^{j-i}_a)^{\prime}(T^{i+1}_a(c))|&=|(T^{j}_a)^{\prime}(T_a(c))|\\ \nonumber&=|(T^{\nu_{1}-1}_a)^{\prime}(T_a(c))| \cdot |(T^{j+1-\nu_{s_j}}_a)^{\prime}(T^{\nu_s}_a(c))|\\ \nonumber&\quad \cdot \bigg (\prod_{\ell=1}^{s_j-1}|(T^{p_{\ell}+1}_a)^{\prime}(T^{\nu_\ell}_a(c))| |(T^{q_\ell}_a)^{\prime}(T^{\nu_\ell+p_\ell+1}_a(c))| \bigg ). \end{align*} $$
$$ \begin{align*} \nonumber|(T^{j-i}_a)^{\prime}(T^{i+1}_a(c))|&=|(T^{j}_a)^{\prime}(T_a(c))|\\ \nonumber&=|(T^{\nu_{1}-1}_a)^{\prime}(T_a(c))| \cdot |(T^{j+1-\nu_{s_j}}_a)^{\prime}(T^{\nu_s}_a(c))|\\ \nonumber&\quad \cdot \bigg (\prod_{\ell=1}^{s_j-1}|(T^{p_{\ell}+1}_a)^{\prime}(T^{\nu_\ell}_a(c))| |(T^{q_\ell}_a)^{\prime}(T^{\nu_\ell+p_\ell+1}_a(c))| \bigg ). \end{align*} $$
Since a satisfies
![]() $(BA)_m$
and
$(BA)_m$
and
![]() $(FA)_m$
for all
$(FA)_m$
for all
![]() $m\le n$
, the bounds in equation (2.15) give
$m\le n$
, the bounds in equation (2.15) give
![]() $\unicode{x3bb} _\mathrm{CE}$
,
$\unicode{x3bb} _\mathrm{CE}$
,
![]() $\gamma _0>0$
and
$\gamma _0>0$
and
![]() $C_0>0$
such that
$C_0>0$
such that

Similarly,
![]() $|(T^{\nu _{1}-1}_a)^{\prime }(T_a(c))|>C_0 e^{\gamma _0 \nu _1}$
. Next, if
$|(T^{\nu _{1}-1}_a)^{\prime }(T_a(c))|>C_0 e^{\gamma _0 \nu _1}$
. Next, if
![]() $j \le \nu _{s_j}+p_{s_j}+1$
, we have
$j \le \nu _{s_j}+p_{s_j}+1$
, we have
where we used equation (2.8) and [Reference de Melo and van StrienDMS, Lemma V.6.1.b, Proposition V.6.1]. If
![]() $j> \nu _{s_j}+p_{s_j}+1$
, we have, using [Reference de Melo and van StrienDMS, Lemma V.6.1.c, Proposition V.6.1],
$j> \nu _{s_j}+p_{s_j}+1$
, we have, using [Reference de Melo and van StrienDMS, Lemma V.6.1.c, Proposition V.6.1],
Summarizing,

Since
![]() $p_\ell {\kern-1.2pt}\ge{\kern-1.2pt} C_0 r_0$
(see after equation (2.22)), we have
$p_\ell {\kern-1.2pt}\ge{\kern-1.2pt} C_0 r_0$
(see after equation (2.22)), we have
![]() $j{\kern-1.2pt}-{\kern-1.2pt}F_j{\kern-1.2pt}\ge{\kern-1.2pt} j C_0 r_0$
while
$j{\kern-1.2pt}-{\kern-1.2pt}F_j{\kern-1.2pt}\ge{\kern-1.2pt} j C_0 r_0$
while
![]() $s_j{\kern-1.2pt}\le{\kern-1.2pt} j/(C_0r_0)$
. We took
$s_j{\kern-1.2pt}\le{\kern-1.2pt} j/(C_0r_0)$
. We took
![]() $r_0$
large enough (see after equation (2.15)) such that
$r_0$
large enough (see after equation (2.15)) such that
Finally, using the trivial bound
![]() $e^{\gamma _0 F_{j}(a)}\ge 1$
, we find
$e^{\gamma _0 F_{j}(a)}\ge 1$
, we find
If
![]() $i\ge 1$
and
$i\ge 1$
and
![]() $\nu _{\ell _i}(a)+p_{\ell _i}(a)<i<\nu _{\ell _i+1}(a)$
for some
$\nu _{\ell _i}(a)+p_{\ell _i}(a)<i<\nu _{\ell _i+1}(a)$
for some
![]() $\ell _i\ge 1$
, then we proceed as for
$\ell _i\ge 1$
, then we proceed as for
![]() $i=0$
, replacing
$i=0$
, replacing
![]() $|(T^{\nu _{1}-1}_a)^{\prime }(T_a(c))|$
by
$|(T^{\nu _{1}-1}_a)^{\prime }(T_a(c))|$
by
![]() $|(T^{\nu _{\ell _i+1}-i}_a)^{\prime }(T^{i+1}_a(c))|$
and setting
$|(T^{\nu _{\ell _i+1}-i}_a)^{\prime }(T^{i+1}_a(c))|$
and setting
![]() $F_{i,j}(a)=\nu _{\ell _i+1}(a)-i+\sum _{\ell \ge \ell _i+1}^{s_j(a)} q_\ell (a)$
. Then,
$F_{i,j}(a)=\nu _{\ell _i+1}(a)-i+\sum _{\ell \ge \ell _i+1}^{s_j(a)} q_\ell (a)$
. Then,

We have
![]() $j-i-F_{i,j}\ge (j-i) C_0 r_0$
, while
$j-i-F_{i,j}\ge (j-i) C_0 r_0$
, while
![]() $s_j-s_i\le (j-i)/(C_0r_0)$
, and we find, using
$s_j-s_i\le (j-i)/(C_0r_0)$
, and we find, using
![]() $e^{\gamma _0 F_{i,j}(a)}\ge 1$
(we do not know or need
$e^{\gamma _0 F_{i,j}(a)}\ge 1$
(we do not know or need
![]() $F_{i,j}(a)\ge (1-\tau )(j-i)$
),
$F_{i,j}(a)\ge (1-\tau )(j-i)$
),
Otherwise,
![]() $\nu _{\ell _i}(a)\le i-1\le \nu _{\ell _i}(a)+p_{\ell _i}(a)$
for some
$\nu _{\ell _i}(a)\le i-1\le \nu _{\ell _i}(a)+p_{\ell _i}(a)$
for some
![]() $\ell _i\ge 1$
. There may be (non-free) returns during the
$\ell _i\ge 1$
. There may be (non-free) returns during the
![]() $\ell _i$
th bound period. To bypass this difficulty, we exploit that the length of the
$\ell _i$
th bound period. To bypass this difficulty, we exploit that the length of the
![]() $\ell $
th bound period is of the order r if
$\ell $
th bound period is of the order r if
![]() $x_{\nu _{\ell }}(a)\in I_{r}$
[Reference de Melo and van StrienDMS, Lemma V.6.1a]. By equation (2.11), we have
$x_{\nu _{\ell }}(a)\in I_{r}$
[Reference de Melo and van StrienDMS, Lemma V.6.1a]. By equation (2.11), we have
![]() $r_{\ell _i}=O(\log (\nu _{\ell _i}))\le C \kappa _0 \log i$
. Thus, the missing factor in the
$r_{\ell _i}=O(\log (\nu _{\ell _i}))\le C \kappa _0 \log i$
. Thus, the missing factor in the
![]() $\ell $
th bound period is
$\ell $
th bound period is
![]() $\le \Lambda ^{ C\kappa _0\log i}\le i^{\kappa _2}$
, and
$\le \Lambda ^{ C\kappa _0\log i}\le i^{\kappa _2}$
, and
Summing over i, and recalling equation (2.33), this establishes equation (2.32).
Next, taking
![]() $a_1,a_2 \in \omega $
, note that equation (2.7) (using the first bound of equation (2.4) if
$a_1,a_2 \in \omega $
, note that equation (2.7) (using the first bound of equation (2.4) if
![]() $i<N_0$
) implies that for all
$i<N_0$
) implies that for all
![]() $N_0\le i\le n$
, recalling
$N_0\le i\le n$
, recalling
![]() $T^{\prime }_a(x)=a(1-2x)$
,
$T^{\prime }_a(x)=a(1-2x)$
,
 $$ \begin{align} \nonumber |T_{a_1}^{\prime}(x_i(a_1))&-T_{a_2}^{\prime}(x_i(a_2))|\\ \nonumber & \leq |T_{a_1}^{\prime}(x_i(a_1)) - T_{a_2}^{\prime} (x_i (a_1))| + |T_{a_2}^{\prime} (x_i (a_1)) - T_{a_2}^{\prime} (x_i (a_2))| \\ &\leq |a_1-a_2| + 2 a_2 |x_i(a_1) - x_i(a_2)| \leq (C + 2 a_2) |x_i(\omega)|. \end{align} $$
$$ \begin{align} \nonumber |T_{a_1}^{\prime}(x_i(a_1))&-T_{a_2}^{\prime}(x_i(a_2))|\\ \nonumber & \leq |T_{a_1}^{\prime}(x_i(a_1)) - T_{a_2}^{\prime} (x_i (a_1))| + |T_{a_2}^{\prime} (x_i (a_1)) - T_{a_2}^{\prime} (x_i (a_2))| \\ &\leq |a_1-a_2| + 2 a_2 |x_i(a_1) - x_i(a_2)| \leq (C + 2 a_2) |x_i(\omega)|. \end{align} $$
(Note that equation (2.35) replaces [Reference SchnellmannSch, (36)].) We claim that there exists
![]() $C^{\prime \prime }$
with
$C^{\prime \prime }$
with
 $$ \begin{align} \bigg|\frac {(T_{a_1}^j)^{\prime}(x_0(a_1))}{(T_{a_2}^j)^{\prime}(x_0(a_2))}\bigg| \leq 1 + C^{\prime\prime} e^{C^{\prime\prime}} j^{2\kappa_0+1+\kappa_2} |x_{j}(\omega)| \, \quad\text{for all } 1\leq j\leq n. \end{align} $$
$$ \begin{align} \bigg|\frac {(T_{a_1}^j)^{\prime}(x_0(a_1))}{(T_{a_2}^j)^{\prime}(x_0(a_2))}\bigg| \leq 1 + C^{\prime\prime} e^{C^{\prime\prime}} j^{2\kappa_0+1+\kappa_2} |x_{j}(\omega)| \, \quad\text{for all } 1\leq j\leq n. \end{align} $$
(The above replaces [Reference SchnellmannSch, (37)].) Indeed, using the classical bound
 $$ \begin{align*} \prod_{i=0}^{j-1} (1+ \upsilon_i) \leq \exp \bigg( \sum_{i=0}^{j-1}\upsilon_i \bigg) \leq 1+ e^{\sum_{i=0}^{j-1}\upsilon_i}\sum_{i=0}^{j-1}\upsilon_i\quad \mbox{if all }\upsilon_i\geq 0, \end{align*} $$
$$ \begin{align*} \prod_{i=0}^{j-1} (1+ \upsilon_i) \leq \exp \bigg( \sum_{i=0}^{j-1}\upsilon_i \bigg) \leq 1+ e^{\sum_{i=0}^{j-1}\upsilon_i}\sum_{i=0}^{j-1}\upsilon_i\quad \mbox{if all }\upsilon_i\geq 0, \end{align*} $$
we have, setting
![]() $C^{\prime \prime }=2C^{\prime }C(C+2a_2 )$
,
$C^{\prime \prime }=2C^{\prime }C(C+2a_2 )$
,
 $$ \begin{align} \nonumber \bigg| \frac{(T_{a_1}^j)^{\prime} (x_0 (a_1))}{(T_{a_2}^j)^{\prime} (x_0 (a_2))} & \bigg| = \bigg| \prod_{i=0}^{j-1} \frac{T_{a_1}^{\prime} (x_i (a_1))}{T_{a_2}^{\prime} (x_i (a_2))} \bigg| \\ \nonumber &\leq 1 + e^{\sum_i |-1 + {T_{a_1}^{\prime} (x_i (a_1))}/{T_{a_2}^{\prime} (x_i (a_2))} |} \cdot \sum_{i=0}^{j-1} \bigg|\frac{T_{a_1}^{\prime} (x_i (a_1))}{T_{a_2}^{\prime} (x_i (a_2))} - 1 \bigg| \\ \nonumber &\leq 1 + e^{(C + 2 a_2) \sum_{i=0}^{j-1} C |x_i(\omega)| i^{\kappa_0}} \cdot (C + 2 a_2) \sum_{i=0}^{j-1} C |x_i(\omega)| i^{\kappa_0} \\ &\leq 1 + e^{C^{\prime\prime} j^{2\kappa_0+1+\kappa_2} |x_{j}(\omega)|} \cdot C^{\prime\prime} j^{2\kappa_0+1+\kappa_2} |x_{j}(\omega)| \quad\text{for all } j\leq n, \end{align} $$
$$ \begin{align} \nonumber \bigg| \frac{(T_{a_1}^j)^{\prime} (x_0 (a_1))}{(T_{a_2}^j)^{\prime} (x_0 (a_2))} & \bigg| = \bigg| \prod_{i=0}^{j-1} \frac{T_{a_1}^{\prime} (x_i (a_1))}{T_{a_2}^{\prime} (x_i (a_2))} \bigg| \\ \nonumber &\leq 1 + e^{\sum_i |-1 + {T_{a_1}^{\prime} (x_i (a_1))}/{T_{a_2}^{\prime} (x_i (a_2))} |} \cdot \sum_{i=0}^{j-1} \bigg|\frac{T_{a_1}^{\prime} (x_i (a_1))}{T_{a_2}^{\prime} (x_i (a_2))} - 1 \bigg| \\ \nonumber &\leq 1 + e^{(C + 2 a_2) \sum_{i=0}^{j-1} C |x_i(\omega)| i^{\kappa_0}} \cdot (C + 2 a_2) \sum_{i=0}^{j-1} C |x_i(\omega)| i^{\kappa_0} \\ &\leq 1 + e^{C^{\prime\prime} j^{2\kappa_0+1+\kappa_2} |x_{j}(\omega)|} \cdot C^{\prime\prime} j^{2\kappa_0+1+\kappa_2} |x_{j}(\omega)| \quad\text{for all } j\leq n, \end{align} $$
where we used equations (2.35) and (2.8) (the first bound of equation (2.4) if
![]() $i<N_0$
) in the second inequality, and equation (2.32) in the last inequality. Setting
$i<N_0$
) in the second inequality, and equation (2.32) in the last inequality. Setting
![]() $M_0:=4\kappa _0+3+2\kappa _2$
, if equation (2.30) holds for
$M_0:=4\kappa _0+3+2\kappa _2$
, if equation (2.30) holds for
![]() $\omega $
, then equation (2.32) gives for all
$\omega $
, then equation (2.32) gives for all
![]() $N_0\le j \leq n$
,
$N_0\le j \leq n$
,
 $$ \begin{align*} C^{\prime\prime} j^{2\kappa_0 +1+\kappa_2} |x_{j}(\omega)| & \leq C^{\prime\prime} j^{2\kappa_0+1+\kappa_2} C^{\prime} j^{\kappa_0+1+\kappa_2}|x_{n}(\omega)|\\ &\leq C^{\prime} \frac{j^{3\kappa_0+3+2\kappa_2}}{n^{M_0/(1-\alpha)}} \le C^{\prime\prime\prime}. \end{align*} $$
$$ \begin{align*} C^{\prime\prime} j^{2\kappa_0 +1+\kappa_2} |x_{j}(\omega)| & \leq C^{\prime\prime} j^{2\kappa_0+1+\kappa_2} C^{\prime} j^{\kappa_0+1+\kappa_2}|x_{n}(\omega)|\\ &\leq C^{\prime} \frac{j^{3\kappa_0+3+2\kappa_2}}{n^{M_0/(1-\alpha)}} \le C^{\prime\prime\prime}. \end{align*} $$
This proves equation (2.36). Similarly,
![]() $ |{ (T_{a_2}^j)^{\prime }(x_0(a_2))}/{(T_{a_1}^j)^{\prime }(x_0(a_1))}| \leq 1+C^{\prime \prime } e^{C^{\prime \prime }} j^{2\kappa _0+1+\kappa _2} |x_{j}(\omega )|$
. Therefore,
$ |{ (T_{a_2}^j)^{\prime }(x_0(a_2))}/{(T_{a_1}^j)^{\prime }(x_0(a_1))}| \leq 1+C^{\prime \prime } e^{C^{\prime \prime }} j^{2\kappa _0+1+\kappa _2} |x_{j}(\omega )|$
. Therefore,
 $$ \begin{align*} \bigg |\frac1{(T_{a_1}^j)^{\prime}(x_0(a_1))}-\frac1{(T_{a_2}^j)^{\prime}(x_0(a_2))}\bigg | \leq C^{\prime\prime\prime}\frac{j^{2\kappa_0+1+\kappa_2} |x_{j}(\omega)|}{|(T_{a_1}^j)^{\prime}(x_0(a_1))|}. \end{align*} $$
$$ \begin{align*} \bigg |\frac1{(T_{a_1}^j)^{\prime}(x_0(a_1))}-\frac1{(T_{a_2}^j)^{\prime}(x_0(a_2))}\bigg | \leq C^{\prime\prime\prime}\frac{j^{2\kappa_0+1+\kappa_2} |x_{j}(\omega)|}{|(T_{a_1}^j)^{\prime}(x_0(a_1))|}. \end{align*} $$
We can then adapt the end of the proof of [Reference SchnellmannSch, (31)]. Comparing each term on the right-hand side of
 $$ \begin{align*} \frac{x_n^{\prime}(a)}{(T_a^n)^{\prime}(x_0(a))} =x_0^{\prime}(a)+\sum_{j=1}^n\frac{(\partial_aT_a)(x_{j-1}(a))}{(T_a^j)^{\prime}(x_0(a))}\quad \text{for all } a\in\tilde \omega\in \mathcal{P}_n, \end{align*} $$
$$ \begin{align*} \frac{x_n^{\prime}(a)}{(T_a^n)^{\prime}(x_0(a))} =x_0^{\prime}(a)+\sum_{j=1}^n\frac{(\partial_aT_a)(x_{j-1}(a))}{(T_a^j)^{\prime}(x_0(a))}\quad \text{for all } a\in\tilde \omega\in \mathcal{P}_n, \end{align*} $$
for
![]() $a=a_1$
and
$a=a_1$
and
![]() $a=a_2$
, we find, since
$a=a_2$
, we find, since
![]() $x_0^{\prime }(a)=\partial _ac_1(a)=1/4$
and
$x_0^{\prime }(a)=\partial _ac_1(a)=1/4$
and
recalling equation (2.5), and applying equation (2.32) and then equation (2.30) for
![]() $M_0=4\kappa _0+3+2\kappa _2$
,
$M_0=4\kappa _0+3+2\kappa _2$
,

Finally, we have, using equation (2.6) (which plays the role of [Reference SchnellmannSch, Lemma 2.4]),
 $$ \begin{align*} \bigg| \frac{x_n^{\prime}(a_1)}{x_n^{\prime}(a_2)} \bigg| & \leq \bigg| \frac{(T_{a_1}^n)^{\prime} (x_0(a_1))}{(T_{a_2}^n)^{\prime} (x_0 (a_2))} \bigg| \bigg( 1 + \tilde C |x_{n} (\omega)|^\alpha \frac{|(T_{a_2}^n)^{\prime}(x_0(a_2))|}{|x_n^{\prime}(a_2)|} \bigg) \\ & \leq 1 + C \tilde C |x_{n} (\omega)|^\alpha.\\[-34pt] \end{align*} $$
$$ \begin{align*} \bigg| \frac{x_n^{\prime}(a_1)}{x_n^{\prime}(a_2)} \bigg| & \leq \bigg| \frac{(T_{a_1}^n)^{\prime} (x_0(a_1))}{(T_{a_2}^n)^{\prime} (x_0 (a_2))} \bigg| \bigg( 1 + \tilde C |x_{n} (\omega)|^\alpha \frac{|(T_{a_2}^n)^{\prime}(x_0(a_2))|}{|x_n^{\prime}(a_2)|} \bigg) \\ & \leq 1 + C \tilde C |x_{n} (\omega)|^\alpha.\\[-34pt] \end{align*} $$
2.4 Uniform decorrelation and Hölder response
The maps
![]() $x_j$
are not the iterates of a fixed dynamical system admitting an invariant measure. To exploit statistical information on the iterates of the mixing CE map
$x_j$
are not the iterates of a fixed dynamical system admitting an invariant measure. To exploit statistical information on the iterates of the mixing CE map
![]() $(T_{a_0},\mu _{a_0})$
, we will ‘switch locally’ from
$(T_{a_0},\mu _{a_0})$
, we will ‘switch locally’ from
![]() $x_j$
to
$x_j$
to
![]() $T_{a_0}^j$
(see Lemma 3.3), using that any
$T_{a_0}^j$
(see Lemma 3.3), using that any
![]() $a\in \Omega _{*}$
satisfies the following uniform decorrelation result for Hölder continuous observables. (The factor
$a\in \Omega _{*}$
satisfies the following uniform decorrelation result for Hölder continuous observables. (The factor
![]() $\|\varphi \|_{L_1(dm)}$
in the right-hand side of [Reference SchnellmannSch, Proposition 4.3] is replaced in Proposition 2.5 by
$\|\varphi \|_{L_1(dm)}$
in the right-hand side of [Reference SchnellmannSch, Proposition 4.3] is replaced in Proposition 2.5 by
![]() $\|\varphi \|_{L^1(d\mu _a)}\le \|\varphi \|_{L^\infty (dm)}$
. This does not impact the use of [Reference SchnellmannSch, p. 36, Proposition 4.3].) For
$\|\varphi \|_{L^1(d\mu _a)}\le \|\varphi \|_{L^\infty (dm)}$
. This does not impact the use of [Reference SchnellmannSch, p. 36, Proposition 4.3].) For
![]() $q>1$
and
$q>1$
and
![]() $s\in [0, 1/q)$
, we denote by
$s\in [0, 1/q)$
, we denote by
![]() $H^s_q(I)=F^s_{q,2}(I)$
the Sobolev space of functions of differentiability s and integrability q supported in I (see [Reference Runst and SickelRS]).
$H^s_q(I)=F^s_{q,2}(I)$
the Sobolev space of functions of differentiability s and integrability q supported in I (see [Reference Runst and SickelRS]).
Proposition 2.5. (Uniform decay of correlations)
For any
![]() $s>0$
and
$s>0$
and
![]() $q>1$
, there exist
$q>1$
, there exist
![]() $C<\infty $
and
$C<\infty $
and
![]() $\rho ^s_q<1$
such that for all
$\rho ^s_q<1$
such that for all
![]() $\varphi \in H^s_q(I)$
,
$\varphi \in H^s_q(I)$
,
![]() $\psi \in L^\infty (dm)$
,
$\psi \in L^\infty (dm)$
,
![]() $a\in \Omega _{*}(a_{*}, \kappa _0)$
,
$a\in \Omega _{*}(a_{*}, \kappa _0)$
,
 $$ \begin{align*} \bigg| \!\int_0^1 \varphi (\psi\circ T_a^n) \, dm - \!\int_0^1\varphi \, dm \!\int_0^1\psi \, d\mu_a \bigg| \leq C \|\varphi\|_{H^s_q}\|\psi\|_{L^1(d\mu_a)}(\rho^s_q)^n\quad\text{for all } n\geq 1. \end{align*} $$
$$ \begin{align*} \bigg| \!\int_0^1 \varphi (\psi\circ T_a^n) \, dm - \!\int_0^1\varphi \, dm \!\int_0^1\psi \, d\mu_a \bigg| \leq C \|\varphi\|_{H^s_q}\|\psi\|_{L^1(d\mu_a)}(\rho^s_q)^n\quad\text{for all } n\geq 1. \end{align*} $$
For any
![]() $\varpi>0$
, there exist
$\varpi>0$
, there exist
![]() $C<\infty $
and
$C<\infty $
and
![]() $\rho _\varpi <1$
such that for all
$\rho _\varpi <1$
such that for all
![]() $\varphi \in C^\varpi $
,
$\varphi \in C^\varpi $
,
![]() $\psi \in L^\infty (dm)$
,
$\psi \in L^\infty (dm)$
,
![]() $a\in \Omega _{*}(a_{*}, \kappa _0)$
,
$a\in \Omega _{*}(a_{*}, \kappa _0)$
,
 $$ \begin{align*} \bigg| \!\int_0^1 \!\varphi (\psi\circ T_a^n)\, d\mu_{a } - \!\int_0^1 \!\varphi \, d\mu_{a} \!\int_0^1 \!\psi\, d\mu_{a} \bigg| \le C \|\varphi\|_\varpi \|\psi\|_{L^1(d\mu_a)} (\rho_\varpi)^{n}\quad \text{for all } n\ge 1. \end{align*} $$
$$ \begin{align*} \bigg| \!\int_0^1 \!\varphi (\psi\circ T_a^n)\, d\mu_{a } - \!\int_0^1 \!\varphi \, d\mu_{a} \!\int_0^1 \!\psi\, d\mu_{a} \bigg| \le C \|\varphi\|_\varpi \|\psi\|_{L^1(d\mu_a)} (\rho_\varpi)^{n}\quad \text{for all } n\ge 1. \end{align*} $$
We also use Hölder bounds on
![]() $a\mapsto \mu _a$
as a distribution (in Lemma 2.8).
$a\mapsto \mu _a$
as a distribution (in Lemma 2.8).
Proposition 2.6. (Fractional response)
For any
![]() $\Theta \in (0,1/2)$
, there exists C such that for all
$\Theta \in (0,1/2)$
, there exists C such that for all
![]() $\varphi \in C^{1/2}$
,
$\varphi \in C^{1/2}$
,
 $$ \begin{align} \bigg| \!\int \varphi \, d\mu_a - \!\int \varphi \, d\mu_{a^{\prime}} \bigg| \leq C |a-a^{\prime}|^\Theta \|\varphi\|_{1/2} \quad \text{for all } a, a^{\prime}\in \Omega_{*} (a_{*},\kappa_0). \end{align} $$
$$ \begin{align} \bigg| \!\int \varphi \, d\mu_a - \!\int \varphi \, d\mu_{a^{\prime}} \bigg| \leq C |a-a^{\prime}|^\Theta \|\varphi\|_{1/2} \quad \text{for all } a, a^{\prime}\in \Omega_{*} (a_{*},\kappa_0). \end{align} $$
Our proof of Proposition 2.5 uses the following facts.
Sublemma 2.7. For any
![]() $a\in \Omega _\mathrm{BC}$
, the density
$a\in \Omega _\mathrm{BC}$
, the density
![]() $h_a$
of
$h_a$
of
![]() $\mu _a$
lies in
$\mu _a$
lies in
![]() $H^s_q(I)$
for all
$H^s_q(I)$
for all
![]() $s\in [0,1/2)$
and
$s\in [0,1/2)$
and
![]() $q \in (1, 2/(1+2s))$
. In addition, for any
$q \in (1, 2/(1+2s))$
. In addition, for any
![]() $(H_0,\kappa _0)$
polynomially recurrent
$(H_0,\kappa _0)$
polynomially recurrent
![]() $a_{*}$
, there exists
$a_{*}$
, there exists
![]() $C_{s,q,a_{*}}<\infty $
such that
$C_{s,q,a_{*}}<\infty $
such that
Proof. In the Misiurewicz case, the first claim is [Reference SedroSe, Theorem 10], using Ruelle’s [Reference RuelleRu, Theorem 9, Remark 16.a] decomposition of
![]() $h_a$
into the sum of a
$h_a$
into the sum of a
![]() $C^1$
function and an exponentially decaying sum of ‘spikes’
$C^1$
function and an exponentially decaying sum of ‘spikes’
![]() $x\mapsto |x-c_k(a)|^{-1/2}$
and square root singularities
$x\mapsto |x-c_k(a)|^{-1/2}$
and square root singularities
![]() $x\mapsto |x-c_k(a)|^{1/2}$
. For a general
$x\mapsto |x-c_k(a)|^{1/2}$
. For a general
![]() $a \in \Omega _\mathrm{BC}$
, set
$a \in \Omega _\mathrm{BC}$
, set
![]() $ T^{-k}_{a,\varsigma }:=(T_a^k|_{U_{k,a,\varsigma }})^{-1}$
for
$ T^{-k}_{a,\varsigma }:=(T_a^k|_{U_{k,a,\varsigma }})^{-1}$
for
![]() $k\ge 1$
and
$k\ge 1$
and
![]() $\varsigma \in \pm $
, where
$\varsigma \in \pm $
, where
![]() $U_{k,a,\varsigma }$
is the monotonicity interval of
$U_{k,a,\varsigma }$
is the monotonicity interval of
![]() $T_a^k$
containing c, located to the right of c for
$T_a^k$
containing c, located to the right of c for
![]() $\varsigma =+$
, to the left of c for
$\varsigma =+$
, to the left of c for
![]() $\varsigma =-$
. Then, since we assumed
$\varsigma =-$
. Then, since we assumed
![]() $\unicode{x3bb} _\mathrm{CE}>e^{ 14 \alpha _\mathrm{BC}}$
in the proof of Proposition 2.2, use [Reference Baladi and SmaniaBS1, Proposition 2.7] that there exist a
$\unicode{x3bb} _\mathrm{CE}>e^{ 14 \alpha _\mathrm{BC}}$
in the proof of Proposition 2.2, use [Reference Baladi and SmaniaBS1, Proposition 2.7] that there exist a
![]() $C^1$
function
$C^1$
function
![]() $\psi _a \colon I \to {\mathbb R}_+$
and
$\psi _a \colon I \to {\mathbb R}_+$
and
![]() $C^\infty $
functions
$C^\infty $
functions
![]() $\Xi ^k_{a,\pm } \colon [0,1] \to [0,1]$
supported in a neighbourhood of
$\Xi ^k_{a,\pm } \colon [0,1] \to [0,1]$
supported in a neighbourhood of
![]() $c_k(a)$
in
$c_k(a)$
in
![]() $T^k_a(U_{k,a,\pm })$
, such that
$T^k_a(U_{k,a,\pm })$
, such that
 $$ \begin{align} h_a(x)=\psi_a(x) + \sum_{k = 1}^{\infty}\sum_{\varsigma\in \{+,-\}}\chi_{k,a}(x) \frac{\Xi^k_{a,\varsigma}(T^{-k}_{a,\varsigma}(x)) \psi_a(T^{-k}_{a,\varsigma}(x)) }{|(T_a^{k})^{\prime}(T^{-k}_{a,\varsigma}(x))|} , \end{align} $$
$$ \begin{align} h_a(x)=\psi_a(x) + \sum_{k = 1}^{\infty}\sum_{\varsigma\in \{+,-\}}\chi_{k,a}(x) \frac{\Xi^k_{a,\varsigma}(T^{-k}_{a,\varsigma}(x)) \psi_a(T^{-k}_{a,\varsigma}(x)) }{|(T_a^{k})^{\prime}(T^{-k}_{a,\varsigma}(x))|} , \end{align} $$
where
![]() $\chi _{k,a}(x)=1_{\pm x<\pm c_k(a)}$
if
$\chi _{k,a}(x)=1_{\pm x<\pm c_k(a)}$
if
![]() $\pm T_a^k$
has a local maximum at c. Setting
$\pm T_a^k$
has a local maximum at c. Setting
![]() $\Psi :=\Xi ^k_{a,\varsigma } \cdot \psi _a$
, we find
$\Psi :=\Xi ^k_{a,\varsigma } \cdot \psi _a$
, we find
![]() $C^1$
functions
$C^1$
functions
![]() $\Psi _{k,\ell }$
for
$\Psi _{k,\ell }$
for
![]() $\ell =1,2,3$
, with
$\ell =1,2,3$
, with
 $$ \begin{align} \frac{\Psi (T^{-k}_{a,\varsigma}(x)) }{|(T_a^{k})^{\prime}(T^{-k}_{a,\varsigma}(x))|} = \frac{\Psi_{k,1}(x)}{|x-c_k(a)|^{1/2} } + \Psi_{k,2}(x) |x-c_k(a)|^{1/2} + \Psi_{k,3}(x) \end{align} $$
$$ \begin{align} \frac{\Psi (T^{-k}_{a,\varsigma}(x)) }{|(T_a^{k})^{\prime}(T^{-k}_{a,\varsigma}(x))|} = \frac{\Psi_{k,1}(x)}{|x-c_k(a)|^{1/2} } + \Psi_{k,2}(x) |x-c_k(a)|^{1/2} + \Psi_{k,3}(x) \end{align} $$
for any
![]() $x\in \operatorname {supp} (\chi _{k,a})$
. Finally, use [Reference SedroSe, Lemmas 11 and 12].
$x\in \operatorname {supp} (\chi _{k,a})$
. Finally, use [Reference SedroSe, Lemmas 11 and 12].
For the second claim, it is convenient to use an alternative decomposition of
![]() $h_a$
. First, recall that [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6] gives a set
$h_a$
. First, recall that [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6] gives a set
![]() $\Omega _{\mathrm {slow}}$
of full measure in the set of mixing CE parameters such that for any
$\Omega _{\mathrm {slow}}$
of full measure in the set of mixing CE parameters such that for any
![]() $\tilde {a} \in \Omega _{\mathrm {slow}}$
and each
$\tilde {a} \in \Omega _{\mathrm {slow}}$
and each
![]() $\kappa _0>1$
, there exist
$\kappa _0>1$
, there exist
![]() $H_0\ge 1$
and a set
$H_0\ge 1$
and a set
![]() $\Delta _0(\tilde {a}, \kappa _0)\subset \Omega _{\mathrm {slow}}$
of
$\Delta _0(\tilde {a}, \kappa _0)\subset \Omega _{\mathrm {slow}}$
of
![]() $(H_0,\kappa _0)$
-polynomially recurrent (and thus transversal) parameters, with
$(H_0,\kappa _0)$
-polynomially recurrent (and thus transversal) parameters, with
![]() $\tilde {a}$
as a Lebesgue density point, such that Proposition 2.5 holds for all
$\tilde {a}$
as a Lebesgue density point, such that Proposition 2.5 holds for all
![]() $a \in \Delta _0$
. (It is unknown whether
$a \in \Delta _0$
. (It is unknown whether
![]() $\tilde a\in \Delta _0$
.) The proof involves constructing a tower for each parameter in
$\tilde a\in \Delta _0$
.) The proof involves constructing a tower for each parameter in
![]() $\Delta _0$
. We claim that, up to reducing the value of
$\Delta _0$
. We claim that, up to reducing the value of
![]() $\epsilon $
in the proof of Proposition 2.2, we can replace
$\epsilon $
in the proof of Proposition 2.2, we can replace
![]() $\tilde a$
by
$\tilde a$
by
![]() $a_{*}$
and
$a_{*}$
and
![]() $\Delta _0$
by
$\Delta _0$
by
![]() $\Omega _{*}(a_{*}, \kappa _0)$
. Indeed,
$\Omega _{*}(a_{*}, \kappa _0)$
. Indeed,
![]() $\Delta _0$
was constructed in [Reference Baladi, Benedicks and SchnellmannBBS, Proposition 2.1], and it suffices to observe that the required uniformity in constants is satisfied by equations (2.5) and (2.8), while [Reference Baladi, Benedicks and SchnellmannBBS, equations (8) and (7)] are exactly [Reference de Melo and van StrienDMS, V.(6.1), V.(6.2) in Proposition V.6.1].
$\Delta _0$
was constructed in [Reference Baladi, Benedicks and SchnellmannBBS, Proposition 2.1], and it suffices to observe that the required uniformity in constants is satisfied by equations (2.5) and (2.8), while [Reference Baladi, Benedicks and SchnellmannBBS, equations (8) and (7)] are exactly [Reference de Melo and van StrienDMS, V.(6.1), V.(6.2) in Proposition V.6.1].
Let then
 $$ \begin{align*} \Pi_a (\hat \psi)(x)=\sum_{j \ge 0, \varsigma \in\pm} \frac{ \unicode{x3bb}^j} {|(T_a^j)^{\prime}(T^{-j}_{a,\varsigma}(x))|} \psi_j(T^{-j}_{a,\varsigma}(x)) \end{align*} $$
$$ \begin{align*} \Pi_a (\hat \psi)(x)=\sum_{j \ge 0, \varsigma \in\pm} \frac{ \unicode{x3bb}^j} {|(T_a^j)^{\prime}(T^{-j}_{a,\varsigma}(x))|} \psi_j(T^{-j}_{a,\varsigma}(x)) \end{align*} $$
(for a suitable
![]() $\unicode{x3bb}>1$
) be the projection from the tower with polynomial recurrence used in [Reference Baladi, Benedicks and SchnellmannBBS], and let
$\unicode{x3bb}>1$
) be the projection from the tower with polynomial recurrence used in [Reference Baladi, Benedicks and SchnellmannBBS], and let
![]() $\hat {\mathcal {L}}_{a}$
be the lift
$\hat {\mathcal {L}}_{a}$
be the lift
![]() $\mathcal {L}_a \Pi _a=\Pi _a \hat {\mathcal {L}}_a$
of the transfer operator
$\mathcal {L}_a \Pi _a=\Pi _a \hat {\mathcal {L}}_a$
of the transfer operator
![]() $\mathcal {L}_a \varphi (x)=\sum _{T_a(y)=x} \varphi (y)/|T^{\prime }_a(y)|$
. Then ([Reference Baladi, Benedicks and SchnellmannBBS, (66)] gives uniform Lasota–Yorke estimates, [Reference Baladi, Benedicks and SchnellmannBBS, Lemmas 3.8, 4.5 and 4.6, Proposition 4.1] give the weak norm bounds needed by Keller and Liverani [Reference Keller and LiveraniKL]), there exist
$\mathcal {L}_a \varphi (x)=\sum _{T_a(y)=x} \varphi (y)/|T^{\prime }_a(y)|$
. Then ([Reference Baladi, Benedicks and SchnellmannBBS, (66)] gives uniform Lasota–Yorke estimates, [Reference Baladi, Benedicks and SchnellmannBBS, Lemmas 3.8, 4.5 and 4.6, Proposition 4.1] give the weak norm bounds needed by Keller and Liverani [Reference Keller and LiveraniKL]), there exist
![]() $C<\infty $
and
$C<\infty $
and
![]() $\theta <1$
such that, letting
$\theta <1$
such that, letting
![]() $\|\cdot \|^{\prime }_a$
be the norm of the Sobolev space
$\|\cdot \|^{\prime }_a$
be the norm of the Sobolev space
![]() $\mathcal {B}_a^{W^1_1}$
of [Reference Baladi, Benedicks and SchnellmannBBS],
$\mathcal {B}_a^{W^1_1}$
of [Reference Baladi, Benedicks and SchnellmannBBS],
where
![]() $\hat h_a$
is the fixed point (the fixed point property determines
$\hat h_a$
is the fixed point (the fixed point property determines
![]() $\hat h_a$
by its value on the level zero of the tower) of
$\hat h_a$
by its value on the level zero of the tower) of
![]() $\hat {\mathcal {L}}_a$
on
$\hat {\mathcal {L}}_a$
on
![]() $\mathcal {B}_a^{W^1_1}$
normalized by
$\mathcal {B}_a^{W^1_1}$
normalized by
![]() $\int \Pi _a \hat h_a \, dm = 1$
, while
$\int \Pi _a \hat h_a \, dm = 1$
, while
![]() $\hat \nu $
, the non-negative measure whose density with respect to Lebesgue in the level j of the tower is
$\hat \nu $
, the non-negative measure whose density with respect to Lebesgue in the level j of the tower is
![]() $\unicode{x3bb} ^j$
, is the fixed point of the dual of
$\unicode{x3bb} ^j$
, is the fixed point of the dual of
![]() $\hat {\mathcal {L}}_a$
(see [Reference Baladi and SmaniaBS1, equation (85)], note that
$\hat {\mathcal {L}}_a$
(see [Reference Baladi and SmaniaBS1, equation (85)], note that
![]() $\nu (\hat h_a)=1$
is automatic). Since
$\nu (\hat h_a)=1$
is automatic). Since
![]() $\Pi _a\hat h_a=h_a$
and the
$\Pi _a\hat h_a=h_a$
and the
![]() $W^1_1$
norm dominates any
$W^1_1$
norm dominates any
![]() $H^s_q$
norm on I if
$H^s_q$
norm on I if
![]() $s\in [0,1)$
and
$s\in [0,1)$
and
![]() ${1< q<1/s}$
(by the Sobolev embedding, more precisely [Reference Runst and SickelRS, Ch. 2], the bounded inclusions
${1< q<1/s}$
(by the Sobolev embedding, more precisely [Reference Runst and SickelRS, Ch. 2], the bounded inclusions
![]() $ W^1_1 \subset W^\sigma _1=F^{\sigma }_{1,1}\subset F^{\sigma }_{1,2}\subset F^{s}_{q,2}=H^s_q$
, if
$ W^1_1 \subset W^\sigma _1=F^{\sigma }_{1,1}\subset F^{\sigma }_{1,2}\subset F^{s}_{q,2}=H^s_q$
, if
![]() $\sigma =1+s-1/q\in (0,1)$
and
$\sigma =1+s-1/q\in (0,1)$
and
![]() $q\in (1,\infty )$
), the decomposition in equation (2.40) combined with the uniform bound in equation (2.41) (for
$q\in (1,\infty )$
), the decomposition in equation (2.40) combined with the uniform bound in equation (2.41) (for
![]() $\hat \varphi $
vanishing on all levels
$\hat \varphi $
vanishing on all levels
![]() $\geq 1$
and constant on level zero of the tower, with
$\geq 1$
and constant on level zero of the tower, with
![]() $\hat \nu (\varphi )=1$
) gives the second claim of the sublemma, using again [Reference SedroSe, Lemmas 11 and 12].
$\hat \nu (\varphi )=1$
) gives the second claim of the sublemma, using again [Reference SedroSe, Lemmas 11 and 12].
Proof of Proposition 2.5
Recall from the proof of Proposition 2.2 that we have
![]() ${\unicode{x3bb} _\mathrm{CE}>e^{14 \alpha _\mathrm{BC}}}$
. By mollification, it is enough to prove both bounds for
${\unicode{x3bb} _\mathrm{CE}>e^{14 \alpha _\mathrm{BC}}}$
. By mollification, it is enough to prove both bounds for
![]() $C^1$
functions
$C^1$
functions
![]() $\varphi $
. It is in fact enough to show the first bound for
$\varphi $
. It is in fact enough to show the first bound for
![]() $\varphi \in C^1$
as follows. Indeed, again by mollification (see e.g. the proof of [Reference SedroSe, Lemma 14]), if the first bound holds for
$\varphi \in C^1$
as follows. Indeed, again by mollification (see e.g. the proof of [Reference SedroSe, Lemma 14]), if the first bound holds for
![]() $\varphi \in C^1$
, then it holds for any
$\varphi \in C^1$
, then it holds for any
![]() $\varphi \in H^s_q(I)$
with
$\varphi \in H^s_q(I)$
with
![]() $q>1$
and
$q>1$
and
![]() $s>0$
. Therefore, since the density
$s>0$
. Therefore, since the density
![]() $h_a$
of
$h_a$
of
![]() $\mu _a$
lies in
$\mu _a$
lies in
![]() $H^s_q(I)$
for all
$H^s_q(I)$
for all
![]() $s\in (0,1/2)$
and
$s\in (0,1/2)$
and
![]() $q \in (1, 2/(1+2s))$
by Sublemma 2.7 (with norm uniformly bounded in a), the second bound follows from the first bound for
$q \in (1, 2/(1+2s))$
by Sublemma 2.7 (with norm uniformly bounded in a), the second bound follows from the first bound for
![]() $\varphi \in C^1$
(using that
$\varphi \in C^1$
(using that
![]() $C^1$
functions are bounded multipliers on
$C^1$
functions are bounded multipliers on
![]() $H^s_q$
).
$H^s_q$
).
Next, we observed in the proof of Sublemma 2.7 that we can replace the set called
![]() $\Delta _0$
in [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6] by
$\Delta _0$
in [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6] by
![]() $\Omega _{*}(a_{*}, \kappa _0)$
. The first bound for Lipschitz continuous
$\Omega _{*}(a_{*}, \kappa _0)$
. The first bound for Lipschitz continuous
![]() $\varphi $
thus follows from the second assertion of [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6], since
$\varphi $
thus follows from the second assertion of [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6], since
![]() $\Omega _{*}\subset [a_{\mathrm {mix}}, 4)$
. Indeed, note first that a is topologically mixing if and only if its renormalization period
$\Omega _{*}\subset [a_{\mathrm {mix}}, 4)$
. Indeed, note first that a is topologically mixing if and only if its renormalization period
![]() $P_{a}$
is equal to one. Second, observe that the constant
$P_{a}$
is equal to one. Second, observe that the constant
![]() $C_{\varphi , \psi }$
in the second claim of [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6] can be replaced by
$C_{\varphi , \psi }$
in the second claim of [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6] can be replaced by
![]() $C \|\varphi \|_\varpi \|\psi \|_{L^1(d\mu _a)}$
for a constant C uniform in a in view of [Reference Baladi, Benedicks and SchnellmannBBS, Lemmas 4.5 and 4.6] and the principle of uniform boundedness. More precisely, using the notation from the proof of Sublemma 2.7, we have
$C \|\varphi \|_\varpi \|\psi \|_{L^1(d\mu _a)}$
for a constant C uniform in a in view of [Reference Baladi, Benedicks and SchnellmannBBS, Lemmas 4.5 and 4.6] and the principle of uniform boundedness. More precisely, using the notation from the proof of Sublemma 2.7, we have
 $$ \begin{align*} &\int (\psi \circ T_a^n) \varphi \, dm = \int \psi \Pi_a (\hat {\mathcal{L}}_a^n(\hat \varphi)) \, dm \quad \mbox{if } \Pi_a(\hat \varphi)=\varphi , \\ & \int (\psi \circ T_a^n) \varphi h_a \, dm= \int \psi \Pi_a(\hat {\mathcal{L}}_a^n(\hat \varphi_a)) \, dm \quad\mbox{if } \Pi_a(\hat \varphi_a)=\varphi h_a. \end{align*} $$
$$ \begin{align*} &\int (\psi \circ T_a^n) \varphi \, dm = \int \psi \Pi_a (\hat {\mathcal{L}}_a^n(\hat \varphi)) \, dm \quad \mbox{if } \Pi_a(\hat \varphi)=\varphi , \\ & \int (\psi \circ T_a^n) \varphi h_a \, dm= \int \psi \Pi_a(\hat {\mathcal{L}}_a^n(\hat \varphi_a)) \, dm \quad\mbox{if } \Pi_a(\hat \varphi_a)=\varphi h_a. \end{align*} $$
Since
![]() $\Pi _a\hat h_a=h_a$
, any Lipschitz continuous
$\Pi _a\hat h_a=h_a$
, any Lipschitz continuous
![]() $\varphi $
can be written as
$\varphi $
can be written as
![]() $\Pi _a (\hat \varphi )$
(take
$\Pi _a (\hat \varphi )$
(take
![]() $\hat \varphi _0= \varphi $
on the level zero and
$\hat \varphi _0= \varphi $
on the level zero and
![]() $\hat \varphi _j\equiv 0$
on levels
$\hat \varphi _j\equiv 0$
on levels
![]() $j\ge 1$
) such that, on the one hand,
$j\ge 1$
) such that, on the one hand,
![]() $\|\hat \varphi \|_a^{\prime }\le C\|\varphi \|_1$
uniformly in a, and, on the other hand,
$\|\hat \varphi \|_a^{\prime }\le C\|\varphi \|_1$
uniformly in a, and, on the other hand,
![]() $\hat \nu (\hat \varphi ) = \int \varphi \, dm$
. We conclude by applying equation (2.41) from the proof of Sublemma 2.7. (The Banach space of [Reference Baladi, Benedicks and SchnellmannBBS] requires that the function on level zero of the tower be supported in
$\hat \nu (\hat \varphi ) = \int \varphi \, dm$
. We conclude by applying equation (2.41) from the proof of Sublemma 2.7. (The Banach space of [Reference Baladi, Benedicks and SchnellmannBBS] requires that the function on level zero of the tower be supported in
![]() $(0,1)$
, so this proof cannot cover the case
$(0,1)$
, so this proof cannot cover the case
![]() $a=4$
.)
$a=4$
.)
Proof of Proposition 2.6
If
![]() $a=a_{*}$
, the bound is an immediate consequence of the first claim of [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6], since we can replace the set denoted
$a=a_{*}$
, the bound is an immediate consequence of the first claim of [Reference Baladi, Benedicks and SchnellmannBBS, Corollary 1.6], since we can replace the set denoted
![]() $\Delta _0$
there by
$\Delta _0$
there by
![]() $\Omega _{*}(a_{*}, \kappa _0)$
, as observed in the proof of Sublemma 2.7 and used in the proof of Proposition 2.5. If
$\Omega _{*}(a_{*}, \kappa _0)$
, as observed in the proof of Sublemma 2.7 and used in the proof of Proposition 2.5. If
![]() $a\ne a_{*}$
, the uniformity of the constants given by Proposition 2.2 ensures that we may construct the reference tower in [Reference Baladi, Benedicks and SchnellmannBBS] at a (instead of
$a\ne a_{*}$
, the uniformity of the constants given by Proposition 2.2 ensures that we may construct the reference tower in [Reference Baladi, Benedicks and SchnellmannBBS] at a (instead of
![]() $a_{*}$
), viewing
$a_{*}$
), viewing
![]() $a^{\prime }$
as a perturbation of a.
$a^{\prime }$
as a perturbation of a.
2.5 Hölder regularity of the variance
 $\sigma _a(\varphi )$
$\sigma _a(\varphi )$
Propositions 2.5 and 2.6 will imply the following regularity of
![]() $a\mapsto \sigma _a(\varphi )$
on
$a\mapsto \sigma _a(\varphi )$
on
![]() $\Omega _{*}$
.
$\Omega _{*}$
.
Lemma 2.8. (Regularity of
 $\sigma _a(\varphi )$
)
$\sigma _a(\varphi )$
)
For any
![]() $\varpi \in (0,1]$
, there exist
$\varpi \in (0,1]$
, there exist
![]() $\theta \in (0,\operatorname \min \{1/2, \varpi \})$
and
$\theta \in (0,\operatorname \min \{1/2, \varpi \})$
and
![]() $C<\infty $
such that for each
$C<\infty $
such that for each
![]() $\varphi \in C^\varpi $
with
$\varphi \in C^\varpi $
with
![]() $\sigma _{a_{*}}(\varphi )>0$
, there exists
$\sigma _{a_{*}}(\varphi )>0$
, there exists
![]() $\epsilon _\varphi>0$
such that
$\epsilon _\varphi>0$
such that
and such that for all
![]() $a,a^{\prime }\in \Omega _{*}(a_{*},\kappa _0)\cap [a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
, we have
$a,a^{\prime }\in \Omega _{*}(a_{*},\kappa _0)\cap [a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
, we have
Proof. Let
![]() $k_0>1$
be a large integer to be chosen at the end of the proof. By the second claim of Proposition 2.5, there exist
$k_0>1$
be a large integer to be chosen at the end of the proof. By the second claim of Proposition 2.5, there exist
![]() $\rho =\rho _\varpi <1$
and
$\rho =\rho _\varpi <1$
and
![]() $C_0$
such that
$C_0$
such that
 $$ \begin{align*} &\sum_{k> k_0} \bigg| \int \bigg( \varphi-\int\varphi \, d\mu_{a} \bigg) \cdot \bigg( \bigg( \varphi - \int\varphi \, d\mu_{a} \bigg) \circ T_{ a}^k \bigg) \, d\mu_{a} \bigg| \\ &\quad\le C_0 \|\varphi\|_\varpi^2 \cdot \frac{\rho^{k_0}}{1-\rho} \quad \text{for all } k_0\ge 1, \text{ for all } a \in \Omega_{a_{*}} , \text{ for all } \varphi \in C^\varpi. \end{align*} $$
$$ \begin{align*} &\sum_{k> k_0} \bigg| \int \bigg( \varphi-\int\varphi \, d\mu_{a} \bigg) \cdot \bigg( \bigg( \varphi - \int\varphi \, d\mu_{a} \bigg) \circ T_{ a}^k \bigg) \, d\mu_{a} \bigg| \\ &\quad\le C_0 \|\varphi\|_\varpi^2 \cdot \frac{\rho^{k_0}}{1-\rho} \quad \text{for all } k_0\ge 1, \text{ for all } a \in \Omega_{a_{*}} , \text{ for all } \varphi \in C^\varpi. \end{align*} $$
Set
![]() $A_a=\int \varphi \, d\mu _a$
. Since
$A_a=\int \varphi \, d\mu _a$
. Since
![]() $\int ((\varphi -A_a)\circ T_a^k )(\varphi -A_a) \, d\mu _a = \int (\varphi \circ T_a^k) \varphi \, d\mu _a -A_a^2$
, we have
$\int ((\varphi -A_a)\circ T_a^k )(\varphi -A_a) \, d\mu _a = \int (\varphi \circ T_a^k) \varphi \, d\mu _a -A_a^2$
, we have
 $$ \begin{align*} |\sigma_a(\varphi)^2-\sigma_{a^{\prime}}(\varphi)^2| &\leq 2 \sum_{k=0}^{k_0-1} \bigg |\int\varphi(\varphi\circ T_a^k) \,d\mu_a - \int\varphi(\varphi\circ T_{a^{\prime}}^k) \, d\mu_{a} \bigg | \\ &\quad + 2\sum_{k=0}^{k_0-1} \bigg |\int\varphi(\varphi\circ T_{a^{\prime}}^k) \, d\mu_a - \int\varphi(\varphi\circ T_{a^{\prime}}^k) \, d\mu_{a^{\prime}} \bigg | \\ &\quad + 2\sum_{k=0}^{k_0-1}\bigg | \bigg( \int \varphi \, d\mu_a \bigg)^2 - \bigg( \int \varphi \, d\mu_{a^{\prime}} \bigg)^2 \bigg | \\ &\quad + 4 C_0 \|\varphi\|^2_\varpi\frac{\rho^{k_0}}{1-\rho} \quad \text{for all } k_0\ge 1. \end{align*} $$
$$ \begin{align*} |\sigma_a(\varphi)^2-\sigma_{a^{\prime}}(\varphi)^2| &\leq 2 \sum_{k=0}^{k_0-1} \bigg |\int\varphi(\varphi\circ T_a^k) \,d\mu_a - \int\varphi(\varphi\circ T_{a^{\prime}}^k) \, d\mu_{a} \bigg | \\ &\quad + 2\sum_{k=0}^{k_0-1} \bigg |\int\varphi(\varphi\circ T_{a^{\prime}}^k) \, d\mu_a - \int\varphi(\varphi\circ T_{a^{\prime}}^k) \, d\mu_{a^{\prime}} \bigg | \\ &\quad + 2\sum_{k=0}^{k_0-1}\bigg | \bigg( \int \varphi \, d\mu_a \bigg)^2 - \bigg( \int \varphi \, d\mu_{a^{\prime}} \bigg)^2 \bigg | \\ &\quad + 4 C_0 \|\varphi\|^2_\varpi\frac{\rho^{k_0}}{1-\rho} \quad \text{for all } k_0\ge 1. \end{align*} $$
Assume for a moment that
![]() $\varpi \ge 1/2$
. The
$\varpi \ge 1/2$
. The
![]() $\varpi $
-Hölder constant of
$\varpi $
-Hölder constant of
![]() $\varphi (\varphi \circ T_{\bar a}^k)$
(for
$\varphi (\varphi \circ T_{\bar a}^k)$
(for
![]() $\bar a=a$
or
$\bar a=a$
or
![]() $a^{\prime }$
) is bounded by
$a^{\prime }$
) is bounded by
![]() $\Lambda ^{ k}\|\varphi \|^2_\varpi $
. Thus, Proposition 2.6 gives for any
$\Lambda ^{ k}\|\varphi \|^2_\varpi $
. Thus, Proposition 2.6 gives for any
![]() $\Theta <1/2$
a constant
$\Theta <1/2$
a constant
![]() $C_1=C_1(\Theta )$
such that for
$C_1=C_1(\Theta )$
such that for
![]() $a, a^{\prime } \in \Omega _{a_{*}}$
and
$a, a^{\prime } \in \Omega _{a_{*}}$
and
![]() $\varphi \in C^\varpi $
,
$\varphi \in C^\varpi $
,
 $$ \begin{align*} &|\sigma_a(\varphi)^2-\sigma_{a^{\prime}}(\varphi)^2| \leq k_0 C_1 \|\varphi\|^2_\varpi \Lambda^{ k_0}|a-a^{\prime}|^\Theta + C_0 \|\varphi\|^2_\varpi \frac{\rho^{k_0}}{1-\rho}\\ &\quad+ 2 \sum_{k=0}^{k_0-1} \bigg |\int\varphi(\varphi\circ T_a^k) \, d\mu_a - \int\varphi(\varphi\circ T_{a^{\prime}}^k) \, d\mu_{a} \bigg |\quad \text{for all } k_0\geq 1. \end{align*} $$
$$ \begin{align*} &|\sigma_a(\varphi)^2-\sigma_{a^{\prime}}(\varphi)^2| \leq k_0 C_1 \|\varphi\|^2_\varpi \Lambda^{ k_0}|a-a^{\prime}|^\Theta + C_0 \|\varphi\|^2_\varpi \frac{\rho^{k_0}}{1-\rho}\\ &\quad+ 2 \sum_{k=0}^{k_0-1} \bigg |\int\varphi(\varphi\circ T_a^k) \, d\mu_a - \int\varphi(\varphi\circ T_{a^{\prime}}^k) \, d\mu_{a} \bigg |\quad \text{for all } k_0\geq 1. \end{align*} $$
Next, equation (2.2) gives that
Therefore, we find
 $$ \begin{align} |\sigma_a(\varphi)^2-\sigma_{a^{\prime}}(\varphi)^2 | &\leq k_0 C_1 \|\varphi\|^2_\varpi \Lambda^{ k_0}|a-a^{\prime}|^\Theta + 4 C_0 \|\varphi\|^2_\varpi \frac{\rho^{k_0}}{1-\rho}\nonumber\\ &\quad+ k_0 \|\varphi\|_{\varpi} ( C\Lambda^{k_0}|a-a^{\prime}|)^{\varpi}. \end{align} $$
$$ \begin{align} |\sigma_a(\varphi)^2-\sigma_{a^{\prime}}(\varphi)^2 | &\leq k_0 C_1 \|\varphi\|^2_\varpi \Lambda^{ k_0}|a-a^{\prime}|^\Theta + 4 C_0 \|\varphi\|^2_\varpi \frac{\rho^{k_0}}{1-\rho}\nonumber\\ &\quad+ k_0 \|\varphi\|_{\varpi} ( C\Lambda^{k_0}|a-a^{\prime}|)^{\varpi}. \end{align} $$
We conclude the proof for
![]() $\varpi \ge 1/2$
by dividing equation (2.43) by
$\varpi \ge 1/2$
by dividing equation (2.43) by
![]() $|a-a^{\prime }|^{\theta }$
for small enough
$|a-a^{\prime }|^{\theta }$
for small enough
![]() $\theta>0$
and optimizing in
$\theta>0$
and optimizing in
![]() $k_0$
, using also
$k_0$
, using also
![]() $(\sigma _a - \sigma _{a^{\prime }}) (\sigma _a + \sigma _{a^{\prime }}) = \sigma _a^2 - \sigma _{a^{\prime }}^2$
.
$(\sigma _a - \sigma _{a^{\prime }}) (\sigma _a + \sigma _{a^{\prime }}) = \sigma _a^2 - \sigma _{a^{\prime }}^2$
.
If
![]() $\varpi \in (0,1/2)$
, mollification gives
$\varpi \in (0,1/2)$
, mollification gives
![]() $\varphi _\upsilon \in C^{1/2}$
and
$\varphi _\upsilon \in C^{1/2}$
and
![]() $C_4$
such that
$C_4$
such that
for all small
![]() $\upsilon>0$
, all
$\upsilon>0$
, all
![]() $0\le k \le k_0$
and all
$0\le k \le k_0$
and all
![]() $\bar a \in \Omega _{a_{*}}$
. To conclude, optimize in
$\bar a \in \Omega _{a_{*}}$
. To conclude, optimize in
![]() ${\upsilon =|a-a^{\prime }|^{\theta _0}}$
for small
${\upsilon =|a-a^{\prime }|^{\theta _0}}$
for small
![]() $\theta _0>0$
, taking
$\theta _0>0$
, taking
![]() $\theta $
smaller (in particular
$\theta $
smaller (in particular
![]() $\theta <\varpi \theta _0$
).
$\theta <\varpi \theta _0$
).
3 Switching locally from the parameter to the phase space
Let
![]() $a_{*}$
,
$a_{*}$
,
![]() $\mathcal {P}_j(a_{*},\kappa _0)$
and
$\mathcal {P}_j(a_{*},\kappa _0)$
and
![]() $\Omega _{*}=\Omega _{*}(a_{*},\kappa _0)$
be as in Proposition 2.2 for
$\Omega _{*}=\Omega _{*}(a_{*},\kappa _0)$
be as in Proposition 2.2 for
![]() $\kappa _0 \ge 11 / (3d_1)$
, and fix
$\kappa _0 \ge 11 / (3d_1)$
, and fix
![]() $\varpi \in (0,1)$
. This section is devoted to Proposition 3.2, the main estimate (analogous to [Reference SchnellmannSch, Proposition 5.1]) towards a law of large numbers for the squares of the blocks which will be defined in §4 (see Lemma 4.2).
$\varpi \in (0,1)$
. This section is devoted to Proposition 3.2, the main estimate (analogous to [Reference SchnellmannSch, Proposition 5.1]) towards a law of large numbers for the squares of the blocks which will be defined in §4 (see Lemma 4.2).
From now on, fix
![]() $\varpi \in (0,1)$
and a
$\varpi \in (0,1)$
and a
![]() $\varpi $
-Hölder continuous function
$\varpi $
-Hölder continuous function
![]() $\varphi \colon I\to {\mathbb R}$
, recalling
$\varphi \colon I\to {\mathbb R}$
, recalling
![]() $\varphi _a$
,
$\varphi _a$
,
![]() $\sigma _a(\varphi )$
from equations (1.6) and (1.3), and assume
$\sigma _a(\varphi )$
from equations (1.6) and (1.3), and assume
![]() $\sigma _{a_{*}}(\varphi )>0$
. Lemma 2.8 gives
$\sigma _{a_{*}}(\varphi )>0$
. Lemma 2.8 gives
![]() $\epsilon _\varphi>0$
such that
$\epsilon _\varphi>0$
such that
If
![]() $\epsilon _\varphi <\epsilon $
, we replace
$\epsilon _\varphi <\epsilon $
, we replace
![]() $\Omega _{*}$
by
$\Omega _{*}$
by
![]() $\Omega _{*}^\varphi $
by replacing
$\Omega _{*}^\varphi $
by replacing
![]() $\epsilon $
in the proof of Proposition 2.2 with
$\epsilon $
in the proof of Proposition 2.2 with
![]() $\epsilon _\varphi $
. (This is harmless as it can only improve the constants.)
$\epsilon _\varphi $
. (This is harmless as it can only improve the constants.)
Remark 3.1. (
 $\theta $
-Hölder–Whitney extensions of
$\theta $
-Hölder–Whitney extensions of
 $\varphi _a$
and
$\varphi _a$
and
 $\xi _n(a)$
)
$\xi _n(a)$
)
By Proposition 2.6, the function
![]() $a \mapsto \int \varphi \, d \mu _a$
is
$a \mapsto \int \varphi \, d \mu _a$
is
![]() $\Theta $
-Hölder continuous on
$\Theta $
-Hölder continuous on
![]() $\Omega _{*}$
for any
$\Omega _{*}$
for any
![]() $\Theta <1/2$
. By Lemma 2.8, the function
$\Theta <1/2$
. By Lemma 2.8, the function
![]() $a \mapsto \sigma _a(\varphi )\ge 0$
is
$a \mapsto \sigma _a(\varphi )\ge 0$
is
![]() $\theta $
-Hölder continuous on
$\theta $
-Hölder continuous on
![]() $\Omega _{*}$
for some
$\Omega _{*}$
for some
![]() $\theta <\operatorname \min \{1/2, \varpi \}$
, and uniformly bounded away from zero on
$\theta <\operatorname \min \{1/2, \varpi \}$
, and uniformly bounded away from zero on
![]() $\Omega _{*}^\varphi $
. Taking
$\Omega _{*}^\varphi $
. Taking
![]() $\Theta \ge \theta $
, the map
$\Theta \ge \theta $
, the map
![]() $a \mapsto \varphi _a(u) = (\varphi (u)-\int \varphi d\mu _a)/\sigma _a$
is
$a \mapsto \varphi _a(u) = (\varphi (u)-\int \varphi d\mu _a)/\sigma _a$
is
![]() $\theta $
-Hölder continuous on
$\theta $
-Hölder continuous on
![]() $\Omega _{*}^\varphi $
uniformly in
$\Omega _{*}^\varphi $
uniformly in
![]() $u\in I$
. By the Whitney extension theorem, we extend each map
$u\in I$
. By the Whitney extension theorem, we extend each map
![]() $a \mapsto \varphi _a(u)$
to a
$a \mapsto \varphi _a(u)$
to a
![]() $\theta $
-Hölder continuous map on
$\theta $
-Hölder continuous map on
![]() $[a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
, uniformly in
$[a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
, uniformly in
![]() $u\in I$
. In addition, there exists
$u\in I$
. In addition, there exists
![]() $\widetilde C < \infty $
such that
$\widetilde C < \infty $
such that
Then, using equation (2.2), we may extend each map
![]() $a \mapsto \xi _n(a)=\varphi _a(T_a^{n+1}(c))$
to a
$a \mapsto \xi _n(a)=\varphi _a(T_a^{n+1}(c))$
to a
![]() $\theta $
-Hölder continuous map on
$\theta $
-Hölder continuous map on
![]() $[a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
, with
$[a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
, with
![]() $\theta $
-Hölder constant bounded by
$\theta $
-Hölder constant bounded by
![]() $C\Lambda ^{\theta (n+1)}$
. Indeed, recalling
$C\Lambda ^{\theta (n+1)}$
. Indeed, recalling
![]() $x_n(a) = T_a^{n+1}(c)$
, just decompose
$x_n(a) = T_a^{n+1}(c)$
, just decompose
Fix
![]() $\alpha \in (0,1)$
such that (in view of the use of equation (2.30) in Corollary 3.4)
$\alpha \in (0,1)$
such that (in view of the use of equation (2.30) in Corollary 3.4)
Fix
![]() $q>1$
and
$q>1$
and
![]() $0<s<\operatorname \min \{\varpi , 1/q\}$
, and let
$0<s<\operatorname \min \{\varpi , 1/q\}$
, and let
where
![]() $\unicode{x3bb} _\mathrm{CE}>1$
is given by equation (2.5), while
$\unicode{x3bb} _\mathrm{CE}>1$
is given by equation (2.5), while
![]() $\rho =\operatorname \max \{\rho ^s_q, \rho _\varpi \}<1$
is given by Proposition 2.5, and
$\rho =\operatorname \max \{\rho ^s_q, \rho _\varpi \}<1$
is given by Proposition 2.5, and
![]() $\theta \in (0,\operatorname \min \{1/2,\varpi \})$
is given by Lemma 2.8. Finally, recalling
$\theta \in (0,\operatorname \min \{1/2,\varpi \})$
is given by Lemma 2.8. Finally, recalling
![]() $\Lambda $
from equation (2.1), let
$\Lambda $
from equation (2.1), let
![]() $\eta \in (0,1/2)$
be so small that
$\eta \in (0,1/2)$
be so small that

Define the expectation
![]() $E(\psi )$
of
$E(\psi )$
of
![]() $\psi \in L^\infty (\Omega _{*}^\varphi )$
by
$\psi \in L^\infty (\Omega _{*}^\varphi )$
by
 $$ \begin{align} E(\psi):= \frac{1}{m (\Omega_{*}^\varphi)} \int_{\Omega_{*}^\varphi} \psi \, dm. \end{align} $$
$$ \begin{align} E(\psi):= \frac{1}{m (\Omega_{*}^\varphi)} \int_{\Omega_{*}^\varphi} \psi \, dm. \end{align} $$
(We restrict to the Cantor set
![]() $\Omega _{*}^\varphi $
here and thus in equation (3.8). The bound in equation (2.9) is used in the proof of equation (3.8) (but not for equation (3.9), Lemma 3.3 or Corollary 3.4).) The following result is the key estimate on
$\Omega _{*}^\varphi $
here and thus in equation (3.8). The bound in equation (2.9) is used in the proof of equation (3.8) (but not for equation (3.9), Lemma 3.3 or Corollary 3.4).) The following result is the key estimate on
![]() $\xi _j(a)=\varphi _a (T_a^{j+1}(c))$
.
$\xi _j(a)=\varphi _a (T_a^{j+1}(c))$
.
Proposition 3.2. There exist
![]() $C_\varphi <\infty $
and
$C_\varphi <\infty $
and
![]() $K<\infty $
such that
$K<\infty $
such that
 $$ \begin{align} \bigg| E \bigg(\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 -n \bigg| \leq C_\varphi \quad \text{for all } k \geq \operatorname\max \{2 K, [2/\eta]\}, \text{ for all } 1\leq n\leq \eta k/2, \end{align} $$
$$ \begin{align} \bigg| E \bigg(\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 -n \bigg| \leq C_\varphi \quad \text{for all } k \geq \operatorname\max \{2 K, [2/\eta]\}, \text{ for all } 1\leq n\leq \eta k/2, \end{align} $$
and, setting (the stretched exponent
![]() $1/4$
for
$1/4$
for
![]() $v(k)$
and the lower bound can be replaced by any number in
$v(k)$
and the lower bound can be replaced by any number in
![]() $(0,1)$
, without changing the statements, up to adjusting intermediate constants)
$(0,1)$
, without changing the statements, up to adjusting intermediate constants)
![]() $v(k) = [k - k^{1/4}]$
for every non-trivial interval
$v(k) = [k - k^{1/4}]$
for every non-trivial interval
![]() $\omega \subset \tilde \omega \in \mathcal {P}_{v(k)}$
with
$\omega \subset \tilde \omega \in \mathcal {P}_{v(k)}$
with
![]() ${\omega \cap \Omega _{*}^\varphi \ne \emptyset} $
and
${\omega \cap \Omega _{*}^\varphi \ne \emptyset} $
and
![]() $\unicode{x3bb} _0^{-k^{1/4}} \leq |x_{v(k)} (\omega )| \leq {v(k)}^{-3/\alpha }$
, we have
$\unicode{x3bb} _0^{-k^{1/4}} \leq |x_{v(k)} (\omega )| \leq {v(k)}^{-3/\alpha }$
, we have
 $$ \begin{align} \bigg| \frac 1 {|\omega|} \int_\omega \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, dm - n \bigg| \leq C_\varphi \quad \text{for all } k \geq [2/\eta], \text{ for all } 1 \leq n \leq \eta k / 2 , \end{align} $$
$$ \begin{align} \bigg| \frac 1 {|\omega|} \int_\omega \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, dm - n \bigg| \leq C_\varphi \quad \text{for all } k \geq [2/\eta], \text{ for all } 1 \leq n \leq \eta k / 2 , \end{align} $$
and, for any sequence
![]() $\Psi _k$
with
$\Psi _k$
with
![]() $C_\Psi :=\sup _k k^{-8/3}\sup |\Psi _k|<\infty $
and for any refinement
$C_\Psi :=\sup _k k^{-8/3}\sup |\Psi _k|<\infty $
and for any refinement
![]() $\mathcal {Q}_{v(k)}$
of
$\mathcal {Q}_{v(k)}$
of
![]() $\mathcal {P}_{v(k)}$
such that
$\mathcal {P}_{v(k)}$
such that
![]() $\unicode{x3bb} _0^{-k^{1/4}} \leq |x_v (\omega )| \leq v^{-3/\alpha }$
for all
$\unicode{x3bb} _0^{-k^{1/4}} \leq |x_v (\omega )| \leq v^{-3/\alpha }$
for all
![]() $\omega \in \mathcal {Q}_{v(k)}$
(we have
$\omega \in \mathcal {Q}_{v(k)}$
(we have
![]() $\unicode{x3bb} _0^{-k^{1/4}} \leq |x_v (\omega )|$
for all
$\unicode{x3bb} _0^{-k^{1/4}} \leq |x_v (\omega )|$
for all
![]() $\omega \in \mathcal {P}_{v(k)}$
by equation (3.21)), setting
$\omega \in \mathcal {P}_{v(k)}$
by equation (3.21)), setting
 $$ \begin{align} \mathcal {Q}_{*,v(k)}:=\{\omega \in \mathcal {Q}_{v(k)} \mid \omega\cap \Omega_{*}^\varphi \ne \emptyset \} , \quad \Omega_{*,v(k)}^{\mathcal {Q}}=\bigcup_{\omega \in \mathcal {Q}_{*,v(k)}}\omega, \end{align} $$
$$ \begin{align} \mathcal {Q}_{*,v(k)}:=\{\omega \in \mathcal {Q}_{v(k)} \mid \omega\cap \Omega_{*}^\varphi \ne \emptyset \} , \quad \Omega_{*,v(k)}^{\mathcal {Q}}=\bigcup_{\omega \in \mathcal {Q}_{*,v(k)}}\omega, \end{align} $$
we have
 $$ \begin{align} \bigg| E( \Psi_k) - \frac{1}{|\Omega_{*,v(k)}^{\mathcal {Q}}|} \int_{\Omega_{*,v(k)}^{\mathcal {Q}}} \Psi_k\, dm \bigg| \leq C_\Psi C_\varphi \quad \text{for all } k \geq [2/\eta]. \end{align} $$
$$ \begin{align} \bigg| E( \Psi_k) - \frac{1}{|\Omega_{*,v(k)}^{\mathcal {Q}}|} \int_{\Omega_{*,v(k)}^{\mathcal {Q}}} \Psi_k\, dm \bigg| \leq C_\Psi C_\varphi \quad \text{for all } k \geq [2/\eta]. \end{align} $$
Proposition 3.2 is proved in §3.1. Like for its analogue [Reference SchnellmannSch, Proposition 5.1], the first step will be to show the local estimate in equation (3.9) using Lemma 3.3 through its Corollary 3.4 (the analogues of [Reference SchnellmannSch, Lemma 5.3, Corollary 5.5]).
Lemma 3.3. (Switching locally from parameter to phase space)
Fix
![]() $\ell _0 \in \{1,2,3, 4\}$
. There exists
$\ell _0 \in \{1,2,3, 4\}$
. There exists
![]() $C<\infty $
such that we have, for any integers
$C<\infty $
such that we have, for any integers
for every
![]() $\tilde {\omega } \in \mathcal {P}_n$
and each non-trivial interval
$\tilde {\omega } \in \mathcal {P}_n$
and each non-trivial interval
![]() $\omega \subset \tilde {\omega }$
with
$\omega \subset \tilde {\omega }$
with
![]() $\omega \cap \Omega _{*}^\varphi \ne \emptyset $
,
$\omega \cap \Omega _{*}^\varphi \ne \emptyset $
,

Corollary 3.4. There exists
![]() $C_3>1$
such that for
$C_3>1$
such that for
![]() $\ell _0$
, n,
$\ell _0$
, n,
![]() $ n_1, \ldots , n_{\ell _0}$
, and
$ n_1, \ldots , n_{\ell _0}$
, and
![]() $\omega $
as in Lemma 3.3, if, in addition,
$\omega $
as in Lemma 3.3, if, in addition,
![]() $ |x_n (\omega )| \le n^{-3/\alpha }$
, then for any
$ |x_n (\omega )| \le n^{-3/\alpha }$
, then for any
![]() $a_0 \in \omega \cap \Omega _{*}^\varphi $
,
$a_0 \in \omega \cap \Omega _{*}^\varphi $
,

Proof. Since equation (3.4) implies equation (2.30) for
![]() $\omega $
, the change of variables
$\omega $
, the change of variables
![]() ${y = x_n (a)}$
on
${y = x_n (a)}$
on
![]() $\omega $
, combined with the distortion estimate in equation (2.31), gives
$\omega $
, combined with the distortion estimate in equation (2.31), gives
 $$ \begin{align} \nonumber &\bigg| \frac1{|\omega|} \int_{\omega } \prod_{\ell=1}^{\ell_0} \xi_{n_\ell}(a)\, da-\frac{1}{|x_n (\omega )|} \int_{x_n (\omega)} \prod_{\ell=1}^{\ell_0} \xi_{n_\ell}(x_n|_\omega^{-1} (y)) \, dy \bigg| \\ &\quad= \frac{1}{|x_n (\omega)|} \bigg| \int_{x_n (\omega)} \prod_{\ell=1}^{\ell_0} \xi_{n_\ell}(x_n|_\omega^{-1} (y)) \bigg( \frac 1{ |x_n^{\prime}(x_n|_\omega^{-1} y)|} \frac{|x_n (\omega )|}{|\omega |}-1\bigg ) dy \bigg| \\ \nonumber &\quad\leq C \frac{|x_n (\omega)|^\alpha}{|x_n (\omega )|} \int_{x_n (\omega)} \prod_{\ell=1}^{\ell_0} |\xi_{n_\ell}(x_n|_\omega^{-1}( y)) |\, dy. \end{align} $$
$$ \begin{align} \nonumber &\bigg| \frac1{|\omega|} \int_{\omega } \prod_{\ell=1}^{\ell_0} \xi_{n_\ell}(a)\, da-\frac{1}{|x_n (\omega )|} \int_{x_n (\omega)} \prod_{\ell=1}^{\ell_0} \xi_{n_\ell}(x_n|_\omega^{-1} (y)) \, dy \bigg| \\ &\quad= \frac{1}{|x_n (\omega)|} \bigg| \int_{x_n (\omega)} \prod_{\ell=1}^{\ell_0} \xi_{n_\ell}(x_n|_\omega^{-1} (y)) \bigg( \frac 1{ |x_n^{\prime}(x_n|_\omega^{-1} y)|} \frac{|x_n (\omega )|}{|\omega |}-1\bigg ) dy \bigg| \\ \nonumber &\quad\leq C \frac{|x_n (\omega)|^\alpha}{|x_n (\omega )|} \int_{x_n (\omega)} \prod_{\ell=1}^{\ell_0} |\xi_{n_\ell}(x_n|_\omega^{-1}( y)) |\, dy. \end{align} $$
Since
![]() $\sup _k\|\xi _k\|_{L^\infty }<\infty $
, the claim then follows from Lemma 3.3.
$\sup _k\|\xi _k\|_{L^\infty }<\infty $
, the claim then follows from Lemma 3.3.
Proof of Lemma 3.3
For
![]() $a_0\in \omega $
as in the statement, the functions
$a_0\in \omega $
as in the statement, the functions
with
are bounded on
![]() $x_n(\omega \cap \Omega ^\varphi _{*})$
. Decomposing
$x_n(\omega \cap \Omega ^\varphi _{*})$
. Decomposing
 $$ \begin{align} \nonumber |\tilde \xi_1 \tilde \xi_2 \tilde \xi_3 \tilde \xi_4 - \tilde \varphi_1 \tilde \varphi_2 \tilde \varphi_3 \tilde \varphi_4| &\leq |(\tilde \xi_1 -\tilde \varphi_1) \tilde \xi_2 \tilde \xi_3 \tilde \xi_4| + |\tilde \varphi_1 (\tilde \xi_2 - \tilde \varphi_2) \tilde \xi_3 \tilde \xi_4| \\ &\quad+ |\tilde \varphi_1 \tilde \varphi_2 (\tilde \xi_3 - \tilde \varphi_3) \tilde \xi_4| + |\tilde \varphi_1 \tilde \varphi_2 \tilde \varphi_3 (\tilde \xi_4 - \tilde \varphi_4) | , \end{align} $$
$$ \begin{align} \nonumber |\tilde \xi_1 \tilde \xi_2 \tilde \xi_3 \tilde \xi_4 - \tilde \varphi_1 \tilde \varphi_2 \tilde \varphi_3 \tilde \varphi_4| &\leq |(\tilde \xi_1 -\tilde \varphi_1) \tilde \xi_2 \tilde \xi_3 \tilde \xi_4| + |\tilde \varphi_1 (\tilde \xi_2 - \tilde \varphi_2) \tilde \xi_3 \tilde \xi_4| \\ &\quad+ |\tilde \varphi_1 \tilde \varphi_2 (\tilde \xi_3 - \tilde \varphi_3) \tilde \xi_4| + |\tilde \varphi_1 \tilde \varphi_2 \tilde \varphi_3 (\tilde \xi_4 - \tilde \varphi_4) | , \end{align} $$
it is enough to find a uniform constant
![]() $\bar C>1$
such that
$\bar C>1$
such that

We will do so by showing the pointwise estimate
For
![]() $a= x_n|_\omega ^{-1} (y)$
, we decompose
$a= x_n|_\omega ^{-1} (y)$
, we decompose
 $$ \begin{align} \nonumber &\tilde \xi_{\ell,\omega} (y) - \tilde \varphi_{\ell,a_0} (y) = \xi_{n_\ell} (a) - \varphi_{a_0} (T_{a_0}^{n_\ell - n} (x_n (a))) \\ \nonumber &\quad= \varphi_a(x_{n_\ell} (a)) - \varphi_{a_0} (T_{a_0}^{n_\ell - n} (x_n (a)))\\ &\quad= \varphi_a(x_{n_\ell} (a)) - \varphi_{a_0} (x_{n_\ell} (a)) + \varphi_{a_0} (x_{n_\ell} (a)) - \varphi_{a_0} (T_{a_0}^{n_\ell - n} (x_n (a))). \end{align} $$
$$ \begin{align} \nonumber &\tilde \xi_{\ell,\omega} (y) - \tilde \varphi_{\ell,a_0} (y) = \xi_{n_\ell} (a) - \varphi_{a_0} (T_{a_0}^{n_\ell - n} (x_n (a))) \\ \nonumber &\quad= \varphi_a(x_{n_\ell} (a)) - \varphi_{a_0} (T_{a_0}^{n_\ell - n} (x_n (a)))\\ &\quad= \varphi_a(x_{n_\ell} (a)) - \varphi_{a_0} (x_{n_\ell} (a)) + \varphi_{a_0} (x_{n_\ell} (a)) - \varphi_{a_0} (T_{a_0}^{n_\ell - n} (x_n (a))). \end{align} $$
Using Remark 3.1, there exists C, independent of
![]() $n_\ell $
, such that
$n_\ell $
, such that
Hence, using our choice in equation (3.5) of
![]() $\unicode{x3bb} _0$
, and since
$\unicode{x3bb} _0$
, and since
![]() $|\omega | \leq C \unicode{x3bb} _\mathrm{CE}^{-n}$
by equation (2.7), we get
$|\omega | \leq C \unicode{x3bb} _\mathrm{CE}^{-n}$
by equation (2.7), we get
For the last two terms in the right-hand side of equation (3.15), note that since
![]() $a=x_n|_\omega ^{-1} (y)$
implies
$a=x_n|_\omega ^{-1} (y)$
implies
![]() $x_{n_\ell }(a) = T^{n_\ell + 1}_a(c) = T^{n_\ell -n}_a (T^{n+1}_a (c)) = T^{n_\ell -n}_a(y)$
, we have, using equation (2.2),
$x_{n_\ell }(a) = T^{n_\ell + 1}_a(c) = T^{n_\ell -n}_a (T^{n+1}_a (c)) = T^{n_\ell -n}_a(y)$
, we have, using equation (2.2),
 $$ \begin{align} &| x_{n_\ell} (x_n|_\omega^{-1} (y)) - T_{a_0}^{n_\ell - n}(y) | =|T_a^{n_\ell - n} (y) - T_{a_0}^{n_\ell - n} (y)| \nonumber\\ &\quad\leq C \Lambda^{n_\ell - n} |a - a_0| \leq C \Lambda^{n_\ell - n}|\omega| \quad \text{for all } y \in x_n(\omega). \end{align} $$
$$ \begin{align} &| x_{n_\ell} (x_n|_\omega^{-1} (y)) - T_{a_0}^{n_\ell - n}(y) | =|T_a^{n_\ell - n} (y) - T_{a_0}^{n_\ell - n} (y)| \nonumber\\ &\quad\leq C \Lambda^{n_\ell - n} |a - a_0| \leq C \Lambda^{n_\ell - n}|\omega| \quad \text{for all } y \in x_n(\omega). \end{align} $$
Then, since
![]() $n_\ell -n\le \eta n$
, our choice of
$n_\ell -n\le \eta n$
, our choice of
![]() $\unicode{x3bb} _0$
,
$\unicode{x3bb} _0$
,
![]() $\eta $
, with equation (3.2) at
$\eta $
, with equation (3.2) at
![]() $a=a_0$
give (we do not need the analogue of [Reference SchnellmannSch, Sublemma 5.4] here)
$a=a_0$
give (we do not need the analogue of [Reference SchnellmannSch, Sublemma 5.4] here)

using again in the last inequality that
![]() $|\omega | \leq C \unicode{x3bb} _\mathrm{CE}^{-n}$
from equation (2.7). We conclude by combining equations (3.17) and (3.19) into equation (3.15).
$|\omega | \leq C \unicode{x3bb} _\mathrm{CE}^{-n}$
from equation (2.7). We conclude by combining equations (3.17) and (3.19) into equation (3.15).
3.1 Proof of Proposition 3.2
We first show equation (3.9). Let
![]() $\omega \subset \tilde \omega \in \mathcal {P}_{[k-k^{1/4}]}$
, with
$\omega \subset \tilde \omega \in \mathcal {P}_{[k-k^{1/4}]}$
, with
![]() $k\ge 2n/\eta $
, be as in the assertion. Writing
$k\ge 2n/\eta $
, be as in the assertion. Writing
 $$ \begin{align*} \int_{\omega} \bigg( \sum_{j=k}^{k+n-1}\xi_j \bigg)^2 \, dm = \sum_{j=k}^{k+n-1} \bigg( \int_{\omega}\xi_j^2 \, dm + 2 \sum_{\ell=j+1}^{k+n-1} \int_{\omega }\xi_j \xi_\ell \, dm \bigg), \end{align*} $$
$$ \begin{align*} \int_{\omega} \bigg( \sum_{j=k}^{k+n-1}\xi_j \bigg)^2 \, dm = \sum_{j=k}^{k+n-1} \bigg( \int_{\omega}\xi_j^2 \, dm + 2 \sum_{\ell=j+1}^{k+n-1} \int_{\omega }\xi_j \xi_\ell \, dm \bigg), \end{align*} $$
it is sufficient to show that
 $$ \begin{align} \sum_{j=k}^{k+n-1} \bigg| 1 - \frac1{|\omega|} \int_{\omega} \bigg( \xi_j^2 + 2 \sum_{\ell=j+1}^{k+n-1} \xi_j \xi_\ell \bigg) \, dm \bigg| = O(1). \end{align} $$
$$ \begin{align} \sum_{j=k}^{k+n-1} \bigg| 1 - \frac1{|\omega|} \int_{\omega} \bigg( \xi_j^2 + 2 \sum_{\ell=j+1}^{k+n-1} \xi_j \xi_\ell \bigg) \, dm \bigg| = O(1). \end{align} $$
Fix
![]() $a_0 \in \omega \cap \Omega _{*}^\varphi $
. By Corollary 3.4 for
$a_0 \in \omega \cap \Omega _{*}^\varphi $
. By Corollary 3.4 for
![]() $\ell _0=2$
, we have, for
$\ell _0=2$
, we have, for
![]() $k\le j \le k+n-1$
,
$k\le j \le k+n-1$
,

(recall
![]() $v=[k-k^{1/4}]$
). Since
$v=[k-k^{1/4}]$
). Since
![]() $0<s<1/q<1$
, we have that
$0<s<1/q<1$
, we have that
![]() ${1}_{x_v(\omega )} \in H^s_q$
, uniformly in v and
${1}_{x_v(\omega )} \in H^s_q$
, uniformly in v and
![]() $\omega $
(see [Reference StrichartzSt]), so the first claim of Proposition 2.5 gives
$\omega $
(see [Reference StrichartzSt]), so the first claim of Proposition 2.5 gives
 $$ \begin{align*} &\int_{x_v(\omega)} (\varphi_{a_0} \circ T_{a_0}^{j-v} ) (\varphi_{a_0} \circ T_{a_0}^{\ell-v} )\, dm \\ &\quad = |x_v(\omega)| \int \varphi_{a_0} \cdot (\varphi_{a_0} \circ T_{a_0}^{\ell-j}) \, d\mu_{a_0} + O(\rho^{j-v}) \quad \text{for all } \ell \geq j. \end{align*} $$
$$ \begin{align*} &\int_{x_v(\omega)} (\varphi_{a_0} \circ T_{a_0}^{j-v} ) (\varphi_{a_0} \circ T_{a_0}^{\ell-v} )\, dm \\ &\quad = |x_v(\omega)| \int \varphi_{a_0} \cdot (\varphi_{a_0} \circ T_{a_0}^{\ell-j}) \, d\mu_{a_0} + O(\rho^{j-v}) \quad \text{for all } \ell \geq j. \end{align*} $$
Hence,

By equations (1.3) and (1.7), we have
 $$ \begin{align*} 1 = \int \varphi_{a_0}^2 \, d \mu_{a_0} + 2 \sum_{i=1}^\infty \int \varphi_{a_0} \cdot \varphi_{a_0} \circ T_{a_0}^i \, d \mu_{a_0}. \end{align*} $$
$$ \begin{align*} 1 = \int \varphi_{a_0}^2 \, d \mu_{a_0} + 2 \sum_{i=1}^\infty \int \varphi_{a_0} \cdot \varphi_{a_0} \circ T_{a_0}^i \, d \mu_{a_0}. \end{align*} $$
Therefore, the second claim of Proposition 2.5 gives
 $$ \begin{align*} \int \bigg( \varphi_{a_0}^2 + 2 \sum_{\ell=j+1}^{k+n-1} \varphi_{a_0} \cdot (\varphi_{a_0} \circ T_{a_0}^{\ell-j}) \bigg) \, d\mu_{a_0} = 1 + O (\rho^{k+n-j}).\end{align*} $$
$$ \begin{align*} \int \bigg( \varphi_{a_0}^2 + 2 \sum_{\ell=j+1}^{k+n-1} \varphi_{a_0} \cdot (\varphi_{a_0} \circ T_{a_0}^{\ell-j}) \bigg) \, d\mu_{a_0} = 1 + O (\rho^{k+n-j}).\end{align*} $$
Hence, we find, for
![]() $k \leq j \leq k + n - 1$
and
$k \leq j \leq k + n - 1$
and
![]() $v = [k - k^{1/4}]$
,
$v = [k - k^{1/4}]$
,

To proceed, we shall use several times that
 $$ \begin{align*} \sup_{n}\sup_k \sum_{j=k}^{k+n-1} \frac{1}{(k+n-j)^2} \le \sup_n \sum_{\ell=1}^n \frac 1 {\ell^2} <\infty. \end{align*} $$
$$ \begin{align*} \sup_{n}\sup_k \sum_{j=k}^{k+n-1} \frac{1}{(k+n-j)^2} \le \sup_n \sum_{\ell=1}^n \frac 1 {\ell^2} <\infty. \end{align*} $$
Clearly,
![]() $\rho ^{k+n-j} \leq {C}/{(k+n-j)^2}$
. For the term
$\rho ^{k+n-j} \leq {C}/{(k+n-j)^2}$
. For the term
![]() $(k+n-j) \rho ^{j-v} |x_v (\omega )|^{-1}$
, we use
$(k+n-j) \rho ^{j-v} |x_v (\omega )|^{-1}$
, we use
![]() $|x_v (\omega )| \geq \unicode{x3bb} _0^{-k^{1/4}}$
and the definition in equation (3.5) of
$|x_v (\omega )| \geq \unicode{x3bb} _0^{-k^{1/4}}$
and the definition in equation (3.5) of
![]() $\unicode{x3bb} _0$
to get, since
$\unicode{x3bb} _0$
to get, since
![]() $k\ge 2n/\eta $
,
$k\ge 2n/\eta $
,

The term
![]() $(k+n-j) \unicode{x3bb} _0^{-(k-k^{1/4})}$
is similar. Finally,
$(k+n-j) \unicode{x3bb} _0^{-(k-k^{1/4})}$
is similar. Finally,
![]() $|x_v (\omega )| \leq v^{-3/\alpha }$
gives
$|x_v (\omega )| \leq v^{-3/\alpha }$
gives
 $$ \begin{align*} \sum_{j=k}^{k+n-1} (k + n - j) |x_v (\omega)|^\alpha \leq \sum_{j=k}^{k+n-1} \frac{k+n-j}{n^3}\leq n \frac{k+n-k}{n^3} = \frac{1}{n}. \end{align*} $$
$$ \begin{align*} \sum_{j=k}^{k+n-1} (k + n - j) |x_v (\omega)|^\alpha \leq \sum_{j=k}^{k+n-1} \frac{k+n-j}{n^3}\leq n \frac{k+n-k}{n^3} = \frac{1}{n}. \end{align*} $$
This proves equation (3.20), and hence equation (3.9).
We will next deduce equations (3.8) and (3.11) from equation (3.9). Fix
![]() $\kappa _1>\kappa _0$
, let
$\kappa _1>\kappa _0$
, let
![]() $N_1(\kappa _1)\ge N_0$
be given by Lemma 2.3 and let
$N_1(\kappa _1)\ge N_0$
be given by Lemma 2.3 and let
![]() $K\ge N_1$
be such that
$K\ge N_1$
be such that
![]() $ k^{\kappa _1}\le \unicode{x3bb} _0^{k^{1/4}}$
for all
$ k^{\kappa _1}\le \unicode{x3bb} _0^{k^{1/4}}$
for all
![]() $k \ge K$
. Then, if
$k \ge K$
. Then, if
![]() $v = v(k) \ge K$
(so that
$v = v(k) \ge K$
(so that
![]() $k\ge K$
), we have
$k\ge K$
), we have
Refining
![]() $\mathcal {P}_v$
to a partition
$\mathcal {P}_v$
to a partition
![]() $\mathcal {Q}_v$
such that
$\mathcal {Q}_v$
such that
we set
![]() $\Omega _{*,v}^{\mathcal {Q}}$
as in equation (3.10) and we decompose
$\Omega _{*,v}^{\mathcal {Q}}$
as in equation (3.10) and we decompose
 $$ \begin{align*} |\Omega^\varphi_{*}| \cdot E \bigg(\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 &= \int_{\Omega^{\mathcal {Q}}_{*,v}} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m - \int_{\Omega^{\mathcal {Q}}_{*,v}\setminus \Omega^\varphi_{*}} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m \\ &= \int_{\Omega_v} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m - \int_{\Omega_v\setminus \Omega^\varphi_{*}} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m. \end{align*} $$
$$ \begin{align*} |\Omega^\varphi_{*}| \cdot E \bigg(\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 &= \int_{\Omega^{\mathcal {Q}}_{*,v}} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m - \int_{\Omega^{\mathcal {Q}}_{*,v}\setminus \Omega^\varphi_{*}} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m \\ &= \int_{\Omega_v} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m - \int_{\Omega_v\setminus \Omega^\varphi_{*}} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m. \end{align*} $$
Then, using equation (2.9),
![]() $\sup _k \sup |\xi _k|<\infty $
,
$\sup _k \sup |\xi _k|<\infty $
,
![]() $\kappa _0 \geq 3 / d_1$
and
$\kappa _0 \geq 3 / d_1$
and
![]() $v(k)\ge k/2\ge n/\eta $
,
$v(k)\ge k/2\ge n/\eta $
,
 $$ \begin{align} \nonumber 0 \leq \int_{\Omega^{\mathcal {Q}}_{*,v}\setminus \Omega^\varphi_{*}} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2\, d m & \leq \int_{\Omega_v\setminus \Omega^\varphi_{*}} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m \\ & \leq C n^2 e_v \leq C n^2 n^{1 - d_1 \kappa_0} \leq C , \end{align} $$
$$ \begin{align} \nonumber 0 \leq \int_{\Omega^{\mathcal {Q}}_{*,v}\setminus \Omega^\varphi_{*}} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2\, d m & \leq \int_{\Omega_v\setminus \Omega^\varphi_{*}} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m \\ & \leq C n^2 e_v \leq C n^2 n^{1 - d_1 \kappa_0} \leq C , \end{align} $$
which shows that
 $$ \begin{align*} \int_{\Omega_{*}^\varphi} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m & = \int_{\Omega^{\mathcal {Q}}_{*,v}} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m + O(1) \\ &= \int_{\Omega_v} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m + O(1). \end{align*} $$
$$ \begin{align*} \int_{\Omega_{*}^\varphi} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m & = \int_{\Omega^{\mathcal {Q}}_{*,v}} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m + O(1) \\ &= \int_{\Omega_v} \bigg( \sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m + O(1). \end{align*} $$
By equation (3.9),
 $$ \begin{align} \int_{\omega} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m = |\omega|(n + O(1)) \quad \text{for all } \omega \in \mathcal {Q}_v^*. \end{align} $$
$$ \begin{align} \int_{\omega} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m = |\omega|(n + O(1)) \quad \text{for all } \omega \in \mathcal {Q}_v^*. \end{align} $$
Summing equation (3.23) over
![]() $\omega \in \mathcal {Q}^*_v$
, we get that
$\omega \in \mathcal {Q}^*_v$
, we get that
 $$ \begin{align*} \int_{\Omega^{\mathcal {Q}}_{*,v}} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m = n + O(1). \end{align*} $$
$$ \begin{align*} \int_{\Omega^{\mathcal {Q}}_{*,v}} \bigg (\sum_{j=k}^{k+n-1} \xi_j \bigg)^2 \, d m = n + O(1). \end{align*} $$
Finally, using again equation (2.9) to see
 $$ \begin{align*} 0 \leq \frac{|\Omega^{\mathcal {Q}}_{*,v}|}{|\Omega^\varphi_{*}|} - 1 \leq \frac{|\Omega_v|}{|\Omega^\varphi_{*}|}-1 = O(e_v) = O(e_n), \end{align*} $$
$$ \begin{align*} 0 \leq \frac{|\Omega^{\mathcal {Q}}_{*,v}|}{|\Omega^\varphi_{*}|} - 1 \leq \frac{|\Omega_v|}{|\Omega^\varphi_{*}|}-1 = O(e_v) = O(e_n), \end{align*} $$
we have established equation (3.8), and also equation (3.11) in the case
![]() ${\Psi _k\kern1.5pt{=}\kern1.5pt(\sum _{j=k}^{k+n-1} \xi _j )^2}$
(note that
${\Psi _k\kern1.5pt{=}\kern1.5pt(\sum _{j=k}^{k+n-1} \xi _j )^2}$
(note that
![]() $|\Psi _k|\le C n^2 \le C k^2$
). For more general
$|\Psi _k|\le C n^2 \le C k^2$
). For more general
![]() $\Psi _k$
, the same argument, using
$\Psi _k$
, the same argument, using
![]() ${d_1 \kappa _0 \ge 11/3}$
in equation (3.22), gives equation (3.11). This ends the proof of Proposition 3.2.
${d_1 \kappa _0 \ge 11/3}$
in equation (3.22), gives equation (3.11). This ends the proof of Proposition 3.2.
4 Proof of Theorem 1.1 via Skorokhod’s representation theorem
We will rearrange the Birkhoff sum as a sum of blocks of polynomial size, approximate the blocks by a martingale and finally apply Skorokhod’s representation theorem to this martingale. The size for the jth block
![]() $\mathbb {I}_j$
is
$\mathbb {I}_j$
is
![]() $j^{2/3}$
, which will give the error exponent
$j^{2/3}$
, which will give the error exponent
![]() $\gamma>2/5$
in our ASIP. (A block size
$\gamma>2/5$
in our ASIP. (A block size
![]() $\#\mathbb {I}_j=j^b$
replaces
$\#\mathbb {I}_j=j^b$
replaces
![]() $3/5$
in equation (4.4) by
$3/5$
in equation (4.4) by
![]() $1/(1+b)$
, so that the first constraint becomes
$1/(1+b)$
, so that the first constraint becomes
![]() $N^\gamma> N^{b/(1+b)}$
, see equation (4.5). Our bounds in equations (4.25) and (4.26) (with Gál–Koksma’s strong law of large numbers, Theorem 4.3, and
$N^\gamma> N^{b/(1+b)}$
, see equation (4.5). Our bounds in equations (4.25) and (4.26) (with Gál–Koksma’s strong law of large numbers, Theorem 4.3, and
![]() $M(N)\sim N^{1/(1+b)}$
) give
$M(N)\sim N^{1/(1+b)}$
) give
![]() $N^{\gamma }> N^{(b+2)/(4(b+1))}$
. Hence,
$N^{\gamma }> N^{(b+2)/(4(b+1))}$
. Hence,
![]() $b=2/3$
is the optimum. In the independent and identically distributed case, a block size
$b=2/3$
is the optimum. In the independent and identically distributed case, a block size
![]() $j^{1/2}$
gives
$j^{1/2}$
gives
![]() $\gamma>1/3$
[Reference Philipp and StoutPS, p. 25], see also the beginning of [Reference SchnellmannSch, §6].)
$\gamma>1/3$
[Reference Philipp and StoutPS, p. 25], see also the beginning of [Reference SchnellmannSch, §6].)
4.1 Blocks
 $\mathbb {I}_M$
. Approximations
$\mathbb {I}_M$
. Approximations
 $\chi _i$
and
$\chi _i$
and
 $y_j$
$y_j$
Fix
![]() $a_{*}$
,
$a_{*}$
,
![]() $\varpi \in (0,1)$
, q,
$\varpi \in (0,1)$
, q,
![]() $s\in (0, \operatorname \min \{\varpi , 1/q\})$
,
$s\in (0, \operatorname \min \{\varpi , 1/q\})$
,
![]() $\rho $
,
$\rho $
,
![]() $\theta $
,
$\theta $
,
![]() $\unicode{x3bb} _0$
,
$\unicode{x3bb} _0$
,
![]() $\eta $
,
$\eta $
,
![]() $\alpha $
,
$\alpha $
,
![]() $\varphi \in C^\varpi $
,
$\varphi \in C^\varpi $
,
![]() $\Omega _{*}^\varphi =\Omega _{*}\cap [a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
as in the beginning of §3. Set
$\Omega _{*}^\varphi =\Omega _{*}\cap [a_{*}-\epsilon _\varphi , a_{*}+\epsilon _\varphi ]$
as in the beginning of §3. Set
 $$ \begin{align*} \mathcal{P}_{*,k}:=\{\omega \in \mathcal{P}_{k} \mid |\omega\cap \Omega_{*}^\varphi|>0\} , \quad \Omega_{*, k}:= \bigcup_{\omega \in \mathcal{P}_{*, k}} \omega, \quad k \ge 1. \end{align*} $$
$$ \begin{align*} \mathcal{P}_{*,k}:=\{\omega \in \mathcal{P}_{k} \mid |\omega\cap \Omega_{*}^\varphi|>0\} , \quad \Omega_{*, k}:= \bigcup_{\omega \in \mathcal{P}_{*, k}} \omega, \quad k \ge 1. \end{align*} $$
Fix
![]() $\gamma \in (2/5, 1/2)$
and
$\gamma \in (2/5, 1/2)$
and
![]() $\delta \in (0,\operatorname \min \{1/5, 2(\gamma -2/5)\})$
. (See Lemma 4.4 for the condition
$\delta \in (0,\operatorname \min \{1/5, 2(\gamma -2/5)\})$
. (See Lemma 4.4 for the condition
![]() $\delta <2(\gamma -2/5)$
.) For
$\delta <2(\gamma -2/5)$
.) For
![]() $i\ge 1$
, we shall approach
$i\ge 1$
, we shall approach
![]() $\xi _i \colon [a_{*}-\epsilon _\varphi ,a_{*}+\epsilon _\varphi ] \to \mathbb {C}$
(see Remark 3.1) by the stepfunction
$\xi _i \colon [a_{*}-\epsilon _\varphi ,a_{*}+\epsilon _\varphi ] \to \mathbb {C}$
(see Remark 3.1) by the stepfunction
with
![]() $\mathcal {F}_k$
the
$\mathcal {F}_k$
the
![]() $\sigma $
-algebra generated by the intervals in
$\sigma $
-algebra generated by the intervals in
![]() $\mathcal {P}_{*,k}$
. Conditional expectations are only defined almost everywhere, but we may set (see equation (3.7))
$\mathcal {P}_{*,k}$
. Conditional expectations are only defined almost everywhere, but we may set (see equation (3.7))
 $$ \begin{align} \chi_i|_{\omega} \equiv \frac{ \int_{\omega \cap \Omega_{*}^\varphi} \xi_i \, dm}{|\omega \cap \Omega_{*}^\varphi|} \quad \text{for all } \omega \in \mathcal{P}_{*,r_i} , \text{ for all } i \ge 1. \end{align} $$
$$ \begin{align} \chi_i|_{\omega} \equiv \frac{ \int_{\omega \cap \Omega_{*}^\varphi} \xi_i \, dm}{|\omega \cap \Omega_{*}^\varphi|} \quad \text{for all } \omega \in \mathcal{P}_{*,r_i} , \text{ for all } i \ge 1. \end{align} $$
Thus,
![]() $\chi _i$
is defined everywhere on
$\chi _i$
is defined everywhere on
![]() $\Omega _{*,r_i}$
, allowing pointwise claims about it.
$\Omega _{*,r_i}$
, allowing pointwise claims about it.
Recalling
![]() $e_\ell $
from equation (2.9) and our assumption
$e_\ell $
from equation (2.9) and our assumption
![]() $\unicode{x3bb} _\mathrm{CE}>e^{ 14 \alpha _\mathrm{BC}}$
in the proof of Proposition 2.2, we have the following basic lemma.
$\unicode{x3bb} _\mathrm{CE}>e^{ 14 \alpha _\mathrm{BC}}$
in the proof of Proposition 2.2, we have the following basic lemma.
Lemma 4.1. For any
![]() $\tilde{\unicode{x3bb}} _\mathrm{CE}\in (e^{\alpha _\mathrm{BC}}, \sqrt {\unicode{x3bb} _\mathrm{CE} } \cdot e^{-\alpha _\mathrm{BC}})$
, there exists C such that
$\tilde{\unicode{x3bb}} _\mathrm{CE}\in (e^{\alpha _\mathrm{BC}}, \sqrt {\unicode{x3bb} _\mathrm{CE} } \cdot e^{-\alpha _\mathrm{BC}})$
, there exists C such that
and for all
![]() $i\ge 1$
,
$i\ge 1$
,
![]() $j\ge 0$
and all
$j\ge 0$
and all
![]() $a\in \Omega _{*,r_i}$
,
$a\in \Omega _{*,r_i}$
,
(The constant C in equation (4.3) goes to infinity as
![]() $\delta \to 0$
, that is, if
$\delta \to 0$
, that is, if
![]() $\gamma \to 2/5$
.)
$\gamma \to 2/5$
.)
Following [Reference Philipp and StoutPS, §3.3], [Reference SchnellmannSch, §6.1], we define inductively consecutive blocks
![]() $\mathbb {I}_j$
of integers and associated functions
$\mathbb {I}_j$
of integers and associated functions
![]() $y_j$
as follows. Let
$y_j$
as follows. Let
![]() $\mathbb {I}_1 = \{1\}$
and let
$\mathbb {I}_1 = \{1\}$
and let
![]() $\mathbb {I}_j$
for
$\mathbb {I}_j$
for
![]() $j\ge 2$
contain
$j\ge 2$
contain
![]() $[j^{2/3}]$
consecutive integers. The first blocks are
$[j^{2/3}]$
consecutive integers. The first blocks are
 $$ \begin{align*} \underbrace{1,}_{\mathbb{I}_1} \underbrace{2,}_{\mathbb{I}_2} \underbrace{3, 4,}_{\mathbb{I}_3} \underbrace{5, 6,}_{\mathbb{I}_4} \underbrace{7, 8,}_{\mathbb{I}_5} \underbrace{9, 10, 11,}_{\mathbb{I}_6} \underbrace{12, 13, 14,}_{\mathbb{I}_7} \underbrace{15, 16, 17, 18,}_{\mathbb{I}_8} \underbrace{19, 20, 21, 22,}_{\mathbb{I}_9} \ldots \end{align*} $$
$$ \begin{align*} \underbrace{1,}_{\mathbb{I}_1} \underbrace{2,}_{\mathbb{I}_2} \underbrace{3, 4,}_{\mathbb{I}_3} \underbrace{5, 6,}_{\mathbb{I}_4} \underbrace{7, 8,}_{\mathbb{I}_5} \underbrace{9, 10, 11,}_{\mathbb{I}_6} \underbrace{12, 13, 14,}_{\mathbb{I}_7} \underbrace{15, 16, 17, 18,}_{\mathbb{I}_8} \underbrace{19, 20, 21, 22,}_{\mathbb{I}_9} \ldots \end{align*} $$
Let
![]() $M = M(N)$
be uniquely defined by
$M = M(N)$
be uniquely defined by
![]() $N \in \mathbb {I}_M$
. There exists C such that
$N \in \mathbb {I}_M$
. There exists C such that
By equation (4.2) in Lemma 4.1, there is C such that, for all
![]() $i\ge 1$
and all
$i\ge 1$
and all
![]() $a \in \Omega _{*, r_i}$
,
$a \in \Omega _{*, r_i}$
,
 $$ \begin{align} \bigg| \sum_{i=1}^N\xi_i(a) - \sum_{j=1}^{M(N)} \sum_{i \in \mathbb{I}_j} \chi_i (a) \bigg| & \le\sum_{i=1}^N|\xi_i(a)-\chi_i(a)|+C\# \mathbb{I}_M \le C N^{2/5} \end{align} $$
$$ \begin{align} \bigg| \sum_{i=1}^N\xi_i(a) - \sum_{j=1}^{M(N)} \sum_{i \in \mathbb{I}_j} \chi_i (a) \bigg| & \le\sum_{i=1}^N|\xi_i(a)-\chi_i(a)|+C\# \mathbb{I}_M \le C N^{2/5} \end{align} $$
for all
![]() $N\ge 1$
. Hence, to prove Theorem 1.1, it is sufficient to consider
$N\ge 1$
. Hence, to prove Theorem 1.1, it is sufficient to consider
 $$ \begin{align*} y_j \colon \Omega_{*, [Cr_j^{5/3}]} \to \mathbb{C}, \quad y_j := \sum_{i \in \mathbb{I}_j} \chi_i , \quad j \ge 1. \end{align*} $$
$$ \begin{align*} y_j \colon \Omega_{*, [Cr_j^{5/3}]} \to \mathbb{C}, \quad y_j := \sum_{i \in \mathbb{I}_j} \chi_i , \quad j \ge 1. \end{align*} $$
Proof of Lemma 4.1
By equation (4.1), since
![]() $\xi _i$
is continuous (see Remark 3.1), for any
$\xi _i$
is continuous (see Remark 3.1), for any
![]() $\omega \in \mathcal {P}_{*,r_i}$
, there exists
$\omega \in \mathcal {P}_{*,r_i}$
, there exists
![]() $a^{\prime }\in \omega $
such that
$a^{\prime }\in \omega $
such that
![]() $\chi _i|_{\omega }=\xi _i(a^{\prime })$
. Revisiting the decomposition in equation (3.3), and using equation (3.2) and the
$\chi _i|_{\omega }=\xi _i(a^{\prime })$
. Revisiting the decomposition in equation (3.3), and using equation (3.2) and the
![]() $\theta $
-Hölder continuity of
$\theta $
-Hölder continuity of
![]() $a\mapsto \varphi _a(u)$
(as for equation (3.16)), we find C such that for all
$a\mapsto \varphi _a(u)$
(as for equation (3.16)), we find C such that for all
![]() $i\ge 1$
and
$i\ge 1$
and
![]() $\omega \in \mathcal {P}_{*,r_i}$
,
$\omega \in \mathcal {P}_{*,r_i}$
,
where we used
![]() $\theta \le \varpi $
and equation (2.7) in the second inequality. This establishes equation (4.2), since for any
$\theta \le \varpi $
and equation (2.7) in the second inequality. This establishes equation (4.2), since for any
![]() $\bar{\unicode{x3bb}} _\mathrm{CE}\in (e^{\alpha _\mathrm{BC}}, \sqrt {\unicode{x3bb} _\mathrm{CE} } \cdot e^{-\alpha _\mathrm{BC}})$
, there exists
$\bar{\unicode{x3bb}} _\mathrm{CE}\in (e^{\alpha _\mathrm{BC}}, \sqrt {\unicode{x3bb} _\mathrm{CE} } \cdot e^{-\alpha _\mathrm{BC}})$
, there exists
![]() $\bar C$
such that
$\bar C$
such that
To show equation (4.6), first note, using equation (2.29), that there exists
![]() $a\in \omega $
such that
$a\in \omega $
such that
 $$ \begin{align*} |x_i(\omega)| \leq C \frac{|x_{r_i}(\omega)|}{|(T_a^{i^\delta})^{\prime}(x_i(a))|}. \end{align*} $$
$$ \begin{align*} |x_i(\omega)| \leq C \frac{|x_{r_i}(\omega)|}{|(T_a^{i^\delta})^{\prime}(x_i(a))|}. \end{align*} $$
Then, if
![]() $a\in \Omega _{*}$
, the polynomial recurrence in equation (2.8) and standard arguments give
$a\in \Omega _{*}$
, the polynomial recurrence in equation (2.8) and standard arguments give
(see e.g. [Reference Baladi and SmaniaBS1, Proposition 3.7] in the exponentially recurrent case). If
![]() $a\notin \Omega _{*}$
, we may use bounded distortion in equation (2.31) (
$a\notin \Omega _{*}$
, we may use bounded distortion in equation (2.31) (
![]() $\alpha =0$
suffices here) since
$\alpha =0$
suffices here) since
![]() $|\omega \cap \Omega _{*}|>0$
.
$|\omega \cap \Omega _{*}|>0$
.
The equality in equation (4.3) follows from the definition since
![]() $\mathcal {F}_{r_i} \subset \mathcal {F}_{r_{i+j}}$
. Indeed, for
$\mathcal {F}_{r_i} \subset \mathcal {F}_{r_{i+j}}$
. Indeed, for
![]() $a \in \omega \in \mathcal {P}_{*,r_i}$
,
$a \in \omega \in \mathcal {P}_{*,r_i}$
,
 $$ \begin{align} |\omega \cap \Omega_{*}^\varphi|\cdot |E(\xi_{i+j} | \mathcal{F}_{r_i} )(a)|&= \int_{\omega \cap \Omega_{*}^\varphi} \xi_{i+j} \, dm\nonumber\\ & =\sum_{\substack{\omega^{\prime} \in \mathcal{P}_{*,r_{i+j}}\\ \omega^{\prime}\subset \omega}} |\omega^{\prime} \cap \Omega_{*}^{\varphi}| \cdot \frac{ \int_{\omega^{\prime} \cap \Omega_{*}^{\varphi}} \xi_{i+j} \, dm}{|\omega^{\prime} \cap \Omega_{*}^{\varphi}|}\nonumber\\ &=\sum_{\substack{\omega^{\prime} \in \mathcal{P}_{*,r_{i+j}}\\ \omega^{\prime}\subset \omega}}|\omega^{\prime} \cap \Omega_{*}^{\varphi}| \cdot \chi_{i+j}|_{\omega^{\prime}} =\sum_{\substack{\omega^{\prime} \in \mathcal{P}_{*,r_{i+j}}\\ \omega^{\prime}\subset \omega}} \int_{\omega^{\prime}\cap \Omega_{*}^{\varphi}} \chi_{i+j}\, dm. \end{align} $$
$$ \begin{align} |\omega \cap \Omega_{*}^\varphi|\cdot |E(\xi_{i+j} | \mathcal{F}_{r_i} )(a)|&= \int_{\omega \cap \Omega_{*}^\varphi} \xi_{i+j} \, dm\nonumber\\ & =\sum_{\substack{\omega^{\prime} \in \mathcal{P}_{*,r_{i+j}}\\ \omega^{\prime}\subset \omega}} |\omega^{\prime} \cap \Omega_{*}^{\varphi}| \cdot \frac{ \int_{\omega^{\prime} \cap \Omega_{*}^{\varphi}} \xi_{i+j} \, dm}{|\omega^{\prime} \cap \Omega_{*}^{\varphi}|}\nonumber\\ &=\sum_{\substack{\omega^{\prime} \in \mathcal{P}_{*,r_{i+j}}\\ \omega^{\prime}\subset \omega}}|\omega^{\prime} \cap \Omega_{*}^{\varphi}| \cdot \chi_{i+j}|_{\omega^{\prime}} =\sum_{\substack{\omega^{\prime} \in \mathcal{P}_{*,r_{i+j}}\\ \omega^{\prime}\subset \omega}} \int_{\omega^{\prime}\cap \Omega_{*}^{\varphi}} \chi_{i+j}\, dm. \end{align} $$
Since
![]() $\sup _k \|\xi _k\|_{L^\infty }<\infty $
, we may and shall assume that
$\sup _k \|\xi _k\|_{L^\infty }<\infty $
, we may and shall assume that
![]() $j \geq 2 i^{\delta }$
to prove the upper bound in equation (4.3). For such j, recalling
$j \geq 2 i^{\delta }$
to prove the upper bound in equation (4.3). For such j, recalling
![]() $\eta \in (0,1/2)$
from equation (3.6), define
$\eta \in (0,1/2)$
from equation (3.6), define
 $$ \begin{align} k=k(i,j)= \operatorname\max \bigg\{ i + [i^\delta] + \eta (j - i^\delta), \bigg \lceil \frac{i+j}{1 + \eta}\bigg \rceil \bigg\} \end{align} $$
$$ \begin{align} k=k(i,j)= \operatorname\max \bigg\{ i + [i^\delta] + \eta (j - i^\delta), \bigg \lceil \frac{i+j}{1 + \eta}\bigg \rceil \bigg\} \end{align} $$
so that
![]() $k\le i+j- \eta /({1+\eta })(j-i^\delta ) \le i+j$
and
$k\le i+j- \eta /({1+\eta })(j-i^\delta ) \le i+j$
and
![]() $i+j \le k(1+\eta )$
.
$i+j \le k(1+\eta )$
.
Since
![]() $\delta $
is fixed, we may and shall assume that i is large enough such that
$\delta $
is fixed, we may and shall assume that i is large enough such that
![]() $k(i,j)\ge N_1$
(with
$k(i,j)\ge N_1$
(with
![]() $N_1$
from Lemma 2.3) and
$N_1$
from Lemma 2.3) and
Since
![]() $k(i,j) \geq r_i$
, we have, similarly as for equation (4.8),
$k(i,j) \geq r_i$
, we have, similarly as for equation (4.8),
We must analyse the above decomposition more closely than in the proof of [Reference SchnellmannSch, Lemma 6.1] as follows. Let
![]() $a \in \tilde \omega \in \mathcal {P}_{*, r_i}$
, then,
$a \in \tilde \omega \in \mathcal {P}_{*, r_i}$
, then,
 $$ \begin{align} &|\tilde \omega\cap \Omega_{*}^\varphi| \cdot |E(E(\xi_{i+j} | \mathcal{F}_{k(i,j)}) | \mathcal{F}_{r_i})(a)| = \bigg | \sum_{\substack{\omega \in \mathcal{P}_{*,k(i,j)}\nonumber\\ \omega \subset \tilde \omega}} \frac {|\omega\cap \Omega^\varphi_{*}|} {|\omega\cap \Omega^\varphi_{*}|} \int_{\omega\cap \Omega_{*}^\varphi} \xi_{i+j} \, d m\bigg | \\ &\quad\le \bigg |\sum_{\substack{\omega \in \mathcal{P}_{*,k(i,j)}\\\omega \subset \tilde \omega}} \frac {|\omega|} {|\omega|} \int_{\omega} \xi_{i+j} \, d m\bigg | + \sup_{\tilde a} \|\varphi_{\tilde a}\|_{L^\infty}\cdot \sum_{\substack{\omega\in \mathcal{P}_{*,k(i,j)}\\ \omega \subset \tilde \omega}} |\omega \setminus (\omega\cap \Omega^\varphi_{*})|. \end{align} $$
$$ \begin{align} &|\tilde \omega\cap \Omega_{*}^\varphi| \cdot |E(E(\xi_{i+j} | \mathcal{F}_{k(i,j)}) | \mathcal{F}_{r_i})(a)| = \bigg | \sum_{\substack{\omega \in \mathcal{P}_{*,k(i,j)}\nonumber\\ \omega \subset \tilde \omega}} \frac {|\omega\cap \Omega^\varphi_{*}|} {|\omega\cap \Omega^\varphi_{*}|} \int_{\omega\cap \Omega_{*}^\varphi} \xi_{i+j} \, d m\bigg | \\ &\quad\le \bigg |\sum_{\substack{\omega \in \mathcal{P}_{*,k(i,j)}\\\omega \subset \tilde \omega}} \frac {|\omega|} {|\omega|} \int_{\omega} \xi_{i+j} \, d m\bigg | + \sup_{\tilde a} \|\varphi_{\tilde a}\|_{L^\infty}\cdot \sum_{\substack{\omega\in \mathcal{P}_{*,k(i,j)}\\ \omega \subset \tilde \omega}} |\omega \setminus (\omega\cap \Omega^\varphi_{*})|. \end{align} $$
Since
![]() $\tilde \omega \in \mathcal {P}_{*, r_i}$
, the bound in equation (2.10) implies
$\tilde \omega \in \mathcal {P}_{*, r_i}$
, the bound in equation (2.10) implies
 $$ \begin{align} \begin{cases} \dfrac{\sum_{\substack{\omega\in \mathcal{P}_{*,k(i,j)}\\ \omega\subset \tilde \omega}} |\omega \setminus (\omega\cap \Omega_{*})| }{|\tilde \omega\cap \Omega_{*}^\varphi|} \le \dfrac{d_0 e_{k(i,j)-r_i} | \tilde \omega|} {(1-d_0 e_{r_i}) | \tilde\omega|} \le C d_0 e_{[\eta(j-i^\delta)]}, \\[6pt] |\tilde \omega | /{|\tilde \omega\cap \Omega_{*}^\varphi|} \le \dfrac{ | \tilde \omega|} {(1-d_0 e_{r_i})| \tilde \omega|}\le C. \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} \dfrac{\sum_{\substack{\omega\in \mathcal{P}_{*,k(i,j)}\\ \omega\subset \tilde \omega}} |\omega \setminus (\omega\cap \Omega_{*})| }{|\tilde \omega\cap \Omega_{*}^\varphi|} \le \dfrac{d_0 e_{k(i,j)-r_i} | \tilde \omega|} {(1-d_0 e_{r_i}) | \tilde\omega|} \le C d_0 e_{[\eta(j-i^\delta)]}, \\[6pt] |\tilde \omega | /{|\tilde \omega\cap \Omega_{*}^\varphi|} \le \dfrac{ | \tilde \omega|} {(1-d_0 e_{r_i})| \tilde \omega|}\le C. \end{cases} \end{align} $$
In view of equations (2.10), (4.12) and (4.11), it suffices to show
 $$ \begin{align*} \frac1{|\omega|} \bigg| \int_{\omega} \xi_{i+j} \, dm \bigg| \le C \operatorname\min (1, e_{[\eta (j- 2 i^\delta)]}) \quad \text{for all } \omega \in \mathcal{P}_{*,k(i,j)}. \end{align*} $$
$$ \begin{align*} \frac1{|\omega|} \bigg| \int_{\omega} \xi_{i+j} \, dm \bigg| \le C \operatorname\min (1, e_{[\eta (j- 2 i^\delta)]}) \quad \text{for all } \omega \in \mathcal{P}_{*,k(i,j)}. \end{align*} $$
Fix
![]() $\omega \in \mathcal {P}_{*,k(i,j)}$
. First note that, by equation (2.31) for
$\omega \in \mathcal {P}_{*,k(i,j)}$
. First note that, by equation (2.31) for
![]() $\alpha =0$
,
$\alpha =0$
,
 $$ \begin{align} \frac1{|\omega|} \bigg| \int_\omega \xi_{i+j}(a) \, da \bigg| \leq \frac{C}{|x_k(\omega)|} \bigg| \int_{x_k(\omega)} \xi_{i+j} (x_k|_\omega^{-1}(y)) \, dy \bigg|. \end{align} $$
$$ \begin{align} \frac1{|\omega|} \bigg| \int_\omega \xi_{i+j}(a) \, da \bigg| \leq \frac{C}{|x_k(\omega)|} \bigg| \int_{x_k(\omega)} \xi_{i+j} (x_k|_\omega^{-1}(y)) \, dy \bigg|. \end{align} $$
Then, on the one hand, Lemma 3.3 for
![]() $\ell _0=1$
gives
$\ell _0=1$
gives
![]() $a_0\in \omega \cap \Omega ^\varphi _{*}$
such that
$a_0\in \omega \cap \Omega ^\varphi _{*}$
such that

On the other hand, recalling
![]() $0<s<1/q$
, since
$0<s<1/q$
, since
![]() $1_{x_k (\omega )}\in H^s_q$
(uniformly in k and
$1_{x_k (\omega )}\in H^s_q$
(uniformly in k and
![]() $\omega $
), the first claim of Proposition 2.5, with
$\omega $
), the first claim of Proposition 2.5, with
![]() $\int \varphi _{a_0} d\mu _{a_0}=0$
, gives (a factor
$\int \varphi _{a_0} d\mu _{a_0}=0$
, gives (a factor
![]() $|x_k (\omega )|^{-1}$
was omitted when applying [Reference SchnellmannSch], Proposition 4.3] on [Reference SchnellmannSch, p. 400]. We fix this by using our polynomial lower bound on
$|x_k (\omega )|^{-1}$
was omitted when applying [Reference SchnellmannSch], Proposition 4.3] on [Reference SchnellmannSch, p. 400]. We fix this by using our polynomial lower bound on
![]() $|x_k(\omega )|$
(considering two different values of
$|x_k(\omega )|$
(considering two different values of
![]() $\delta $
should work for [Reference SchnellmannSch]))
$\delta $
should work for [Reference SchnellmannSch]))
 $$ \begin{align} &\frac1{|x_{k}(\omega)|} \bigg| \int_{x_{k}(\omega)} \varphi_{a_0}(T_{a_0}^{i+j-k}(y)) \,dy \bigg| \leq C \cdot {k(i,j)^{(\kappa_0+1)}} \rho^{i+j-k(i,j)} \nonumber \\ &\quad \leq C \cdot (i+j)^{\kappa_0+1} \rho^{\eta(j-i^\delta)/(1+\eta)} \leq C \cdot (2j)^{(\kappa_0+1)/\delta} \rho^{\eta j/(2+2\eta)}. \end{align} $$
$$ \begin{align} &\frac1{|x_{k}(\omega)|} \bigg| \int_{x_{k}(\omega)} \varphi_{a_0}(T_{a_0}^{i+j-k}(y)) \,dy \bigg| \leq C \cdot {k(i,j)^{(\kappa_0+1)}} \rho^{i+j-k(i,j)} \nonumber \\ &\quad \leq C \cdot (i+j)^{\kappa_0+1} \rho^{\eta(j-i^\delta)/(1+\eta)} \leq C \cdot (2j)^{(\kappa_0+1)/\delta} \rho^{\eta j/(2+2\eta)}. \end{align} $$
(We used
![]() $|x_{k}(\omega )|> Ck^{-\kappa _0+1}$
from Lemma 2.3.) Putting together equations (4.13), (4.14), (4.15) and (4.10), we conclude the proof of equation (4.3).
$|x_{k}(\omega )|> Ck^{-\kappa _0+1}$
from Lemma 2.3.) Putting together equations (4.13), (4.14), (4.15) and (4.10), we conclude the proof of equation (4.3).
4.2 Law of large numbers for
 $y_j^2$
$y_j^2$
Recall that
![]() $\gamma \in (2/5,1/2)$
is fixed. The main ingredient in the proof of Theorem 1.1 is the following analogue of [Reference SchnellmannSch, Lemma 6.2], itself inspired by [Reference Philipp and StoutPS, Lemma 3.3.1].
$\gamma \in (2/5,1/2)$
is fixed. The main ingredient in the proof of Theorem 1.1 is the following analogue of [Reference SchnellmannSch, Lemma 6.2], itself inspired by [Reference Philipp and StoutPS, Lemma 3.3.1].
Lemma 4.2. For
![]() $m_{*}$
-a.e.
$m_{*}$
-a.e.
![]() $a \in \Omega _{*}^\varphi $
, there exists
$a \in \Omega _{*}^\varphi $
, there exists
![]() $C(a)$
such that
$C(a)$
such that
 $$ \begin{align} \bigg| N - \sum_{j=1}^{M(N)}y_j^2(a) \bigg| \leq C(a) N^{2\gamma} \quad \text{for all } N \geq 1. \end{align} $$
$$ \begin{align} \bigg| N - \sum_{j=1}^{M(N)}y_j^2(a) \bigg| \leq C(a) N^{2\gamma} \quad \text{for all } N \geq 1. \end{align} $$
The proof of Lemma 4.2 (which uses Proposition 3.2 and equation (4.2), but not equation (4.3)) is based on the following theorem ([Reference Gál and KoksmaGK], see also [Reference Philipp and StoutPS, Theorem A.1]).
Theorem 4.3. (Gál–Koksma’s strong law of large numbers)
Let
![]() $z_j$
,
$z_j$
,
![]() $j\ge 1$
, be zero-mean random variables. Assume there exist
$j\ge 1$
, be zero-mean random variables. Assume there exist
![]() $p\ge 1$
and
$p\ge 1$
and
![]() $C<\infty $
with
$C<\infty $
with
 $$ \begin{align*} E \bigg( \sum_{j = m + 1}^{m + n} z_j \bigg)^2 \leq C((m + n)^p - m^p) \quad \text{for all }\ m \geq 0\ \text{and}\ n \geq 1. \end{align*} $$
$$ \begin{align*} E \bigg( \sum_{j = m + 1}^{m + n} z_j \bigg)^2 \leq C((m + n)^p - m^p) \quad \text{for all }\ m \geq 0\ \text{and}\ n \geq 1. \end{align*} $$
Then, for all
![]() $\iota>0$
, we have
$\iota>0$
, we have
![]() $(1/{n^{p/2 + \iota }}) \sum _{j = 1}^n z_j \to 0$
almost surely.
$(1/{n^{p/2 + \iota }}) \sum _{j = 1}^n z_j \to 0$
almost surely.
Proof of Lemma 4.2
Set
![]() $w_j = \sum _{i \in \mathbb {I}_j} \xi _i$
. Since
$w_j = \sum _{i \in \mathbb {I}_j} \xi _i$
. Since
![]() $y_j^2 - w_j^2 = (y_j + w_j) (y_j - w_j)$
and
$y_j^2 - w_j^2 = (y_j + w_j) (y_j - w_j)$
and
![]() $|y_j + w_j|\leq C j^{2/3}$
, the bound in equation (4.2) gives C such that
$|y_j + w_j|\leq C j^{2/3}$
, the bound in equation (4.2) gives C such that
![]() $|y_j^2 - w_j^2| \leq C j^{2/3} \tilde{\unicode{x3bb}} _\mathrm{CE}^{-\theta j^\delta }$
for all
$|y_j^2 - w_j^2| \leq C j^{2/3} \tilde{\unicode{x3bb}} _\mathrm{CE}^{-\theta j^\delta }$
for all
![]() $j\ge 1$
and
$j\ge 1$
and
![]() $a \in \Omega _{*, r_{Cj^{5/3}}}$
. Hence,
$a \in \Omega _{*, r_{Cj^{5/3}}}$
. Hence,
![]() $\sup _{a\in \Omega _{*}^\varphi }\sum _{j \geq 1} |y_j^2 - w_j^2|$
is finite, and it suffices to show equation (4.16) with
$\sup _{a\in \Omega _{*}^\varphi }\sum _{j \geq 1} |y_j^2 - w_j^2|$
is finite, and it suffices to show equation (4.16) with
![]() $y_j$
replaced by
$y_j$
replaced by
![]() $w_j$
.
$w_j$
.
By equation (3.8), we have
![]() $| E (w_j^2) - \# \mathbb {I}_j | \leq C$
, and, since
$| E (w_j^2) - \# \mathbb {I}_j | \leq C$
, and, since
![]() $\sum _{j=1}^{M(N)} \# \mathbb {I}_j = N$
, we get
$\sum _{j=1}^{M(N)} \# \mathbb {I}_j = N$
, we get
![]() $ | \sum _{j=1}^{M(N)} {E( w_j^2 )- N | \leq C M(N)}$
. Therefore,
$ | \sum _{j=1}^{M(N)} {E( w_j^2 )- N | \leq C M(N)}$
. Therefore,
 $$ \begin{align} \bigg| N - \sum_{j=1}^{M(N)} w_j^2 \bigg| \leq CM(N) + \bigg| \sum_{j=1}^{M(N)} w_j^2 - E( w_j^2 )\bigg|. \end{align} $$
$$ \begin{align} \bigg| N - \sum_{j=1}^{M(N)} w_j^2 \bigg| \leq CM(N) + \bigg| \sum_{j=1}^{M(N)} w_j^2 - E( w_j^2 )\bigg|. \end{align} $$
Assume there exists C such that
 $$ \begin{align} E \bigg( \sum_{j = m + 1}^{m + n} w_j^2 - E (w_j^2) \bigg)^2 \leq C( (m + n)^{8/3 } -m^{8/3}) \quad \text{for all } m \geq 0,\ n \geq 1. \end{align} $$
$$ \begin{align} E \bigg( \sum_{j = m + 1}^{m + n} w_j^2 - E (w_j^2) \bigg)^2 \leq C( (m + n)^{8/3 } -m^{8/3}) \quad \text{for all } m \geq 0,\ n \geq 1. \end{align} $$
Then, Theorem 4.3 (Gál–Koksma) applied to
![]() $\iota \in (0, 10(\gamma -2/5)/3]$
,
$\iota \in (0, 10(\gamma -2/5)/3]$
,
![]() $p=8/3$
and the zero-mean random variables
$p=8/3$
and the zero-mean random variables
![]() $z_j=w_j^2-E( w_j^2)$
, implies that
$z_j=w_j^2-E( w_j^2)$
, implies that
 $$ \begin{align*} \sum_{j = 1}^{M(N)} w_j^2 - E (w_j^2) = o (M^{{4}/{3} + \iota}) \quad \mbox{almost surely}. \end{align*} $$
$$ \begin{align*} \sum_{j = 1}^{M(N)} w_j^2 - E (w_j^2) = o (M^{{4}/{3} + \iota}) \quad \mbox{almost surely}. \end{align*} $$
Hence, equation (4.17) gives
![]() $ | N - \sum _{j=1}^{M(N)} w_j^2(a) | \leq C(a) N^{4/5 + 3\iota /5}\le C(a)N^{2\gamma } $
, almost surely (recall
$ | N - \sum _{j=1}^{M(N)} w_j^2(a) | \leq C(a) N^{4/5 + 3\iota /5}\le C(a)N^{2\gamma } $
, almost surely (recall
![]() $M(N) \sim N^{3/5}$
by equation (4.4)). It remains to prove equation (4.18).
$M(N) \sim N^{3/5}$
by equation (4.4)). It remains to prove equation (4.18).
By Jensen’s inequality, we have
![]() $(E(w_j^2))^2 \leq E(w_j^4)$
and, therefore,
$(E(w_j^2))^2 \leq E(w_j^4)$
and, therefore,
 $$ \begin{align} E \bigg(\sum_{j = m + 1}^{m + n} w_j^2 - E (w_j^2) \bigg)^2 \leq 2 \sum_{j = m + 1}^{m + n} \bigg( E (w_j^4) + \sum_{k = j + 1}^{m + n} |E (w_j^2 w_k^2) - E (w_j^2) E (w_k^2)| \bigg). \end{align} $$
$$ \begin{align} E \bigg(\sum_{j = m + 1}^{m + n} w_j^2 - E (w_j^2) \bigg)^2 \leq 2 \sum_{j = m + 1}^{m + n} \bigg( E (w_j^4) + \sum_{k = j + 1}^{m + n} |E (w_j^2 w_k^2) - E (w_j^2) E (w_k^2)| \bigg). \end{align} $$
We consider first
![]() $E(w_j^4)$
. Fix
$E(w_j^4)$
. Fix
![]() $\upsilon \in (0,1/6)$
and, for
$\upsilon \in (0,1/6)$
and, for
![]() $j\ge 1$
, let
$j\ge 1$
, let
Then, since
![]() $\#( \{\, \vec v \in \mathbb {I}_j^4 \mid v_1 \leq v_2 \leq v_3 \leq v_4\} \setminus S_j)\le (j^{2/3 + \upsilon })^2 = j^{4/3+2\upsilon }$
, we find
$\#( \{\, \vec v \in \mathbb {I}_j^4 \mid v_1 \leq v_2 \leq v_3 \leq v_4\} \setminus S_j)\le (j^{2/3 + \upsilon })^2 = j^{4/3+2\upsilon }$
, we find
 $$ \begin{align} \int\limits_{\Omega_{*}^\varphi} w_j(a)^4 \, da &= \sum_{\vec v \in \mathbb{I}_j} \bigg| \int_{\Omega_{*}^\varphi} \prod_{\ell = 1}^4 \xi_{v_\ell} (a) \, da \bigg| \nonumber \leq C \sum_{\substack{\vec v \in \mathbb{I}_j^4 \\ v_1 \leq \cdots \leq v_4}} \bigg| \int_{\Omega_{*}^\varphi } \prod_{\ell=1}^4 \xi_{v_\ell} (a) \, da \bigg| \nonumber \\ & \leq C \sum_{\vec v\in S_j} \bigg| \int_{\Omega_{*}^\varphi} \prod_{\ell=1}^4\xi_{v_\ell}(a) \, da \bigg| + C j^{4/3+2\upsilon}. \end{align} $$
$$ \begin{align} \int\limits_{\Omega_{*}^\varphi} w_j(a)^4 \, da &= \sum_{\vec v \in \mathbb{I}_j} \bigg| \int_{\Omega_{*}^\varphi} \prod_{\ell = 1}^4 \xi_{v_\ell} (a) \, da \bigg| \nonumber \leq C \sum_{\substack{\vec v \in \mathbb{I}_j^4 \\ v_1 \leq \cdots \leq v_4}} \bigg| \int_{\Omega_{*}^\varphi } \prod_{\ell=1}^4 \xi_{v_\ell} (a) \, da \bigg| \nonumber \\ & \leq C \sum_{\vec v\in S_j} \bigg| \int_{\Omega_{*}^\varphi} \prod_{\ell=1}^4\xi_{v_\ell}(a) \, da \bigg| + C j^{4/3+2\upsilon}. \end{align} $$
Let
![]() $\vec v\in S_j$
be such that
$\vec v\in S_j$
be such that
![]() $v_4-v_3 \geq j^\upsilon $
. For
$v_4-v_3 \geq j^\upsilon $
. For
![]() $\omega \in \mathcal {P}_{v_3}$
such that
$\omega \in \mathcal {P}_{v_3}$
such that
![]() $\omega \cap \Omega _{*}^\varphi \ne \emptyset $
, the change of variable in equation (3.13), together with an easy variant of Lemma 3.3 deduced from equation (3.14), give
$\omega \cap \Omega _{*}^\varphi \ne \emptyset $
, the change of variable in equation (3.13), together with an easy variant of Lemma 3.3 deduced from equation (3.14), give
![]() $a_0\in \omega \cap \Omega _{*}^\varphi $
such that
$a_0\in \omega \cap \Omega _{*}^\varphi $
such that

For
![]() $y\in x_{v_3}(\omega )$
, setting
$y\in x_{v_3}(\omega )$
, setting
![]() $a = x_{v_3}|_\omega ^{-1} (y)$
, and recalling Remark 3.1, we find
$a = x_{v_3}|_\omega ^{-1} (y)$
, and recalling Remark 3.1, we find
 $$ \begin{align*} &|\xi_{v_\ell} (x_{v_3}|_\omega^{-1} (y)) - \varphi_{a_0} (x_{v_\ell} \circ x_{v_3}|_\omega^{-1}(y))| \\ &\quad = |\varphi_a (x_{v_\ell} \circ x_{v_3}|_\omega^{-1}(y)) - \varphi_{a_0} (x_{v_\ell} \circ x_{v_3}|_\omega^{-1}(y))| \leq C |\omega|^\theta \end{align*} $$
$$ \begin{align*} &|\xi_{v_\ell} (x_{v_3}|_\omega^{-1} (y)) - \varphi_{a_0} (x_{v_\ell} \circ x_{v_3}|_\omega^{-1}(y))| \\ &\quad = |\varphi_a (x_{v_\ell} \circ x_{v_3}|_\omega^{-1}(y)) - \varphi_{a_0} (x_{v_\ell} \circ x_{v_3}|_\omega^{-1}(y))| \leq C |\omega|^\theta \end{align*} $$
for
![]() $\ell =1, 2, 3$
. Thus, equations (2.9) and (2.7) imply (using
$\ell =1, 2, 3$
. Thus, equations (2.9) and (2.7) imply (using
![]() $\sup _k\|\xi _k\|_{L^\infty }<\infty $
)
$\sup _k\|\xi _k\|_{L^\infty }<\infty $
)

We claim that for
![]() $\ell =1,2,3$
, and for each
$\ell =1,2,3$
, and for each
![]() $\omega \in \mathcal {P}_{*, v_3}$
,
$\omega \in \mathcal {P}_{*, v_3}$
,
Indeed, by equation (2.29), there exists
![]() $a \in \omega $
such that
$a \in \omega $
such that
Thus, if
![]() $a\in \Omega _{*}$
, standard arguments (see e.g. [Reference Baladi and SmaniaBS1, Proposition 3.7], using our polynomial recurrence in equation (2.8)) give the claim. Otherwise, since
$a\in \Omega _{*}$
, standard arguments (see e.g. [Reference Baladi and SmaniaBS1, Proposition 3.7], using our polynomial recurrence in equation (2.8)) give the claim. Otherwise, since
![]() $|\omega \cap \Omega _{*}|>0$
, we may use equation (2.31) as for equation (4.6).
$|\omega \cap \Omega _{*}|>0$
, we may use equation (2.31) as for equation (4.6).
Therefore, we find C such that for each
![]() $v_3$
and
$v_3$
and
![]() $\omega \in \mathcal {P}_{*, v_3}$
,
$\omega \in \mathcal {P}_{*, v_3}$
,
 $$ \begin{align*} \| 1_{x_{v_3} (\omega)} \cdot \prod_{\ell=1}^3(\varphi_{a_0} \circ x_{v_\ell} \circ x_{v_3}|_\omega^{-1}) \|_{H^s_q} \leq C (v_1 v_2)^{\varpi \kappa_0}\| \varphi_{a_0} \|^2_{C^\varpi} \| \varphi_{a_0} \|_{H^s_q}. \end{align*} $$
$$ \begin{align*} \| 1_{x_{v_3} (\omega)} \cdot \prod_{\ell=1}^3(\varphi_{a_0} \circ x_{v_\ell} \circ x_{v_3}|_\omega^{-1}) \|_{H^s_q} \leq C (v_1 v_2)^{\varpi \kappa_0}\| \varphi_{a_0} \|^2_{C^\varpi} \| \varphi_{a_0} \|_{H^s_q}. \end{align*} $$
Indeed, on the one hand, there exists C such that, for any
![]() $C^2$
map
$C^2$
map
![]() $\mathcal {T}$
, we have
$\mathcal {T}$
, we have
On the other hand, since
![]() $0<s<1/q<1$
, the characteristic function of an interval is a bounded multiplier on
$0<s<1/q<1$
, the characteristic function of an interval is a bounded multiplier on
![]() $H^s_q(I)$
(uniformly in the size of the interval), and since
$H^s_q(I)$
(uniformly in the size of the interval), and since
![]() $s<\varpi $
, a function in
$s<\varpi $
, a function in
![]() $C^\varpi $
is a bounded multiplier on
$C^\varpi $
is a bounded multiplier on
![]() $H^s_q(I)$
[Reference StrichartzSt, Reference ThomineTh].
$H^s_q(I)$
[Reference StrichartzSt, Reference ThomineTh].
Hence, by the first claim of Proposition 2.5 (with equation (3.2) and
![]() $\int \varphi _{a_0} d\mu _{a_0}=0$
), we have
$\int \varphi _{a_0} d\mu _{a_0}=0$
), we have
 $$ \begin{align*} \frac{| \int_{x_{v_3}(\omega)} ( \prod_{\ell=1}^3 \varphi_{a_0}(x_{v_\ell} \circ x_{v_3}|_\omega^{-1}) ) \varphi_{a_0}(T_{a_0}^{v_4-v_3}) \, dy |}{|x_{v_3}(\omega)|} &\le C (v_1 v_2)^{\varpi \kappa_0} \frac{\rho^{v_4-v_3}}{|x_{v_3}(\omega)|}\\ &\le C j^{10\varpi \kappa_0/3} v_3^{\kappa_1}\rho^{j^\upsilon}. \end{align*} $$
$$ \begin{align*} \frac{| \int_{x_{v_3}(\omega)} ( \prod_{\ell=1}^3 \varphi_{a_0}(x_{v_\ell} \circ x_{v_3}|_\omega^{-1}) ) \varphi_{a_0}(T_{a_0}^{v_4-v_3}) \, dy |}{|x_{v_3}(\omega)|} &\le C (v_1 v_2)^{\varpi \kappa_0} \frac{\rho^{v_4-v_3}}{|x_{v_3}(\omega)|}\\ &\le C j^{10\varpi \kappa_0/3} v_3^{\kappa_1}\rho^{j^\upsilon}. \end{align*} $$
(We used Lemma 2.3 and that
![]() $v_\ell \in \mathbb {I}_j$
implies
$v_\ell \in \mathbb {I}_j$
implies
![]() $v_\ell \leq C j^{5/3}$
.) Next,
$v_\ell \leq C j^{5/3}$
.) Next,

for all
![]() $\vec v\in S_j$
with
$\vec v\in S_j$
with
![]() $v_4-v_3\ge j^\upsilon $
(if j is large enough).
$v_4-v_3\ge j^\upsilon $
(if j is large enough).
Let now
![]() $\vec v\in S_j$
with
$\vec v\in S_j$
with
![]() $v_2-v_1\ge j^\upsilon $
. Then applying directly Lemma 3.3 with
$v_2-v_1\ge j^\upsilon $
. Then applying directly Lemma 3.3 with
![]() $\ell _0=4$
, a similar reasoning gives
$\ell _0=4$
, a similar reasoning gives
![]() $|\int _{\Omega _{*}^\varphi }\prod _{\ell =1}^4\xi _{v_\ell } \, da|\le C e_{[Cj^{5/3}]}$
.
$|\int _{\Omega _{*}^\varphi }\prod _{\ell =1}^4\xi _{v_\ell } \, da|\le C e_{[Cj^{5/3}]}$
.
Finally, since
![]() $\# S_j\le \# \mathbb {I}_j^4 \le j^{8/3}$
and
$\# S_j\le \# \mathbb {I}_j^4 \le j^{8/3}$
and
![]() $e_j\le j^{-d_1 \kappa _0+1}$
with
$e_j\le j^{-d_1 \kappa _0+1}$
with
![]() $d_1 \kappa _0\ge 3>9/5$
, the bound in equation (4.20) gives C such that
$d_1 \kappa _0\ge 3>9/5$
, the bound in equation (4.20) gives C such that
(For the purposes of the present lemma, a version of equation (4.23) with
![]() $Cj^{5/3}$
in the right-hand side would suffice. The stronger statement is needed for equation (4.31).)
$Cj^{5/3}$
in the right-hand side would suffice. The stronger statement is needed for equation (4.31).)
We next bound
![]() $|E (w_j^2 w_k^2) - E (w_j^2) E(w_k^2)|$
for
$|E (w_j^2 w_k^2) - E (w_j^2) E(w_k^2)|$
for
![]() $k\ge j+1$
. If
$k\ge j+1$
. If
![]() $k = j + 1$
, by Cauchy’s inequality and equation (4.23),
$k = j + 1$
, by Cauchy’s inequality and equation (4.23),
By equation (3.8), we have
![]() $ E (w_{j}^2) E( w_{j + 1}^2) \leq C j^{4/3}$
. Hence,
$ E (w_{j}^2) E( w_{j + 1}^2) \leq C j^{4/3}$
. Hence,
Assume now that
![]() $k \geq j+2$
. By construction,
$k \geq j+2$
. By construction,
![]() $y_j$
is constant on elements of
$y_j$
is constant on elements of
![]() $\mathcal {P}_v$
if
$\mathcal {P}_v$
if
![]() ${ v \geq r_{j_1}=j_1 + [j_1^\delta ]} $
, where
${ v \geq r_{j_1}=j_1 + [j_1^\delta ]} $
, where
![]() $j_1$
is the largest number in
$j_1$
is the largest number in
![]() $\mathbb {I}_j$
. Let
$\mathbb {I}_j$
. Let
Then, for large enough j, using that
![]() $x\mapsto x-x^{1/4}$
is increasing for large x, we find
$x\mapsto x-x^{1/4}$
is increasing for large x, we find
Since
![]() $k\ge j+2$
and
$k\ge j+2$
and
![]() $\delta < \tfrac 14$
, we have that
$\delta < \tfrac 14$
, we have that
![]() $y_j$
is constant on elements of
$y_j$
is constant on elements of
![]() $\mathcal {P}_v$
for
$\mathcal {P}_v$
for
Lemma 2.3 gives
![]() $|x_{v (k_0)}(\omega )| \geq \unicode{x3bb} _0^{-{k_0}^{1/4}}$
if
$|x_{v (k_0)}(\omega )| \geq \unicode{x3bb} _0^{-{k_0}^{1/4}}$
if
![]() $\omega \in \mathcal {P}_{v(k_0)}$
. Thus, there exists a refinement
$\omega \in \mathcal {P}_{v(k_0)}$
. Thus, there exists a refinement
![]() $\mathcal {Q}_{v(k_0)}$
of
$\mathcal {Q}_{v(k_0)}$
of
![]() $\mathcal {P}_{v(k_0)}$
such that
$\mathcal {P}_{v(k_0)}$
such that
Therefore, for large enough k, the local bound in equation (3.9) in Proposition 3.2 gives for all
![]() $\omega \in \mathcal {Q}_{v}$
with non-empty intersection with
$\omega \in \mathcal {Q}_{v}$
with non-empty intersection with
![]() $\Omega _{*}^\varphi $
that
$\Omega _{*}^\varphi $
that
 $$ \begin{align*} \bigg| \frac{1}{|\omega|} \int_\omega w_k^2 \, dm -\# \mathbb{I}_k \bigg| \leq C , \end{align*} $$
$$ \begin{align*} \bigg| \frac{1}{|\omega|} \int_\omega w_k^2 \, dm -\# \mathbb{I}_k \bigg| \leq C , \end{align*} $$
since
![]() $n=\# \mathbb {I}_k=[k^{2/3}]\le \eta k_0/2$
. As in equation (3.10), we write
$n=\# \mathbb {I}_k=[k^{2/3}]\le \eta k_0/2$
. As in equation (3.10), we write
![]() $\mathcal {Q}_{*,v}$
for the set of
$\mathcal {Q}_{*,v}$
for the set of
![]() $\omega \in \mathcal {Q}_{v}$
with non-empty intersection with
$\omega \in \mathcal {Q}_{v}$
with non-empty intersection with
![]() $\Omega _{*}^\varphi $
, and
$\Omega _{*}^\varphi $
, and
![]() $\Omega _{*,v}^{\mathcal {Q}} = \cup \mathcal {Q}_{*,v}$
. Thus, using that
$\Omega _{*,v}^{\mathcal {Q}} = \cup \mathcal {Q}_{*,v}$
. Thus, using that
![]() $y_j$
is constant on each
$y_j$
is constant on each
![]() $\omega \in \mathcal {Q}_v$
(since
$\omega \in \mathcal {Q}_v$
(since
![]() $\mathcal {Q}_{v}$
refines
$\mathcal {Q}_{v}$
refines
![]() $\mathcal {P}_{v}$
),
$\mathcal {P}_{v}$
),
 $$ \begin{align*} \int_{\Omega_{*,v}^{\mathcal {Q}}} (y_j^2 w_k^2) \, d m &= \sum_{\omega \in \mathcal{Q}_{v}^*} |\omega| \cdot y_j^2|_\omega \cdot \frac{1}{|\omega|} \int_\omega w_k^2\, dm\\ & \in \bigg[ \int_{\Omega_{*,v}^{\mathcal {Q}}} y_j^2 \, dm (\# \mathbb{I}_k - C), \int_{\Omega_{*,v}^{\mathcal {Q}}} y_j^2 \, dm (\# \mathbb{I}_k + C) \bigg]. \end{align*} $$
$$ \begin{align*} \int_{\Omega_{*,v}^{\mathcal {Q}}} (y_j^2 w_k^2) \, d m &= \sum_{\omega \in \mathcal{Q}_{v}^*} |\omega| \cdot y_j^2|_\omega \cdot \frac{1}{|\omega|} \int_\omega w_k^2\, dm\\ & \in \bigg[ \int_{\Omega_{*,v}^{\mathcal {Q}}} y_j^2 \, dm (\# \mathbb{I}_k - C), \int_{\Omega_{*,v}^{\mathcal {Q}}} y_j^2 \, dm (\# \mathbb{I}_k + C) \bigg]. \end{align*} $$
Recall that
![]() $j\le k-2$
. Since
$j\le k-2$
. Since
![]() $|y_j^2| \le Cj^{4/3}\le Ck^{4/3}$
, we get
$|y_j^2| \le Cj^{4/3}\le Ck^{4/3}$
, we get
 $$ \begin{align*} \frac 1 {m(\Omega_{*,v}^{\mathcal {Q}})}\int_{\Omega_{*,v}^{\mathcal {Q}}} y_j^2 \, d m = E(y_j^2) + O(1), \end{align*} $$
$$ \begin{align*} \frac 1 {m(\Omega_{*,v}^{\mathcal {Q}})}\int_{\Omega_{*,v}^{\mathcal {Q}}} y_j^2 \, d m = E(y_j^2) + O(1), \end{align*} $$
by equation (3.11) applied to
![]() $\Psi _k=y_j^2$
, and since
$\Psi _k=y_j^2$
, and since
![]() $|y_j^2w_k^2| \le C k^{8/3}$
, we have
$|y_j^2w_k^2| \le C k^{8/3}$
, we have
 $$ \begin{align*} \frac 1 {m(\Omega_{*,v}^{\mathcal {Q}})}\int_{\Omega_{*,v}^{\mathcal {Q}}} (y_j^2 w_k^2) \, d m = E(y_j^2 w_k^2) + O(1), \end{align*} $$
$$ \begin{align*} \frac 1 {m(\Omega_{*,v}^{\mathcal {Q}})}\int_{\Omega_{*,v}^{\mathcal {Q}}} (y_j^2 w_k^2) \, d m = E(y_j^2 w_k^2) + O(1), \end{align*} $$
by equation (3.11) applied to
![]() $\Psi _k=(y_j^2 w_k^2)$
. That is,
$\Psi _k=(y_j^2 w_k^2)$
. That is,
Next, the global estimate in equation (3.8) in Proposition 3.2 gives
![]() $|E (y_j^2) E (w_k^2) - \# \mathbb {I}_k E (y_j^2)| \leq C E (y_j^2)$
. Therefore, (the expression
$|E (y_j^2) E (w_k^2) - \# \mathbb {I}_k E (y_j^2)| \leq C E (y_j^2)$
. Therefore, (the expression
![]() $\#\mathbb {I}_j=j^b=j^{2/3}$
in the right-hand side already leads to
$\#\mathbb {I}_j=j^b=j^{2/3}$
in the right-hand side already leads to
![]() $\gamma>2/5$
. A block size
$\gamma>2/5$
. A block size
![]() $\#\mathbb {I}_j=j^b$
replaces
$\#\mathbb {I}_j=j^b$
replaces
![]() $3/5$
in equation (4.4) by
$3/5$
in equation (4.4) by
![]() $1/(1+b)$
, so that the first constraint becomes
$1/(1+b)$
, so that the first constraint becomes
![]() $N^\gamma> N^{b/(1+b)}$
, see equation (4.5). Our bounds in equations (4.25) and (4.26) (with Gál–Koksma’s strong law of large numbers, Theorem 4.3, and
$N^\gamma> N^{b/(1+b)}$
, see equation (4.5). Our bounds in equations (4.25) and (4.26) (with Gál–Koksma’s strong law of large numbers, Theorem 4.3, and
![]() $M(N)\sim N^{1/(1+b)}$
) give
$M(N)\sim N^{1/(1+b)}$
) give
![]() ${N^{\gamma }> N^{(b+2)/(4(b+1))}}$
. Hence,
${N^{\gamma }> N^{(b+2)/(4(b+1))}}$
. Hence,
![]() $b=2/3$
is the optimum. In the independent and identically distributed case, a block size
$b=2/3$
is the optimum. In the independent and identically distributed case, a block size
![]() $j^{1/2}$
gives
$j^{1/2}$
gives
![]() $\gamma>1/3$
[Reference Philipp and StoutPS, p. 25], see also the beginning of [Reference SchnellmannSch, §6])
$\gamma>1/3$
[Reference Philipp and StoutPS, p. 25], see also the beginning of [Reference SchnellmannSch, §6])
Hence, for large enough j and all
![]() $k\ge j+2$
, since
$k\ge j+2$
, since
![]() $\sup |w_j+y_j|\le C \# \mathbb {I}_{j}$
,
$\sup |w_j+y_j|\le C \# \mathbb {I}_{j}$
,

(We used equation (4.2) to get
![]() $\sup |w_j - y_j|\le C \# \mathbb {I}_j \tilde{\unicode{x3bb}} _\mathrm{CE}^{-\theta j^{5\delta /3}}$
.)
$\sup |w_j - y_j|\le C \# \mathbb {I}_j \tilde{\unicode{x3bb}} _\mathrm{CE}^{-\theta j^{5\delta /3}}$
.)
Finally, we plug equations (4.25), (4.24) and (4.23) into equation (4.19), and get, since
![]() ${2\upsilon <1/3}$
,
${2\upsilon <1/3}$
,

This proves equation (4.18).
4.3 Martingale differences
 $Y_j$
. Skorokhod’s representation theorem
$Y_j$
. Skorokhod’s representation theorem
As in Schnellmann’s adaptation of [Reference Philipp and StoutPS, §§3.4 and 3.5] in [Reference SchnellmannSch, §6.3], let
![]() $\mathcal {L}_j$
be the
$\mathcal {L}_j$
be the
![]() $\sigma $
-algebra generated by
$\sigma $
-algebra generated by
![]() $\{y_\ell \}_{1\le \ell \le j}$
, and set
$\{y_\ell \}_{1\le \ell \le j}$
, and set
Then,
![]() $\{Y_j,\mathcal {L}_j\}$
is a martingale difference sequence. Using equation (4.3), we show that
$\{Y_j,\mathcal {L}_j\}$
is a martingale difference sequence. Using equation (4.3), we show that
![]() $\{Y_j\}$
inherits the law of large numbers established for
$\{Y_j\}$
inherits the law of large numbers established for
![]() $\{y_j\}$
in Lemma 4.2:
$\{y_j\}$
in Lemma 4.2:
Lemma 4.4. For
![]() $m_{*}$
-a.e.
$m_{*}$
-a.e.
![]() $a \in \Omega _{*}^\varphi $
, there exists
$a \in \Omega _{*}^\varphi $
, there exists
![]() $C(a)$
such that
$C(a)$
such that
 $$ \begin{align} \bigg| N -\sum_{j=1}^{M(N)} Y_j^2 (a) \bigg| \leq C(a)N^{2\gamma} \quad \text{for all } N \geq 1, \end{align} $$
$$ \begin{align} \bigg| N -\sum_{j=1}^{M(N)} Y_j^2 (a) \bigg| \leq C(a)N^{2\gamma} \quad \text{for all } N \geq 1, \end{align} $$
and
 $$ \begin{align} \bigg| \sum_{j = 1}^{M(N)} E (Y_j^2 \mid \mathcal{L}_{j - 1}) - Y_j^2 (a) \bigg| \leq C(a) N^{2 \gamma} \quad \text{for all } N \geq 1. \end{align} $$
$$ \begin{align} \bigg| \sum_{j = 1}^{M(N)} E (Y_j^2 \mid \mathcal{L}_{j - 1}) - Y_j^2 (a) \bigg| \leq C(a) N^{2 \gamma} \quad \text{for all } N \geq 1. \end{align} $$
Proof. Recalling the
![]() $\sigma $
-algebra
$\sigma $
-algebra
![]() $\mathcal {F}_{r_i}$
generated by the intervals in
$\mathcal {F}_{r_i}$
generated by the intervals in
![]() $\mathcal {P}_{r_i}$
, we have
$\mathcal {P}_{r_i}$
, we have
![]() ${\mathcal {L}_{\ell -1} \subset \mathcal {F}_{r_{i(\ell )}}}$
, where
${\mathcal {L}_{\ell -1} \subset \mathcal {F}_{r_{i(\ell )}}}$
, where
![]() $i(\ell ) = \operatorname \max \{i \in \mathbb {I}_{\ell - 1} \} \leq C \ell ^{5/3}$
by equation (4.4). Then,
$i(\ell ) = \operatorname \max \{i \in \mathbb {I}_{\ell - 1} \} \leq C \ell ^{5/3}$
by equation (4.4). Then,
 $$ \begin{align*} u_\ell = \sum_{j \geq 1} E ( E(\xi_{i(\ell) + j} \mid \mathcal{F}_{r_{i(\ell)}}) \mid \mathcal{L}_{\ell - 1}). \end{align*} $$
$$ \begin{align*} u_\ell = \sum_{j \geq 1} E ( E(\xi_{i(\ell) + j} \mid \mathcal{F}_{r_{i(\ell)}}) \mid \mathcal{L}_{\ell - 1}). \end{align*} $$
Since
![]() $\sum _{j=1}^\infty e_j < \infty $
, the bound in equation (4.3) in Lemma 4.1 gives
$\sum _{j=1}^\infty e_j < \infty $
, the bound in equation (4.3) in Lemma 4.1 gives
 $$ \begin{align} |u_\ell (a)| \leq \sum_{j \geq 1} C \operatorname\min \{1, e_{ [ \eta (j - 2 i(\ell)^\delta))]} \} \leq \frac {2 C}{ \eta} i(\ell)^\delta \leq C \ell^{5 \delta / 3}. \end{align} $$
$$ \begin{align} |u_\ell (a)| \leq \sum_{j \geq 1} C \operatorname\min \{1, e_{ [ \eta (j - 2 i(\ell)^\delta))]} \} \leq \frac {2 C}{ \eta} i(\ell)^\delta \leq C \ell^{5 \delta / 3}. \end{align} $$
Put
![]() $v_j = u_j - u_{j + 1}$
, so that
$v_j = u_j - u_{j + 1}$
, so that
![]() $Y_j^2 = y_j^2 -2 y_j v_j + v_j^2$
.
$Y_j^2 = y_j^2 -2 y_j v_j + v_j^2$
.
We claim that equation (4.28) follows if for a.e.
![]() $a\in \Omega ^\varphi _{*}$
, there exists C such that
$a\in \Omega ^\varphi _{*}$
, there exists C such that
![]() $\sum _{j = 1}^{M(N)} v_j^2 \leq C N^{4 \gamma -1}$
. Indeed, since
$\sum _{j = 1}^{M(N)} v_j^2 \leq C N^{4 \gamma -1}$
. Indeed, since
![]() $\gamma <1/2$
, Lemma 4.2 and Cauchy’s inequality then give (using
$\gamma <1/2$
, Lemma 4.2 and Cauchy’s inequality then give (using
![]() $\sum _{j = 1}^{M(N)} y_j^2\le C N$
)
$\sum _{j = 1}^{M(N)} y_j^2\le C N$
)
 $$ \begin{align*} \bigg| N - \sum_{j = 1}^{M(N)} Y_j^2 \bigg| & = \bigg| N - \sum_{j = 1}^{M(N)} (y_j^2 -2 y_j v_j + v_j^2) \bigg| \\ & \leq \bigg| N - \sum_{j = 1}^{M(N)} y_j^2 \bigg|+ \sum_{j=1}^{M(N)} v_j^2 + 2 \sqrt{\sum_{j = 1}^{M(N)} y_j^2 \sum_{j = 1}^{M(N)} v_j^2 } \\ & \leq C(a) N^{2 \gamma} + C N^{2 \gamma} + C \sqrt{N N^{4 \gamma-1}} \leq C(a) N^{2 \gamma}. \end{align*} $$
$$ \begin{align*} \bigg| N - \sum_{j = 1}^{M(N)} Y_j^2 \bigg| & = \bigg| N - \sum_{j = 1}^{M(N)} (y_j^2 -2 y_j v_j + v_j^2) \bigg| \\ & \leq \bigg| N - \sum_{j = 1}^{M(N)} y_j^2 \bigg|+ \sum_{j=1}^{M(N)} v_j^2 + 2 \sqrt{\sum_{j = 1}^{M(N)} y_j^2 \sum_{j = 1}^{M(N)} v_j^2 } \\ & \leq C(a) N^{2 \gamma} + C N^{2 \gamma} + C \sqrt{N N^{4 \gamma-1}} \leq C(a) N^{2 \gamma}. \end{align*} $$
However, since we have
![]() $ v_j^2 \leq C j^{10\delta /3}$
(by equation (4.30)), we find, using
$ v_j^2 \leq C j^{10\delta /3}$
(by equation (4.30)), we find, using
![]() $\delta <2(\gamma -2/5)$
,
$\delta <2(\gamma -2/5)$
,
 $$ \begin{align*} \sum_{j = 1}^{M(N)} v_j^2 \leq C M^{1 + 10 \delta / 3} \leq N^{3 / 5 + 2 \delta} \leq C N^{4 \gamma-1}. \end{align*} $$
$$ \begin{align*} \sum_{j = 1}^{M(N)} v_j^2 \leq C M^{1 + 10 \delta / 3} \leq N^{3 / 5 + 2 \delta} \leq C N^{4 \gamma-1}. \end{align*} $$
It remains to prove equation (4.29). Set
![]() $R_j = Y_j^2 - E (Y_j^2 \mid \mathcal {L}_{j - 1})$
and observe that
$R_j = Y_j^2 - E (Y_j^2 \mid \mathcal {L}_{j - 1})$
and observe that
![]() $\{R_j, \mathcal {L}_j\}$
is a martingale difference sequence. By Minkowski’s inequality
$\{R_j, \mathcal {L}_j\}$
is a martingale difference sequence. By Minkowski’s inequality
 $$ \begin{align*} E (R_j^2) \leq \bigg(\sqrt{E (Y_j^4)} + \sqrt{E (E (Y_j^2 \mid \mathcal{L}_{j-1})^2)} \bigg)^2 \leq \bigg( 2 \sqrt{E ( Y_j^4)} \bigg)^2 = 4 E (Y_j^4). \end{align*} $$
$$ \begin{align*} E (R_j^2) \leq \bigg(\sqrt{E (Y_j^4)} + \sqrt{E (E (Y_j^2 \mid \mathcal{L}_{j-1})^2)} \bigg)^2 \leq \bigg( 2 \sqrt{E ( Y_j^4)} \bigg)^2 = 4 E (Y_j^4). \end{align*} $$
Since
![]() $Y_j = y_j - v_j$
, we have, again by Minkowski’s inequality,
$Y_j = y_j - v_j$
, we have, again by Minkowski’s inequality,
 $$ \begin{align*} E (R_j^2) &\leq 4 E (Y_j^4) \leq 4 ( (E( y_j^4))^{\frac{1}{4}} + (E ( v_j^4))^{\frac{1}{4}} )^4 \leq C (E (y_j^4) + E (v_j^4)) \\ &\leq C (E (w_j^4 )+ E (|w_j^4 - y_j^4|) + E(v_j^4)). \end{align*} $$
$$ \begin{align*} E (R_j^2) &\leq 4 E (Y_j^4) \leq 4 ( (E( y_j^4))^{\frac{1}{4}} + (E ( v_j^4))^{\frac{1}{4}} )^4 \leq C (E (y_j^4) + E (v_j^4)) \\ &\leq C (E (w_j^4 )+ E (|w_j^4 - y_j^4|) + E(v_j^4)). \end{align*} $$
Since
![]() $w_j^4 - y_j^4 = (w_j^2 + y_j^2) (w_j + y_j) (w_j - y_j)$
, we get from equation (4.2) that
$w_j^4 - y_j^4 = (w_j^2 + y_j^2) (w_j + y_j) (w_j - y_j)$
, we get from equation (4.2) that
![]() $E (|w_j^4 - y_j^4|)$
is uniformly bounded. By equation (4.30), we have
$E (|w_j^4 - y_j^4|)$
is uniformly bounded. By equation (4.30), we have
![]() $|u_j| \leq C j^{5\delta /3}$
. Hence,
$|u_j| \leq C j^{5\delta /3}$
. Hence,
![]() $|v_j| \leq |u_j| + |u_{j-1}| \leq C j^{5\delta /3}$
and
$|v_j| \leq |u_j| + |u_{j-1}| \leq C j^{5\delta /3}$
and
![]() $E (v_j^4) \leq C j^{20 \delta /3} \leq C j^{4/3}$
, since
$E (v_j^4) \leq C j^{20 \delta /3} \leq C j^{4/3}$
, since
![]() $\delta <1/5$
. For arbitrary
$\delta <1/5$
. For arbitrary
![]() $\iota>0$
, the bound in equation (4.23) gives C such that
$\iota>0$
, the bound in equation (4.23) gives C such that
![]() $E (w_j^4) \leq C j^{4/3 + \iota }$
. Thus,
$E (w_j^4) \leq C j^{4/3 + \iota }$
. Thus,
 $$ \begin{align} \sum_{j \geq 1} \frac{E (R_j^2) }{j^{7/3 + \iota}}< \infty , \end{align} $$
$$ \begin{align} \sum_{j \geq 1} \frac{E (R_j^2) }{j^{7/3 + \iota}}< \infty , \end{align} $$
and a martingale result (see [Reference ChowCh]) implies that
![]() $\sum _{j\ge 1}R_j/j^{7/6+\iota }$
converges almost surely. For
$\sum _{j\ge 1}R_j/j^{7/6+\iota }$
converges almost surely. For
![]() $m_{*}$
-a.e.
$m_{*}$
-a.e.
![]() $a \in \Omega _{*}^\varphi $
, Kronecker’s lemma gives
$a \in \Omega _{*}^\varphi $
, Kronecker’s lemma gives
![]() $C(a)$
with
$C(a)$
with
 $$ \begin{align*} \sum_{j = 1}^{M(N)} R_j \leq C(a) M^{7/6 + \iota} \leq C(a) N^{21/30 + \iota} , \end{align*} $$
$$ \begin{align*} \sum_{j = 1}^{M(N)} R_j \leq C(a) M^{7/6 + \iota} \leq C(a) N^{21/30 + \iota} , \end{align*} $$
using equation (4.4) in the last inequality. Since
![]() $21 / 30 < 2 \gamma $
, this establishes equation (4.29).
$21 / 30 < 2 \gamma $
, this establishes equation (4.29).
We shall apply the following embedding result. (See [Reference Hall and HeydeHH, Theorem A.1].)
Theorem 4.5. (Skorokhod’s representation theorem)
For any zero-mean square-integrable martingale
![]() $\{ \sum _{k=1}^j Y_k,\ \mathcal {L}_j \mid j \geq 1\} $
, there exist a probability space supporting a (standard) Brownian motion W, and non-negative variables
$\{ \sum _{k=1}^j Y_k,\ \mathcal {L}_j \mid j \geq 1\} $
, there exist a probability space supporting a (standard) Brownian motion W, and non-negative variables
![]() $\{T_k, \, \, k \geq 1\}$
, such that
$\{T_k, \, \, k \geq 1\}$
, such that
![]() $\{ \sum _{k=1}^j Y_k \}_{j \geq 1}$
and
$\{ \sum _{k=1}^j Y_k \}_{j \geq 1}$
and
![]() $\{ W(\sum _{k=1}^j T_k) \}_{j \geq 1}$
have the same distribution, and, in addition, letting
$\{ W(\sum _{k=1}^j T_k) \}_{j \geq 1}$
have the same distribution, and, in addition, letting
![]() $\mathcal {G}_0$
be the trivial
$\mathcal {G}_0$
be the trivial
![]() $\sigma $
-algebra (the empty set and the entire space), and
$\sigma $
-algebra (the empty set and the entire space), and
![]() $\mathcal {G}_j$
, for
$\mathcal {G}_j$
, for
![]() $j\ge 1$
, be the
$j\ge 1$
, be the
![]() $\sigma $
-algebra generated by
$\sigma $
-algebra generated by
 $$ \begin{align*} \{ W(t) \mid 0 \leq t \leq \tau_j\} \quad\mbox{where } \tau_j:=\sum_{k=1}^j T_k, \end{align*} $$
$$ \begin{align*} \{ W(t) \mid 0 \leq t \leq \tau_j\} \quad\mbox{where } \tau_j:=\sum_{k=1}^j T_k, \end{align*} $$
then
![]() $\tau _j$
is
$\tau _j$
is
![]() $\mathcal {G}_j$
-measurable, while
$\mathcal {G}_j$
-measurable, while
![]() $E (T_1 \mid \mathcal {G}_{0}) = E (W(T_1) ^2 \mid \mathcal {G}_{0})$
, and
$E (T_1 \mid \mathcal {G}_{0}) = E (W(T_1) ^2 \mid \mathcal {G}_{0})$
, and
By the last claim of Theorem 4.5 and properties of Brownian motion,
almost surely. (Indeed, letting
![]() $W_1$
be an independent copy of W, we have
$W_1$
be an independent copy of W, we have
![]() ${W(\tau _j) = W_1 (\tau _{j-1} + T_j) = W_1 (\tau _{j-1}) + W (T_j)}$
in distribution, so that
${W(\tau _j) = W_1 (\tau _{j-1} + T_j) = W_1 (\tau _{j-1}) + W (T_j)}$
in distribution, so that
![]() $W(\tau _j) - W(\tau _{j-1}) = W(T_j)$
in distribution.)
$W(\tau _j) - W(\tau _{j-1}) = W(T_j)$
in distribution.)
We need one last lemma. Recall that
![]() $\gamma \in (2/5,1/2)$
is fixed.
$\gamma \in (2/5,1/2)$
is fixed.
Lemma 4.6. (Strong law of large numbers for the sequence
 $T_j$
)
$T_j$
)
For
![]() $m_{*}$
-a.e.
$m_{*}$
-a.e.
![]() $a\in \Omega _{*}^\varphi $
, there exists
$a\in \Omega _{*}^\varphi $
, there exists
![]() $C(a)$
such that
$C(a)$
such that
 $$ \begin{align} \bigg| N - \sum_{j = 1}^{M(N)} T_j \bigg| \leq C(a) N^{2 \gamma} \quad \text{for all } N \geq 1. \end{align} $$
$$ \begin{align} \bigg| N - \sum_{j = 1}^{M(N)} T_j \bigg| \leq C(a) N^{2 \gamma} \quad \text{for all } N \geq 1. \end{align} $$
Proof. To start, apply Theorem 4.5 to the martingale difference sequence
![]() $Y_j$
from equation (4.27), with
$Y_j$
from equation (4.27), with
![]() $\mathcal {L}_j$
generated by
$\mathcal {L}_j$
generated by
![]() $\{y_\ell \}_{1\le \ell \le j}$
. Let
$\{y_\ell \}_{1\le \ell \le j}$
. Let
![]() $\tilde {Y}_j = W(\tau _j) - W(\tau _{j-1})$
, so that
$\tilde {Y}_j = W(\tau _j) - W(\tau _{j-1})$
, so that
![]() ${W (\tau _j) = \sum _{k=1}^j \tilde {Y}_{k}}$
and
${W (\tau _j) = \sum _{k=1}^j \tilde {Y}_{k}}$
and
![]() $\tilde {Y}_j = W(T_j)$
. By equation (4.32), we have, almost surely,
$\tilde {Y}_j = W(T_j)$
. By equation (4.32), we have, almost surely,
 $$ \begin{align*} N - \sum_{j = 1}^{M(N)} T_j &= \bigg [N - \sum_{j = 1}^M \tilde{Y}_j^2 \bigg] + \sum_{j = 1}^M [\tilde{Y_j}^2 - E(\tilde{Y}_j^2 \mid\mathcal{G}_{j-1})] \\ &\quad + \sum_{j = 1}^M [ E(T_j \mid \mathcal{G}_{j-1}) - T_j ] \quad\text{for all } N\ge 1. \end{align*} $$
$$ \begin{align*} N - \sum_{j = 1}^{M(N)} T_j &= \bigg [N - \sum_{j = 1}^M \tilde{Y}_j^2 \bigg] + \sum_{j = 1}^M [\tilde{Y_j}^2 - E(\tilde{Y}_j^2 \mid\mathcal{G}_{j-1})] \\ &\quad + \sum_{j = 1}^M [ E(T_j \mid \mathcal{G}_{j-1}) - T_j ] \quad\text{for all } N\ge 1. \end{align*} $$
Then, since
![]() $Y_j$
and
$Y_j$
and
![]() $\tilde {Y}_j$
have the same distribution, the bound in equation (4.28) in Lemma 4.4 gives
$\tilde {Y}_j$
have the same distribution, the bound in equation (4.28) in Lemma 4.4 gives
![]() $C(a)$
such that, for all
$C(a)$
such that, for all
![]() $N\ge 1$
, the first sum in the right-hand side above is not larger than
$N\ge 1$
, the first sum in the right-hand side above is not larger than
![]() $C(a) N^{2\gamma }$
.
$C(a) N^{2\gamma }$
.
For the second sum in the right-hand side above, we use equation (4.29). Since conditional expectations can be expressed in terms of distributions, equation (4.29) is also valid with
![]() $Y_j$
replaced by
$Y_j$
replaced by
![]() $\tilde {Y}_j$
. Thus, the second sum in the right-hand side is also bounded by
$\tilde {Y}_j$
. Thus, the second sum in the right-hand side is also bounded by
![]() $C(a) N^{2\gamma }$
for all
$C(a) N^{2\gamma }$
for all
![]() $N \geq 1$
.
$N \geq 1$
.
Finally, let
![]() $R_j = E (T_j \mid \mathcal {G}_{j-1}) - T_j$
. Then,
$R_j = E (T_j \mid \mathcal {G}_{j-1}) - T_j$
. Then,
![]() $\{R_j, \mathcal {G}_j\}$
is a martingale difference sequence by equation (4.32). As in the proof of equation (4.29), we can estimate
$\{R_j, \mathcal {G}_j\}$
is a martingale difference sequence by equation (4.32). As in the proof of equation (4.29), we can estimate
![]() $ E( R_j^2) \leq 4 E (W(T_j)^4)$
, and thus there exists
$ E( R_j^2) \leq 4 E (W(T_j)^4)$
, and thus there exists
![]() $C(a)$
such that, for all
$C(a)$
such that, for all
![]() $N\ge 1$
, we have
$N\ge 1$
, we have
![]() $\sum _{j=1}^{M(N)} R_j \leq C N^{21/30 + \iota } \leq C(a) N^{2 \gamma }$
almost surely.
$\sum _{j=1}^{M(N)} R_j \leq C N^{21/30 + \iota } \leq C(a) N^{2 \gamma }$
almost surely.
Proof of Theorem 1.1
Just like Schnellmann, we follow the proof of [Reference Philipp and StoutPS, Lemma 3.5.3], replacing their
![]() $1/2-\alpha /2+\gamma $
by
$1/2-\alpha /2+\gamma $
by
![]() $\gamma $
, and replacing Lemma 3.5.1 there by our Lemma 4.6. We then obtain that, almost surely,
$\gamma $
, and replacing Lemma 3.5.1 there by our Lemma 4.6. We then obtain that, almost surely,
 $$ \begin{align*} \bigg| \sum_{j = 1}^{M(N)} Y_j - W (N) \bigg| = O (N^\gamma). \end{align*} $$
$$ \begin{align*} \bigg| \sum_{j = 1}^{M(N)} Y_j - W (N) \bigg| = O (N^\gamma). \end{align*} $$
Then, using equations (4.30) and (4.4), we find
 $$ \begin{align} \bigg| \sum_{j=1}^{M(N)} y_j - Y_j \bigg| = \bigg| \sum_{j=1}^{M(N)} (u_{j+1} - u_j) \bigg| = |u_{M(N)+1} - u_1| \le C N^{\delta}. \end{align} $$
$$ \begin{align} \bigg| \sum_{j=1}^{M(N)} y_j - Y_j \bigg| = \bigg| \sum_{j=1}^{M(N)} (u_{j+1} - u_j) \bigg| = |u_{M(N)+1} - u_1| \le C N^{\delta}. \end{align} $$
Since
![]() $\delta <2/5$
, and recalling equation (4.5), this establishes Theorem 1.1.
$\delta <2/5$
, and recalling equation (4.5), this establishes Theorem 1.1.
Acknowledgements
M.A. and T.P. thank the Institute for Theoretical Studies, ETH Zürich, for its hospitality in 2022. V.B.’s research is supported by the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation programme (grant agreement No 787304). V.B. is grateful to the K. and A. Wallenberg Foundation, for an invitation to Lund University in 2021, and to J. Sedro for the reference [Reference MoreiraMo]. V.B. and T.P. thank Q. Berger and F. Pène for useful conversations and acknowledge the hospitality of CIRM (Luminy) and the Lorentz Center (Leiden), where part of this work was carried out in 2022. We are grateful to the referee for a very careful reading which allowed us to remove several small mistakes.