Published online by Cambridge University Press: 08 December 2021
We prove a general principle satisfied by weakly precompact sets of Lipschitz-free spaces. By this principle, certain infinite dimensional phenomena in Lipschitz-free spaces over general metric spaces may be reduced to the same phenomena in free spaces over their compact subsets. As easy consequences we derive several new and some known results. The main new results are: 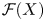 $\mathcal {F}(X)$ is weakly sequentially complete for every superreflexive Banach space
$\mathcal {F}(X)$ is weakly sequentially complete for every superreflexive Banach space 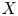 $X$, and
$X$, and 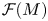 $\mathcal {F}(M)$ has the Schur property and the approximation property for every scattered complete metric space
$\mathcal {F}(M)$ has the Schur property and the approximation property for every scattered complete metric space 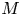 $M$.
$M$.
Please note a has been issued for this article.