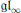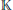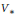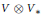Let $V$ be a vector space over a field $\mathbb{K}$ of characteristic zero and ${{V}_{*}}$ be a space of linear functionals on $V$ which separate the points of $V$. We consider $V\,\otimes \,{{V}_{*}}$ as a Lie algebra of finite rank operators on $V$, and set 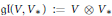$\mathfrak{g}\mathfrak{l}(V,\,{{V}_{*}})\,:=\,V\,\otimes \,{{V}_{*}}$. We define a Cartan subalgebra of 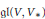$\mathfrak{g}\mathfrak{l}(V,{{V}_{*}})$ as the centralizer of a maximal subalgebra every element of which is semisimple, and then give the following description of all Cartan subalgebras of 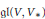$\mathfrak{g}\mathfrak{l}(V,{{V}_{*}})$ under the assumption that 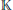$\mathbb{K}$ is algebraically closed. A subalgebra of 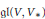$\mathfrak{g}\mathfrak{l}(V,{{V}_{*}})$ is a Cartan subalgebra if and only if it equals ${{\oplus }_{j}}({{V}_{j}}\,\otimes {{({{V}_{j}})}_{*}})\,\oplus \,({{V}^{0}}\,\otimes \,V_{*}^{0})$ for some one-dimensional subspaces 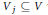${{V}_{j}}\subseteq V$ and 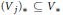${{\text{(}{{V}_{j}}\text{)}}_{*}}\subseteq {{V}_{*}}$ with 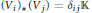${{({{V}_{i}})}_{*}}({{V}_{j}})\,=\,{{\delta }_{ij}}\mathbb{K}$ and such that the spaces 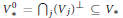$V_{*}^{0}=\bigcap{_{j}}{{({{V}_{j}})}^{\bot }}\subseteq {{V}_{*}}$ and ${{V}^{0}}=\bigcap{_{j}}{{\left( {{({{V}_{j}})}_{*}} \right)}^{\bot }}\subseteq V$ satisfy 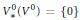$V_{*}^{0}({{V}^{0}})\,=\,\{0\}$. We then discuss explicit constructions of subspaces ${{V}_{j}}$ and ${{({{V}_{j}})}_{*}}$ as above. Our second main result claims that a Cartan subalgebra of 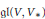$\mathfrak{g}\mathfrak{l}(V,{{V}_{*}})$ can be described alternatively as a locally nilpotent self-normalizing subalgebra whose adjoint representation is locally finite, or as a subalgebra 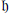$\mathfrak{h}$ which coincides with the maximal locally nilpotent 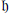$\mathfrak{h}$-submodule of 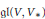$\mathfrak{g}\mathfrak{l}(V,{{V}_{*}})$, and such that the adjoint representation of 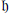$\mathfrak{h}$ is locally finite.