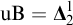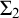1 Introduction
A set of reals, meaning a subset of the Baire space
![]() $\omega ^\omega $
, is called universally Baire if its preimages under all continuous functions from all topological spaces have the Baire property (Feng et al. [Reference Feng, Magidor and Woodin2]). We denote the pointclass of all universally Baire sets of reals by uB. The universally Baire sets of reals include the
$\omega ^\omega $
, is called universally Baire if its preimages under all continuous functions from all topological spaces have the Baire property (Feng et al. [Reference Feng, Magidor and Woodin2]). We denote the pointclass of all universally Baire sets of reals by uB. The universally Baire sets of reals include the
![]() ${\boldsymbol {\Sigma }}^1_1$
(analytic) and
${\boldsymbol {\Sigma }}^1_1$
(analytic) and
![]() ${\boldsymbol {\Pi }}^1_1$
(coanalytic) sets of reals, but not necessarily the
${\boldsymbol {\Pi }}^1_1$
(coanalytic) sets of reals, but not necessarily the
![]() ${\Delta ^1_2}$
sets of reals. Every universally Baire set of reals is Lebesgue measurable and has the Baire property. If there is a Woodin cardinal then every universally Baire set of reals has the perfect set property,Footnote
1
whereas if
${\Delta ^1_2}$
sets of reals. Every universally Baire set of reals is Lebesgue measurable and has the Baire property. If there is a Woodin cardinal then every universally Baire set of reals has the perfect set property,Footnote
1
whereas if
![]() $\omega _1^L = \omega _1$
then there is a set of reals that is
$\omega _1^L = \omega _1$
then there is a set of reals that is
![]() $\Pi ^1_1$
, hence universally Baire, but fails to have the perfect set property (Gödel; see Kanamori [Reference Kanamori8, Theorem 13.12]).
$\Pi ^1_1$
, hence universally Baire, but fails to have the perfect set property (Gödel; see Kanamori [Reference Kanamori8, Theorem 13.12]).
In this article we will describe the exact consistency strength of the theory ZFC+ “every universally Baire set of reals has the perfect set property” in terms of a large cardinal that we call virtually Shelah for supercompactness (VSS).Footnote
2
It is likely that this theory was already known to be much weaker than a Woodin cardinal, since it is not difficult to force it over L if
![]() $0^\sharp $
exists, but we are not aware of reference for this.
$0^\sharp $
exists, but we are not aware of reference for this.
First we briefly review the notion of a virtual large cardinal property. Many large cardinal properties are defined in terms of elementary embeddings
![]() $j : M \to N$
where M and N are structures. (If M and N are sets with no structure given, we consider them as structures with the
$j : M \to N$
where M and N are structures. (If M and N are sets with no structure given, we consider them as structures with the
![]() $\in $
relation). Such a definition can be weakened to a “virtual” large cardinal property by only requiring j to exist in some generic extension of V. Examples of virtual large cardinal properties are remarkability, which is a virtual form of Magidor’s characterization of supercompactness (Schindler [Reference Schindler12, Lemma 1.6]), and the generic Vopěnka principle, which is a virtual form of Vopěnka’s principle (Bagaria et al. [Reference Bagaria, Gitman and Schindler1]).
$\in $
relation). Such a definition can be weakened to a “virtual” large cardinal property by only requiring j to exist in some generic extension of V. Examples of virtual large cardinal properties are remarkability, which is a virtual form of Magidor’s characterization of supercompactness (Schindler [Reference Schindler12, Lemma 1.6]), and the generic Vopěnka principle, which is a virtual form of Vopěnka’s principle (Bagaria et al. [Reference Bagaria, Gitman and Schindler1]).
For a thorough introduction to virtual large cardinal properties, see Gitman and Schindler [Reference Gitman and Schindler5]. For an application of virtual large cardinals to descriptive set theory involving
![]() $\aleph _1$
-Suslin sets instead of universally Baire sets, see Wilson [Reference Wilson18].
$\aleph _1$
-Suslin sets instead of universally Baire sets, see Wilson [Reference Wilson18].
Note that for set-sized structures M and N in V, if some generic extension of V contains an elementary embedding of M into N, then by the absoluteness of elementary embeddability of countable structures (see Bagaria et al. [Reference Bagaria, Gitman and Schindler1, Lemma 2.6]) every generic extension of V by the poset
![]() $\operatorname {\mathrm {Col}}(\omega ,M)$
contains such an elementary embedding. The converse implication holds also, of course, and we may abbreviate these equivalent conditions by the phrase “there is a generic elementary embedding of M into N.”
$\operatorname {\mathrm {Col}}(\omega ,M)$
contains such an elementary embedding. The converse implication holds also, of course, and we may abbreviate these equivalent conditions by the phrase “there is a generic elementary embedding of M into N.”
Recall that a cardinal
![]() $\kappa $
is called Shelah if for every function
$\kappa $
is called Shelah if for every function
![]() $f : \kappa \to \kappa $
there is a transitive class M and an elementary embedding
$f : \kappa \to \kappa $
there is a transitive class M and an elementary embedding
![]() $j:V \to M$
with
$j:V \to M$
with
![]() $\operatorname {\mathrm {crit}}(j) = \kappa $
and
$\operatorname {\mathrm {crit}}(j) = \kappa $
and
![]() $V_{j(f)(\kappa )} \subset M$
. Note that for any ordinal
$V_{j(f)(\kappa )} \subset M$
. Note that for any ordinal
![]() $\lambda \ge \kappa +1$
, the restriction
$\lambda \ge \kappa +1$
, the restriction
![]() $j \restriction V_\lambda $
is sufficient to derive an extender E whose ultrapower embedding
$j \restriction V_\lambda $
is sufficient to derive an extender E whose ultrapower embedding
![]() $j_E$
witnesses the Shelah property of
$j_E$
witnesses the Shelah property of
![]() $\kappa $
as well as j does. Making the convenient choice
$\kappa $
as well as j does. Making the convenient choice
![]() $\lambda = \max \{j(f)(\kappa ),\kappa +1\}$
, we obtain the following definition as a kind of virtualization.Footnote
3
$\lambda = \max \{j(f)(\kappa ),\kappa +1\}$
, we obtain the following definition as a kind of virtualization.Footnote
3
Definition 1.1. A cardinal
![]() $\kappa $
is virtually Shelah for supercompactness (VSS) if for every function
$\kappa $
is virtually Shelah for supercompactness (VSS) if for every function
![]() $f : \kappa \to \kappa $
there is an ordinal
$f : \kappa \to \kappa $
there is an ordinal
![]() $\lambda> \kappa $
, a transitive set M with
$\lambda> \kappa $
, a transitive set M with
![]() $V_\lambda \subset M$
, and a generic elementary embedding
$V_\lambda \subset M$
, and a generic elementary embedding
![]() $j : V_\lambda \to M$
with
$j : V_\lambda \to M$
with
![]() $\operatorname {\mathrm {crit}}(j) = \kappa $
and
$\operatorname {\mathrm {crit}}(j) = \kappa $
and
![]() $j(f)(\kappa ) \le \lambda $
.
$j(f)(\kappa ) \le \lambda $
.
The VSS property follows immediately from the Shelah property but is much weaker: Proposition 3.1 will show that if
![]() $0^\sharp $
exists then every Silver indiscernible is VSS in L, a result that is typical of virtual large cardinal properties.
$0^\sharp $
exists then every Silver indiscernible is VSS in L, a result that is typical of virtual large cardinal properties.
Our main result is stated below. It relates models of ZFC with VSS cardinals to models of ZFC in which the universally Baire sets are few in number and have nice properties. It also includes a combinatorial statement about order types of countable sets of ordinals.
Theorem 1.2. The following statements are equiconsistent modulo ZFC.
-
1. There is a VSS cardinal.
-
2.
 $\left |\operatorname {\mathrm {uB}}\right | = \omega _1$
.
$\left |\operatorname {\mathrm {uB}}\right | = \omega _1$
. -
3. Every set of reals in
 $L(\mathbb {R},\operatorname {\mathrm {uB}})$
is Lebesgue measurable.
$L(\mathbb {R},\operatorname {\mathrm {uB}})$
is Lebesgue measurable. -
4. Every set of reals in
 $L(\mathbb {R},\operatorname {\mathrm {uB}})$
has the perfect set property.
$L(\mathbb {R},\operatorname {\mathrm {uB}})$
has the perfect set property. -
5. Every universally Baire set of reals has the perfect set property.
-
6. For every function
 $f: \omega _1 \to \omega _1$
there is an ordinal
$f: \omega _1 \to \omega _1$
there is an ordinal
 $\lambda> \omega _1$
such that for a stationary set of
$\lambda> \omega _1$
such that for a stationary set of
 $\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
we have
$\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
we have
 $\sigma \cap \omega _1 \in \omega _1$
and
$\sigma \cap \omega _1 \in \omega _1$
and
 $\operatorname {\mathrm {o.\!t.}}(\sigma ) \ge f(\sigma \cap \omega _1)$
.Footnote
4
$\operatorname {\mathrm {o.\!t.}}(\sigma ) \ge f(\sigma \cap \omega _1)$
.Footnote
4
We will show that if statement 1 holds then statements 2–4 hold after forcing with the Levy collapse poset to make a VSS cardinal equal to
![]() $\omega _1$
. Clearly statement 4 implies statement 5, and we will show that statements 2 and 3 also imply statement 5. We will show that statement 5 implies statement 6. Finally, we will show that if statement 6 holds, then statement 1 holds in L as witnessed by
$\omega _1$
. Clearly statement 4 implies statement 5, and we will show that statements 2 and 3 also imply statement 5. We will show that statement 5 implies statement 6. Finally, we will show that if statement 6 holds, then statement 1 holds in L as witnessed by
![]() $\omega _1^V$
.
$\omega _1^V$
.
We will also prove an equiconsistency result at a slightly higher level of consistency strength, namely that of a
![]() $\Sigma _2$
-reflecting VSS cardinal. A cardinal
$\Sigma _2$
-reflecting VSS cardinal. A cardinal
![]() $\kappa $
is called
$\kappa $
is called
![]() $\Sigma _n$
-reflecting if it is inaccessible and
$\Sigma _n$
-reflecting if it is inaccessible and
![]() $V_\kappa \prec _{\Sigma _n} V$
. This definition is particularly natural in the case
$V_\kappa \prec _{\Sigma _n} V$
. This definition is particularly natural in the case
![]() $n = 2$
because the
$n = 2$
because the
![]() $\Sigma _2$
statements about a parameter a are the statements that can be expressed in the form “there is an ordinal
$\Sigma _2$
statements about a parameter a are the statements that can be expressed in the form “there is an ordinal
![]() $\lambda $
such that
$\lambda $
such that
![]() $V_\lambda \models \varphi [a]$
” where
$V_\lambda \models \varphi [a]$
” where
![]() $\varphi $
is a formula in the language of set theory. Because the existence of a VSS cardinal above any given cardinal
$\varphi $
is a formula in the language of set theory. Because the existence of a VSS cardinal above any given cardinal
![]() $\alpha $
is a
$\alpha $
is a
![]() $\Sigma _2$
statement about
$\Sigma _2$
statement about
![]() $\alpha $
, if
$\alpha $
, if
![]() $\kappa $
is a
$\kappa $
is a
![]() $\Sigma _2$
-reflecting VSS cardinal then
$\Sigma _2$
-reflecting VSS cardinal then
![]() $V_\kappa $
satisfies ZFC+ “there is a proper class of VSS cardinals.”
$V_\kappa $
satisfies ZFC+ “there is a proper class of VSS cardinals.”
The existence of a
![]() $\Sigma _2$
-reflecting cardinal is equiconsistent modulo ZFC with the statement
$\Sigma _2$
-reflecting cardinal is equiconsistent modulo ZFC with the statement
![]() ${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
by Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.3], who showed that if
${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
by Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.3], who showed that if
![]() $\kappa $
is
$\kappa $
is
![]() $\Sigma _2$
-reflecting then
$\Sigma _2$
-reflecting then
![]() ${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
holds after the Levy collapse forcing to make
${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
holds after the Levy collapse forcing to make
![]() $\kappa $
equal to
$\kappa $
equal to
![]() $\omega _1$
, and conversely that if
$\omega _1$
, and conversely that if
![]() ${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
then
${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
then
![]() $\omega _1^V$
is
$\omega _1^V$
is
![]() $\Sigma _2$
-reflecting in L.
$\Sigma _2$
-reflecting in L.
The reverse inclusion
![]() $\operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
is consistent relative to ZFC by Larson and Shelah [Reference Larson and Shelah9], who showed that if
$\operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
is consistent relative to ZFC by Larson and Shelah [Reference Larson and Shelah9], who showed that if
![]() $V = L[x]$
for some real x then there is a proper forcing extension in which every universally measurable set of reals—and hence every universally Baire set of reals—is
$V = L[x]$
for some real x then there is a proper forcing extension in which every universally measurable set of reals—and hence every universally Baire set of reals—is
![]() ${\boldsymbol {\Delta }}^1_2$
. Of course this proper forcing is not the Levy collapse; as we will show, forcing both inclusions to hold simultaneously requires more large cardinals in the ground model.
${\boldsymbol {\Delta }}^1_2$
. Of course this proper forcing is not the Levy collapse; as we will show, forcing both inclusions to hold simultaneously requires more large cardinals in the ground model.
Combining the argument of Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.3] with parts of the proof of Theorem 1.2, we will show:
Theorem 1.3. The following statements are equiconsistent modulo ZFC.
-
1. There is a
 $\Sigma _2$
-reflecting VSS cardinal.
$\Sigma _2$
-reflecting VSS cardinal. -
2.
 $\operatorname {\mathrm {uB}} = {\boldsymbol {\Delta }}^1_2$
.
$\operatorname {\mathrm {uB}} = {\boldsymbol {\Delta }}^1_2$
.
The remaining sections of this paper are outlined as follows. In Section 2 we will prove some consequences of Definition 1.1 that will be needed for our main results, including a reformulation of the definition in which
![]() $\kappa $
is
$\kappa $
is
![]() $j(\operatorname {\mathrm {crit}}(j))$
instead of
$j(\operatorname {\mathrm {crit}}(j))$
instead of
![]() $\operatorname {\mathrm {crit}}(j)$
, justifying the name “virtually Shelah for supercompactness” (Proposition 2.5). In Section 3 we will prove some relations between VSS cardinals and other large cardinals that will not be needed for our main results. In Section 4 we will review some properties of universally Baire sets and establish some equivalent conditions for a universally Baire set to be thin, meaning to contain no perfect subset. In Section 5 we will prove Theorem 1.2. In Section 6 we will prove Theorem 1.3.
$\operatorname {\mathrm {crit}}(j)$
, justifying the name “virtually Shelah for supercompactness” (Proposition 2.5). In Section 3 we will prove some relations between VSS cardinals and other large cardinals that will not be needed for our main results. In Section 4 we will review some properties of universally Baire sets and establish some equivalent conditions for a universally Baire set to be thin, meaning to contain no perfect subset. In Section 5 we will prove Theorem 1.2. In Section 6 we will prove Theorem 1.3.
2 Consequences of the VSS property
Recall that a cardinal
![]() $\kappa $
is called ineffable if for every sequence of sets
$\kappa $
is called ineffable if for every sequence of sets
![]() $\langle A_\alpha : \alpha < \kappa \rangle $
such that
$\langle A_\alpha : \alpha < \kappa \rangle $
such that
![]() $A_\alpha \subset \alpha $
for all
$A_\alpha \subset \alpha $
for all
![]() $\alpha < \kappa $
, there is a set
$\alpha < \kappa $
, there is a set
![]() $A \subset \kappa $
such that
$A \subset \kappa $
such that
![]() $\{\alpha < \kappa : A \cap \alpha = A_\alpha \}$
is stationary. The following result is typical of virtual large cardinals (see Schindler [Reference Schindler12, Lemma 1.4]).
$\{\alpha < \kappa : A \cap \alpha = A_\alpha \}$
is stationary. The following result is typical of virtual large cardinals (see Schindler [Reference Schindler12, Lemma 1.4]).
Proposition 2.1. Every VSS cardinal is ineffable.
Proof Let
![]() $\kappa $
be a VSS cardinal. Then there is an ordinal
$\kappa $
be a VSS cardinal. Then there is an ordinal
![]() $\lambda> \kappa $
, a transitive set M such that
$\lambda> \kappa $
, a transitive set M such that
![]() $V_\lambda \subset M$
, and a generic elementary embedding
$V_\lambda \subset M$
, and a generic elementary embedding
![]() $j : V_\lambda \to M$
with
$j : V_\lambda \to M$
with
![]() $\operatorname {\mathrm {crit}}(j) = \kappa $
. (Here we will not need
$\operatorname {\mathrm {crit}}(j) = \kappa $
. (Here we will not need
![]() $j(f)(\kappa ) \le \lambda $
for any particular function f.)
$j(f)(\kappa ) \le \lambda $
for any particular function f.)
Let
![]() $\vec {A}$
be a
$\vec {A}$
be a
![]() $\kappa $
-sequence of sets such that
$\kappa $
-sequence of sets such that
![]() $\vec {A}(\alpha ) \subset \alpha $
for every ordinal
$\vec {A}(\alpha ) \subset \alpha $
for every ordinal
![]() $\alpha < \kappa $
. Then we may define a subset
$\alpha < \kappa $
. Then we may define a subset
![]() $A \subset \kappa $
by
$A \subset \kappa $
by
![]() $A = j(\vec {A})(\kappa )$
. We will show that the set
$A = j(\vec {A})(\kappa )$
. We will show that the set
is stationary. Letting C be a club set in
![]() $\kappa $
we have
$\kappa $
we have
![]() $\kappa \in j(C)$
, and because
$\kappa \in j(C)$
, and because
we have
![]() $\kappa \in j(S)$
also, so it follows that
$\kappa \in j(S)$
also, so it follows that
and by the elementarity of j we have
![]() $C \cap S \ne \emptyset $
.
$C \cap S \ne \emptyset $
.
A better lower bound for the consistency strength of VSS cardinals will be given in Section 3 along with an upper bound. For our main results we will only need the fact that every VSS cardinal is an inaccessible limit of inaccessible cardinals, which is a consequence of Proposition 2.1.
The following lemma shows (among other things) that the domain of a generic elementary embedding witnessing the VSS property may be taken to be an inaccessible rank initial segment of V, which implies that the domain and codomain both satisfy ZFC.
Lemma 2.2. Let
![]() $\kappa $
be a VSS cardinal and let
$\kappa $
be a VSS cardinal and let
![]() $f : \kappa \to \kappa $
. Then there is an inaccessible cardinal
$f : \kappa \to \kappa $
. Then there is an inaccessible cardinal
![]() $\lambda> \kappa $
, a transitive model M of ZFC with
$\lambda> \kappa $
, a transitive model M of ZFC with
![]() $V_\lambda \subset M$
, and a generic elementary embedding
$V_\lambda \subset M$
, and a generic elementary embedding
![]() $j : V_\lambda \to M$
with
$j : V_\lambda \to M$
with
![]() $\operatorname {\mathrm {crit}}(j) = \kappa $
and
$\operatorname {\mathrm {crit}}(j) = \kappa $
and
![]() $j(f)(\kappa ) < \lambda < j(\kappa )$
.
$j(f)(\kappa ) < \lambda < j(\kappa )$
.
Proof Because
![]() $\kappa $
is a limit of inaccessible cardinals we may define a function
$\kappa $
is a limit of inaccessible cardinals we may define a function
![]() $g : \kappa \to \kappa $
such that
$g : \kappa \to \kappa $
such that
![]() $g(\alpha )$
is the least inaccessible cardinal greater than
$g(\alpha )$
is the least inaccessible cardinal greater than
![]() $\max \{f(\alpha ), \alpha \}$
for all
$\max \{f(\alpha ), \alpha \}$
for all
![]() $\alpha < \kappa $
. Because
$\alpha < \kappa $
. Because
![]() $\kappa $
is VSS with respect to the function
$\kappa $
is VSS with respect to the function
![]() $g+1$
defined by
$g+1$
defined by
![]() $\alpha \mapsto g(\alpha )+1$
, there is an ordinal
$\alpha \mapsto g(\alpha )+1$
, there is an ordinal
![]() $\beta> \kappa $
, a transitive set N with
$\beta> \kappa $
, a transitive set N with
![]() $V_\beta \subset N$
, and a generic elementary embedding
$V_\beta \subset N$
, and a generic elementary embedding
By the definition of g from f and the elementarity of j it follows that
![]() $j(g)(\kappa )$
is the least inaccessible cardinal in N greater than
$j(g)(\kappa )$
is the least inaccessible cardinal in N greater than
![]() $\max \{j(f)(\kappa ), \kappa \}$
. Because
$\max \{j(f)(\kappa ), \kappa \}$
. Because
![]() $j(g)$
is a function from
$j(g)$
is a function from
![]() $j(\kappa )$
to
$j(\kappa )$
to
![]() $j(\kappa )$
we have
$j(\kappa )$
we have
![]() $j(g)(\kappa ) < j(\kappa )$
. Letting
$j(g)(\kappa ) < j(\kappa )$
. Letting
![]() $\lambda = j(g)(\kappa )$
we therefore have
$\lambda = j(g)(\kappa )$
we therefore have
![]() $\lambda> \kappa $
and
$\lambda> \kappa $
and
Because
![]() $\lambda < \beta $
and
$\lambda < \beta $
and
![]() $V_\beta \subset N$
, the inaccessibility of
$V_\beta \subset N$
, the inaccessibility of
![]() $\lambda $
is absolute from N to V. Define
$\lambda $
is absolute from N to V. Define
![]() $j_1 = j \restriction V_\lambda $
and M =
$j_1 = j \restriction V_\lambda $
and M =
![]() $j(V_\lambda ) = V_{j(\lambda )}^N$
. Then
$j(V_\lambda ) = V_{j(\lambda )}^N$
. Then
![]() $j_1 : V_\lambda \to M$
is a generic elementary embedding with
$j_1 : V_\lambda \to M$
is a generic elementary embedding with
![]() $\operatorname {\mathrm {crit}}(j_1) = \kappa $
and
$\operatorname {\mathrm {crit}}(j_1) = \kappa $
and
![]() $j_1(f)(\kappa ) < \lambda < j_1(\kappa )$
as desired. Because
$j_1(f)(\kappa ) < \lambda < j_1(\kappa )$
as desired. Because
![]() $V_\lambda $
satisfies ZFC it follows by the elementarity of
$V_\lambda $
satisfies ZFC it follows by the elementarity of
![]() $j_1$
that M satisfies ZFC.
$j_1$
that M satisfies ZFC.
It follows from Lemma 2.2 that every VSS cardinal has an inaccessible cardinal above it. Because inaccessibility is preserved by small forcing, combining this fact with the proof of Theorem 1.2 (as outlined following the statement of the theorem) yields the following curious consequence.
Proposition 2.3. The following statements are equiconsistent modulo ZFC.
-
1. Every universally Baire set of reals has the perfect set property.
-
2. Every universally Baire set of reals has the perfect set property and there is an inaccessible cardinal.
Note that the natural attempt to show that statement 2 has strictly higher consistency strength than statement 1 fails because for an inaccessible cardinal
![]() $\lambda $
, the pointclass
$\lambda $
, the pointclass
![]() $\operatorname {\mathrm {uB}}^{V_\lambda }$
might not be equal to
$\operatorname {\mathrm {uB}}^{V_\lambda }$
might not be equal to
![]() $\operatorname {\mathrm {uB}}$
. For example, if
$\operatorname {\mathrm {uB}}$
. For example, if
![]() $V = L[U]$
where U is a normal measure on
$V = L[U]$
where U is a normal measure on
![]() $\lambda $
, then it follows from Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.4] that
$\lambda $
, then it follows from Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.4] that
![]() $\boldsymbol {\Sigma }^1_2 \subset \operatorname {\mathrm {uB}}^{V_\lambda }$
and
$\boldsymbol {\Sigma }^1_2 \subset \operatorname {\mathrm {uB}}^{V_\lambda }$
and
![]() $\boldsymbol {\Sigma }^1_2 \not \subset \operatorname {\mathrm {uB}}$
.
$\boldsymbol {\Sigma }^1_2 \not \subset \operatorname {\mathrm {uB}}$
.
Like other known virtual large cardinal properties, the virtual Shelah-for-supercompactness property is downward absolute to L:
Proposition 2.4. Every VSS cardinal is VSS in L.
Proof Let
![]() $\kappa $
be a VSS cardinal and let
$\kappa $
be a VSS cardinal and let
![]() $f : \kappa \to \kappa $
be a function in L. Then by Lemma 2.2 there is an inaccessible cardinal
$f : \kappa \to \kappa $
be a function in L. Then by Lemma 2.2 there is an inaccessible cardinal
![]() $\lambda> \kappa $
, a transitive model M of ZFC with
$\lambda> \kappa $
, a transitive model M of ZFC with
![]() $V_\lambda \subset M$
, and a generic elementary embedding
$V_\lambda \subset M$
, and a generic elementary embedding
![]() $j : V_\lambda \to M$
with
$j : V_\lambda \to M$
with
![]() $\operatorname {\mathrm {crit}}(j) = \kappa $
and
$\operatorname {\mathrm {crit}}(j) = \kappa $
and
![]() $j(f)(\kappa ) < \lambda < j(\kappa )$
. Note that
$j(f)(\kappa ) < \lambda < j(\kappa )$
. Note that
![]() $L^{V_\lambda } = L_\lambda $
and
$L^{V_\lambda } = L_\lambda $
and
![]() $L^M = L_\theta $
where
$L^M = L_\theta $
where
![]() $\theta = \mathrm {Ord}^M$
. Moreover, because
$\theta = \mathrm {Ord}^M$
. Moreover, because
![]() $\lambda $
is inaccessible we have
$\lambda $
is inaccessible we have
![]() $L_\lambda = V_\lambda ^L$
. For the elementary embedding
$L_\lambda = V_\lambda ^L$
. For the elementary embedding
![]() $j_1 = j \restriction V_\lambda ^L$
we have
$j_1 = j \restriction V_\lambda ^L$
we have
Let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,V_\lambda ^L)$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
$G \subset \operatorname {\mathrm {Col}}(\omega ,V_\lambda ^L)$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
![]() $j_2 \in L[G]$
such that
$j_2 \in L[G]$
such that
We have
![]() $V_\lambda ^L = L_\lambda \subset L_\theta $
and
$V_\lambda ^L = L_\lambda \subset L_\theta $
and
![]() $j_2(f)(\kappa ) = j(f)(\kappa ) < \lambda $
, so
$j_2(f)(\kappa ) = j(f)(\kappa ) < \lambda $
, so
![]() $j_2$
witnesses the VSS property for
$j_2$
witnesses the VSS property for
![]() $\kappa $
in L with respect to f.
$\kappa $
in L with respect to f.
In Proposition 2.5, we characterize VSS cardinals by a property in which
![]() $\kappa $
is the image of the critical point, as in the characterizations of supercompact and virtually supercompact (i.e., remarkable) cardinals by Magidor [Reference Magidor10, Theorem 1] and Schindler [Reference Schindler12, Lemma 1.6] respectively. Note that if statement 2 of Proposition 2.5 is unvirtualized by requiring j to exist in V, the result is equivalent to the property called “Shelah for supercompactness” by Perlmutter [Reference Perlmutter11, Definition 2.7]. This justifies the name “virtually Shelah for supercompactness.”
$\kappa $
is the image of the critical point, as in the characterizations of supercompact and virtually supercompact (i.e., remarkable) cardinals by Magidor [Reference Magidor10, Theorem 1] and Schindler [Reference Schindler12, Lemma 1.6] respectively. Note that if statement 2 of Proposition 2.5 is unvirtualized by requiring j to exist in V, the result is equivalent to the property called “Shelah for supercompactness” by Perlmutter [Reference Perlmutter11, Definition 2.7]. This justifies the name “virtually Shelah for supercompactness.”
Proposition 2.5. For every cardinal
![]() $\kappa $
the following statements are equivalent:
$\kappa $
the following statements are equivalent:
-
1.
 $\kappa $
is VSS.
$\kappa $
is VSS. -
2. For every function
 $f : \kappa \to \kappa $
there are ordinals
$f : \kappa \to \kappa $
there are ordinals
 $\bar {\lambda }$
and
$\bar {\lambda }$
and
 $\lambda $
and a generic elementary embedding
$\lambda $
and a generic elementary embedding
 $j :V_{\bar {\lambda }} \to V_\lambda $
with the properties
$j :V_{\bar {\lambda }} \to V_\lambda $
with the properties
 $j(\operatorname {\mathrm {crit}}(j)) = \kappa $
and
$j(\operatorname {\mathrm {crit}}(j)) = \kappa $
and
 $f \in \operatorname {\mathrm {ran}}(j)$
and
$f \in \operatorname {\mathrm {ran}}(j)$
and
 $f(\operatorname {\mathrm {crit}}(j)) \le \bar {\lambda }$
.Footnote
5
$f(\operatorname {\mathrm {crit}}(j)) \le \bar {\lambda }$
.Footnote
5
Proof (1)
![]() $\!\implies\! $
(2): Assume that
$\!\implies\! $
(2): Assume that
![]() $\kappa $
is VSS and let
$\kappa $
is VSS and let
![]() $f: \kappa \to \kappa $
. By Lemma 2.2 there is an inaccessible cardinal
$f: \kappa \to \kappa $
. By Lemma 2.2 there is an inaccessible cardinal
![]() $\lambda> \kappa $
, a transitive model M of ZFC with
$\lambda> \kappa $
, a transitive model M of ZFC with
![]() $V_\lambda \subset M$
, and a generic elementary embedding
$V_\lambda \subset M$
, and a generic elementary embedding
Define
![]() $\beta = \max \{j(f)(\kappa ), \kappa +1\}$
, so
$\beta = \max \{j(f)(\kappa ), \kappa +1\}$
, so
![]() $\kappa < \beta < \lambda $
and
$\kappa < \beta < \lambda $
and
![]() $V_\beta ^M = V_\beta $
. Let
$V_\beta ^M = V_\beta $
. Let
![]() $j_1 = j \restriction V_\beta $
and note that
$j_1 = j \restriction V_\beta $
and note that
Let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,V_\beta )$
be a V-generic filter. Because
$G \subset \operatorname {\mathrm {Col}}(\omega ,V_\beta )$
be a V-generic filter. Because
![]() $M[G]$
is wellfounded, by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
$M[G]$
is wellfounded, by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
![]() $j_2 \in M[G]$
such that
$j_2 \in M[G]$
such that
Then we have
because
![]() $j(f)(\kappa ) \le \beta $
. By the elementarity of j and the definability of forcing there is an ordinal
$j(f)(\kappa ) \le \beta $
. By the elementarity of j and the definability of forcing there is an ordinal
![]() $\bar {\beta } < \lambda $
such that, letting
$\bar {\beta } < \lambda $
such that, letting
![]() $g \subset \operatorname {\mathrm {Col}}(\omega ,V_{\bar {\beta }})$
be a V-generic filter, there is an elementary embedding
$g \subset \operatorname {\mathrm {Col}}(\omega ,V_{\bar {\beta }})$
be a V-generic filter, there is an elementary embedding
![]() $j_3 \in V_\lambda [g]$
with
$j_3 \in V_\lambda [g]$
with
Therefore statement 2 holds for f.
(2)
![]() $\!\implies\! $
(1): Assume that statement 2 holds and let
$\!\implies\! $
(1): Assume that statement 2 holds and let
![]() $f: \kappa \to \kappa $
. Note that statement 2 implies the ineffability of
$f: \kappa \to \kappa $
. Note that statement 2 implies the ineffability of
![]() $\kappa $
by an argument similar to Proposition 2.1. It follows that
$\kappa $
by an argument similar to Proposition 2.1. It follows that
![]() $\kappa $
is a limit of inaccessible cardinals, so by increasing the values of f we may assume that for all
$\kappa $
is a limit of inaccessible cardinals, so by increasing the values of f we may assume that for all
![]() $\alpha < \kappa $
,
$\alpha < \kappa $
,
![]() $f(\alpha )$
is an inaccessible cardinal greater than
$f(\alpha )$
is an inaccessible cardinal greater than
![]() $\alpha $
and we may furthermore define
$\alpha $
and we may furthermore define
![]() $f^+(\alpha )$
to be the least inaccessible cardinal greater than
$f^+(\alpha )$
to be the least inaccessible cardinal greater than
![]() $f(\alpha )$
. Applying statement 2 to the function
$f(\alpha )$
. Applying statement 2 to the function
![]() $f^+: \kappa \to \kappa $
yields ordinals
$f^+: \kappa \to \kappa $
yields ordinals
![]() $\bar {\lambda }$
and
$\bar {\lambda }$
and
![]() $\lambda $
and a generic elementary embedding
$\lambda $
and a generic elementary embedding
where
![]() $\bar {\kappa } = \operatorname {\mathrm {crit}}(j)$
. By restricting j if necessary we may assume that
$\bar {\kappa } = \operatorname {\mathrm {crit}}(j)$
. By restricting j if necessary we may assume that
![]() $\bar {\lambda }$
is equal to
$\bar {\lambda }$
is equal to
![]() $f^+(\bar {\kappa })$
and is therefore an inaccessible cardinal less than
$f^+(\bar {\kappa })$
and is therefore an inaccessible cardinal less than
![]() $\kappa $
. Let
$\kappa $
. Let
![]() $\bar {\beta } = f(\bar {\kappa })$
and
$\bar {\beta } = f(\bar {\kappa })$
and
![]() $\beta = j(\bar {\beta })$
.
$\beta = j(\bar {\beta })$
.
Note that
![]() $f^+ \in \operatorname {\mathrm {ran}}(j)$
implies
$f^+ \in \operatorname {\mathrm {ran}}(j)$
implies
![]() $f \in \operatorname {\mathrm {ran}}(j)$
because for all
$f \in \operatorname {\mathrm {ran}}(j)$
because for all
![]() $\alpha < \kappa $
,
$\alpha < \kappa $
,
![]() $f(\alpha )$
is definable in
$f(\alpha )$
is definable in
![]() $V_\lambda $
as the largest inaccessible cardinal less than
$V_\lambda $
as the largest inaccessible cardinal less than
![]() $f^+(\alpha )$
, so we may define
$f^+(\alpha )$
, so we may define
![]() $\bar {f} = j^{-1}(f)$
. Then we have
$\bar {f} = j^{-1}(f)$
. Then we have
![]() $\bar {f} : \bar {\kappa } \to \bar {\kappa }$
and
$\bar {f} : \bar {\kappa } \to \bar {\kappa }$
and
![]() $\bar {\beta } = j(\bar {f})(\bar {\kappa })$
. Note that
$\bar {\beta } = j(\bar {f})(\bar {\kappa })$
. Note that
For the elementary embedding
![]() $j_1 = j \restriction V_{\bar {\beta }}$
we have
$j_1 = j \restriction V_{\bar {\beta }}$
we have
Let
![]() $g \subset \operatorname {\mathrm {Col}}(\omega , V_{\bar {\beta }})$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
$g \subset \operatorname {\mathrm {Col}}(\omega , V_{\bar {\beta }})$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
![]() $j_2 \in V[g]$
such that
$j_2 \in V[g]$
such that
Letting
![]() $M = V_\beta $
we therefore have
$M = V_\beta $
we therefore have
Because
![]() $\bar {\lambda }$
is inaccessible in V and remains so in
$\bar {\lambda }$
is inaccessible in V and remains so in
![]() $V[g]$
, a Skolem hull argument in
$V[g]$
, a Skolem hull argument in
![]() $V[g]$
yields a transitive set
$V[g]$
yields a transitive set
![]() $M' \in V_{\bar {\lambda }}[g]$
and an elementary embedding
$M' \in V_{\bar {\lambda }}[g]$
and an elementary embedding
![]() $j_3 \in V_{\bar {\lambda }}[g]$
such that
$j_3 \in V_{\bar {\lambda }}[g]$
such that
Let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega , V_\beta )$
be a V-generic filter. By the elementarity of j and the definability of forcing, there is a transitive set
$G \subset \operatorname {\mathrm {Col}}(\omega , V_\beta )$
be a V-generic filter. By the elementarity of j and the definability of forcing, there is a transitive set
![]() $M'' \in V_{\lambda }[G]$
and an elementary embedding
$M'' \in V_{\lambda }[G]$
and an elementary embedding
![]() $j_4 \in V_{\lambda }[G]$
with
$j_4 \in V_{\lambda }[G]$
with
Therefore
![]() $\kappa $
is VSS with respect to f.
$\kappa $
is VSS with respect to f.
3 Relation to other large cardinal properties
It is clear from the definitions that every Shelah cardinal (and a fortiori every Shelah-for-supercompactness cardinal) is VSS. In fact the VSS property is much weaker than either of these traditional large cardinal properties by the following result, which is typical of virtual large cardinals:
Proposition 3.1. If
![]() $0^\sharp $
exists then every Silver indiscernible is a VSS cardinal in L.
$0^\sharp $
exists then every Silver indiscernible is a VSS cardinal in L.
Proof Assume that
![]() $0^\sharp $
exists and let
$0^\sharp $
exists and let
![]() $\kappa $
be a Silver indiscernible. Then there is an elementary embedding
$\kappa $
be a Silver indiscernible. Then there is an elementary embedding
![]() $j: L \to L$
with
$j: L \to L$
with
![]() $\operatorname {\mathrm {crit}}(j) = \kappa $
. Let
$\operatorname {\mathrm {crit}}(j) = \kappa $
. Let
![]() $f : \kappa \to \kappa $
be a function in L and define
$f : \kappa \to \kappa $
be a function in L and define
![]() $\lambda = \max \{j(f)(\kappa ), \kappa +1\}$
. For the elementary embedding
$\lambda = \max \{j(f)(\kappa ), \kappa +1\}$
. For the elementary embedding
![]() $j_1 = j \restriction V_\lambda ^L$
we have
$j_1 = j \restriction V_\lambda ^L$
we have
Let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,V_\lambda ^L)$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
$G \subset \operatorname {\mathrm {Col}}(\omega ,V_\lambda ^L)$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
![]() $j_2 \in L[G]$
such that
$j_2 \in L[G]$
such that
We have
![]() $j_2(f)(\kappa ) = j(f)(\kappa ) \le \lambda $
, so
$j_2(f)(\kappa ) = j(f)(\kappa ) \le \lambda $
, so
![]() $j_2$
witnesses the VSS property for
$j_2$
witnesses the VSS property for
![]() $\kappa $
in L with respect to the function f.
$\kappa $
in L with respect to the function f.
We can obtain a better upper bound for the consistency strength of VSS cardinals in terms of the hierarchy of
![]() $\alpha $
-iterable cardinals defined by Gitman [Reference Gitman3]. If
$\alpha $
-iterable cardinals defined by Gitman [Reference Gitman3]. If
![]() $0^\sharp $
exists then every Silver indiscernible is
$0^\sharp $
exists then every Silver indiscernible is
![]() $\alpha $
-iterable in L for every ordinal
$\alpha $
-iterable in L for every ordinal
![]() $\alpha < \omega _1^L$
by Gitman and Welch [Reference Gitman and Welch6, Theorem 3.11], and we will show that 2-iterable cardinals already exceed VSS cardinals in consistency strength. We will not need the definition of
$\alpha < \omega _1^L$
by Gitman and Welch [Reference Gitman and Welch6, Theorem 3.11], and we will show that 2-iterable cardinals already exceed VSS cardinals in consistency strength. We will not need the definition of
![]() $\alpha $
-iterability below, only a certain established consequence of it in the case
$\alpha $
-iterability below, only a certain established consequence of it in the case
![]() $\alpha = 2$
.
$\alpha = 2$
.
Proposition 3.2. If
![]() $\kappa $
is a
$\kappa $
is a
![]() $2$
-iterable cardinal then
$2$
-iterable cardinal then
![]() $\kappa $
is a stationary limit of cardinals that are VSS in
$\kappa $
is a stationary limit of cardinals that are VSS in
![]() $V_\kappa $
.
$V_\kappa $
.
Proof Assume that
![]() $\kappa $
is 2-iterable. Then by Gitman and Welch [Reference Gitman and Welch6, Theorem 4.7] there is a transitive model M of ZFC with
$\kappa $
is 2-iterable. Then by Gitman and Welch [Reference Gitman and Welch6, Theorem 4.7] there is a transitive model M of ZFC with
![]() $V_\kappa \in M$
and an elementary embedding
$V_\kappa \in M$
and an elementary embedding
![]() $j : M \to N$
with critical point equal to
$j : M \to N$
with critical point equal to
![]() $\kappa $
where N is a transitive model of ZFC and
$\kappa $
where N is a transitive model of ZFC and
![]() $M = V_{j(\kappa )}^N$
.
$M = V_{j(\kappa )}^N$
.
First, we show that
![]() $\kappa $
is VSS in N. Let
$\kappa $
is VSS in N. Let
![]() $f : \kappa \to \kappa $
be in N and therefore also in M. Define
$f : \kappa \to \kappa $
be in N and therefore also in M. Define
![]() $\lambda = \max \{j(f)(\kappa ), \kappa + 1\}$
. Because
$\lambda = \max \{j(f)(\kappa ), \kappa + 1\}$
. Because
![]() $j(f)$
is a function from
$j(f)$
is a function from
![]() $j(\kappa )$
to
$j(\kappa )$
to
![]() $j(\kappa )$
we have
$j(\kappa )$
we have
![]() $\lambda < j(\kappa ) = \mathrm {Ord}^M$
, so
$\lambda < j(\kappa ) = \mathrm {Ord}^M$
, so
![]() $V_\lambda ^M = V_\lambda ^N$
and we have an elementary embedding
$V_\lambda ^M = V_\lambda ^N$
and we have an elementary embedding
![]() $j_1 = j \restriction V_\lambda ^M$
with
$j_1 = j \restriction V_\lambda ^M$
with
Let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,V_\lambda ^N)$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
$G \subset \operatorname {\mathrm {Col}}(\omega ,V_\lambda ^N)$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
![]() $j_2 \in N[G]$
such that
$j_2 \in N[G]$
such that
Because
![]() $j_2(f)(\kappa ) = j(f)(\kappa ) \le \lambda $
, this elementary embedding
$j_2(f)(\kappa ) = j(f)(\kappa ) \le \lambda $
, this elementary embedding
![]() $j_2$
witnesses the VSS property for
$j_2$
witnesses the VSS property for
![]() $\kappa $
in N with respect to f.
$\kappa $
in N with respect to f.
Now let C be club in
![]() $\kappa $
. Then
$\kappa $
. Then
![]() $\kappa \in j(C)$
, so the model N satisfies the statement “there is a VSS cardinal in
$\kappa \in j(C)$
, so the model N satisfies the statement “there is a VSS cardinal in
![]() $j(C)$
” and by the elementarity of j it follows that the model M satisfies the statement “there is a VSS cardinal in C.” Let
$j(C)$
” and by the elementarity of j it follows that the model M satisfies the statement “there is a VSS cardinal in C.” Let
![]() $\bar {\kappa } \in C$
be VSS in M. Because we have
$\bar {\kappa } \in C$
be VSS in M. Because we have
![]() $j(\bar {\kappa }) = \bar {\kappa }$
and
$j(\bar {\kappa }) = \bar {\kappa }$
and
it follows by the elementarity of j that
![]() $\bar {\kappa }$
is VSS in
$\bar {\kappa }$
is VSS in
![]() $V_\kappa $
.
$V_\kappa $
.
Because the set of all ordinals
![]() $\alpha < \kappa $
such that
$\alpha < \kappa $
such that
![]() $V_\alpha \prec _{\Sigma _2} V_\kappa $
is club in
$V_\alpha \prec _{\Sigma _2} V_\kappa $
is club in
![]() $\kappa $
, the conclusion of Proposition 3.2 implies that
$\kappa $
, the conclusion of Proposition 3.2 implies that
![]() $\kappa $
is a stationary limit of cardinals that are both
$\kappa $
is a stationary limit of cardinals that are both
![]() $\Sigma _2$
-reflecting and VSS in
$\Sigma _2$
-reflecting and VSS in
![]() $V_\kappa $
. (Note that the VSS property is upward absolute from
$V_\kappa $
. (Note that the VSS property is upward absolute from
![]() $V_\kappa $
to V but the
$V_\kappa $
to V but the
![]() $\Sigma _2$
-reflecting property might not be.) It follows that ZFC+ “there is a
$\Sigma _2$
-reflecting property might not be.) It follows that ZFC+ “there is a
![]() $\Sigma _2$
-reflecting VSS cardinal” has lower consistency strength than ZFC+ “there is a 2-iterable cardinal.”
$\Sigma _2$
-reflecting VSS cardinal” has lower consistency strength than ZFC+ “there is a 2-iterable cardinal.”
Recall that every VSS cardinal is ineffable by Proposition 2.1. We can obtain a better lower bound for the consistency strength of VSS cardinals in terms of the virtually A-extendible cardinals defined by Gitman and Hamkins [Reference Gitman and Hamkins4, Definition 6]: For a cardinal
![]() $\alpha $
and a class A (meaning either a definable class in ZFC or more generally an arbitrary class in GBC) we say that
$\alpha $
and a class A (meaning either a definable class in ZFC or more generally an arbitrary class in GBC) we say that
![]() $\alpha $
is virtually A-extendible if for every ordinal
$\alpha $
is virtually A-extendible if for every ordinal
![]() $\beta> \alpha $
there is an ordinal
$\beta> \alpha $
there is an ordinal
![]() $\theta $
and a generic elementary embedding
$\theta $
and a generic elementary embedding
with
![]() $ \operatorname {\mathrm {crit}}(j) = \alpha $
and
$ \operatorname {\mathrm {crit}}(j) = \alpha $
and
![]() $j(\alpha )> \beta $
.
$j(\alpha )> \beta $
.
Proposition 3.3. If
![]() $\kappa $
is a VSS cardinal then the structure
$\kappa $
is a VSS cardinal then the structure
![]() $\langle V_\kappa , V_{\kappa +1}; \mathord {\in }\rangle $
satisfies the statement “for every class A there is a virtually A-extendible cardinal.”Footnote
6
$\langle V_\kappa , V_{\kappa +1}; \mathord {\in }\rangle $
satisfies the statement “for every class A there is a virtually A-extendible cardinal.”Footnote
6
Proof Let
![]() $\kappa $
be a VSS cardinal, let
$\kappa $
be a VSS cardinal, let
![]() $A \subset V_\kappa $
, and assume toward a contradiction that no cardinal less than
$A \subset V_\kappa $
, and assume toward a contradiction that no cardinal less than
![]() $\kappa $
is virtually A-extendible in
$\kappa $
is virtually A-extendible in
![]() $\langle V_\kappa , V_{\kappa +1}; \mathord {\in }\rangle $
. Then we may define a function
$\langle V_\kappa , V_{\kappa +1}; \mathord {\in }\rangle $
. Then we may define a function
![]() $f:\kappa \to \kappa $
such that for every ordinal
$f:\kappa \to \kappa $
such that for every ordinal
![]() $\alpha < \kappa $
,
$\alpha < \kappa $
,
![]() $f(\alpha )$
is the least ordinal greater than
$f(\alpha )$
is the least ordinal greater than
![]() $\alpha $
such that for every ordinal
$\alpha $
such that for every ordinal
![]() $\theta < \kappa $
there is no generic elementary embedding from
$\theta < \kappa $
there is no generic elementary embedding from
![]() $\langle V_{f(\alpha )}; \mathord {\in },A \cap V_{f(\alpha )}\rangle $
to
$\langle V_{f(\alpha )}; \mathord {\in },A \cap V_{f(\alpha )}\rangle $
to
![]() $\langle V_\theta; \mathord {\in },A \cap V_\theta \rangle $
that has critical point
$\langle V_\theta; \mathord {\in },A \cap V_\theta \rangle $
that has critical point
![]() $\alpha $
and maps
$\alpha $
and maps
![]() $\alpha $
above
$\alpha $
above
![]() $f(\alpha )$
.
$f(\alpha )$
.
Because
![]() $\kappa $
is VSS, by Lemma 2.2 there is an inaccessible cardinal
$\kappa $
is VSS, by Lemma 2.2 there is an inaccessible cardinal
![]() $\lambda> \kappa $
, a transitive model M of ZFC with
$\lambda> \kappa $
, a transitive model M of ZFC with
![]() $V_\lambda \subset M$
, and a generic elementary embedding
$V_\lambda \subset M$
, and a generic elementary embedding
Defining
![]() $\beta = j(f)(\kappa )$
, we have
$\beta = j(f)(\kappa )$
, we have
Let
![]() $j_1 = j \restriction V_\beta $
, considered as an elementary embedding whose domain is the structure
$j_1 = j \restriction V_\beta $
, considered as an elementary embedding whose domain is the structure
![]() $\langle V_\beta; \mathord {\in }, j(A) \cap V_\beta \rangle $
. Then we have
$\langle V_\beta; \mathord {\in }, j(A) \cap V_\beta \rangle $
. Then we have
Let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,V_\beta )$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
$G \subset \operatorname {\mathrm {Col}}(\omega ,V_\beta )$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
![]() $j_2 \in M[G]$
such that
$j_2 \in M[G]$
such that
Note that
![]() $j_2(\kappa ) = j(\kappa )> j(f)(\kappa ) = \beta $
. By the elementarity of j and the definability of forcing it follows that there is a cardinal
$j_2(\kappa ) = j(\kappa )> j(f)(\kappa ) = \beta $
. By the elementarity of j and the definability of forcing it follows that there is a cardinal
![]() $\alpha < \kappa $
such that, letting
$\alpha < \kappa $
such that, letting
![]() $g \subset \operatorname {\mathrm {Col}}(\omega ,V_{f(\alpha )})$
be a V-generic filter, there is an elementary embedding
$g \subset \operatorname {\mathrm {Col}}(\omega ,V_{f(\alpha )})$
be a V-generic filter, there is an elementary embedding
![]() $j_3 \in V_\lambda [g]$
with
$j_3 \in V_\lambda [g]$
with
Because
![]() $V_\lambda \subset M$
we have
$V_\lambda \subset M$
we have
![]() $j_3 \in M[g]$
, and because
$j_3 \in M[g]$
, and because
![]() $\beta < j(\kappa )$
it then follows by the elementarity of j that there is an ordinal
$\beta < j(\kappa )$
it then follows by the elementarity of j that there is an ordinal
![]() $\theta < \kappa $
and an elementary embedding
$\theta < \kappa $
and an elementary embedding
![]() $j_4 \in V_\lambda [g]$
such that
$j_4 \in V_\lambda [g]$
such that
The existence of such a generic elementary embedding
![]() $j_4$
contradicts the definition of the function f.
$j_4$
contradicts the definition of the function f.
We can state a further consequence of the VSS property in terms of the generic Vopěnka principle defined by Bagaria et al. [Reference Bagaria, Gitman and Schindler1], which says that for every proper class of structures of the same type there is a generic elementary embedding from one of the structures into another. The generic Vopěnka principle, formalized as a statement in GBC, follows from the existence of a virtually A-extendible cardinal for every class A by Gitman and Hamkins [Reference Gitman and Hamkins4, Theorem 7].Footnote 7 Combining this fact with Proposition 3.3 gives the following result.
Corollary 3.4. If
![]() $\kappa $
is a VSS cardinal then the structure
$\kappa $
is a VSS cardinal then the structure
![]() $\langle V_\kappa , V_{\kappa +1}; \mathord {\in }\rangle $
satisfies the generic Vopěnka principle.
$\langle V_\kappa , V_{\kappa +1}; \mathord {\in }\rangle $
satisfies the generic Vopěnka principle.
Remark 3.5. Gitman and Hamkins [Reference Gitman and Hamkins4, Theorem 7] showed more specifically that the generic Vopěnka principle is equivalent to the existence of a proper class of weakly virtually A-extendible cardinals for every class A, where the definition of weak virtual A-extendibility is obtained from the definition of virtual A-extendibility by removing the requirement that the image of the critical point is greater than the rank of the domain structure. Solovay et al. [Reference Solovay, Reinhardt and Kanamori16, Theorem 6.9] proved an analogous result in the non-virtual setting, where Kunen’s inconsistency erases the distinction between weak extendibility and extendibility: Vopěnka’s principle is equivalent to the existence of an A-extendible cardinal for every class A.
4 Thin universally Baire sets of reals
In this section we will establish some equivalent conditions for a universally Baire set of reals to be thin, meaning to have no perfect subset. To do this we will need a characterization of universal Baireness in terms of trees and forcing due to Feng et al. [Reference Feng, Magidor and Woodin2].
For a class X, a tree on X is a subset of
![]() $X^{\mathord {<}\omega }$
that is closed under initial segments. For a tree T on X we let
$X^{\mathord {<}\omega }$
that is closed under initial segments. For a tree T on X we let
![]() $[T]$
denote the set of all infinite branches of T. Note that
$[T]$
denote the set of all infinite branches of T. Note that
![]() $[T]$
is a closed subset of
$[T]$
is a closed subset of
![]() $X^\omega $
(where X has the discrete topology) and conversely every closed subset of
$X^\omega $
(where X has the discrete topology) and conversely every closed subset of
![]() $X^\omega $
is the set of branches of some tree on X.
$X^\omega $
is the set of branches of some tree on X.
We will typically consider trees on
![]() $\omega \times \mathrm {Ord}$
, whose elements we may think of either as finite sequences of pairs or as pairs of equal-length finite sequences. Because we require trees to be sets, a tree on
$\omega \times \mathrm {Ord}$
, whose elements we may think of either as finite sequences of pairs or as pairs of equal-length finite sequences. Because we require trees to be sets, a tree on
![]() $\omega \times \mathrm {Ord}$
is actually a tree on
$\omega \times \mathrm {Ord}$
is actually a tree on
![]() $\omega \times \gamma $
for some ordinal
$\omega \times \gamma $
for some ordinal
![]() $\gamma $
, but there is usually no need to specify a particular ordinal.
$\gamma $
, but there is usually no need to specify a particular ordinal.
The letter
![]() $\operatorname {\mathrm {p}}$
denotes projection:
$\operatorname {\mathrm {p}}$
denotes projection:
For a tree T on
![]() $\omega \times \mathrm {Ord}$
and a real
$\omega \times \mathrm {Ord}$
and a real
![]() $x \in \omega ^\omega $
we define the section
$x \in \omega ^\omega $
we define the section
![]() $T_x$
of T as the set of all
$T_x$
of T as the set of all
![]() $s \in \mathrm {Ord}^{\mathord {<}\omega }$
such that
$s \in \mathrm {Ord}^{\mathord {<}\omega }$
such that
![]() $\langle x \restriction \left | s \right |, s\rangle \in T$
. Note that
$\langle x \restriction \left | s \right |, s\rangle \in T$
. Note that
![]() $T_x$
is a tree on
$T_x$
is a tree on
![]() $\mathrm {Ord}$
that is illfounded if and only if
$\mathrm {Ord}$
that is illfounded if and only if
![]() $x \in \operatorname {\mathrm {p}}[T]$
, so the statement
$x \in \operatorname {\mathrm {p}}[T]$
, so the statement
![]() $x \in \operatorname {\mathrm {p}}[T]$
is absolute to all transitive models of ZFC containing x and T by the absoluteness of wellfoundedness.
$x \in \operatorname {\mathrm {p}}[T]$
is absolute to all transitive models of ZFC containing x and T by the absoluteness of wellfoundedness.
A pair of trees
![]() $\langle T,\tilde {T}\rangle $
on
$\langle T,\tilde {T}\rangle $
on
![]() $\omega \times \mathrm {Ord}$
is complementing if
$\omega \times \mathrm {Ord}$
is complementing if
and for a poset
![]() $\mathbb {P}$
it is
$\mathbb {P}$
it is
![]() $\mathbb {P}$
-absolutely complementing if it is complementing in every generic extension of V by
$\mathbb {P}$
-absolutely complementing if it is complementing in every generic extension of V by
![]() $\mathbb {P}$
. The statement
$\mathbb {P}$
. The statement
![]() $\operatorname {\mathrm {p}}[T] \cap \operatorname {\mathrm {p}}[\tilde {T}] = \emptyset $
is generically absolute by the absoluteness of wellfoundedness of the tree of all triples
$\operatorname {\mathrm {p}}[T] \cap \operatorname {\mathrm {p}}[\tilde {T}] = \emptyset $
is generically absolute by the absoluteness of wellfoundedness of the tree of all triples
![]() $\langle r,s_1,s_2\rangle $
such that
$\langle r,s_1,s_2\rangle $
such that
![]() $\langle r, s_1\rangle \in T$
and
$\langle r, s_1\rangle \in T$
and
![]() $\langle r,s_2\rangle \in \tilde {T}$
, so a complementing pair of trees
$\langle r,s_2\rangle \in \tilde {T}$
, so a complementing pair of trees
![]() $\langle T,\tilde {T}\rangle $
is
$\langle T,\tilde {T}\rangle $
is
![]() $\mathbb {P}$
-absolutely complementing if and only if
$\mathbb {P}$
-absolutely complementing if and only if
![]() $\operatorname {\mathrm {p}}[T] \cup \operatorname {\mathrm {p}}[\tilde {T}] = \omega ^\omega $
in every generic extension of V by
$\operatorname {\mathrm {p}}[T] \cup \operatorname {\mathrm {p}}[\tilde {T}] = \omega ^\omega $
in every generic extension of V by
![]() $\mathbb {P}$
. A tree T is called
$\mathbb {P}$
. A tree T is called
![]() $\mathbb {P}$
-absolutely complemented if there is a tree
$\mathbb {P}$
-absolutely complemented if there is a tree
![]() $\tilde {T}$
such that the pair
$\tilde {T}$
such that the pair
![]() $\langle T,\tilde {T}\rangle $
is
$\langle T,\tilde {T}\rangle $
is
![]() $\mathbb {P}$
-absolutely complementing.
$\mathbb {P}$
-absolutely complementing.
We say that a set of reals A is
![]() $\mathbb {P}$
-Baire if
$\mathbb {P}$
-Baire if
![]() $A = \operatorname {\mathrm {p}}[T]$
for some
$A = \operatorname {\mathrm {p}}[T]$
for some
![]() $\mathbb {P}$
-absolutely complemented tree T on
$\mathbb {P}$
-absolutely complemented tree T on
![]() $\omega \times \mathrm {Ord}$
. By Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 2.1] a set of reals is universally Baire if and only if it is
$\omega \times \mathrm {Ord}$
. By Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 2.1] a set of reals is universally Baire if and only if it is
![]() $\mathbb {P}$
-Baire for every poset
$\mathbb {P}$
-Baire for every poset
![]() $\mathbb {P}$
. We will adopt this characterization of universal Baireness as our definition from now on.
$\mathbb {P}$
. We will adopt this characterization of universal Baireness as our definition from now on.
For a cardinal
![]() $\kappa $
, we say that a set of reals is
$\kappa $
, we say that a set of reals is
![]() $\kappa $
-universally Baire if it is
$\kappa $
-universally Baire if it is
![]() $\mathbb {P}$
-Baire for every poset
$\mathbb {P}$
-Baire for every poset
![]() $\mathbb {P}$
of cardinality less than
$\mathbb {P}$
of cardinality less than
![]() $\kappa $
. We denote the pointclass of all
$\kappa $
. We denote the pointclass of all
![]() $\kappa $
-universally Baire sets of reals by
$\kappa $
-universally Baire sets of reals by
![]() $\operatorname {\mathrm {uB}}_\kappa $
. Note that if
$\operatorname {\mathrm {uB}}_\kappa $
. Note that if
![]() $\kappa $
is inaccessible, then a set of reals is
$\kappa $
is inaccessible, then a set of reals is
![]() $\kappa $
-universally Baire if and only if it is
$\kappa $
-universally Baire if and only if it is
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
-Baire.Footnote
8
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
-Baire.Footnote
8
If a set of reals A is
![]() $\mathbb {P}$
-Baire and
$\mathbb {P}$
-Baire and
![]() $G \subset \mathbb {P}$
is a V-generic filter, then the canonical extension of A to
$G \subset \mathbb {P}$
is a V-generic filter, then the canonical extension of A to
![]() $V[G]$
is the set of reals
$V[G]$
is the set of reals
![]() $A^{V[G]}$
in
$A^{V[G]}$
in
![]() $V[G]$
defined by
$V[G]$
defined by
where T is a
![]() $\mathbb {P}$
-absolutely complemented tree in V such that
$\mathbb {P}$
-absolutely complemented tree in V such that
![]() $A = \operatorname {\mathrm {p}}[T]^V$
. By a standard argument using the absoluteness of wellfoundedness, this definition of the canonical extension does not depend on the choice of T.
$A = \operatorname {\mathrm {p}}[T]^V$
. By a standard argument using the absoluteness of wellfoundedness, this definition of the canonical extension does not depend on the choice of T.
For every positive integer n, all of the above definitions and facts about universally Baire sets of reals can easily be generalized to universally Baire n-ary relations on the reals by replacing trees on
![]() $\omega \times \mathrm {Ord}$
with trees on
$\omega \times \mathrm {Ord}$
with trees on
![]() $\omega ^n \times \mathrm {Ord}$
.
$\omega ^n \times \mathrm {Ord}$
.
Now we can establish some equivalent conditions for thinness. The equivalence of statements 1 and 2 seems to be well known (and perhaps the others are also) but we are not aware of a reference.
Lemma 4.1. For every universally Baire set of reals A, the following statements are equivalent in ZFC.
-
1. A is thin.
-
2.
 $A^{V[G]} = A^V$
for every generic extension
$A^{V[G]} = A^V$
for every generic extension
 $V[G]$
of V.
$V[G]$
of V. -
3. For every
 $n < \omega $
, every subset of
$n < \omega $
, every subset of
 $A^n$
is universally Baire.
$A^n$
is universally Baire. -
4. There is a universally Baire wellordering of A.
Proof (1)
![]() $\!\implies\! $
(2): Assume that statement 2 fails, so there is a generic extension
$\!\implies\! $
(2): Assume that statement 2 fails, so there is a generic extension
![]() $V[G]$
of V by a poset
$V[G]$
of V by a poset
![]() $\mathbb {P}$
and a real
$\mathbb {P}$
and a real
![]() $x \in A^{V[G]} \setminus V$
. Letting
$x \in A^{V[G]} \setminus V$
. Letting
![]() $\langle T,\tilde {T}\rangle $
be a
$\langle T,\tilde {T}\rangle $
be a
![]() $\mathbb {P}$
-absolutely complementing pair of trees for A in V we have
$\mathbb {P}$
-absolutely complementing pair of trees for A in V we have
![]() $x \in \operatorname {\mathrm {p}}[T]^{V[G]} \setminus L[T]$
, so by Mansfield’s theorem in
$x \in \operatorname {\mathrm {p}}[T]^{V[G]} \setminus L[T]$
, so by Mansfield’s theorem in
![]() $V[G]$
(see Jech [Reference Jech7, Lemma 25.24]) there is a perfect tree
$V[G]$
(see Jech [Reference Jech7, Lemma 25.24]) there is a perfect tree
![]() $U \in L[T]$
on
$U \in L[T]$
on
![]() $\omega $
such that
$\omega $
such that
![]() $[U] \subset \operatorname {\mathrm {p}}[T]$
in
$[U] \subset \operatorname {\mathrm {p}}[T]$
in
![]() $V[G]$
. This implies
$V[G]$
. This implies
![]() $[U] \subset A$
in V, so A is not thin.
$[U] \subset A$
in V, so A is not thin.
(2)
![]() $\!\implies\! $
(3): Assume statement 2 and let
$\!\implies\! $
(3): Assume statement 2 and let
![]() $\mathbb {P}$
be a poset. Then there is a tree
$\mathbb {P}$
be a poset. Then there is a tree
![]() $T_{\neg A}$
on
$T_{\neg A}$
on
![]() $\omega \times \mathrm {Ord}$
such that
$\omega \times \mathrm {Ord}$
such that
Let
![]() $n< \omega $
. From
$n< \omega $
. From
![]() $T_{\neg A}$
, one can define a tree
$T_{\neg A}$
, one can define a tree
![]() $T_{\neg A^n}$
on
$T_{\neg A^n}$
on
![]() $\omega ^n \times \mathrm {Ord}$
such that
$\omega ^n \times \mathrm {Ord}$
such that
Now let
![]() $B \subset A^n$
. We will show that B is
$B \subset A^n$
. We will show that B is
![]() $\mathbb {P}$
-Baire. Take trees
$\mathbb {P}$
-Baire. Take trees
![]() $T_B$
and
$T_B$
and
![]() $T_{A^n \setminus B}$
on
$T_{A^n \setminus B}$
on
![]() $\omega ^n \times \left |B\right |$
and
$\omega ^n \times \left |B\right |$
and
![]() $\omega ^n \times \left |A^n \setminus B\right |$
respectively that project to B and
$\omega ^n \times \left |A^n \setminus B\right |$
respectively that project to B and
![]() $A^n \setminus B$
respectively in every generic extension. (Such trees can be trivially defined for every pointset.) From
$A^n \setminus B$
respectively in every generic extension. (Such trees can be trivially defined for every pointset.) From
![]() $T_{\neg A^n}$
and
$T_{\neg A^n}$
and
![]() $T_{A^n \setminus B}$
, one can define a tree
$T_{A^n \setminus B}$
, one can define a tree
![]() $T_{\neg B}$
on
$T_{\neg B}$
on
![]() $\omega ^n \times \mathrm {Ord}$
such that every generic extension satisfies
$\omega ^n \times \mathrm {Ord}$
such that every generic extension satisfies
![]() $\operatorname {\mathrm {p}}[T_{\neg B}] = \operatorname {\mathrm {p}}[T_{\neg A^n}] \cup \operatorname {\mathrm {p}}[T_{A^n \setminus B}]$
. Then we have
$\operatorname {\mathrm {p}}[T_{\neg B}] = \operatorname {\mathrm {p}}[T_{\neg A^n}] \cup \operatorname {\mathrm {p}}[T_{A^n \setminus B}]$
. Then we have
so the pair of trees
![]() $\langle T_B, T_{\neg {B}}\rangle $
witnesses that B is
$\langle T_B, T_{\neg {B}}\rangle $
witnesses that B is
![]() $\mathbb {P}$
-Baire.
$\mathbb {P}$
-Baire.
(3)
![]() $\!\implies\! $
(4): This follows directly from the existence of a wellordering of A given by the axiom of choice.
$\!\implies\! $
(4): This follows directly from the existence of a wellordering of A given by the axiom of choice.
(4)
![]() $\!\implies\! $
(1): Suppose toward a contradiction that some universally Baire set of reals A has a universally Baire wellordering but is not thin. Because A has a perfect subset there is a continuous injection
$\!\implies\! $
(1): Suppose toward a contradiction that some universally Baire set of reals A has a universally Baire wellordering but is not thin. Because A has a perfect subset there is a continuous injection
![]() $f : 2^\omega \to \omega ^\omega $
whose range is contained in A. Taking the preimage of a universally Baire wellordering of A under the continuous function
$f : 2^\omega \to \omega ^\omega $
whose range is contained in A. Taking the preimage of a universally Baire wellordering of A under the continuous function
![]() $f \times f$
we obtain a wellordering of
$f \times f$
we obtain a wellordering of
![]() $2^\omega $
with the Baire property, which leads to a contradiction using the Kuratowski–Ulam theorem (see Kanamori [Reference Kanamori8, Corollary 13.10]).
$2^\omega $
with the Baire property, which leads to a contradiction using the Kuratowski–Ulam theorem (see Kanamori [Reference Kanamori8, Corollary 13.10]).
5 Proof of Theorem 1.2
The following “universally Baire reflection” lemma is the key to obtaining consequences of the VSS property by forcing. Our statement of the lemma will use the following definition. For a V-generic filter G on the Levy collapse poset
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
and an ordinal
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
and an ordinal
![]() $\alpha < \kappa $
we define
$\alpha < \kappa $
we define
![]() $G \restriction \alpha = G \cap \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\alpha )$
, which is a V-generic filter on
$G \restriction \alpha = G \cap \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\alpha )$
, which is a V-generic filter on
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\alpha )$
.
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\alpha )$
.
Lemma 5.1. Let
![]() $\kappa $
be a VSS cardinal, let
$\kappa $
be a VSS cardinal, let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
be a V-generic filter, and let A be a universally Baire set of reals in
$G \subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
be a V-generic filter, and let A be a universally Baire set of reals in
![]() $V[G]$
. Then there is an ordinal
$V[G]$
. Then there is an ordinal
![]() $\alpha < \kappa $
and a
$\alpha < \kappa $
and a
![]() $\kappa $
-universally Baire set of reals
$\kappa $
-universally Baire set of reals
![]() $A_0$
in
$A_0$
in
![]() $V[G\restriction \alpha ]$
such that
$V[G\restriction \alpha ]$
such that
![]() $A = A_0^{V[G]}$
.
$A = A_0^{V[G]}$
.
Proof Suppose toward a contradiction that for every ordinal
![]() $\alpha < \kappa $
we have
$\alpha < \kappa $
we have
Then for every ordinal
![]() $\alpha < \kappa $
, because
$\alpha < \kappa $
, because
![]() $\kappa $
is inaccessible in
$\kappa $
is inaccessible in
![]() $V[G \restriction \alpha ]$
and we have
$V[G \restriction \alpha ]$
and we have
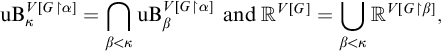 $$ \begin{align*} \operatorname{\mathrm{uB}}_\kappa^{V[G \restriction \alpha]} = \bigcap_{\beta < \kappa} \operatorname{\mathrm{uB}}_\beta^{V[G \restriction \alpha]} \text{ and } \mathbb{R}^{V[G]} = \bigcup_{\beta < \kappa} \mathbb{R}^{V[G\restriction \beta]},\end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{uB}}_\kappa^{V[G \restriction \alpha]} = \bigcap_{\beta < \kappa} \operatorname{\mathrm{uB}}_\beta^{V[G \restriction \alpha]} \text{ and } \mathbb{R}^{V[G]} = \bigcup_{\beta < \kappa} \mathbb{R}^{V[G\restriction \beta]},\end{align*} $$
it follows that for all sufficiently large ordinals
![]() $\beta < \kappa $
we have
$\beta < \kappa $
we have
![]() $\beta> \alpha $
and
$\beta> \alpha $
and
Then by the
![]() $\kappa $
-chain condition for
$\kappa $
-chain condition for
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
it follows that there is a function
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
it follows that there is a function
![]() $f : \kappa \to \kappa $
in V such that for all
$f : \kappa \to \kappa $
in V such that for all
![]() $\alpha < \kappa $
we have
$\alpha < \kappa $
we have
![]() $f(\alpha )> \alpha $
and
$f(\alpha )> \alpha $
and
Because
![]() $\kappa $
is a limit of inaccessible cardinals in V we may additionally assume that
$\kappa $
is a limit of inaccessible cardinals in V we may additionally assume that
![]() $f(\alpha )$
is inaccessible in V for every ordinal
$f(\alpha )$
is inaccessible in V for every ordinal
![]() $\alpha < \kappa $
.
$\alpha < \kappa $
.
Because
![]() $\kappa $
is VSS, by Lemma 2.2 there is an inaccessible cardinal
$\kappa $
is VSS, by Lemma 2.2 there is an inaccessible cardinal
![]() $\lambda> \kappa $
, a transitive model M of ZFC in V with
$\lambda> \kappa $
, a transitive model M of ZFC in V with
![]() $V_\lambda \subset M$
, and a generic elementary embedding
$V_\lambda \subset M$
, and a generic elementary embedding
Defining
![]() $\beta = j(f)(\kappa )$
, we have
$\beta = j(f)(\kappa )$
, we have
Note that
![]() $\beta $
is inaccessible in M by our assumption on f and the elementarity of j, and because
$\beta $
is inaccessible in M by our assumption on f and the elementarity of j, and because
![]() $V_\lambda \subset M$
and
$V_\lambda \subset M$
and
![]() $\beta < \lambda $
this implies that
$\beta < \lambda $
this implies that
![]() $\beta $
is also inaccessible in V.
$\beta $
is also inaccessible in V.
We can extend j to a generic elementary embedding
where
![]() $H\subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}j(\kappa ))$
is an V-generic filter such that
$H\subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}j(\kappa ))$
is an V-generic filter such that
![]() $H \restriction \kappa = G$
. By the fact that
$H \restriction \kappa = G$
. By the fact that
![]() $\beta = j(f)(\kappa )$
and the elementarity of
$\beta = j(f)(\kappa )$
and the elementarity of
![]() ${\hat \jmath }$
it follows that
${\hat \jmath }$
it follows that
We will now obtain a contradiction by showing
In
![]() $V[G]$
, because A is universally Baire we may take a
$V[G]$
, because A is universally Baire we may take a
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\beta )$
-absolutely complementing pair of trees
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\beta )$
-absolutely complementing pair of trees
![]() $\langle T,\tilde {T}\rangle $
on
$\langle T,\tilde {T}\rangle $
on
![]() $\omega \times \mathrm {Ord}$
such that
$\omega \times \mathrm {Ord}$
such that
![]() $\operatorname {\mathrm {p}}[T] = A$
. We may assume that
$\operatorname {\mathrm {p}}[T] = A$
. We may assume that
![]() $\langle T,\tilde {T}\rangle \in V_{\lambda }[G]$
: if necessary, replace T and
$\langle T,\tilde {T}\rangle \in V_{\lambda }[G]$
: if necessary, replace T and
![]() $\tilde {T}$
by their images under the transitive collapse of an elementary substructure of a sufficiently large rank initial segment of
$\tilde {T}$
by their images under the transitive collapse of an elementary substructure of a sufficiently large rank initial segment of
![]() $V[G]$
containing
$V[G]$
containing
![]() $V_\beta [G] \cup \{\beta ,T,\tilde {T}\}$
and having cardinality
$V_\beta [G] \cup \{\beta ,T,\tilde {T}\}$
and having cardinality
![]() $\beta $
. Because
$\beta $
. Because
![]() $V_\lambda \subset M$
we have
$V_\lambda \subset M$
we have
![]() $V_\lambda [G] \subset M[G]$
and the pair
$V_\lambda [G] \subset M[G]$
and the pair
![]() $\langle T,\tilde {T}\rangle \in V_{\lambda }[G]$
witnesses
$\langle T,\tilde {T}\rangle \in V_{\lambda }[G]$
witnesses
![]() $A \in \operatorname {\mathrm {uB}}^{M[G]}_{\beta }$
as desired.
$A \in \operatorname {\mathrm {uB}}^{M[G]}_{\beta }$
as desired.
We have
![]() $\langle {\hat \jmath }(T), {\hat \jmath }(\tilde {T})\rangle \in M[H]$
and it follows by the elementarity of
$\langle {\hat \jmath }(T), {\hat \jmath }(\tilde {T})\rangle \in M[H]$
and it follows by the elementarity of
![]() ${\hat \jmath }$
that
${\hat \jmath }$
that
Mapping branches pointwise by j gives the inclusions
which are absolute to transitive models containing these trees, so in particular they hold in
![]() $M[H]$
. Because the pair
$M[H]$
. Because the pair
![]() $\langle T,\tilde {T}\rangle $
is
$\langle T,\tilde {T}\rangle $
is
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\beta )$
-absolutely complementing in
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\beta )$
-absolutely complementing in
![]() $V[G]$
and the pair
$V[G]$
and the pair
![]() $\langle {\hat \jmath }(T), {\hat \jmath }(\tilde {T})\rangle $
is complementing in
$\langle {\hat \jmath }(T), {\hat \jmath }(\tilde {T})\rangle $
is complementing in
![]() $M[H]$
, these inclusions become equalities when restricted to the reals of
$M[H]$
, these inclusions become equalities when restricted to the reals of
![]() $M[H \restriction \beta ]$
, so
$M[H \restriction \beta ]$
, so
completing the desired contradiction.
We can use Lemma 5.1 to show that the consistency of statement 1 of Theorem 1.2 (which says there is a VSS cardinal) implies the consistency of statements 2–4, and the corresponding statement for the Baire property:
Proposition 5.2. Let
![]() $\kappa $
be a VSS cardinal and let
$\kappa $
be a VSS cardinal and let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
be a V-generic filter. Then the following statements hold in
$G \subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
be a V-generic filter. Then the following statements hold in
![]() $V[G]$
:
$V[G]$
:
-
•
 $\left |\operatorname {\mathrm {uB}}\right | = \omega _1$
.
$\left |\operatorname {\mathrm {uB}}\right | = \omega _1$
. -
• Every set of reals in
 $L(\operatorname {\mathrm {uB}}, \mathbb {R})$
is Lebesgue measurable, has the Baire property, and has the perfect set property.
$L(\operatorname {\mathrm {uB}}, \mathbb {R})$
is Lebesgue measurable, has the Baire property, and has the perfect set property.
Proof By Lemma 5.1 every universally Baire set of reals in
![]() $V[G]$
is definable in a uniform way from V, G, and elements of
$V[G]$
is definable in a uniform way from V, G, and elements of
![]() $V_\kappa $
, namely an ordinal
$V_\kappa $
, namely an ordinal
![]() $\alpha < \kappa $
and a
$\alpha < \kappa $
and a
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\alpha )$
-name for a
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\alpha )$
-name for a
![]() $\kappa $
-universally Baire set of reals. (Recall that the canonical extension of a
$\kappa $
-universally Baire set of reals. (Recall that the canonical extension of a
![]() $\kappa $
-universally Baire set of reals does not depend on any choice of trees.) Because the rank initial segment
$\kappa $
-universally Baire set of reals does not depend on any choice of trees.) Because the rank initial segment
![]() $V_\kappa $
of the ground model has cardinality
$V_\kappa $
of the ground model has cardinality
![]() $\omega _1$
in
$\omega _1$
in
![]() $V[G]$
, it follows that
$V[G]$
, it follows that
![]() $V[G]$
satisfies
$V[G]$
satisfies
![]() $\left |\operatorname {\mathrm {uB}}\right | = \omega _1$
.
$\left |\operatorname {\mathrm {uB}}\right | = \omega _1$
.
Now let B be a set of reals in
![]() $L(\operatorname {\mathrm {uB}}, \mathbb {R})^{V[G]}$
. Then B is definable in
$L(\operatorname {\mathrm {uB}}, \mathbb {R})^{V[G]}$
. Then B is definable in
![]() $V[G]$
from a universally Baire set A, a real x, and an ordinal
$V[G]$
from a universally Baire set A, a real x, and an ordinal
![]() $\xi $
. By Lemma 5.1 there is an ordinal
$\xi $
. By Lemma 5.1 there is an ordinal
![]() $\alpha < \kappa $
and a tree T on
$\alpha < \kappa $
and a tree T on
![]() $\omega \times \mathrm {Ord}$
in
$\omega \times \mathrm {Ord}$
in
![]() $V[G \restriction \alpha ]$
such that
$V[G \restriction \alpha ]$
such that
![]() $A = \operatorname {\mathrm {p}}[T]^{V[G]}$
. Letting
$A = \operatorname {\mathrm {p}}[T]^{V[G]}$
. Letting
![]() $\dot {T} \in V$
be a
$\dot {T} \in V$
be a
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\alpha )$
name for T and letting
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\alpha )$
name for T and letting
![]() $y \in V[G]$
be a real coding x and the hereditarily countable set
$y \in V[G]$
be a real coding x and the hereditarily countable set
![]() $G \restriction \alpha $
, the set B is definable in
$G \restriction \alpha $
, the set B is definable in
![]() $V[G]$
from the parameter
$V[G]$
from the parameter
![]() $\langle \xi , \dot {T}\rangle \in V$
and the real parameter y. Then B has the three claimed regularity properties by Lemmas III.1.4, III.1.6, and III.1.7 respectively of Solovay [Reference Solovay14].
$\langle \xi , \dot {T}\rangle \in V$
and the real parameter y. Then B has the three claimed regularity properties by Lemmas III.1.4, III.1.6, and III.1.7 respectively of Solovay [Reference Solovay14].
Proceeding with the proof of Theorem 1.2, note that statement 4 of the theorem, which says that every set of reals in
![]() $L(\operatorname {\mathrm {uB}}, \mathbb {R})$
has the perfect set property, obviously implies statement 5 of the theorem, which says that every universally Baire set of reals has the perfect set property.
$L(\operatorname {\mathrm {uB}}, \mathbb {R})$
has the perfect set property, obviously implies statement 5 of the theorem, which says that every universally Baire set of reals has the perfect set property.
We will show that statements 2 and 3 also imply statement 5.
Assume that statement 5 fails, so there is an uncountable thin universally Baire set A. Then by Lemma 4.1 every subset of A is also universally Baire, so
![]() $\left |\operatorname {\mathrm {uB}}\right | \ge 2^{\omega _1}$
and therefore statement 2 fails. To show that statement 3 fails, meaning that there is a non-measurable set of reals in
$\left |\operatorname {\mathrm {uB}}\right | \ge 2^{\omega _1}$
and therefore statement 2 fails. To show that statement 3 fails, meaning that there is a non-measurable set of reals in
![]() $L(\operatorname {\mathrm {uB}}, \mathbb {R})$
, it suffices by Shelah [Reference Shelah13, Theorem 5.1B] to show that
$L(\operatorname {\mathrm {uB}}, \mathbb {R})$
, it suffices by Shelah [Reference Shelah13, Theorem 5.1B] to show that
![]() $L(\operatorname {\mathrm {uB}}, \mathbb {R})$
satisfies DC and the statement “there is a set of reals of cardinality
$L(\operatorname {\mathrm {uB}}, \mathbb {R})$
satisfies DC and the statement “there is a set of reals of cardinality
![]() $\aleph _1$
.” Because every countable sequence of universally Baire sets (or reals) is coded by a single universally Baire set (or real), DC in V implies
$\aleph _1$
.” Because every countable sequence of universally Baire sets (or reals) is coded by a single universally Baire set (or real), DC in V implies
![]() $\text {DC}_{\operatorname {\mathrm {uB}} \cup \mathbb {R}}$
in
$\text {DC}_{\operatorname {\mathrm {uB}} \cup \mathbb {R}}$
in
![]() $L(\operatorname {\mathrm {uB}}, \mathbb {R})$
, which in turn implies DC in
$L(\operatorname {\mathrm {uB}}, \mathbb {R})$
, which in turn implies DC in
![]() $L(\operatorname {\mathrm {uB}}, \mathbb {R})$
by the proof of Solovay [Reference Solovay15, Lemma 1.4]. Because A is universally Baire and thin, it has a universally Baire wellordering by Lemma 4.1. Because A is uncountable, this wellordering has an initial segment of order type
$L(\operatorname {\mathrm {uB}}, \mathbb {R})$
by the proof of Solovay [Reference Solovay15, Lemma 1.4]. Because A is universally Baire and thin, it has a universally Baire wellordering by Lemma 4.1. Because A is uncountable, this wellordering has an initial segment of order type
![]() $\omega _1$
, giving a set of reals of cardinality
$\omega _1$
, giving a set of reals of cardinality
![]() $\aleph _1$
in
$\aleph _1$
in
![]() $L(\operatorname {\mathrm {uB}}, \mathbb {R})$
as desired.
$L(\operatorname {\mathrm {uB}}, \mathbb {R})$
as desired.
Next we show that statement 5 implies statement 6:
Lemma 5.3. Assume that every universally Baire set of reals has the perfect set property. Then for every function
![]() $f: \omega _1 \to \omega _1$
there is an ordinal
$f: \omega _1 \to \omega _1$
there is an ordinal
![]() $\lambda> \omega _1$
such that for a stationary set of
$\lambda> \omega _1$
such that for a stationary set of
![]() $\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
we have
$\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
we have
![]() $\sigma \cap \omega _1 \in \omega _1$
and
$\sigma \cap \omega _1 \in \omega _1$
and
![]() $\operatorname {\mathrm {o.\!t.}}(\sigma ) \ge f(\sigma \cap \omega _1)$
.
$\operatorname {\mathrm {o.\!t.}}(\sigma ) \ge f(\sigma \cap \omega _1)$
.
Proof Assume toward a contradiction that some function
![]() $f: \omega _1 \to \omega _1$
fails to have this property. For every ordinal
$f: \omega _1 \to \omega _1$
fails to have this property. For every ordinal
![]() $\lambda> \omega _1$
the set
$\lambda> \omega _1$
the set
![]() $\{\sigma \in \mathcal {P}_{\omega _1}(\lambda ) : \sigma \cap \omega _1 \in \omega _1\}$
is always club in
$\{\sigma \in \mathcal {P}_{\omega _1}(\lambda ) : \sigma \cap \omega _1 \in \omega _1\}$
is always club in
![]() $\mathcal {P}_{\omega _1}(\lambda )$
, so it follows from our assumption that the set of all
$\mathcal {P}_{\omega _1}(\lambda )$
, so it follows from our assumption that the set of all
![]() $\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
such that
$\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
such that
![]() $\sigma \cap \omega _1 \in \omega _1$
and
$\sigma \cap \omega _1 \in \omega _1$
and
![]() $\operatorname {\mathrm {o.\!t.}}(\sigma ) < f(\sigma \cap \omega _1)$
contains a club set in
$\operatorname {\mathrm {o.\!t.}}(\sigma ) < f(\sigma \cap \omega _1)$
contains a club set in
![]() $\mathcal {P}_{\omega _1}(\lambda )$
. (This statement holds for
$\mathcal {P}_{\omega _1}(\lambda )$
. (This statement holds for
![]() $\lambda \le \omega _1$
as well, since it is weaker for smaller
$\lambda \le \omega _1$
as well, since it is weaker for smaller
![]() $\lambda $
.) Increasing the values of f if necessary, we may assume that f is a strictly increasing function.
$\lambda $
.) Increasing the values of f if necessary, we may assume that f is a strictly increasing function.
We may consider every real x as coding a structure
![]() $\langle \omega; E_x \rangle $
where
$\langle \omega; E_x \rangle $
where
![]() $E_x$
is a binary relation on
$E_x$
is a binary relation on
![]() $\omega $
. More precisely, for all
$\omega $
. More precisely, for all
![]() $m,n \in \omega $
we let
$m,n \in \omega $
we let
![]() $\langle m,n\rangle \in E_x$
if and only if
$\langle m,n\rangle \in E_x$
if and only if
![]() $x(2^m3^n) = 0$
. We may use AC to choose for every countable ordinal
$x(2^m3^n) = 0$
. We may use AC to choose for every countable ordinal
![]() $\beta $
a real
$\beta $
a real
![]() $x_\beta $
that codes
$x_\beta $
that codes
![]() $\beta $
in the sense that
$\beta $
in the sense that
![]() $\langle \omega; E_{x_\beta }\rangle \cong \langle \beta , \in \rangle $
. We claim that the set of reals
$\langle \omega; E_{x_\beta }\rangle \cong \langle \beta , \in \rangle $
. We claim that the set of reals
which is uncountable, is also universally Baire and thin. This will contradict our assumption that every universally Baire set of reals has the perfect set property.
Because
![]() $\left |A\right | = \omega _1$
we may trivially define a tree T on
$\left |A\right | = \omega _1$
we may trivially define a tree T on
![]() $\omega \times \omega _1$
from A such that
$\omega \times \omega _1$
from A such that
![]() $\operatorname {\mathrm {p}}[T] = A$
in every generic extension of V. To prove the claim, it will therefore suffice to show that for every poset
$\operatorname {\mathrm {p}}[T] = A$
in every generic extension of V. To prove the claim, it will therefore suffice to show that for every poset
![]() $\mathbb {P}$
there is a tree
$\mathbb {P}$
there is a tree
![]() $\tilde {T}$
on
$\tilde {T}$
on
![]() $\omega \times \mathrm {Ord}$
such that the pair
$\omega \times \mathrm {Ord}$
such that the pair
![]() $\langle T,\tilde {T}\rangle $
is
$\langle T,\tilde {T}\rangle $
is
![]() $\mathbb {P}$
-absolutely complementing, which for this trivial tree T simply means
$\mathbb {P}$
-absolutely complementing, which for this trivial tree T simply means
This will show that A is universally Baire by definition, and also that A is thin by condition 2 of Lemma 4.1. (Note that our assumption on f is only needed to prove universal Baireness of A. The thinness of A follows from the more general fact that the set
![]() $\{x_\beta : \beta <\omega _1\}$
is thin, which can be proved using the boundedness lemma; see Jech [Reference Jech7, Corollary 25.14].)
$\{x_\beta : \beta <\omega _1\}$
is thin, which can be proved using the boundedness lemma; see Jech [Reference Jech7, Corollary 25.14].)
Fix a poset
![]() $\mathbb {P}$
and let
$\mathbb {P}$
and let
![]() $\eta = \max \{|\mathbb {P}|^+,\omega _1\}$
. Using our hypothesis on the existence of club sets, for every ordinal
$\eta = \max \{|\mathbb {P}|^+,\omega _1\}$
. Using our hypothesis on the existence of club sets, for every ordinal
![]() $\lambda $
in the interval
$\lambda $
in the interval
![]() $[\omega _1,\eta )$
we may choose a function
$[\omega _1,\eta )$
we may choose a function
such that for every
![]() $g_\lambda $
-closed set
$g_\lambda $
-closed set
![]() $\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
we have
$\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
we have
![]() $\sigma \cap \omega _1 \in \omega _1$
and
$\sigma \cap \omega _1 \in \omega _1$
and
![]() $\operatorname {\mathrm {o.\!t.}}(\sigma ) < f(\sigma \cap \omega _1)$
. (If
$\operatorname {\mathrm {o.\!t.}}(\sigma ) < f(\sigma \cap \omega _1)$
. (If
![]() $\mathbb {P}$
is countable then this interval is empty and there is nothing to do in this step.) Moreover, we can choose
$\mathbb {P}$
is countable then this interval is empty and there is nothing to do in this step.) Moreover, we can choose
![]() $g_\lambda $
to satisfy the additional property that for every
$g_\lambda $
to satisfy the additional property that for every
![]() $g_\lambda $
-closed set
$g_\lambda $
-closed set
![]() $\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
the set
$\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
the set
![]() $\sigma \cap \omega _1$
is f-closed. Then because f is strictly increasing, these properties imply that for every
$\sigma \cap \omega _1$
is f-closed. Then because f is strictly increasing, these properties imply that for every
![]() $g_\lambda $
-closed set
$g_\lambda $
-closed set
![]() $\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
the order type of
$\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
the order type of
![]() $\sigma $
is not in the range of f.
$\sigma $
is not in the range of f.
As a first step in defining the tree
![]() $\tilde {T}$
, note that there is a tree
$\tilde {T}$
, note that there is a tree
![]() $\tilde {T}_1$
on
$\tilde {T}_1$
on
![]() $\omega \times \omega $
in V such that in every generic extension of V we have
$\omega \times \omega $
in V such that in every generic extension of V we have
because this set of reals is
![]() $\Sigma ^1_1$
.
$\Sigma ^1_1$
.
As a second step in defining
![]() $\tilde {T}$
, note that for every ordinal
$\tilde {T}$
, note that for every ordinal
![]() $\beta < \omega _1^V$
there is a tree
$\beta < \omega _1^V$
there is a tree
![]() $\tilde {T}_{2,\beta }$
on
$\tilde {T}_{2,\beta }$
on
![]() $\omega \times \omega $
in V such that in every generic extension of V we have
$\omega \times \omega $
in V such that in every generic extension of V we have
because this set of reals is
![]() $\Sigma ^1_1(x_\beta )$
where
$\Sigma ^1_1(x_\beta )$
where
![]() $x_\beta $
is our chosen code of
$x_\beta $
is our chosen code of
![]() $\beta $
. (The condition
$\beta $
. (The condition
![]() $x \notin A$
in the definition of this set either subtracts the single point
$x \notin A$
in the definition of this set either subtracts the single point
![]() $x_\beta $
from the set or leaves it unchanged, according to whether or not
$x_\beta $
from the set or leaves it unchanged, according to whether or not
![]() $\beta $
is in the range of f.)
$\beta $
is in the range of f.)
As a third step in defining
![]() $\tilde {T}$
, note that for every ordinal
$\tilde {T}$
, note that for every ordinal
![]() $\lambda \in [\omega _1^V, \eta )$
there is a tree
$\lambda \in [\omega _1^V, \eta )$
there is a tree
![]() $\tilde {T}_{3,\lambda }$
on
$\tilde {T}_{3,\lambda }$
on
![]() $\omega \times \lambda $
in V such that in every generic extension of V we have
$\omega \times \lambda $
in V such that in every generic extension of V we have
To show this, it suffices to represent the set of all such reals x as the projection of a closed subset of
![]() $\omega ^\omega \times \lambda ^\omega $
onto its first coordinate. Using a definable pairing function
$\omega ^\omega \times \lambda ^\omega $
onto its first coordinate. Using a definable pairing function
![]() $\lambda \times \omega \cong \lambda $
, it equivalently suffices to represent the set of all such reals x as the projection of a closed subset of
$\lambda \times \omega \cong \lambda $
, it equivalently suffices to represent the set of all such reals x as the projection of a closed subset of
![]() $\omega ^\omega \times \lambda ^\omega \times \omega ^\omega $
onto its first coordinate. An example of such a closed set is the set of all triples
$\omega ^\omega \times \lambda ^\omega \times \omega ^\omega $
onto its first coordinate. An example of such a closed set is the set of all triples
![]() $\langle x, h, y\rangle \in \omega ^\omega \times \lambda ^\omega \times \omega ^\omega $
such that h is an embedding
$\langle x, h, y\rangle \in \omega ^\omega \times \lambda ^\omega \times \omega ^\omega $
such that h is an embedding
![]() $\langle \omega; E_x\rangle \to \langle \lambda ;\mathord {\in }\rangle $
and y is a function telling us how far we have to look ahead in order to verify that the range of h is
$\langle \omega; E_x\rangle \to \langle \lambda ;\mathord {\in }\rangle $
and y is a function telling us how far we have to look ahead in order to verify that the range of h is
![]() $g_\lambda $
-closed. To be precise, we require that for all
$g_\lambda $
-closed. To be precise, we require that for all
![]() $n < \omega $
the pointwise image of the set
$n < \omega $
the pointwise image of the set
![]() $\{ h(i) : i < n \}^{\mathord {<}n}$
under
$\{ h(i) : i < n \}^{\mathord {<}n}$
under
![]() $g_\lambda $
is contained in
$g_\lambda $
is contained in
![]() $\{ h(i) : i < y(n) \}$
.
$\{ h(i) : i < y(n) \}$
.
Now we can define a tree
![]() $\tilde {T}$
on
$\tilde {T}$
on
![]() $\omega \times \eta $
in V as an amalgamation of these trees, so in every generic extension of V we have
$\omega \times \eta $
in V as an amalgamation of these trees, so in every generic extension of V we have
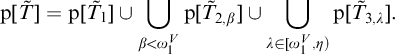 $$ \begin{align*}\operatorname{\mathrm{p}}[\tilde{T}] = \operatorname{\mathrm{p}}[\tilde{T}_1] \cup \bigcup_{\beta < \omega_1^V} \operatorname{\mathrm{p}}[\tilde{T}_{2,\beta}] \cup \bigcup_{\lambda \in [\omega_1^V, \eta)} \operatorname{\mathrm{p}}[\tilde{T}_{3,\lambda}].\end{align*} $$
$$ \begin{align*}\operatorname{\mathrm{p}}[\tilde{T}] = \operatorname{\mathrm{p}}[\tilde{T}_1] \cup \bigcup_{\beta < \omega_1^V} \operatorname{\mathrm{p}}[\tilde{T}_{2,\beta}] \cup \bigcup_{\lambda \in [\omega_1^V, \eta)} \operatorname{\mathrm{p}}[\tilde{T}_{3,\lambda}].\end{align*} $$
Let
![]() $G \subset \mathbb {P}$
be a V-generic filter and let x be a real in
$G \subset \mathbb {P}$
be a V-generic filter and let x be a real in
![]() $V[G]$
. We want to show
$V[G]$
. We want to show
Assume that
![]() $x \in A$
. Then clearly we have
$x \in A$
. Then clearly we have
![]() $x \notin \operatorname {\mathrm {p}}[\tilde {T}_1]$
and
$x \notin \operatorname {\mathrm {p}}[\tilde {T}_1]$
and
![]() $x \notin \operatorname {\mathrm {p}}[\tilde {T}_{2,\beta }]$
for all
$x \notin \operatorname {\mathrm {p}}[\tilde {T}_{2,\beta }]$
for all
![]() $\beta < \omega _1^V$
. By the definition of A we have
$\beta < \omega _1^V$
. By the definition of A we have
![]() $x = x_\beta $
for some
$x = x_\beta $
for some
![]() $\beta $
in the range of f. Now let
$\beta $
in the range of f. Now let
![]() $\lambda \in [\omega _1^V, \eta )$
. As previously noted, the order type of a
$\lambda \in [\omega _1^V, \eta )$
. As previously noted, the order type of a
![]() $g_\lambda $
-closed set
$g_\lambda $
-closed set
![]() $\sigma \in \mathcal {P}_{\omega _1}(\lambda )^V$
cannot be in the range of f, so we have
$\sigma \in \mathcal {P}_{\omega _1}(\lambda )^V$
cannot be in the range of f, so we have
![]() $x \notin \operatorname {\mathrm {p}}[\tilde {T}_{3,\lambda }]$
in V and equivalently in
$x \notin \operatorname {\mathrm {p}}[\tilde {T}_{3,\lambda }]$
in V and equivalently in
![]() $V[G]$
. Therefore
$V[G]$
. Therefore
![]() $x \notin \operatorname {\mathrm {p}}[\tilde {T}]$
.
$x \notin \operatorname {\mathrm {p}}[\tilde {T}]$
.
Conversely, assume that
![]() $x \notin A$
. If
$x \notin A$
. If
![]() $\langle \omega ;E_x\rangle $
is not a wellordering then we have
$\langle \omega ;E_x\rangle $
is not a wellordering then we have
![]() $x \in \operatorname {\mathrm {p}}[\tilde {T}_1]$
. If
$x \in \operatorname {\mathrm {p}}[\tilde {T}_1]$
. If
![]() $\langle \omega ;E_x\rangle $
is a wellordering of order type less than
$\langle \omega ;E_x\rangle $
is a wellordering of order type less than
![]() $\omega _1^V$
then let
$\omega _1^V$
then let
![]() $\beta $
be its order type and note that
$\beta $
be its order type and note that
![]() $x \in \operatorname {\mathrm {p}}[\tilde {T}_{2,\beta }]$
. If
$x \in \operatorname {\mathrm {p}}[\tilde {T}_{2,\beta }]$
. If
![]() $\langle \omega ;E_x\rangle $
is a wellordering of order type greater than or equal to
$\langle \omega ;E_x\rangle $
is a wellordering of order type greater than or equal to
![]() $\omega _1^V$
then let
$\omega _1^V$
then let
![]() $\lambda $
be its order type. Note that
$\lambda $
be its order type. Note that
![]() $\lambda < \eta $
because
$\lambda < \eta $
because
![]() $\eta $
was chosen to be large enough that forcing with
$\eta $
was chosen to be large enough that forcing with
![]() $\mathbb {P}$
does not collapse it to be countable. Therefore
$\mathbb {P}$
does not collapse it to be countable. Therefore
![]() $\lambda \in [\omega _1^V,\eta )$
and we have
$\lambda \in [\omega _1^V,\eta )$
and we have
![]() $x \in \operatorname {\mathrm {p}}[\tilde {T}_{3,\lambda }]$
because
$x \in \operatorname {\mathrm {p}}[\tilde {T}_{3,\lambda }]$
because
![]() $\langle \omega ;E_x\rangle \cong \langle \lambda ;\mathord {\in }\rangle $
and the set
$\langle \omega ;E_x\rangle \cong \langle \lambda ;\mathord {\in }\rangle $
and the set
![]() $\lambda \in \mathcal {P}_{\omega _1}(\lambda )^{V[G]}$
is trivially
$\lambda \in \mathcal {P}_{\omega _1}(\lambda )^{V[G]}$
is trivially
![]() $g_\lambda $
-closed. In every case we have shown the desired conclusion that
$g_\lambda $
-closed. In every case we have shown the desired conclusion that
![]() $x \in \operatorname {\mathrm {p}}[\tilde {T}]$
.
$x \in \operatorname {\mathrm {p}}[\tilde {T}]$
.
Finally, we show that if statement 6 holds then statement 1 holds in L:
Lemma 5.4. Assume that for every function
![]() $f: \omega _1 \to \omega _1$
there is an ordinal
$f: \omega _1 \to \omega _1$
there is an ordinal
![]() $\lambda> \omega _1$
such that for a stationary set of
$\lambda> \omega _1$
such that for a stationary set of
![]() $\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
we have
$\sigma \in \mathcal {P}_{\omega _1}(\lambda )$
we have
![]() $\sigma \cap \omega _1 \in \omega _1$
and
$\sigma \cap \omega _1 \in \omega _1$
and
![]() $\operatorname {\mathrm {o.\!t.}}(\sigma ) \ge f(\sigma \cap \omega _1)$
. Then
$\operatorname {\mathrm {o.\!t.}}(\sigma ) \ge f(\sigma \cap \omega _1)$
. Then
![]() $\omega _1^V$
is VSS in L.
$\omega _1^V$
is VSS in L.
Proof Let
![]() $\kappa = \omega _1^V$
. First we will show that
$\kappa = \omega _1^V$
. First we will show that
![]() $\kappa $
is inaccessible in L. Because
$\kappa $
is inaccessible in L. Because
![]() $\kappa $
is regular in V it is regular in L, so by GCH in L it remains to show that
$\kappa $
is regular in V it is regular in L, so by GCH in L it remains to show that
![]() $\kappa $
is a limit cardinal in L. Suppose toward a contradiction that there is a cardinal
$\kappa $
is a limit cardinal in L. Suppose toward a contradiction that there is a cardinal
![]() $\eta < \kappa $
of L such that
$\eta < \kappa $
of L such that
![]() $(\eta ^+)^L = \kappa $
and define the function
$(\eta ^+)^L = \kappa $
and define the function
![]() $f : \kappa \to \kappa $
in L such that for every ordinal
$f : \kappa \to \kappa $
in L such that for every ordinal
![]() $\alpha < \kappa $
,
$\alpha < \kappa $
,
![]() $f(\alpha )$
is the least ordinal
$f(\alpha )$
is the least ordinal
![]() $\beta> \max \{\alpha , \eta \}$
such that
$\beta> \max \{\alpha , \eta \}$
such that
![]() $L_\beta \models \left | \alpha \right | \le \eta $
. (Note that
$L_\beta \models \left | \alpha \right | \le \eta $
. (Note that
![]() $\beta < \kappa $
by Gödel’s condensation lemma.)
$\beta < \kappa $
by Gödel’s condensation lemma.)
By our assumption there is an ordinal
![]() $\lambda> \kappa $
such that for a stationary set of
$\lambda> \kappa $
such that for a stationary set of
![]() $\sigma \in \mathcal {P}_\kappa (\lambda )$
in V we have
$\sigma \in \mathcal {P}_\kappa (\lambda )$
in V we have
![]() $\sigma \cap \kappa \in \kappa $
and
$\sigma \cap \kappa \in \kappa $
and
![]() $\operatorname {\mathrm {o.\!t.}}(\sigma ) \ge f(\sigma \cap \kappa )$
. Because the set of all
$\operatorname {\mathrm {o.\!t.}}(\sigma ) \ge f(\sigma \cap \kappa )$
. Because the set of all
![]() $X \in \mathcal {P}_\kappa (L_\lambda )$
such that
$X \in \mathcal {P}_\kappa (L_\lambda )$
such that
![]() $X \prec L_\lambda $
,
$X \prec L_\lambda $
,
![]() $X \cap \kappa \in \kappa $
, and
$X \cap \kappa \in \kappa $
, and
![]() $\eta \cup \{\eta \} \subset X$
is club in
$\eta \cup \{\eta \} \subset X$
is club in
![]() $\mathcal {P}_\kappa (L_\lambda )$
, there is such a set X with the additional property that
$\mathcal {P}_\kappa (L_\lambda )$
, there is such a set X with the additional property that
![]() $\bar {\lambda } \ge f(\bar {\kappa })$
where
$\bar {\lambda } \ge f(\bar {\kappa })$
where
![]() $\bar {\kappa } = X \cap \kappa $
and
$\bar {\kappa } = X \cap \kappa $
and
![]() $\bar {\lambda } = \operatorname {\mathrm {o.\!t.}}(X \cap \lambda )$
. Note that
$\bar {\lambda } = \operatorname {\mathrm {o.\!t.}}(X \cap \lambda )$
. Note that
By the condensation lemma, X is the range of an elementary embedding
By the definition of f we have
![]() $L_{f(\bar {\kappa })} \models \left | \bar {\kappa } \right | \le \eta $
, and because
$L_{f(\bar {\kappa })} \models \left | \bar {\kappa } \right | \le \eta $
, and because
![]() $f(\bar {\kappa }) \le \bar {\lambda }$
it follows that
$f(\bar {\kappa }) \le \bar {\lambda }$
it follows that
![]() $L_{\bar {\lambda }} \models \left | \bar {\kappa } \right | \le \eta $
. Then we have
$L_{\bar {\lambda }} \models \left | \bar {\kappa } \right | \le \eta $
. Then we have
![]() $L_\lambda \models \left | \kappa \right | \le \eta $
by the elementarity of j, contradicting the fact that
$L_\lambda \models \left | \kappa \right | \le \eta $
by the elementarity of j, contradicting the fact that
![]() $\kappa $
is a cardinal in V.
$\kappa $
is a cardinal in V.
Now because
![]() $\kappa $
is inaccessible in L it follows that
$\kappa $
is inaccessible in L it follows that
![]() $L_\beta = V_\beta ^L$
for a cofinal set of ordinals
$L_\beta = V_\beta ^L$
for a cofinal set of ordinals
![]() $\beta < \kappa $
. We will use this fact to show that L satisfies statement 2 of Proposition 2.5, which is an equivalent condition for
$\beta < \kappa $
. We will use this fact to show that L satisfies statement 2 of Proposition 2.5, which is an equivalent condition for
![]() $\kappa $
to be VSS.
$\kappa $
to be VSS.
Let
![]() $f : \kappa \to \kappa $
be a function in L and define the function
$f : \kappa \to \kappa $
be a function in L and define the function
![]() $g: \kappa \to \kappa $
in L where for every ordinal
$g: \kappa \to \kappa $
in L where for every ordinal
![]() $\alpha < \kappa $
,
$\alpha < \kappa $
,
![]() $g(\alpha )$
is the least ordinal
$g(\alpha )$
is the least ordinal
![]() $\beta \ge \max \{f(\alpha ), \alpha +1\}$
such that
$\beta \ge \max \{f(\alpha ), \alpha +1\}$
such that
![]() $L_\beta = V_\beta ^L$
. Applying our assumption to the function
$L_\beta = V_\beta ^L$
. Applying our assumption to the function
![]() $g + 1$
, we obtain an ordinal
$g + 1$
, we obtain an ordinal
![]() $\lambda> \kappa $
such that for a stationary set of
$\lambda> \kappa $
such that for a stationary set of
![]() $\sigma \in \mathcal {P}_\kappa (\lambda )$
in V we have
$\sigma \in \mathcal {P}_\kappa (\lambda )$
in V we have
![]() $\sigma \cap \kappa \in \kappa $
and
$\sigma \cap \kappa \in \kappa $
and
![]() $\operatorname {\mathrm {o.\!t.}}(\sigma )> g(\sigma \cap \kappa )$
. By increasing
$\operatorname {\mathrm {o.\!t.}}(\sigma )> g(\sigma \cap \kappa )$
. By increasing
![]() $\lambda $
if necessary, we may assume that
$\lambda $
if necessary, we may assume that
![]() $L_\lambda = V_\lambda ^L$
.
$L_\lambda = V_\lambda ^L$
.
Because the set of all
![]() $X \in \mathcal {P}_\kappa (L_\lambda )$
such that
$X \in \mathcal {P}_\kappa (L_\lambda )$
such that
![]() $X \prec L_\lambda $
,
$X \prec L_\lambda $
,
![]() $X \cap \kappa \in \kappa $
, and
$X \cap \kappa \in \kappa $
, and
![]() $f \in X$
is club in
$f \in X$
is club in
![]() $\mathcal {P}_\kappa (L_\lambda )$
, there is such a set X with the additional property that
$\mathcal {P}_\kappa (L_\lambda )$
, there is such a set X with the additional property that
![]() $\bar {\lambda }> g(\bar {\kappa })$
where
$\bar {\lambda }> g(\bar {\kappa })$
where
![]() $\bar {\kappa } = X \cap \kappa $
and
$\bar {\kappa } = X \cap \kappa $
and
![]() $\bar {\lambda } = \operatorname {\mathrm {o.\!t.}}(X \cap \lambda )$
. By the condensation lemma, X is the range of an elementary embedding
$\bar {\lambda } = \operatorname {\mathrm {o.\!t.}}(X \cap \lambda )$
. By the condensation lemma, X is the range of an elementary embedding
Define
![]() $\bar {\beta } = g(\bar {\kappa })$
and
$\bar {\beta } = g(\bar {\kappa })$
and
![]() $\beta = j(\bar {\beta })$
and
$\beta = j(\bar {\beta })$
and
![]() $\bar {f} = j^{-1}(f)$
. Note that
$\bar {f} = j^{-1}(f)$
. Note that
We have
![]() $L_{\bar {\beta }} = V_{\bar {\beta }}^L$
because
$L_{\bar {\beta }} = V_{\bar {\beta }}^L$
because
![]() $\bar {\beta }$
is in the range of the function g. Therefore
$\bar {\beta }$
is in the range of the function g. Therefore
![]() $L_{\bar {\lambda }} \models L_{\bar {\beta }} = V_{\bar {\beta }}$
and it follows by the elementarity of j that
$L_{\bar {\lambda }} \models L_{\bar {\beta }} = V_{\bar {\beta }}$
and it follows by the elementarity of j that
![]() $L_\lambda \models L_\beta = V_\beta $
. Because
$L_\lambda \models L_\beta = V_\beta $
. Because
![]() $L_\lambda = V_\lambda ^L$
, this implies
$L_\lambda = V_\lambda ^L$
, this implies
![]() $L_\beta = V_\beta ^L$
. For the elementary embedding
$L_\beta = V_\beta ^L$
. For the elementary embedding
![]() $j_1 = j \restriction V_{\bar {\beta }}^L$
we have
$j_1 = j \restriction V_{\bar {\beta }}^L$
we have
Let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,V_{\bar {\beta }}^L)$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
$G \subset \operatorname {\mathrm {Col}}(\omega ,V_{\bar {\beta }}^L)$
be a V-generic filter. Then by the absoluteness of elementary embeddability of countable structures there is an elementary embedding
![]() $j_2 \in L[G]$
such that
$j_2 \in L[G]$
such that
Because
![]() $f(\bar {\kappa }) \le g(\bar {\kappa }) = \bar {\beta }$
, this elementary embedding
$f(\bar {\kappa }) \le g(\bar {\kappa }) = \bar {\beta }$
, this elementary embedding
![]() $j_2$
witnesses statement 2 of Proposition 2.5 for
$j_2$
witnesses statement 2 of Proposition 2.5 for
![]() $\kappa $
in L with respect to the function f.
$\kappa $
in L with respect to the function f.
This completes the proof of Theorem 1.2. The following question remains open.
Question 5.5. What is the consistency strength of the theory ZFC+ “every set of reals in
![]() $L(\mathbb {R},\operatorname {\mathrm {uB}})$
has the Baire property”?
$L(\mathbb {R},\operatorname {\mathrm {uB}})$
has the Baire property”?
An upper bound for the consistency strength is ZFC+ “there is a VSS cardinal” by Proposition 5.2. We do not know any nontrivial lower bound.
6 Proof of Theorem 1.3
When forcing with the Levy collapse over L, the VSS property can be used to limit the complexity of the universally Baire sets of reals in the generic extension:
Proposition 6.1. Let
![]() $\kappa $
be a VSS cardinal in L and let
$\kappa $
be a VSS cardinal in L and let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
be an L-generic filter. Then
$G \subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
be an L-generic filter. Then
![]() $L[G] \models \operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
.
$L[G] \models \operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
.
Proof Let A be a universally Baire set of reals in
![]() $L[G]$
. Then by Lemma 5.1 there is an ordinal
$L[G]$
. Then by Lemma 5.1 there is an ordinal
![]() $\alpha < \kappa $
and a
$\alpha < \kappa $
and a
![]() $\kappa $
-universally Baire set of reals
$\kappa $
-universally Baire set of reals
![]() $A_0$
in
$A_0$
in
![]() $L[G\restriction \alpha ]$
such that
$L[G\restriction \alpha ]$
such that
![]() $A = A_0^{L[G]}$
. Increasing
$A = A_0^{L[G]}$
. Increasing
![]() $\alpha $
if necessary, we may assume that it is a successor ordinal, so
$\alpha $
if necessary, we may assume that it is a successor ordinal, so
![]() $G\restriction \alpha $
is countable in
$G\restriction \alpha $
is countable in
![]() $L[G\restriction \alpha ]$
and therefore
$L[G\restriction \alpha ]$
and therefore
![]() $L[G\restriction \alpha ] = L[z]$
for some real
$L[G\restriction \alpha ] = L[z]$
for some real
![]() $z \in L[G]$
. Take a
$z \in L[G]$
. Take a
![]() $\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
-absolutely complementing pair of trees
$\operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
-absolutely complementing pair of trees
![]() $\langle T, \tilde {T}\rangle $
in
$\langle T, \tilde {T}\rangle $
in
![]() $L[z]$
such that
$L[z]$
such that
![]() $\operatorname {\mathrm {p}}[T]^{L[z]} = A_0$
and therefore
$\operatorname {\mathrm {p}}[T]^{L[z]} = A_0$
and therefore
![]() $\operatorname {\mathrm {p}}[T]^{L[G]} = A$
. Define the sets of reals
$\operatorname {\mathrm {p}}[T]^{L[G]} = A$
. Define the sets of reals
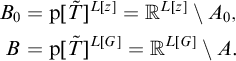 $$ \begin{align*} B_0 &= \operatorname{\mathrm{p}}[\tilde{T}]^{L[z]} = \mathbb{R}^{L[z]} \setminus A_0,\\ B &= \operatorname{\mathrm{p}}[\tilde{T}]^{L[G]} = \mathbb{R}^{L[G]} \setminus A. \end{align*} $$
$$ \begin{align*} B_0 &= \operatorname{\mathrm{p}}[\tilde{T}]^{L[z]} = \mathbb{R}^{L[z]} \setminus A_0,\\ B &= \operatorname{\mathrm{p}}[\tilde{T}]^{L[G]} = \mathbb{R}^{L[G]} \setminus A. \end{align*} $$
We claim that for every real
![]() $x \in L[G]$
we have
$x \in L[G]$
we have
![]() $x \in A$
if and only if there is a tree
$x \in A$
if and only if there is a tree
![]() $T' \in L[z]$
such that
$T' \in L[z]$
such that
![]() $x \in \operatorname {\mathrm {p}}[T']$
and
$x \in \operatorname {\mathrm {p}}[T']$
and
![]() $\operatorname {\mathrm {p}}[T'] \cap B_0 = \emptyset $
. To prove the forward direction of the claim, note that if
$\operatorname {\mathrm {p}}[T'] \cap B_0 = \emptyset $
. To prove the forward direction of the claim, note that if
![]() $x \in A$
then we can simply let
$x \in A$
then we can simply let
![]() $T' = T$
.
$T' = T$
.
To prove the reverse direction of the claim, let x be a real in
![]() $L[G]$
and assume that
$L[G]$
and assume that
![]() $x \in \operatorname {\mathrm {p}}[T']$
for some tree
$x \in \operatorname {\mathrm {p}}[T']$
for some tree
![]() $T' \in L[z]$
such that
$T' \in L[z]$
such that
![]() $\operatorname {\mathrm {p}}[T'] \cap B_0 = \emptyset $
. Because
$\operatorname {\mathrm {p}}[T'] \cap B_0 = \emptyset $
. Because
![]() $\operatorname {\mathrm {p}}[T'] \cap B_0 = \emptyset $
and
$\operatorname {\mathrm {p}}[T'] \cap B_0 = \emptyset $
and
![]() $B_0 = \operatorname {\mathrm {p}}[\tilde {T}]^{L[z]}$
we have
$B_0 = \operatorname {\mathrm {p}}[\tilde {T}]^{L[z]}$
we have
The statement
![]() $\operatorname {\mathrm {p}}[T'] \cap \operatorname {\mathrm {p}}[\tilde {T}] = \emptyset $
is absolute between
$\operatorname {\mathrm {p}}[T'] \cap \operatorname {\mathrm {p}}[\tilde {T}] = \emptyset $
is absolute between
![]() $L[z]$
and
$L[z]$
and
![]() $L[G]$
by the absoluteness of wellfoundedness of the tree of all triples
$L[G]$
by the absoluteness of wellfoundedness of the tree of all triples
![]() $\langle r, s_1, s_2\rangle $
such that
$\langle r, s_1, s_2\rangle $
such that
![]() $\langle r,s_1\rangle \in T'$
and
$\langle r,s_1\rangle \in T'$
and
![]() $\langle r,s_2\rangle \in \tilde {T}$
, so because
$\langle r,s_2\rangle \in \tilde {T}$
, so because
![]() $x \in \operatorname {\mathrm {p}}[T']$
we have
$x \in \operatorname {\mathrm {p}}[T']$
we have
![]() $x \notin \operatorname {\mathrm {p}}[\tilde {T}]$
and therefore
$x \notin \operatorname {\mathrm {p}}[\tilde {T}]$
and therefore
![]() $x \in \operatorname {\mathrm {p}}[T] = A$
.
$x \in \operatorname {\mathrm {p}}[T] = A$
.
We can use the claim to define A in
![]() $L[G]$
without reference to T. Because the class
$L[G]$
without reference to T. Because the class
![]() $L[z]$
is
$L[z]$
is
![]() $\Sigma _1(z)$
and the statement
$\Sigma _1(z)$
and the statement
![]() $y \notin \operatorname {\mathrm {p}}[T']$
(where y is a real) is witnessed by the existence of a rank function for the section tree
$y \notin \operatorname {\mathrm {p}}[T']$
(where y is a real) is witnessed by the existence of a rank function for the section tree
![]() $T_y$
, it follows from the claim that A is
$T_y$
, it follows from the claim that A is
![]() $\Sigma _1(z,B_0)$
. Because
$\Sigma _1(z,B_0)$
. Because
![]() $\text {HC} \prec _{\Sigma _1} V$
, this implies that A is
$\text {HC} \prec _{\Sigma _1} V$
, this implies that A is
![]() $\Sigma _1^{\text {HC}}(z,B_0)$
and is therefore
$\Sigma _1^{\text {HC}}(z,B_0)$
and is therefore
![]() $\Sigma ^1_2(z,b)$
where b is a real coding
$\Sigma ^1_2(z,b)$
where b is a real coding
![]() $B_0$
. A symmetric argument shows that B is
$B_0$
. A symmetric argument shows that B is
![]() ${\boldsymbol {\Sigma }}^1_2$
in
${\boldsymbol {\Sigma }}^1_2$
in
![]() $L[G]$
, so A and B are both
$L[G]$
, so A and B are both
![]() ${\boldsymbol {\Delta }}^1_2$
in
${\boldsymbol {\Delta }}^1_2$
in
![]() $L[G]$
.
$L[G]$
.
Proposition 6.1 is not useful as a relative consistency result because the theory ZFC+ “
![]() $\operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
” is equiconsistent with ZFC by the theorem of Larson and Shelah mentioned in the introduction. However, we can combine Proposition 6.1 with the theorem of Feng et al. mentioned in the introduction to obtain an equiconsistency result at the level of a
$\operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
” is equiconsistent with ZFC by the theorem of Larson and Shelah mentioned in the introduction. However, we can combine Proposition 6.1 with the theorem of Feng et al. mentioned in the introduction to obtain an equiconsistency result at the level of a
![]() $\Sigma _2$
-reflecting VSS cardinal:
$\Sigma _2$
-reflecting VSS cardinal:
Proof of Theorem 1.3 Assume that there is a
![]() $\Sigma _2$
-reflecting VSS cardinal
$\Sigma _2$
-reflecting VSS cardinal
![]() $\kappa $
. Then
$\kappa $
. Then
![]() $\kappa $
is clearly
$\kappa $
is clearly
![]() $\Sigma _2$
-reflecting in L and moreover it is VSS in L by Lemma 2.4. Let
$\Sigma _2$
-reflecting in L and moreover it is VSS in L by Lemma 2.4. Let
![]() $G \subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
be an L-generic filter. Because
$G \subset \operatorname {\mathrm {Col}}(\omega ,\mathord {<}\kappa )$
be an L-generic filter. Because
![]() $\kappa $
is
$\kappa $
is
![]() $\Sigma _2$
-reflecting in L we have
$\Sigma _2$
-reflecting in L we have
![]() ${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
in
${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
in
![]() $L[G]$
by the proof of Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.3]. On the other hand, because
$L[G]$
by the proof of Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.3]. On the other hand, because
![]() $\kappa $
is VSS in L we have
$\kappa $
is VSS in L we have
![]() $\operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
in
$\operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
in
![]() $L[G]$
by Proposition 6.1.
$L[G]$
by Proposition 6.1.
Conversely, assume
![]() $\operatorname {\mathrm {uB}} = {\boldsymbol {\Delta }}^1_2$
. Because
$\operatorname {\mathrm {uB}} = {\boldsymbol {\Delta }}^1_2$
. Because
![]() ${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
, the cardinal
${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
, the cardinal
![]() $\omega _1^V$
is
$\omega _1^V$
is
![]() $\Sigma _2$
-reflecting in L by the proof of Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.3]. Also because
$\Sigma _2$
-reflecting in L by the proof of Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 3.3]. Also because
![]() ${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
, every
${\boldsymbol {\Delta }}^1_2 \subset \operatorname {\mathrm {uB}}$
, every
![]() ${\boldsymbol {\Sigma }}^1_2$
set of reals has the perfect set property by Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 2.4]. Combining this with the assumption that
${\boldsymbol {\Sigma }}^1_2$
set of reals has the perfect set property by Feng et al. [Reference Feng, Magidor and Woodin2, Theorem 2.4]. Combining this with the assumption that
![]() $\operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
shows that every
$\operatorname {\mathrm {uB}} \subset {\boldsymbol {\Delta }}^1_2$
shows that every
![]() $\operatorname {\mathrm {uB}}$
set has the perfect set property, so
$\operatorname {\mathrm {uB}}$
set has the perfect set property, so
![]() $\omega _1^V$
is VSS in L by Lemmas 5.3 and 5.4.
$\omega _1^V$
is VSS in L by Lemmas 5.3 and 5.4.
Acknowledgments
We thank Menachem Magidor, Stamatis Dimopoulos, Paul Larson, and the referee for their helpful comments and suggestions.