1. Introduction
Accurate predictions of the aerodynamic performance are usually the first task of the design of high-speed flying vehicles, which are severely influenced by the laminar–turbulent transition because the drag and heat flux undergo drastic increase during the transition process (Zhong & Wang Reference Zhong and Wang2012). For low environmental perturbations, the boundary-layer transition follows a natural route, for which the receptivity, linear instability, nonlinear interaction of the normal instability mode and turbulence appear in sequence (Kachanov Reference Kachanov1994; Wu Reference Wu2019). As reviewed by Mack (Reference Mack, Dwoyer and Hussaini1987), there exist a multitude of instability modes in hypersonic boundary layers, which are referred to as the Mack first, second and high-order modes, and the second mode is more unstable. The traditional method to predict the natural transition is based on the accumulated growth of the linear instability modes, referred to as the e-N method (Smith Reference Smith1956), which, however, often provides unsatisfactory predictions due to its exclusion of the receptivity process, the effect of surface imperfections, and the nonlinear instability mechanisms. Much attention has been paid to these issues in the recent years. The theoretical descriptions of the hypersonic receptivity mechanism include the leading-edge receptivity (Fedorov & Khokhlov Reference Fedorov and Khokhlov2001; Goldstein & Ricco Reference Goldstein and Ricco2018), the local receptivity (Dong, Liu & Wu Reference Dong, Liu and Wu2020; Zhao, He & Dong Reference Zhao, He and Dong2023), and the receptivity at the neutral point as an optimal excitation (Qin & Wu Reference Qin and Wu2016; Hernández & Wu Reference Hernández and Wu2019). For hypersonic boundary layers with blunt noses, the receptivity efficiency, including the impacts of the bow-shaped shock and entropy layer, was studied by direct numerical simulations (DNS) (Wan, Su & Chen Reference Wan, Su and Chen2020; Niu & Su Reference Niu and Su2023). The impacts of local roughness elements and thermal sources on the Mack instability evolution were formulated by Dong & Zhao (Reference Dong and Zhao2021) and Zhao & Dong (Reference Zhao and Dong2022), respectively. The mechanism of fundamental resonance in hypersonic boundary layers was observed numerically by Hader & Fasel (Reference Hader and Fasel2019), and subsequently formulated using the asymptotic technique by Song, Dong & Zhao (Reference Song, Dong and Zhao2024). A comprehensive review of the nonlinear instability theory can be found in Wu (Reference Wu2019).
When the intensity of the freestream perturbations is moderate, the transition may occur bypassing the appearance of normal instability modes, referred to as the bypass transition. Characterising the freestream vortical disturbances (FSVDs) and their entrainment into the boundary layer is a crucial issue describing the bypass transition process. In the incompressible regime and for relatively weak FSVDs, the mathematical theory for entrainment was formulated by Leib, Wundrow & Goldstein (Reference Leib, Wundrow and Goldstein1999), who showed that long-wavelength (low-frequency) FSVDs generate streaks, and the latter were governed by linear boundary-region equations together with appropriate initial and boundary conditions. The theory was extended by Ricco, Luo & Wu (Reference Ricco, Luo and Wu2011) to the more realistic case of strong FSVDs, where nonlinear boundary-region equations describe the generation and evolution of streaks. The streaks do not break down themselves, but they could support the amplification of the high-frequency perturbations due to the secondary instability (SI) mechanism (Andersson et al. Reference Andersson, Brandt, Bottaro and Henningson2001; Ricco et al. Reference Ricco, Luo and Wu2011; Xu, Zhang & Wu Reference Xu, Zhang and Wu2017). These modes would eventually cause the emergence of the turbulent spots (Wu, Moin & Hickey Reference Wu, Moin and Hickey2014; Wu Reference Wu2023), and their excitation by high-frequency FSVDs was studied by Zhang, Dong & Zhang (Reference Zhang, Dong and Zhang2018). Simulations for the bypass transition by extending the computational domain to include the region upstream of the leading edge can be found in Nagarajan, Lele & Ferziger (Reference Nagarajan, Lele and Ferziger2007) and Ovchinnikov, Choudhari & Piomelli (Reference Ovchinnikov, Choudhari and Piomelli2008). The theory for excitation of streaks by FSVDs in compressible flows was formulated by Ricco & Wu (Reference Ricco and Wu2007) and Marensi, Ricco & Wu (Reference Marensi, Ricco and Wu2017), using the linear and nonlinear boundary-region equations, respectively. Theoretical studies of the bypass transition in hypersonic boundary layers are quite insufficient, and the major difficulty is attributed to the impact of the shock wave forming in the leading-edge vicinity, which, however, could be overcome by numerics. Recently, numerical calculations of the optimal growth of non-modal perturbations in blunt-cone hypersonic boundary layers were conducted by Paredes et al. (Reference Paredes, Choudhari, Li, Jewell and Kimmel2019a,Reference Paredes, Choudhari, Li, Jewell, Kimmel, Marineau and Grossirb). It was found that the amplification rate of the optimal non-modal perturbations increases with the radius of the nose tip, and the bypass transition may appear if the nose radius is over a threshold. A subsequent analysis for hypersonic boundary layers over an elliptic cone, performed by Quintanilha et al. (Reference Quintanilha, Paredes, Hanifi and Theofilis2022), reported strong transient growth of the non-modal perturbations in the vicinity of the cone tip and in the azimuthal locations away from both the centreline and the attachment line. It needs to be noted that the optimal perturbations calculated in these papers do not have a clear connection with the FSVDs, which is the central issue in the bypass transition.
In reality, there may be more complex factors to affect the boundary-layer transition at the surface of cruising hypersonic flying vehicles. The surface vibration, induced by either the mechanical vibration or the aeroelasticity, is a typical factor, but its mechanism affecting the transition onset is not well understood. Usually, the vibration frequency is rather low compared to the normal instability modes in hypersonic boundary layers, and their interaction could be too weak to cause an appreciable impact on natural transition. However, the vibration could affect the non-modal streaks because their frequency bands could be close to each other, leading to potential influences on the bypass transition. For an incompressible boundary layer, Ricco (Reference Ricco2011) studied numerically the effect of steady spanwise wall forcing with a streamwise distribution on the linear evolution of streaks excited by FSVDs, and it was found that the streaks could be attenuated if the forcing has a streamwise length scale comparable with the FSVD-induced streaks and a spanwise length scale comparable to the boundary-layer thickness. Subsequently, Hicks & Ricco (Reference Hicks and Ricco2015) considered the effect of a spanwise oscillating wall on the streak development. In their formulation, the wavelengths of the streaks excited by low-frequency FSVDs are very long, which is taken to be comparable with the Reynolds number based on the boundary-layer thickness ![]() $R_\delta$. Thus even if the amplitude of the spanwise velocity induced by the wall oscillation is as small as
$R_\delta$. Thus even if the amplitude of the spanwise velocity induced by the wall oscillation is as small as ![]() $O(R_\delta ^{-1})$, the evolution of the streaks would be influenced by the oscillation. In this case, the spanwise generalised Stokes layer, together with the Blasius flow, was considered as the base flow for analysis of the streak evolution. It was found that the wall oscillation with this magnitude can enhance or suppress the streak energy remarkably, depending on the oscillation amplitude and frequency, as well as the FSVD property. Similar observations were made by Hack & Zaki (Reference Hack and Zaki2014, Reference Hack and Zaki2015) using DNS, although their inflow conditions, the continuous modes of the Orr–Sommerfeld and Squire equations, were not appropriate (Wu & Dong Reference Wu and Dong2016). The spanwise oscillating wall was also considered as a control strategy for the delay of bypass transition, as shown numerically in Negi et al. (Reference Negi, Mishra, Schlatter and Skote2019). Additionally, the wall oscillation may also be employed as a drag reduction strategy in turbulent boundary layers (Jung, Mangiavacchi & Akhavan Reference Jung, Mangiavacchi and Akhavan1992; Touber & Leschziner Reference Touber and Leschziner2012; Ricco, Skote & Leschziner Reference Ricco, Skote and Leschziner2021).
$O(R_\delta ^{-1})$, the evolution of the streaks would be influenced by the oscillation. In this case, the spanwise generalised Stokes layer, together with the Blasius flow, was considered as the base flow for analysis of the streak evolution. It was found that the wall oscillation with this magnitude can enhance or suppress the streak energy remarkably, depending on the oscillation amplitude and frequency, as well as the FSVD property. Similar observations were made by Hack & Zaki (Reference Hack and Zaki2014, Reference Hack and Zaki2015) using DNS, although their inflow conditions, the continuous modes of the Orr–Sommerfeld and Squire equations, were not appropriate (Wu & Dong Reference Wu and Dong2016). The spanwise oscillating wall was also considered as a control strategy for the delay of bypass transition, as shown numerically in Negi et al. (Reference Negi, Mishra, Schlatter and Skote2019). Additionally, the wall oscillation may also be employed as a drag reduction strategy in turbulent boundary layers (Jung, Mangiavacchi & Akhavan Reference Jung, Mangiavacchi and Akhavan1992; Touber & Leschziner Reference Touber and Leschziner2012; Ricco, Skote & Leschziner Reference Ricco, Skote and Leschziner2021).
However, the aforementioned works on the wall vibration effect were all focusing on low-speed boundary layers. For hypersonic boundary layers, the scenarios may be quite different. First, because the velocity of the oncoming stream is very high, the dimensionless vibrating amplitude should be much lower than that for low-speed boundary layers. Second, the frequency of the hypersonic boundary-layer instability is usually several hundred kilohertz, which is usually much higher than the vibration frequency of the wall. Third, the entrainment of the FSVDs to hypersonic boundary layers and the subsequent excitation of non-modal streaks may be severely affected by the compressible effect (the temperature perturbation for a non-modal mode also shows a streaky feature, but with an even stronger amplitude, which was found to be an important feature by Ricco & Wu (Reference Ricco and Wu2007) and Marensi et al. (Reference Marensi, Ricco and Wu2017)), as well as the interaction between the non-modal streaks and the wall vibration. Therefore, in this paper, we consider the effect of spanwise wall vibration on the evolution of the non-modal streaks subject to FSVDs in hypersonic boundary layers.
The rest of the paper is organised as follows. In § 2, we introduce the physical model and mathematical details for calculating the response of a vibrating boundary layer to low-frequency FSVDs and nonlinear evolution of the excited boundary-layer streaky perturbations. The numerical approach for analysing the growth and the amplitude accumulation of the SI modes of the streaky profiles is introduced in § 2.6. The numerical results of the linear evolution of the non-modal streaks are shown in § 3, and the nonlinear development of the streaks and their SI analysis are shown in § 4. Concluding remarks are provided in § 5.
2. Physical model and mathematical descriptions
2.1. Physical model
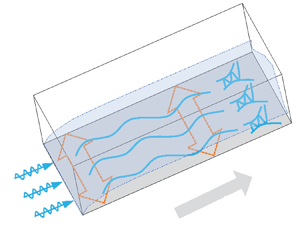
In this paper, we study a hypersonic boundary-layer flow over a flat plate that is vibrating laterally, as sketched in figure 1. The plate is assumed to be infinitely thin, such that a rather weak oblique attached shock wave is formed from its leading edge. We introduce low-frequency FSVDs in the oncoming stream, which enter the boundary layer from the leading edge and generate streaks in the downstream locations. The streaks may undergo transient growths, and reach finite amplitudes if the FSVDs are relatively strong. Then the SI is likely to be excited, leading to bypass transition to turbulence eventually. Meanwhile, the vibrating wall induces another group of low-frequency perturbations to the flow field, which may interact with the FSVD-induced streaks, affecting their SI and leading to premature downstream transition or its delay.

Figure 1. Sketch of the physical model, where the perturbations are defined in (2.3).
The flow field is described by the three-dimensional (3-D) Cartesian coordinate ![]() $(x^*,y^*,z^*)$, with its origin located at the leading edge of the plate, where
$(x^*,y^*,z^*)$, with its origin located at the leading edge of the plate, where ![]() $x^*$,
$x^*$, ![]() $y^*$ and
$y^*$ and ![]() $z^*$ denote the streamwise, wall-normal and spanwise directions, respectively. The typical spanwise wavelength of the FSVDs,
$z^*$ denote the streamwise, wall-normal and spanwise directions, respectively. The typical spanwise wavelength of the FSVDs, ![]() $\varLambda$, is taken to be the reference length, which is also roughly the length scale of the downstream boundary-layer thickness. The dimensionless coordinate system is
$\varLambda$, is taken to be the reference length, which is also roughly the length scale of the downstream boundary-layer thickness. The dimensionless coordinate system is ![]() $(x,y,z)=(x^*,y^*,z^*)/\varLambda$. The velocity field
$(x,y,z)=(x^*,y^*,z^*)/\varLambda$. The velocity field ![]() ${\boldsymbol {u}}=(u,v,w)$, density
${\boldsymbol {u}}=(u,v,w)$, density ![]() $\rho$, temperature
$\rho$, temperature ![]() $T$, pressure
$T$, pressure ![]() $p$, dynamic viscosity
$p$, dynamic viscosity ![]() $\mu$ and time
$\mu$ and time ![]() $t$ are normalised by
$t$ are normalised by ![]() $U_\infty$,
$U_\infty$, ![]() $\rho _\infty$,
$\rho _\infty$, ![]() $T_\infty$,
$T_\infty$, ![]() $\rho _\infty U_\infty ^2$,
$\rho _\infty U_\infty ^2$, ![]() $\mu _\infty$ and
$\mu _\infty$ and ![]() $\varLambda /U_\infty$, respectively. The flow is governed by two dimensionless parameters, the Reynolds number and the Mach number, which are defined as
$\varLambda /U_\infty$, respectively. The flow is governed by two dimensionless parameters, the Reynolds number and the Mach number, which are defined as
where ![]() $a_\infty$ denotes the acoustic speed in the freestream.
$a_\infty$ denotes the acoustic speed in the freestream.
2.2. Governing equations
The governing equations for a perfect-gas flow are the 3-D Navier–Stokes (N–S) equations, which can be found in Dong & Zhao (Reference Dong and Zhao2021). The Sutherland viscosity law is employed, and we choose the ratio of the specific heats ![]() $\gamma = 1.4$ and the Prandtl number
$\gamma = 1.4$ and the Prandtl number ![]() $Pr = 0.72$.
$Pr = 0.72$.
The instantaneous flow field ![]() ${\boldsymbol \varphi }\equiv (u,v,w,\rho,T,p)$ is decomposed as a two-dimensional (2-D) steady base flow
${\boldsymbol \varphi }\equiv (u,v,w,\rho,T,p)$ is decomposed as a two-dimensional (2-D) steady base flow ![]() ${\boldsymbol {\varPhi }}_B\equiv (U_B,V_B,0,\rho _B,T_B,P_B)$ and an unsteady perturbation
${\boldsymbol {\varPhi }}_B\equiv (U_B,V_B,0,\rho _B,T_B,P_B)$ and an unsteady perturbation ![]() $\breve {\boldsymbol \varphi }\equiv (\breve {u}, \breve {v}, \breve {w}, \breve {\rho }, \breve {T}, \breve {p})$:
$\breve {\boldsymbol \varphi }\equiv (\breve {u}, \breve {v}, \breve {w}, \breve {\rho }, \breve {T}, \breve {p})$:
In principle, the evolution of the perturbation ![]() $\breve {\boldsymbol \varphi }$ can be calculated by DNS; however, this is too expensive, and most of the computational load is spent on the unnecessary high-order harmonics. Therefore, in this paper, we develop a more efficient means, namely, the harmonic weakly nonlinear Navier–Stokes (HWNNS) approach plus the nonlinear parabolised stability equation (NPSE) approach, which will be introduced in detail in §§ 2.4 and 2.5.
$\breve {\boldsymbol \varphi }$ can be calculated by DNS; however, this is too expensive, and most of the computational load is spent on the unnecessary high-order harmonics. Therefore, in this paper, we develop a more efficient means, namely, the harmonic weakly nonlinear Navier–Stokes (HWNNS) approach plus the nonlinear parabolised stability equation (NPSE) approach, which will be introduced in detail in §§ 2.4 and 2.5.
The excited perturbations ![]() $\breve {\boldsymbol \varphi }$ include the boundary-layer response to an FSVD
$\breve {\boldsymbol \varphi }$ include the boundary-layer response to an FSVD ![]() $\breve {\boldsymbol \varphi }_f$ (with frequency
$\breve {\boldsymbol \varphi }_f$ (with frequency ![]() $\omega _f$), a vibration-induced perturbation
$\omega _f$), a vibration-induced perturbation ![]() $\breve {\boldsymbol \varphi }_s$ (with frequency
$\breve {\boldsymbol \varphi }_s$ (with frequency ![]() $\omega _s$), the excited perturbation due to their mutual interaction,
$\omega _s$), the excited perturbation due to their mutual interaction, ![]() $\breve \varphi _+$ (with frequency
$\breve \varphi _+$ (with frequency ![]() $\omega _f+\omega _s$) and
$\omega _f+\omega _s$) and ![]() $\breve \varphi _-$ (with frequency
$\breve \varphi _-$ (with frequency ![]() $\omega _f-\omega _s$), and their high-order harmonics. As confirmed by DNS, the amplitudes of the high-order harmonics are lower than those of the direct-interaction-driven perturbations
$\omega _f-\omega _s$), and their high-order harmonics. As confirmed by DNS, the amplitudes of the high-order harmonics are lower than those of the direct-interaction-driven perturbations ![]() $\breve {\varphi }_\pm$. Therefore, the former are truncated in the HWNNS analysis. We consider the frequencies of the FSVD and wall vibration to be low, i.e.
$\breve {\varphi }_\pm$. Therefore, the former are truncated in the HWNNS analysis. We consider the frequencies of the FSVD and wall vibration to be low, i.e. ![]() $\omega _f\ll 1$ and
$\omega _f\ll 1$ and ![]() $\omega _s\ll 1$; then the boundary-layer perturbations
$\omega _s\ll 1$; then the boundary-layer perturbations ![]() $\breve {\boldsymbol {\varphi }}$ have long length scales in the streamwise direction,
$\breve {\boldsymbol {\varphi }}$ have long length scales in the streamwise direction, ![]() $\lambda _x\gg 1$. Thus
$\lambda _x\gg 1$. Thus ![]() $\partial _x\sim \lambda _x^{-1}\ll 1$. Note that even when the frequency is zero, the streamwise length scale is still finite due to the presence of the non-parallelism of the base flow, which is
$\partial _x\sim \lambda _x^{-1}\ll 1$. Note that even when the frequency is zero, the streamwise length scale is still finite due to the presence of the non-parallelism of the base flow, which is ![]() $O(R)$. Because the wall is vibrating laterally as a whole, there is no specific length scale for
$O(R)$. Because the wall is vibrating laterally as a whole, there is no specific length scale for ![]() $\breve {\boldsymbol {\varphi }}_s$ in the
$\breve {\boldsymbol {\varphi }}_s$ in the ![]() $z$-direction, thus
$z$-direction, thus ![]() $\breve {\boldsymbol {\varphi }}_s$ is independent of
$\breve {\boldsymbol {\varphi }}_s$ is independent of ![]() $z$. Additionally, from a preliminary analysis, it is found that the vibration-induced perturbation only has a non-zero
$z$. Additionally, from a preliminary analysis, it is found that the vibration-induced perturbation only has a non-zero ![]() $\breve w_s$ component, namely,
$\breve w_s$ component, namely, ![]() $\breve {\boldsymbol \varphi }_s=(0,0,\breve w_s(x,y),0,0,0)$. The spanwise length scales of
$\breve {\boldsymbol \varphi }_s=(0,0,\breve w_s(x,y),0,0,0)$. The spanwise length scales of ![]() $\breve {\boldsymbol \varphi }_f$ and
$\breve {\boldsymbol \varphi }_f$ and ![]() $\breve {\boldsymbol \varphi }_\pm$ are determined by the spanwise wavelength of the FSVD, which is taken to be
$\breve {\boldsymbol \varphi }_\pm$ are determined by the spanwise wavelength of the FSVD, which is taken to be ![]() $O(1)$ from the normalisation in § 2.1; then we have
$O(1)$ from the normalisation in § 2.1; then we have ![]() $\partial _z(\breve {\boldsymbol \varphi }_{f,\pm })\sim \breve {\boldsymbol \varphi }_{f,\pm }$.
$\partial _z(\breve {\boldsymbol \varphi }_{f,\pm })\sim \breve {\boldsymbol \varphi }_{f,\pm }$.
Since the base-flow velocity is ![]() $U_B=O(1)$, the streamwise convection of the boundary-layer perturbations scales like
$U_B=O(1)$, the streamwise convection of the boundary-layer perturbations scales like ![]() $U_B\,\partial _x \breve u_{f,\pm }\sim \lambda _x^{-1}\breve {u}_{f,\pm }$, while the vibration-induced spanwise redistribution is
$U_B\,\partial _x \breve u_{f,\pm }\sim \lambda _x^{-1}\breve {u}_{f,\pm }$, while the vibration-induced spanwise redistribution is ![]() $w_s\, \partial _z \breve {u}_{f,\pm }\sim w_s\breve {u}_{f,\pm }$, where
$w_s\, \partial _z \breve {u}_{f,\pm }\sim w_s\breve {u}_{f,\pm }$, where ![]() $w_s=\breve w_s\exp ({-{\rm i}\omega _s t})+\breve w_s^C \exp ({{\rm i}\omega _s t})$ denotes the spanwise velocity induced by the wall vibration. In what follows, a superscript
$w_s=\breve w_s\exp ({-{\rm i}\omega _s t})+\breve w_s^C \exp ({{\rm i}\omega _s t})$ denotes the spanwise velocity induced by the wall vibration. In what follows, a superscript ![]() $C$ denotes the complex conjugate. Following Ricco (Reference Ricco2011) and Hicks & Ricco (Reference Hicks and Ricco2015), if we take
$C$ denotes the complex conjugate. Following Ricco (Reference Ricco2011) and Hicks & Ricco (Reference Hicks and Ricco2015), if we take ![]() $w_s\sim \lambda _x^{-1}\ll 1$, then the leading-order balance in the momentum equations determines
$w_s\sim \lambda _x^{-1}\ll 1$, then the leading-order balance in the momentum equations determines ![]() $U_B\,\partial _x \breve {\varphi }_f\sim \breve {w}_s\,\partial _z\breve u_-+\breve {w}_s^C\,\partial _z\breve {u}_+$,
$U_B\,\partial _x \breve {\varphi }_f\sim \breve {w}_s\,\partial _z\breve u_-+\breve {w}_s^C\,\partial _z\breve {u}_+$, ![]() $U_B\,\partial _x \breve {\varphi }_+\sim \breve {w}_s\, \partial _z\breve {u}_f$ and
$U_B\,\partial _x \breve {\varphi }_+\sim \breve {w}_s\, \partial _z\breve {u}_f$ and ![]() $U_B\,\partial _x \breve {\varphi }_-\sim \breve {w}_s^C\,\partial _z\breve {u}_f$. Thus the perturbations
$U_B\,\partial _x \breve {\varphi }_-\sim \breve {w}_s^C\,\partial _z\breve {u}_f$. Thus the perturbations ![]() $\breve \varphi _f$ and
$\breve \varphi _f$ and ![]() $\breve \varphi _\pm$ are coupled. Additionally, the back action of the mutual interaction of
$\breve \varphi _\pm$ are coupled. Additionally, the back action of the mutual interaction of ![]() $\breve {\boldsymbol \varphi }_f$ and
$\breve {\boldsymbol \varphi }_f$ and ![]() $\breve {\boldsymbol {\varphi }}_\pm$ on
$\breve {\boldsymbol {\varphi }}_\pm$ on ![]() $\breve {w}_s$ is rather small; an estimate can be obtained by analysing the spanwise momentum equation of
$\breve {w}_s$ is rather small; an estimate can be obtained by analysing the spanwise momentum equation of ![]() $\breve {\boldsymbol {\varphi }}_s$ as follows. The convection term in the
$\breve {\boldsymbol {\varphi }}_s$ as follows. The convection term in the ![]() $z$ momentum equation scales as
$z$ momentum equation scales as ![]() $U_B\,\partial _x \breve {w}_s\sim \lambda ^{-1}_x\breve {w}_s$, while the mutual interaction of
$U_B\,\partial _x \breve {w}_s\sim \lambda ^{-1}_x\breve {w}_s$, while the mutual interaction of ![]() $\breve {\boldsymbol \varphi }_f$ and
$\breve {\boldsymbol \varphi }_f$ and ![]() $\breve {\boldsymbol \varphi }_\pm$ scales as
$\breve {\boldsymbol \varphi }_\pm$ scales as ![]() $\breve {u}_f\,\partial _x\breve {w}_-\sim \lambda _x^{-2}\tilde {u}_f\tilde {u}_-$, where the condition
$\breve {u}_f\,\partial _x\breve {w}_-\sim \lambda _x^{-2}\tilde {u}_f\tilde {u}_-$, where the condition ![]() $\tilde {w}_-\sim \lambda _x^{-1}\tilde {u}_-$ is imposed from the continuity equation. As mentioned before, we take
$\tilde {w}_-\sim \lambda _x^{-1}\tilde {u}_-$ is imposed from the continuity equation. As mentioned before, we take ![]() $\breve {w}_s\sim \lambda _x^{-1}$; then the mutual interaction of
$\breve {w}_s\sim \lambda _x^{-1}$; then the mutual interaction of ![]() $\breve {\boldsymbol \varphi }_f$ and
$\breve {\boldsymbol \varphi }_f$ and ![]() $\breve {\boldsymbol \varphi }_\pm$ is negligible. In such a situation, the vibration-induced perturbation, to leading order, is governed by the linearised N–S system without any inhomogeneous forcing. The high-order harmonics, including the perturbations excited by the self-interactions of
$\breve {\boldsymbol \varphi }_\pm$ is negligible. In such a situation, the vibration-induced perturbation, to leading order, is governed by the linearised N–S system without any inhomogeneous forcing. The high-order harmonics, including the perturbations excited by the self-interactions of ![]() $\breve {\boldsymbol \varphi }_f$,
$\breve {\boldsymbol \varphi }_f$, ![]() $\breve {\boldsymbol \varphi }_\pm$ and their mutual interactions, are
$\breve {\boldsymbol \varphi }_\pm$ and their mutual interactions, are ![]() $O(\breve {\boldsymbol \varphi }_f^2)$, much smaller than the magnitude of each individual. Because
$O(\breve {\boldsymbol \varphi }_f^2)$, much smaller than the magnitude of each individual. Because ![]() $\breve {\boldsymbol \varphi }_s$ only has non-zero
$\breve {\boldsymbol \varphi }_s$ only has non-zero ![]() $\breve {w}_s$ component with no spanwise length scale, its self-interaction does not induce further perturbations.
$\breve {w}_s$ component with no spanwise length scale, its self-interaction does not induce further perturbations.
From the above analysis, we can express the perturbation field as
 \begin{align}
\breve{\boldsymbol\varphi}&=\sum\left[{\mathcal{E}}_s\,\tilde{\boldsymbol\varphi}_s(x,y)\exp({-{\rm
i}\omega_s t}) +{\mathcal{E}}_f\exp({{\rm i} k_3 z})\left(
\tilde{\boldsymbol\varphi}_f(x,y)\exp({-{\rm i}\omega_f
t})\right.\right.\nonumber\\ &\quad +\left.\left.\!\tilde{\boldsymbol\varphi}_+(x,y)\exp({-{\rm
i}(\omega_f+\omega_s)
t})+\tilde{\boldsymbol\varphi}_-(x,y)\exp({-{\rm
i}(\omega_f-\omega_s)
t})\right)+\cdots\right]\nonumber\\
&\quad +\text{c.c.}+\text{h.o.h.},
\end{align}
\begin{align}
\breve{\boldsymbol\varphi}&=\sum\left[{\mathcal{E}}_s\,\tilde{\boldsymbol\varphi}_s(x,y)\exp({-{\rm
i}\omega_s t}) +{\mathcal{E}}_f\exp({{\rm i} k_3 z})\left(
\tilde{\boldsymbol\varphi}_f(x,y)\exp({-{\rm i}\omega_f
t})\right.\right.\nonumber\\ &\quad +\left.\left.\!\tilde{\boldsymbol\varphi}_+(x,y)\exp({-{\rm
i}(\omega_f+\omega_s)
t})+\tilde{\boldsymbol\varphi}_-(x,y)\exp({-{\rm
i}(\omega_f-\omega_s)
t})\right)+\cdots\right]\nonumber\\
&\quad +\text{c.c.}+\text{h.o.h.},
\end{align}
where ![]() ${\mathcal {E}}_{s,f}\ll 1$ denotes the amplitude of each individual perturbation,
${\mathcal {E}}_{s,f}\ll 1$ denotes the amplitude of each individual perturbation, ![]() $\omega \in \mathbb {R}$ is the dimensionless frequency,
$\omega \in \mathbb {R}$ is the dimensionless frequency, ![]() $k_3 \in \mathbb {R}$ is the dimensionless spanwise wavenumber of the FSVD,
$k_3 \in \mathbb {R}$ is the dimensionless spanwise wavenumber of the FSVD, ![]() $\tilde {\boldsymbol \varphi } \in \mathbb {C}$ is the perturbation profile, and c.c. is the complex conjugate. Note that for simplicity, we consider only one spanwise wavenumber
$\tilde {\boldsymbol \varphi } \in \mathbb {C}$ is the perturbation profile, and c.c. is the complex conjugate. Note that for simplicity, we consider only one spanwise wavenumber ![]() $k_3$ in the HWNNS calculations. However, in the subsequent NPSE calculations, perturbations with both positive and negative spanwise wavenumbers
$k_3$ in the HWNNS calculations. However, in the subsequent NPSE calculations, perturbations with both positive and negative spanwise wavenumbers ![]() $\pm k_3$ are considered, as will be demonstrated in (4.1), which is consistent with the set-up of Ricco et al. (Reference Ricco, Luo and Wu2011) and Marensi et al. (Reference Marensi, Ricco and Wu2017). In the vicinity of the leading edge, because the base flow shows strong non-parallelism and the amplitudes of the high-order harmonics (h.o.h.) are negligibly small, we employ the harmonic linearised Navier–Stokes (HLNS) approach (Zhao, Dong & Yang Reference Zhao, Dong and Yang2019) to calculate
$\pm k_3$ are considered, as will be demonstrated in (4.1), which is consistent with the set-up of Ricco et al. (Reference Ricco, Luo and Wu2011) and Marensi et al. (Reference Marensi, Ricco and Wu2017). In the vicinity of the leading edge, because the base flow shows strong non-parallelism and the amplitudes of the high-order harmonics (h.o.h.) are negligibly small, we employ the harmonic linearised Navier–Stokes (HLNS) approach (Zhao, Dong & Yang Reference Zhao, Dong and Yang2019) to calculate ![]() $\tilde {\boldsymbol \varphi }_s$, and the HWNNS approach to calculate
$\tilde {\boldsymbol \varphi }_s$, and the HWNNS approach to calculate ![]() $\tilde {\boldsymbol \varphi }_f$ and
$\tilde {\boldsymbol \varphi }_f$ and ![]() $\tilde {\boldsymbol \varphi }_\pm$ (to be introduced in § 2.4.2); in the downstream region, where the base flow has reached the self-similarity state but the higher-order harmonics have reached the finite-amplitude state, the NPSE approach (Zhao et al. Reference Zhao, Zhang, Liu and Luo2016) is employed to calculate the mutual interaction of the Fourier modes.
$\tilde {\boldsymbol \varphi }_\pm$ (to be introduced in § 2.4.2); in the downstream region, where the base flow has reached the self-similarity state but the higher-order harmonics have reached the finite-amplitude state, the NPSE approach (Zhao et al. Reference Zhao, Zhang, Liu and Luo2016) is employed to calculate the mutual interaction of the Fourier modes.
2.3. Numerical approaches for the base-flow calculation
The 2-D base flow ![]() ${\boldsymbol {\varPhi }}_B(x,y)$ is governed by the steady N–S equations. In the numerical process, we select a rectangular box (
${\boldsymbol {\varPhi }}_B(x,y)$ is governed by the steady N–S equations. In the numerical process, we select a rectangular box (![]() $x \in [x_0,x_I]$,
$x \in [x_0,x_I]$, ![]() $y\in [0,y_J]$, with
$y\in [0,y_J]$, with ![]() $x_0=-0.426$,
$x_0=-0.426$, ![]() $x_I=300$ and
$x_I=300$ and ![]() $y_J=90$) as the computational domain, shown in figure 2, and discretise it into
$y_J=90$) as the computational domain, shown in figure 2, and discretise it into ![]() $(I+1)\times (J+1)$ grid points. Here, the inlet of the computational domain
$(I+1)\times (J+1)$ grid points. Here, the inlet of the computational domain ![]() $x_0$ is chosen to be slightly upstream of the plate leading edge for numerical convenience, and it has been confirmed that changing
$x_0$ is chosen to be slightly upstream of the plate leading edge for numerical convenience, and it has been confirmed that changing ![]() $x_0$ to another negative value does not change the numerical results. Each grid point is labelled by
$x_0$ to another negative value does not change the numerical results. Each grid point is labelled by ![]() $(x_i,y_j)$, with
$(x_i,y_j)$, with ![]() $i\in [0,I]$ and
$i\in [0,I]$ and ![]() $j\in [0,J]$ in the streamwise and wall-normal directions, respectively. Using the Lax–Friedrichs flux splitting method, the convective terms are discretised by the fifth-order weighted essentially non-oscillatory (WENO) scheme (Jiang & Shu Reference Jiang and Shu1996); the viscous terms are discretised by the fourth-order central finite difference scheme; the lower–upper symmetric Gauss–Seidel (LU-SGS) method is used for time advancing. The boundary conditions are set as follows. The no-slip, no-penetration and isothermal conditions
$j\in [0,J]$ in the streamwise and wall-normal directions, respectively. Using the Lax–Friedrichs flux splitting method, the convective terms are discretised by the fifth-order weighted essentially non-oscillatory (WENO) scheme (Jiang & Shu Reference Jiang and Shu1996); the viscous terms are discretised by the fourth-order central finite difference scheme; the lower–upper symmetric Gauss–Seidel (LU-SGS) method is used for time advancing. The boundary conditions are set as follows. The no-slip, no-penetration and isothermal conditions ![]() $(U_B, V_B, W_B, T_B)=(0,0,0,T_w)$ are imposed at the wall (
$(U_B, V_B, W_B, T_B)=(0,0,0,T_w)$ are imposed at the wall (![]() $x\in [0,x_I]$ and
$x\in [0,x_I]$ and ![]() $y=0$), where
$y=0$), where ![]() $T_w$ denotes the dimensionless wall temperature. The inflow condition (
$T_w$ denotes the dimensionless wall temperature. The inflow condition (![]() $x=x_0$,
$x=x_0$, ![]() $y\in [0,y_J]$), the centreline condition (
$y\in [0,y_J]$), the centreline condition (![]() $x\in (x_0,0)$,
$x\in (x_0,0)$, ![]() $y=0$) and the upper boundary condition (
$y=0$) and the upper boundary condition (![]() $x\in (x_0,x_I]$,
$x\in (x_0,x_I]$, ![]() $y=y_J$) are set to be the oncoming stream; the extrapolated boundary condition is employed at the outlet (
$y=y_J$) are set to be the oncoming stream; the extrapolated boundary condition is employed at the outlet (![]() $x=x_I$,
$x=x_I$, ![]() $y\in (0,y_J)$).
$y\in (0,y_J)$).

Figure 2. Sketch of the computational domain and the boundary conditions of the base-flow calculation (not to scale).
2.4. The HLNS and HWNNS approaches describing the perturbation evolution
2.4.1. The HLNS approach for calculating the boundary-layer response to lateral vibrations
As illustrated in § 2.2, the vibration-induced perturbation is governed by the linearised N–S equation system, which therefore can be solved by the HLNS approach. The excited perturbation field ![]() $\tilde {\boldsymbol \varphi }_s(x,y) \exp ({-{\rm i}\omega _s t})$, which is independent of
$\tilde {\boldsymbol \varphi }_s(x,y) \exp ({-{\rm i}\omega _s t})$, which is independent of ![]() $z$, satisfies the linear system
$z$, satisfies the linear system
where ![]() ${\mathcal {L}}$ represents the linearised N–S operator. The coefficient matrices
${\mathcal {L}}$ represents the linearised N–S operator. The coefficient matrices ![]() $\tilde {\boldsymbol {D}}$,
$\tilde {\boldsymbol {D}}$, ![]() $\tilde {\boldsymbol {A}}$,
$\tilde {\boldsymbol {A}}$, ![]() $\tilde {\boldsymbol {B}}$,
$\tilde {\boldsymbol {B}}$, ![]() ${\boldsymbol {V}}_{xx}$,
${\boldsymbol {V}}_{xx}$, ![]() ${\boldsymbol {V}}_{yy}$ and
${\boldsymbol {V}}_{yy}$ and ![]() ${\boldsymbol {V}}_{xy}$ can be found in the appendix of Zhao et al. (Reference Zhao, Dong and Yang2019). Such an approach that allows the time-domain problems to be solved quickly by converting them into Fourier series has also been applied in some previous works (Gopinath & Jameson Reference Gopinath and Jameson2005, Reference Gopinath and Jameson2006). The perturbations at all boundaries are set to be zero except at the wall, where
${\boldsymbol {V}}_{xy}$ can be found in the appendix of Zhao et al. (Reference Zhao, Dong and Yang2019). Such an approach that allows the time-domain problems to be solved quickly by converting them into Fourier series has also been applied in some previous works (Gopinath & Jameson Reference Gopinath and Jameson2005, Reference Gopinath and Jameson2006). The perturbations at all boundaries are set to be zero except at the wall, where ![]() $\tilde {w}_s=1$ is imposed. We introduce the unknown vector
$\tilde {w}_s=1$ is imposed. We introduce the unknown vector ![]() $\tilde {\boldsymbol {q}}\equiv (\tilde {\boldsymbol {\varphi }}_{f,0,0}, \tilde {\boldsymbol \varphi }_{f,0,1},\ldots, \tilde {\boldsymbol \varphi }_{f,0,J},\ldots, \tilde {\boldsymbol \varphi }_{f,I,0},\ldots, \tilde {\boldsymbol \varphi }_{f,I,J})$, where
$\tilde {\boldsymbol {q}}\equiv (\tilde {\boldsymbol {\varphi }}_{f,0,0}, \tilde {\boldsymbol \varphi }_{f,0,1},\ldots, \tilde {\boldsymbol \varphi }_{f,0,J},\ldots, \tilde {\boldsymbol \varphi }_{f,I,0},\ldots, \tilde {\boldsymbol \varphi }_{f,I,J})$, where ![]() $\tilde {\boldsymbol \varphi }_{f,i,j}$ denotes the value of
$\tilde {\boldsymbol \varphi }_{f,i,j}$ denotes the value of ![]() $\tilde {\boldsymbol \varphi }_{f}$ at
$\tilde {\boldsymbol \varphi }_{f}$ at ![]() $(x_i, y_j)$. Then
$(x_i, y_j)$. Then ![]() $\tilde {\boldsymbol {q}}$ is governed by a system of linear algebraic equations,
$\tilde {\boldsymbol {q}}$ is governed by a system of linear algebraic equations,
where the coefficient matrix ![]() $\boldsymbol {M}$ can be readily derived from (2.4), and
$\boldsymbol {M}$ can be readily derived from (2.4), and ![]() $\tilde {\boldsymbol {r}}$ denotes the inhomogeneous forcing from the wall. Here, the streamwise non-parallelism is retained, such that the rapid distortion of the perturbation around the leading edge can be calculated. The computational domain for the HLNS calculation is the same as that for the base-flow calculation; see domain (I) in figure 3. It needs to be noted that our numerical scheme for HLNS and the following HWNNS is not adequate to deal with the perturbations around a shock wave. However, in the present flat-plate model, the leading-edge oblique shock wave is rather weak, and its impact on the oncoming perturbations is quite limited. Therefore, our scheme works well on describing the perturbation evolution, as confirmed by comparing with the DNS results in Appendix B.
$\tilde {\boldsymbol {r}}$ denotes the inhomogeneous forcing from the wall. Here, the streamwise non-parallelism is retained, such that the rapid distortion of the perturbation around the leading edge can be calculated. The computational domain for the HLNS calculation is the same as that for the base-flow calculation; see domain (I) in figure 3. It needs to be noted that our numerical scheme for HLNS and the following HWNNS is not adequate to deal with the perturbations around a shock wave. However, in the present flat-plate model, the leading-edge oblique shock wave is rather weak, and its impact on the oncoming perturbations is quite limited. Therefore, our scheme works well on describing the perturbation evolution, as confirmed by comparing with the DNS results in Appendix B.

Figure 3. Sketch of the computational domains for the HLNS/HWNNS and NPSE calculations.
It is worth noting that the selection of the primitive variables ![]() $\tilde {\boldsymbol {\varphi }}$ as the unknowns in (2.4) and the following equation system (2.6) is not unique, and an alternative option is to choose the conserved variables. The latter approach is particularly advantageous when dealing with strong shock waves in the flow field. However, in the present problem, as the oblique shock wave forming from the leading edge is relatively weak, both approaches would yield identical results.
$\tilde {\boldsymbol {\varphi }}$ as the unknowns in (2.4) and the following equation system (2.6) is not unique, and an alternative option is to choose the conserved variables. The latter approach is particularly advantageous when dealing with strong shock waves in the flow field. However, in the present problem, as the oblique shock wave forming from the leading edge is relatively weak, both approaches would yield identical results.
2.4.2. The HWNNS approach for calculation of the excited perturbations  $\tilde {\boldsymbol {\varphi }}_f$ and
$\tilde {\boldsymbol {\varphi }}_f$ and  $\tilde {\boldsymbol {\varphi }}_\pm$
$\tilde {\boldsymbol {\varphi }}_\pm$
According to the scaling estimate in § 2.2, the excited perturbations ![]() $\tilde {\boldsymbol {\varphi }}_f$ and
$\tilde {\boldsymbol {\varphi }}_f$ and ![]() $\tilde {\boldsymbol {\varphi }}_\pm$ are governed by the inhomogeneous equations
$\tilde {\boldsymbol {\varphi }}_\pm$ are governed by the inhomogeneous equations
where ![]() $\boldsymbol {b}$ is a coefficient matrix appearing as inhomogeneous forcing of the linearised N–S operator. The generic form of
$\boldsymbol {b}$ is a coefficient matrix appearing as inhomogeneous forcing of the linearised N–S operator. The generic form of ![]() $\boldsymbol {b} {(\tilde {\boldsymbol \varphi }_1,\omega )\,\tilde {\boldsymbol \varphi }_2}=[b^{(1)}, b^{(2)}, b^{(3)}, b^{(4)}, b^{(5)}]^{{\rm T}}$ is expressed as
$\boldsymbol {b} {(\tilde {\boldsymbol \varphi }_1,\omega )\,\tilde {\boldsymbol \varphi }_2}=[b^{(1)}, b^{(2)}, b^{(3)}, b^{(4)}, b^{(5)}]^{{\rm T}}$ is expressed as
 \begin{equation} \left.\begin{gathered} b^{(1)}={-}{{\rm i}}k_3 \tilde{w}_1 \tilde{\rho}_2, \quad b^{(2)}={-}{{\rm i}}k_3 \rho_B \tilde{w}_1 \tilde{u}_2, \quad b^{(3)}={-}{{\rm i}}k_3 \rho_B \tilde{w}_1 \tilde{v}_2, \\ b^{(4)}={-}\rho_B (\tilde{w}_{1,x} \tilde{u}_2 +\tilde{w}_{1,y} \tilde{v}_2 +{{\rm i}}k_3 \tilde{w}_{1} \tilde{w}_2) - (U_B \tilde{w}_{1,x} + V_B \tilde{w}_{1,y} - {{\rm i}}\omega \tilde{w}_1)\tilde{\rho}_2,\\ b^{(5)}=\frac{-{{\rm i}}k_3 \tilde{w}_1}{\gamma} \left[\rho_B \tilde{T}_2 + (1-\gamma)T_B\tilde{\rho}_2\right]. \end{gathered}\right\} \end{equation}
\begin{equation} \left.\begin{gathered} b^{(1)}={-}{{\rm i}}k_3 \tilde{w}_1 \tilde{\rho}_2, \quad b^{(2)}={-}{{\rm i}}k_3 \rho_B \tilde{w}_1 \tilde{u}_2, \quad b^{(3)}={-}{{\rm i}}k_3 \rho_B \tilde{w}_1 \tilde{v}_2, \\ b^{(4)}={-}\rho_B (\tilde{w}_{1,x} \tilde{u}_2 +\tilde{w}_{1,y} \tilde{v}_2 +{{\rm i}}k_3 \tilde{w}_{1} \tilde{w}_2) - (U_B \tilde{w}_{1,x} + V_B \tilde{w}_{1,y} - {{\rm i}}\omega \tilde{w}_1)\tilde{\rho}_2,\\ b^{(5)}=\frac{-{{\rm i}}k_3 \tilde{w}_1}{\gamma} \left[\rho_B \tilde{T}_2 + (1-\gamma)T_B\tilde{\rho}_2\right]. \end{gathered}\right\} \end{equation}
It is easy to see that in the incompressible limit, if the high-order interactions are truncated, then (2.6) is equivalent to (2.14) of Hicks & Ricco (Reference Hicks and Ricco2015). The only difference is that in our system, the vibration-induced spanwise redistribution term ![]() $\boldsymbol {b}$ appears as an inhomogeneous forcing term on the right-hand side of each equation, while in Hicks & Ricco (Reference Hicks and Ricco2015), the Stokes layer induced by the lateral vibration is considered as a part of the base flow. The high-order interactions will be considered by the NPSE in § 2.5. Since
$\boldsymbol {b}$ appears as an inhomogeneous forcing term on the right-hand side of each equation, while in Hicks & Ricco (Reference Hicks and Ricco2015), the Stokes layer induced by the lateral vibration is considered as a part of the base flow. The high-order interactions will be considered by the NPSE in § 2.5. Since ![]() $\tilde {\varphi }_s$ is obtained beforehand, the system (2.6) is indeed a coupled linear system, but in the numerical process (as will be shown at the end of this subsection) we treat these terms as the first-order nonlinear forcing in each iterative step. The advantage of this treatment is that the linear operator
$\tilde {\varphi }_s$ is obtained beforehand, the system (2.6) is indeed a coupled linear system, but in the numerical process (as will be shown at the end of this subsection) we treat these terms as the first-order nonlinear forcing in each iterative step. The advantage of this treatment is that the linear operator ![]() $\mathcal {L}$ on the left-hand side of each subequation of (2.6) is the same as that in (2.4), rendering an easy extension of the HLNS framework. Because only the first-order nonlinear terms are included here, this approach is named the harmonic weakly nonlinear Navier–Stokes (HWNNS) approach. At first glance, the small quantity
$\mathcal {L}$ on the left-hand side of each subequation of (2.6) is the same as that in (2.4), rendering an easy extension of the HLNS framework. Because only the first-order nonlinear terms are included here, this approach is named the harmonic weakly nonlinear Navier–Stokes (HWNNS) approach. At first glance, the small quantity ![]() ${\mathcal {E}}_s$ appearing on the right-hand side of each equation in (2.6) is a little unsatisfactory. However, we would like to emphasise that the linear operator
${\mathcal {E}}_s$ appearing on the right-hand side of each equation in (2.6) is a little unsatisfactory. However, we would like to emphasise that the linear operator ![]() $\mathcal {L}$ is also small due to the low-frequency and long-wavelength nature (i.e.
$\mathcal {L}$ is also small due to the low-frequency and long-wavelength nature (i.e. ![]() ${\sim }\lambda _x^{-1}$), and the magnitudes of
${\sim }\lambda _x^{-1}$), and the magnitudes of ![]() ${\mathcal {E}}_s$ and
${\mathcal {E}}_s$ and ![]() $\mathcal {L}$ are taken to be comparable in this paper.
$\mathcal {L}$ are taken to be comparable in this paper.
For a still-wall case where ![]() ${{\mathcal {E}}}_s=0$, the perturbations
${{\mathcal {E}}}_s=0$, the perturbations ![]() $\tilde {\boldsymbol \varphi }_\pm$ are both zero, and (2.6a) is reduced to a homogeneous equation system, with the inhomogeneous forcing appearing only at the oncoming stream. An FSVD with frequency
$\tilde {\boldsymbol \varphi }_\pm$ are both zero, and (2.6a) is reduced to a homogeneous equation system, with the inhomogeneous forcing appearing only at the oncoming stream. An FSVD with frequency ![]() $\omega _f$ and the streamwise, wall-normal and spanwise wavenumbers
$\omega _f$ and the streamwise, wall-normal and spanwise wavenumbers ![]() $(k_1,k_2,k_3)$ is expressed as
$(k_1,k_2,k_3)$ is expressed as
where ![]() $\hat {\boldsymbol \varphi }_f\equiv (\hat {u}_f,\hat {v}_f,\hat {w}_f,0,0,0)$. Substituting (2.8) into the linearised N–S equations, we obtain the dispersion relation of the FSVD,
$\hat {\boldsymbol \varphi }_f\equiv (\hat {u}_f,\hat {v}_f,\hat {w}_f,0,0,0)$. Substituting (2.8) into the linearised N–S equations, we obtain the dispersion relation of the FSVD, ![]() $k_1=\omega _f+O(R^{-1/2})$ (Wu & Dong Reference Wu and Dong2016). Note that we are interested in the entrainment of the low-frequency perturbations, so
$k_1=\omega _f+O(R^{-1/2})$ (Wu & Dong Reference Wu and Dong2016). Note that we are interested in the entrainment of the low-frequency perturbations, so ![]() $k_1\ll k_2\sim k_3\sim 1$. Without loss of generality, we take the spanwise vorticity to be zero, i.e.
$k_1\ll k_2\sim k_3\sim 1$. Without loss of generality, we take the spanwise vorticity to be zero, i.e. ![]() $k_1 \hat {v}_f-k_2 \hat {u}_f=0$. Note that this is an arbitrary choice, but the excitation process of the boundary-layer perturbation for this condition is representative, as long as the magnitudes of the wavenumbers in the three directions are fixed. Combining with the continuity equation (
$k_1 \hat {v}_f-k_2 \hat {u}_f=0$. Note that this is an arbitrary choice, but the excitation process of the boundary-layer perturbation for this condition is representative, as long as the magnitudes of the wavenumbers in the three directions are fixed. Combining with the continuity equation (![]() $k_1 \hat {u}_f+k_2\hat {v}_f+k_3\hat {w}_f=0$), and taking the unit perturbation kinetic energy as the normalisation condition (
$k_1 \hat {u}_f+k_2\hat {v}_f+k_3\hat {w}_f=0$), and taking the unit perturbation kinetic energy as the normalisation condition (![]() $(\hat {u}_f^2+\hat {v}_f^2+\hat {w}_f^2)/2=1$), we obtain
$(\hat {u}_f^2+\hat {v}_f^2+\hat {w}_f^2)/2=1$), we obtain
 \begin{equation} \left(\hat{u}_f,\hat{v}_f,\hat{w}_f\right)=\sqrt{\frac{2}{(k_1^2+k_2^2)(k_1^2+k_2^2+k_3^2)}}\left({-}k_1k_3,-k_2k_3,k_1^2+k_2^2\right). \end{equation}
\begin{equation} \left(\hat{u}_f,\hat{v}_f,\hat{w}_f\right)=\sqrt{\frac{2}{(k_1^2+k_2^2)(k_1^2+k_2^2+k_3^2)}}\left({-}k_1k_3,-k_2k_3,k_1^2+k_2^2\right). \end{equation} For (2.6a), the boundary conditions at (![]() $x=x_0$,
$x=x_0$, ![]() $y\in [0,y_J]$), (
$y\in [0,y_J]$), (![]() $x\in (x_0,x_I)$,
$x\in (x_0,x_I)$, ![]() $y=y_J$) and (
$y=y_J$) and (![]() $x\in (x_0,0)$,
$x\in (x_0,0)$, ![]() $y=0$) are the FSVD
$y=0$) are the FSVD ![]() $\tilde {\boldsymbol \varphi }_f=\hat {\boldsymbol \varphi }_f \exp ({\textrm {i}(k_1x+k_2y)})$, appearing as an inhomogeneous forcing to the linear system; the extrapolated boundary condition is employed at the outlet
$\tilde {\boldsymbol \varphi }_f=\hat {\boldsymbol \varphi }_f \exp ({\textrm {i}(k_1x+k_2y)})$, appearing as an inhomogeneous forcing to the linear system; the extrapolated boundary condition is employed at the outlet ![]() $x=x_I$; the zero-perturbation conditions are imposed at the other boundaries.
$x=x_I$; the zero-perturbation conditions are imposed at the other boundaries.
Thus for a still wall, the excited perturbation ![]() $\tilde {\boldsymbol \varphi }_f$ is obtained by directly solving the HLNS equation system
$\tilde {\boldsymbol \varphi }_f$ is obtained by directly solving the HLNS equation system ![]() ${\mathcal {L}}(\omega _f)\, \tilde {\boldsymbol \varphi }_f(x,y)=0$, whereas for a spanwise vibrating wall, an iterative scheme is employed to deal with the coupled system (2.6).
${\mathcal {L}}(\omega _f)\, \tilde {\boldsymbol \varphi }_f(x,y)=0$, whereas for a spanwise vibrating wall, an iterative scheme is employed to deal with the coupled system (2.6).
(i) Set
 $\tilde {\varphi }_\pm =0$ at the beginning.
$\tilde {\varphi }_\pm =0$ at the beginning.(ii) Solve for
 $\tilde {\varphi }_f$ from (2.6a) subject to the freestream forcing.
$\tilde {\varphi }_f$ from (2.6a) subject to the freestream forcing.(iii) Calculate the inhomogeneous forcing of (2.6b,c) from the obtained
 $\tilde {\varphi }_f$, and solve for
$\tilde {\varphi }_f$, and solve for  $\tilde {\varphi }_\pm$.
$\tilde {\varphi }_\pm$.(iv) Calculate the inhomogeneous forcing of (2.6a), and go to step (ii) until the solution converges.
In steps (ii) (except for the first round of the iteration) and (iii), each governing equation has a harmonic linear operator on its left-hand side, and only the first-order nonlinear interaction terms are retained on its right-hand side, which is the reason for the name of the HWNNS approach.
2.5. The NPSE for the nonlinear evolution of the boundary-layer perturbations
In the HWNNS calculations illustrated in § 2.4, the high-order harmonics are truncated, which may be important in the downstream regions. Therefore, the NPSE approach (Chang & Malik Reference Chang and Malik1994; Zhao et al. Reference Zhao, Zhang, Liu and Luo2016) is employed in the downstream region; see domain (II) in figure 3. Such a treatment is acceptable for the following reasons: (1) in the vicinity of the leading edge, the perturbation deforms rapidly, but with a small amplitude, for which the HLNS/HWNNS approach is rather efficient; (2) in the downstream region, i.e. ![]() $x\geqslant X_0$, the perturbation evolves slowly in the
$x\geqslant X_0$, the perturbation evolves slowly in the ![]() $x$-direction, but with a sufficiently high amplitude for nonlinearity, for which the NPSE approach works well. Note that in § 2.4, the HLNS/HWNNS calculations were performed from
$x$-direction, but with a sufficiently high amplitude for nonlinearity, for which the NPSE approach works well. Note that in § 2.4, the HLNS/HWNNS calculations were performed from ![]() $x_0$ to
$x_0$ to ![]() $x_I$, but the flow field in
$x_I$, but the flow field in ![]() $x\in (X_0,x_I)$ is not needed physically. However, for code verification, we can compare this flow field with the NPSE and DNS calculations for a small
$x\in (X_0,x_I)$ is not needed physically. However, for code verification, we can compare this flow field with the NPSE and DNS calculations for a small ![]() ${{\mathcal {E}}}_f$, say
${{\mathcal {E}}}_f$, say ![]() ${{\mathcal {E}}}_f=10^{-8}$, as will be shown in figures 14 and 30.
${{\mathcal {E}}}_f=10^{-8}$, as will be shown in figures 14 and 30.
In Ricco et al. (Reference Ricco, Luo and Wu2011), Marensi et al. (Reference Marensi, Ricco and Wu2017), Xu et al. (Reference Xu, Zhang and Wu2017) and Xu, Liu & Wu (Reference Xu, Liu and Wu2020), the entrainment of FSVDs and the excitation of the boundary-layer streaks were formulated by the nonlinear boundary-region equation, which is able to deal with both the rapid distortion in the leading-edge vicinity and the nonlinearity in the downstream region. For the present physical model, our numerical approach, combining HLNS/HWNNS with the NPSE, in principle reproduces the nonlinear boundary-region calculations. However, our approach is easy to apply to more complex situations, such as a hypersonic boundary layer over a blunt body, for which the impacts of the bow-shaped shock wave and the entropy layer need to be considered. The present work can be regarded as the first step to solve more complex problems.
In the NPSE, the perturbation ![]() $\breve {\boldsymbol {\varphi }}(x,y,z,t)$ in (2.3) is expressed in terms of a truncated Fourier series,
$\breve {\boldsymbol {\varphi }}(x,y,z,t)$ in (2.3) is expressed in terms of a truncated Fourier series,
 \begin{gather} \breve{\boldsymbol\varphi}(x,y,z,t) = \sum_{m={-}\mathcal{M}}^\mathcal{M} \sum_{n={-}\mathcal{N}}^{\mathcal{N}} {{\mathcal{E}}}_{m,n}\,\tilde{\boldsymbol\varphi}_{m,n}(x,y) \exp\left({{\rm i} \left[\int_{X_0}^{x}\alpha _{m,n}(\bar{x} )\,{{\rm d}}\kern0.07em\bar{x} +n\beta _0z-m\omega _0t \right]}\right), \end{gather}
\begin{gather} \breve{\boldsymbol\varphi}(x,y,z,t) = \sum_{m={-}\mathcal{M}}^\mathcal{M} \sum_{n={-}\mathcal{N}}^{\mathcal{N}} {{\mathcal{E}}}_{m,n}\,\tilde{\boldsymbol\varphi}_{m,n}(x,y) \exp\left({{\rm i} \left[\int_{X_0}^{x}\alpha _{m,n}(\bar{x} )\,{{\rm d}}\kern0.07em\bar{x} +n\beta _0z-m\omega _0t \right]}\right), \end{gather}
where ![]() $\omega _0\in \mathbb {R}$ and
$\omega _0\in \mathbb {R}$ and ![]() $\beta _0\in \mathbb {R}$ are the fundamental frequency and spanwise wavenumber, respectively,
$\beta _0\in \mathbb {R}$ are the fundamental frequency and spanwise wavenumber, respectively, ![]() $m$ and
$m$ and ![]() $n$ denote the orders of
$n$ denote the orders of ![]() $\omega$ and
$\omega$ and ![]() $\beta$, respectively,
$\beta$, respectively, ![]() ${{\mathcal {E}}}_{m,n}\ll 1$ and
${{\mathcal {E}}}_{m,n}\ll 1$ and ![]() $\alpha _{m,n}\in \mathbb {C}$ represent the amplitude and complex wavenumber of the harmonic mode, respectively,
$\alpha _{m,n}\in \mathbb {C}$ represent the amplitude and complex wavenumber of the harmonic mode, respectively, ![]() $\tilde {\boldsymbol \varphi }_{m,n}\equiv (\tilde {u}_{m,n},\tilde {v}_{m,n},\tilde {w}_{m,n}, \tilde {\rho }_{m,n},\tilde {T}_{m,n},\tilde {p}_{m,n})$ is the complex shape function that varies slowly with
$\tilde {\boldsymbol \varphi }_{m,n}\equiv (\tilde {u}_{m,n},\tilde {v}_{m,n},\tilde {w}_{m,n}, \tilde {\rho }_{m,n},\tilde {T}_{m,n},\tilde {p}_{m,n})$ is the complex shape function that varies slowly with ![]() $x$,
$x$, ![]() $X_0=60$ represents the inlet of the NPSE domain, and
$X_0=60$ represents the inlet of the NPSE domain, and ![]() $\mathcal {M}$ and
$\mathcal {M}$ and ![]() $\mathcal {N}$ denote the limiting orders of the truncation for the frequency and spanwise wavenumber, respectively. Here,
$\mathcal {N}$ denote the limiting orders of the truncation for the frequency and spanwise wavenumber, respectively. Here, ![]() $\omega _0$ is the common divisor of
$\omega _0$ is the common divisor of ![]() $\omega _f$ and
$\omega _f$ and ![]() $\omega _s$ if both frequencies are non-zero. Otherwise,
$\omega _s$ if both frequencies are non-zero. Otherwise, ![]() $\omega _0$ should be selected as the non-zero value of
$\omega _0$ should be selected as the non-zero value of ![]() $\omega _f$ and
$\omega _f$ and ![]() $\omega _s$. The value
$\omega _s$. The value ![]() $\beta _0$ is chosen to be
$\beta _0$ is chosen to be ![]() $k_3$.
$k_3$.
Substituting (2.10) into the compressible N–S equations, subtracting out the base-flow terms, and neglecting the terms associated with ![]() $\partial _{xx}\tilde {\boldsymbol \varphi }_{m,n}$ and
$\partial _{xx}\tilde {\boldsymbol \varphi }_{m,n}$ and ![]() $\partial _{xy}\tilde {\boldsymbol \varphi }_{m,n}$, we obtain the NPSEs
$\partial _{xy}\tilde {\boldsymbol \varphi }_{m,n}$, we obtain the NPSEs
where the coefficient matrices ![]() $\tilde {\boldsymbol {A}}_{m,n}$,
$\tilde {\boldsymbol {A}}_{m,n}$, ![]() $\tilde {\boldsymbol {B}}_{m,n}$,
$\tilde {\boldsymbol {B}}_{m,n}$, ![]() $\tilde {\boldsymbol {D}}_{m,n}$,
$\tilde {\boldsymbol {D}}_{m,n}$, ![]() $\boldsymbol {V}_{yy}$ and the nonlinear term
$\boldsymbol {V}_{yy}$ and the nonlinear term ![]() $\tilde {\boldsymbol {F}}_{m,n}$ can be found in Zhao et al. (Reference Zhao, Zhang, Liu and Luo2016). Since the
$\tilde {\boldsymbol {F}}_{m,n}$ can be found in Zhao et al. (Reference Zhao, Zhang, Liu and Luo2016). Since the ![]() $\partial _{xx}$ terms are neglected, the elliptic N–S equations are parabolised, such that a marching scheme can be employed in the numerical process. The NPSE calculation begins at a position
$\partial _{xx}$ terms are neglected, the elliptic N–S equations are parabolised, such that a marching scheme can be employed in the numerical process. The NPSE calculation begins at a position ![]() $x=X_0$, as depicted in figure 3. This position is chosen to be downstream of the rapid distortion region at the leading edge, and upstream of the region where higher-order harmonics become significant. The inflow perturbation profiles and their amplitudes are obtained through the HLNS calculations. Denote the Fourier components of the FSVD
$x=X_0$, as depicted in figure 3. This position is chosen to be downstream of the rapid distortion region at the leading edge, and upstream of the region where higher-order harmonics become significant. The inflow perturbation profiles and their amplitudes are obtained through the HLNS calculations. Denote the Fourier components of the FSVD ![]() $\tilde {\boldsymbol \varphi }_f$ and vibration
$\tilde {\boldsymbol \varphi }_f$ and vibration ![]() $\tilde {\boldsymbol \varphi }_s$ by
$\tilde {\boldsymbol \varphi }_s$ by ![]() $(m_1,1)$ and
$(m_1,1)$ and ![]() $(m_2,0)$, respectively. The perturbations
$(m_2,0)$, respectively. The perturbations ![]() $\tilde {u}_{m,n}$,
$\tilde {u}_{m,n}$, ![]() $\tilde {v}_{m,n}$,
$\tilde {v}_{m,n}$, ![]() $\tilde {w}_{m,n}$ and
$\tilde {w}_{m,n}$ and ![]() $\tilde {T}_{m,n}$ are all set to be zero at the wall, except
$\tilde {T}_{m,n}$ are all set to be zero at the wall, except ![]() $\tilde {w}_{m_2,0}=1$. At the upper boundary, we set (
$\tilde {w}_{m_2,0}=1$. At the upper boundary, we set (![]() $\tilde {u}_{m_1,1},\tilde {v}_{m_1,1},\tilde {w}_{m_1,1})= \hat {\boldsymbol \varphi }_f\exp ({\textrm {i}(k_1x+k_2 y)})$ and
$\tilde {u}_{m_1,1},\tilde {v}_{m_1,1},\tilde {w}_{m_1,1})= \hat {\boldsymbol \varphi }_f\exp ({\textrm {i}(k_1x+k_2 y)})$ and ![]() $\partial _y\tilde {v}_{0,0}=0$, and the other quantities are all set to be zero. If
$\partial _y\tilde {v}_{0,0}=0$, and the other quantities are all set to be zero. If ![]() $\tilde {\boldsymbol {F}}_{m,n}$ is set to be zero, then the system (2.11) is reduced to the linear parabolised stability equation (LPSE), which can be used to trace the linear amplification of each Fourier component. The NPSE code has been applied in a few previous works (Zhao et al. Reference Zhao, Zhang, Liu and Luo2016; Song, Zhao & Dong Reference Song, Zhao and Dong2023a), which were confirmed to be sufficiently accurate in comparison with DNS.
$\tilde {\boldsymbol {F}}_{m,n}$ is set to be zero, then the system (2.11) is reduced to the linear parabolised stability equation (LPSE), which can be used to trace the linear amplification of each Fourier component. The NPSE code has been applied in a few previous works (Zhao et al. Reference Zhao, Zhang, Liu and Luo2016; Song, Zhao & Dong Reference Song, Zhao and Dong2023a), which were confirmed to be sufficiently accurate in comparison with DNS.
2.6. The SI analysis of streaks
The streaks themselves do not lead to transition; however, they could support rapid growths of high-frequency perturbations via the SI regime. Since the length scale of the SI modes is much shorter than that of the streaky base flow, we can introduce the parallel-flow assumption for the SI analysis. The streaky base flow is expressed as ![]() $\breve {\boldsymbol {\varPhi }}_B(y,z;\breve {x},\breve {t})\equiv [\breve {U}_B,0,0,\breve {\rho }_B,\breve {T}_B,\breve {P}_B]$, which is a superposition of the 2-D base flow and the NPSE solutions at a streamwise position
$\breve {\boldsymbol {\varPhi }}_B(y,z;\breve {x},\breve {t})\equiv [\breve {U}_B,0,0,\breve {\rho }_B,\breve {T}_B,\breve {P}_B]$, which is a superposition of the 2-D base flow and the NPSE solutions at a streamwise position ![]() $\breve {x}$ and a time instant
$\breve {x}$ and a time instant ![]() $\breve {t}$:
$\breve {t}$:
 \begin{align}
\breve{\boldsymbol{\varPhi}}_B(y,z;\breve{x},\breve{t}) &=
{\boldsymbol{\varPhi}}_B(y;\breve{x})\nonumber\\ &\quad
+\sum_{m={-}\mathcal{ M}}^\mathcal{ M}
\sum_{n={-}\mathcal{N}}^\mathcal{N} {{\mathcal{E}}}_{m,n}\,
\tilde{\boldsymbol{\varphi}}_{m,n}(y;\breve{x})\nonumber\\
&\quad \times \exp({{\rm
i}(n k_3 z-m\omega_0 \breve{t})}) \exp\left({{\rm i}
\int_{X_0}^{\breve{x}} \alpha_{m,n}(\bar{x})\,{{\rm
d}}\kern0.07em\bar{x}}\right),
\end{align}
\begin{align}
\breve{\boldsymbol{\varPhi}}_B(y,z;\breve{x},\breve{t}) &=
{\boldsymbol{\varPhi}}_B(y;\breve{x})\nonumber\\ &\quad
+\sum_{m={-}\mathcal{ M}}^\mathcal{ M}
\sum_{n={-}\mathcal{N}}^\mathcal{N} {{\mathcal{E}}}_{m,n}\,
\tilde{\boldsymbol{\varphi}}_{m,n}(y;\breve{x})\nonumber\\
&\quad \times \exp({{\rm
i}(n k_3 z-m\omega_0 \breve{t})}) \exp\left({{\rm i}
\int_{X_0}^{\breve{x}} \alpha_{m,n}(\bar{x})\,{{\rm
d}}\kern0.07em\bar{x}}\right),
\end{align}
where ![]() $\mathcal {M}$,
$\mathcal {M}$, ![]() $\mathcal {N}$,
$\mathcal {N}$, ![]() ${{\mathcal {E}}}$,
${{\mathcal {E}}}$, ![]() $\tilde {\boldsymbol {\varphi }}$ and
$\tilde {\boldsymbol {\varphi }}$ and ![]() $\omega _0$ were defined in (2.10).
$\omega _0$ were defined in (2.10).
For simplicity, we perform the temporal-mode SI analysis, and the perturbations ![]() $\breve {\boldsymbol {\varphi }}_{SI}(x,y,z,t)\equiv (\breve {u}_{SI}, \breve {v}_{SI}, \breve {w}_{SI}, \breve {\rho }_{SI},\breve {T}_{SI},\breve {p}_{SI})$ are expressed as
$\breve {\boldsymbol {\varphi }}_{SI}(x,y,z,t)\equiv (\breve {u}_{SI}, \breve {v}_{SI}, \breve {w}_{SI}, \breve {\rho }_{SI},\breve {T}_{SI},\breve {p}_{SI})$ are expressed as
 \begin{equation} \left.\begin{gathered} \breve{\boldsymbol\varphi}_{SI}(x,y,z,t)={{\mathcal{E}}}_{SI}\,\tilde{\boldsymbol{\varphi}}_{SI}(y,z) \exp({{\rm i}(\alpha x-\omega t)}) \exp({{\rm i} \sigma_d k_3 z})+\text{c.c.},\\ {}\tilde{\boldsymbol\varphi}_{SI}(y,z)=\sum_{n={-}\mathcal{ N}_{SI}}^{\mathcal{ N}_{SI}} \hat{\boldsymbol\varphi}_{SI,n}(y) \exp({{\rm i} n k_3 z}), \end{gathered}\right\} \end{equation}
\begin{equation} \left.\begin{gathered} \breve{\boldsymbol\varphi}_{SI}(x,y,z,t)={{\mathcal{E}}}_{SI}\,\tilde{\boldsymbol{\varphi}}_{SI}(y,z) \exp({{\rm i}(\alpha x-\omega t)}) \exp({{\rm i} \sigma_d k_3 z})+\text{c.c.},\\ {}\tilde{\boldsymbol\varphi}_{SI}(y,z)=\sum_{n={-}\mathcal{ N}_{SI}}^{\mathcal{ N}_{SI}} \hat{\boldsymbol\varphi}_{SI,n}(y) \exp({{\rm i} n k_3 z}), \end{gathered}\right\} \end{equation}
where ![]() $\alpha \in \mathbb {R}$ and
$\alpha \in \mathbb {R}$ and ![]() $\omega =\omega _r+\textrm {i}\omega _i \in \mathbb {C}$ are the streamwise wavenumber and complex frequency of the SI mode, respectively, with
$\omega =\omega _r+\textrm {i}\omega _i \in \mathbb {C}$ are the streamwise wavenumber and complex frequency of the SI mode, respectively, with ![]() $\omega _i$ denoting its temporal growth rate. Here,
$\omega _i$ denoting its temporal growth rate. Here, ![]() ${{\mathcal {E}}}_{SI}$ is the amplitude, and
${{\mathcal {E}}}_{SI}$ is the amplitude, and ![]() $\mathcal { N}_{SI}$ is the truncation order of the Fourier series. In this paper, we set
$\mathcal { N}_{SI}$ is the truncation order of the Fourier series. In this paper, we set ![]() $\mathcal { N}_{SI}=8$. Also,
$\mathcal { N}_{SI}=8$. Also, ![]() $\sigma _d\in [0, 0.5]$ is a detuning parameter, which is zero for a fundamental mode, 0.5 for a subharmonic mode and another value for the detuned mode, and all of them could be unstable with comparable growth rates; see Ren & Fu (Reference Ren and Fu2015). As a representative study, we focus only on the fundamental mode in this paper. Substituting (2.12) and (2.13) into the linearised N–S equations, we arrive at a linear equation system,
$\sigma _d\in [0, 0.5]$ is a detuning parameter, which is zero for a fundamental mode, 0.5 for a subharmonic mode and another value for the detuned mode, and all of them could be unstable with comparable growth rates; see Ren & Fu (Reference Ren and Fu2015). As a representative study, we focus only on the fundamental mode in this paper. Substituting (2.12) and (2.13) into the linearised N–S equations, we arrive at a linear equation system,
The coefficient matrices ![]() $\boldsymbol {\tilde {D}}_{SI}$,
$\boldsymbol {\tilde {D}}_{SI}$, ![]() $\boldsymbol {\tilde {B}}_{SI}$ and
$\boldsymbol {\tilde {B}}_{SI}$ and ![]() $\boldsymbol {\tilde {C}}_{SI}$ are given by
$\boldsymbol {\tilde {C}}_{SI}$ are given by
where the coefficient matrices ![]() $\boldsymbol {D}$,
$\boldsymbol {D}$, ![]() $\boldsymbol {\varGamma }$,
$\boldsymbol {\varGamma }$, ![]() $\boldsymbol {A}$,
$\boldsymbol {A}$, ![]() $\boldsymbol {B}$,
$\boldsymbol {B}$, ![]() $\boldsymbol {C}$,
$\boldsymbol {C}$, ![]() ${\boldsymbol {V}}_{yy}$,
${\boldsymbol {V}}_{yy}$, ![]() ${\boldsymbol {V}}_{zz}$,
${\boldsymbol {V}}_{zz}$, ![]() $\boldsymbol {V}_{yz}$,
$\boldsymbol {V}_{yz}$, ![]() ${\boldsymbol {V}}_{xx}$,
${\boldsymbol {V}}_{xx}$, ![]() ${\boldsymbol {V}}_{xy}$ and
${\boldsymbol {V}}_{xy}$ and ![]() ${\boldsymbol {V}}_{xz}$ depend on
${\boldsymbol {V}}_{xz}$ depend on ![]() $\breve {\boldsymbol {\varPhi }}_B$ and can be found in the appendix of Zhao et al. (Reference Zhao, Dong and Yang2019). The system (2.14) with the homogeneous boundary conditions forms a generalised eigenvalue system,
$\breve {\boldsymbol {\varPhi }}_B$ and can be found in the appendix of Zhao et al. (Reference Zhao, Dong and Yang2019). The system (2.14) with the homogeneous boundary conditions forms a generalised eigenvalue system,
with ![]() $\omega$ being the eigenvalue, and the coefficient matrices
$\omega$ being the eigenvalue, and the coefficient matrices ![]() $\mathcal {A}$ and
$\mathcal {A}$ and ![]() $\mathcal {B}$ are readily derived from (2.14). The Arnoldi iterative method is employed to search the eigenvalues initially, and the Rayleigh quotient iteration method is used to confirm its accuracy, as has been used in Han et al. (Reference Han, Yuan, Dong and Fan2021) and Song, Dong & Zhao (Reference Song, Dong and Zhao2023b).
$\mathcal {B}$ are readily derived from (2.14). The Arnoldi iterative method is employed to search the eigenvalues initially, and the Rayleigh quotient iteration method is used to confirm its accuracy, as has been used in Han et al. (Reference Han, Yuan, Dong and Fan2021) and Song, Dong & Zhao (Reference Song, Dong and Zhao2023b).
3. Numerical results of the base flow and the linear evolution of the perturbations
3.1. Base flow
The controlling parameters selected in this paper are listed in table 1. The base-flow calculation is based on the 2-D domain ![]() $x\in [-0.426,300]$ and
$x\in [-0.426,300]$ and ![]() $y\in [0,90]$. We employ 1201 grid points in the
$y\in [0,90]$. We employ 1201 grid points in the ![]() $x$-direction, with 515 grid points clustered near the leading edge (
$x$-direction, with 515 grid points clustered near the leading edge (![]() $x\leqslant 60$), and 301 grid points clustered near the wall are employed in the
$x\leqslant 60$), and 301 grid points clustered near the wall are employed in the ![]() $y$-direction. Figure 4(a) shows the contours of the pressure
$y$-direction. Figure 4(a) shows the contours of the pressure ![]() $P_B$, where the yellow line forming from the leading edge with an oblique angle
$P_B$, where the yellow line forming from the leading edge with an oblique angle ![]() $9.54^{\circ }$, approximately equal to
$9.54^{\circ }$, approximately equal to ![]() $\tan ^{-1}(1/M)$, denotes the oblique attached shock wave. Figures 4(b) and 4(c) compare the
$\tan ^{-1}(1/M)$, denotes the oblique attached shock wave. Figures 4(b) and 4(c) compare the ![]() $U_B$ and
$U_B$ and ![]() $T_B$ profiles with the compressible Blasius solutions at different streamwise positions. The agreement between the two families of curves confirms the accuracy of our base-flow calculations.
$T_B$ profiles with the compressible Blasius solutions at different streamwise positions. The agreement between the two families of curves confirms the accuracy of our base-flow calculations.
Table 1. Parameters for case studies.

Figure 4. Base flow. (a) Contours of the mean pressure ![]() $P_B$. (b,c) Comparisons of the streamwise velocity
$P_B$. (b,c) Comparisons of the streamwise velocity ![]() $U_B$ and temperature
$U_B$ and temperature ![]() $T_B$ profiles with the compressible Blasius solutions at
$T_B$ profiles with the compressible Blasius solutions at ![]() $x=15$, 30, 60, 120 and 240.
$x=15$, 30, 60, 120 and 240.
3.2. Instability property
Although we are not primarily focusing on the excitation and evolution of normal modes in this paper, they could exist in a downstream position, where the local Reynolds number is relatively large. For supersonic boundary layers with ![]() $M>4$, there exist multiple unstable modes, and the second mode is usually more unstable (Mack Reference Mack, Dwoyer and Hussaini1987). In this paper, we are interested in the 3-D low-frequency perturbations, and the neutral curves of the 3-D Mack first modes for
$M>4$, there exist multiple unstable modes, and the second mode is usually more unstable (Mack Reference Mack, Dwoyer and Hussaini1987). In this paper, we are interested in the 3-D low-frequency perturbations, and the neutral curves of the 3-D Mack first modes for ![]() $\beta =0.33$, 1, 2 and 2.67 in the
$\beta =0.33$, 1, 2 and 2.67 in the ![]() $x{-}\omega$ plane are plotted in figure 5(a). For
$x{-}\omega$ plane are plotted in figure 5(a). For ![]() $\omega <0.2$ and
$\omega <0.2$ and ![]() $\beta \leqslant 1$, the frequency of the neutral first mode decreases with increase of
$\beta \leqslant 1$, the frequency of the neutral first mode decreases with increase of ![]() $x$, indicating an enlargement of the unstable zone in the low-frequency band. For higher
$x$, indicating an enlargement of the unstable zone in the low-frequency band. For higher ![]() $\beta$ values, the unstable zone shrinks, and no unstable first mode is observed for
$\beta$ values, the unstable zone shrinks, and no unstable first mode is observed for ![]() $\omega \leqslant 0.05$. In figure 5(b), the neutral curve in the
$\omega \leqslant 0.05$. In figure 5(b), the neutral curve in the ![]() $\omega{-}x$ plane for
$\omega{-}x$ plane for ![]() $\beta =1$ in a wider frequency band is plotted, and the Mack second mode and the first mode of the upper branch, appearing when
$\beta =1$ in a wider frequency band is plotted, and the Mack second mode and the first mode of the upper branch, appearing when ![]() $\omega =O(1)$, are excluded in the discussion of this paper.
$\omega =O(1)$, are excluded in the discussion of this paper.

Figure 5. (a) Neutral curves of the 3-D Mack modes for ![]() $\beta = 0.33$, 1, 2 and 2.67. (b) Neutral curves of the first and second modes in a wider frequency band for
$\beta = 0.33$, 1, 2 and 2.67. (b) Neutral curves of the first and second modes in a wider frequency band for ![]() $\beta = 1$.
$\beta = 1$.
3.3. Linear response of a still boundary layer to FSVDs
In this subsection, we consider the results for still walls. For the HLNS calculations, the same computational domain as in § 3.1 is used, but the number of grid points in the ![]() $x$-direction is reduced to 401. To characterise the ratio of
$x$-direction is reduced to 401. To characterise the ratio of ![]() $k_2$ to
$k_2$ to ![]() $k_3$, we introduce an oblique wave angle
$k_3$, we introduce an oblique wave angle ![]() $\varTheta \equiv \tan ^{-1}(k_2/k_3)$. Choosing
$\varTheta \equiv \tan ^{-1}(k_2/k_3)$. Choosing ![]() $k_2=k_3=1$ (
$k_2=k_3=1$ (![]() $\varTheta =45^\circ$), we plot the contours of the perturbation field
$\varTheta =45^\circ$), we plot the contours of the perturbation field ![]() $\tilde {\boldsymbol \varphi }_f$ in figure 6. For
$\tilde {\boldsymbol \varphi }_f$ in figure 6. For ![]() $\omega _f=0$, the perturbations
$\omega _f=0$, the perturbations ![]() $\tilde {v}_f$ and
$\tilde {v}_f$ and ![]() $\tilde {w}_f$ show long streamwise length scales in the external stream, and a mild kink appears at the oblique shock wave. In the boundary layer,
$\tilde {w}_f$ show long streamwise length scales in the external stream, and a mild kink appears at the oblique shock wave. In the boundary layer, ![]() $\tilde v_f$ and
$\tilde v_f$ and ![]() $\tilde {w}_f$ are rather small, but the perturbations
$\tilde {w}_f$ are rather small, but the perturbations ![]() $\tilde {u}_f$ and
$\tilde {u}_f$ and ![]() $\tilde {T}_f$ undergo drastic amplifications, agreeing with Leib et al. (Reference Leib, Wundrow and Goldstein1999) and Ricco & Wu (Reference Ricco and Wu2007). For an FSVD with
$\tilde {T}_f$ undergo drastic amplifications, agreeing with Leib et al. (Reference Leib, Wundrow and Goldstein1999) and Ricco & Wu (Reference Ricco and Wu2007). For an FSVD with ![]() $\omega _f=0.17$, the overall behaviour of the perturbation field remains the same, but a finite streamwise length scale is observed due to the finite wavelength of the FSVD.
$\omega _f=0.17$, the overall behaviour of the perturbation field remains the same, but a finite streamwise length scale is observed due to the finite wavelength of the FSVD.

Figure 6. Contours of the real parts of ![]() $\tilde {u}_f$,
$\tilde {u}_f$, ![]() $\tilde {v}_f$,
$\tilde {v}_f$, ![]() $\tilde {w}_f$ and
$\tilde {w}_f$ and ![]() $\tilde {T}_f$ excited by the low-frequency FSVDs with
$\tilde {T}_f$ excited by the low-frequency FSVDs with ![]() $k_2=k_3=1$, for (a,c,e,g)
$k_2=k_3=1$, for (a,c,e,g) ![]() $\omega _f=0$, (b,d, f,h)
$\omega _f=0$, (b,d, f,h) ![]() $\omega _f=0.17$. The dashed lines mark the nominal boundary-layer thickness.
$\omega _f=0.17$. The dashed lines mark the nominal boundary-layer thickness.
Figure 7(a) plots the streamwise evolution of the amplitude of the streamwise velocity perturbation ![]() $\tilde {U}_f(x)=\max _y(|\tilde {u}_f(x,y)|)$ for five representative frequencies (
$\tilde {U}_f(x)=\max _y(|\tilde {u}_f(x,y)|)$ for five representative frequencies (![]() $\omega _f=0$, 0.05, 0.1, 0.17 and 0.33). Each curve shows a drastic increase in the vicinity of the leading edge, i.e.
$\omega _f=0$, 0.05, 0.1, 0.17 and 0.33). Each curve shows a drastic increase in the vicinity of the leading edge, i.e. ![]() $x<15$, indicating a rapid adjustment of the perturbation in a region where the non-parallelism of the mean flow is strong; after a mild decay, it grows until the end of the calculation. Overall, the amplitude
$x<15$, indicating a rapid adjustment of the perturbation in a region where the non-parallelism of the mean flow is strong; after a mild decay, it grows until the end of the calculation. Overall, the amplitude ![]() $\tilde {U}_f$ increases as the frequency increases. The temperature perturbation
$\tilde {U}_f$ increases as the frequency increases. The temperature perturbation ![]() $\tilde {T}_f=\max _y(|\tilde {T}_f(x,y)|)$ is one order of magnitude greater than that of
$\tilde {T}_f=\max _y(|\tilde {T}_f(x,y)|)$ is one order of magnitude greater than that of ![]() $\tilde {U}_f$, agreeing with the solutions given by the boundary-region equations in Ricco & Wu (Reference Ricco and Wu2007). In the downstream region, the non-stationary perturbations would enter the unstable zone of the Mack first mode, and a comparison with the predictions by linear stability theory (LST), shown by the open symbols, and LPSE, shown by the filled symbols, is provided. The disparity between the LST and LPSE calculations arises from the consideration of non-parallelism in the base flow, which is neglected in the former but retained in the latter. The overall agreement between the HLNS calculations and the LST/LPSE predictions is evident in the region approximately downstream of the neutral points of the Mack first mode, shown by the vertical lines. A clearer observation of each curve is provided by plotting the streamwise evolution of the growth rates (
$\tilde {U}_f$, agreeing with the solutions given by the boundary-region equations in Ricco & Wu (Reference Ricco and Wu2007). In the downstream region, the non-stationary perturbations would enter the unstable zone of the Mack first mode, and a comparison with the predictions by linear stability theory (LST), shown by the open symbols, and LPSE, shown by the filled symbols, is provided. The disparity between the LST and LPSE calculations arises from the consideration of non-parallelism in the base flow, which is neglected in the former but retained in the latter. The overall agreement between the HLNS calculations and the LST/LPSE predictions is evident in the region approximately downstream of the neutral points of the Mack first mode, shown by the vertical lines. A clearer observation of each curve is provided by plotting the streamwise evolution of the growth rates (![]() $\tilde {U}_f^{-1}\,\textrm {d}\tilde {U}_f/\textrm {d}\kern0.7pt x$ for HLNS and LPSE, and
$\tilde {U}_f^{-1}\,\textrm {d}\tilde {U}_f/\textrm {d}\kern0.7pt x$ for HLNS and LPSE, and ![]() $-\alpha _i$ for LST) in figure 7(c). Again, the HLNS calculations agree well with the LPSE predictions for
$-\alpha _i$ for LST) in figure 7(c). Again, the HLNS calculations agree well with the LPSE predictions for ![]() $\omega _f\geqslant 0.10$, and the LST curves underpredict the growth rate due to the neglect of the non-parallelism. Because the growth rate for
$\omega _f\geqslant 0.10$, and the LST curves underpredict the growth rate due to the neglect of the non-parallelism. Because the growth rate for ![]() $\omega _f=0.05$ is quite low, the agreement between the HLNS and LPSE calculations is expected in further downstream regions. In the region upstream of the neutral point, all the normal modes are damped, but their non-orthogonal nature determines that they could show an algebraic growth, referring to as the non-modal growth. The non-modal growth rate in the leading-edge vicinity oscillates remarkably, indicating the rapid distortion of the perturbation due to the strong non-parallelism. The transition from non-modal perturbation to normal instability mode was also reported by Ricco & Wu (Reference Ricco and Wu2007). Because the Mack mode is unstable only in travelling wave forms, the boundary-layer response to the FSVD with
$\omega _f=0.05$ is quite low, the agreement between the HLNS and LPSE calculations is expected in further downstream regions. In the region upstream of the neutral point, all the normal modes are damped, but their non-orthogonal nature determines that they could show an algebraic growth, referring to as the non-modal growth. The non-modal growth rate in the leading-edge vicinity oscillates remarkably, indicating the rapid distortion of the perturbation due to the strong non-parallelism. The transition from non-modal perturbation to normal instability mode was also reported by Ricco & Wu (Reference Ricco and Wu2007). Because the Mack mode is unstable only in travelling wave forms, the boundary-layer response to the FSVD with ![]() $\omega _f=0$ is always non-modal.
$\omega _f=0$ is always non-modal.

Figure 7. Evolution of (a) ![]() $\tilde {U}_f$, (b)
$\tilde {U}_f$, (b) ![]() $\tilde {T}_f$, and (c) the growth rate
$\tilde {T}_f$, and (c) the growth rate ![]() $-\alpha _i$, for
$-\alpha _i$, for ![]() $k_2=k_3=1$. The vertical lines denote the intersection positions of the non-modal and modal perturbations.
$k_2=k_3=1$. The vertical lines denote the intersection positions of the non-modal and modal perturbations.
Figures 8(a,c,e,g) display the perturbation profiles with ![]() $\omega _f=0$. As
$\omega _f=0$. As ![]() $x$ approaches downstream, the amplitudes of
$x$ approaches downstream, the amplitudes of ![]() $|\tilde {u}_f|$ and
$|\tilde {u}_f|$ and ![]() $|\tilde {T}_f|$ exhibit significant amplification, while those of
$|\tilde {T}_f|$ exhibit significant amplification, while those of ![]() $|\tilde {v}_f|$ and
$|\tilde {v}_f|$ and ![]() $|\tilde {w}_f|$ remain relatively unchanged. This behaviour is a characteristic feature of non-modal streaks, where the amplification of
$|\tilde {w}_f|$ remain relatively unchanged. This behaviour is a characteristic feature of non-modal streaks, where the amplification of ![]() $\tilde {u}_f$ and
$\tilde {u}_f$ and ![]() $\tilde {T}_f$ is attributed to the lift-up mechanism. Notably, the peak of the temperature profile is closer to the boundary-layer edge compared to the peak of the
$\tilde {T}_f$ is attributed to the lift-up mechanism. Notably, the peak of the temperature profile is closer to the boundary-layer edge compared to the peak of the ![]() $\tilde {u}_f$ profile. In contrast, for
$\tilde {u}_f$ profile. In contrast, for ![]() $\omega _f=0.17$, as depicted in figures 8(b,d, f,h), the amplitudes of
$\omega _f=0.17$, as depicted in figures 8(b,d, f,h), the amplitudes of ![]() $|\tilde {u}_f|$,
$|\tilde {u}_f|$, ![]() $|\tilde {v}_f|$,
$|\tilde {v}_f|$, ![]() $|\tilde {w}_f|$ and
$|\tilde {w}_f|$ and ![]() $|\tilde {T}_f|$ grow progressively as they propagate downstream. These profiles align with the linear prediction of the Mack first mode obtained by LST, thus confirming the modal nature of the perturbations for
$|\tilde {T}_f|$ grow progressively as they propagate downstream. These profiles align with the linear prediction of the Mack first mode obtained by LST, thus confirming the modal nature of the perturbations for ![]() $\omega =0.17$ and
$\omega =0.17$ and ![]() $x\geqslant 120$.
$x\geqslant 120$.

Figure 8. Wall-normal profiles of ![]() $|\tilde {u}_f|$,
$|\tilde {u}_f|$, ![]() $|\tilde {v}_f|$,
$|\tilde {v}_f|$, ![]() $|\tilde {w}_f|$ and
$|\tilde {w}_f|$ and ![]() $|\tilde {T}_f|$ at
$|\tilde {T}_f|$ at ![]() $x=120$, 180, 240 and 300, where
$x=120$, 180, 240 and 300, where ![]() $k_2=k_3=1$, for (a,c,e,g)
$k_2=k_3=1$, for (a,c,e,g) ![]() $\omega _f=0$, (b,d, f,h)
$\omega _f=0$, (b,d, f,h) ![]() $\omega _f=0.17$. The results are normalised by the maximum of
$\omega _f=0.17$. The results are normalised by the maximum of ![]() $|\tilde {u}_f|$ at
$|\tilde {u}_f|$ at ![]() $x=300$. The horizontal dashed lines denote the nominal boundary-layer thickness
$x=300$. The horizontal dashed lines denote the nominal boundary-layer thickness ![]() $\delta _{99}$.
$\delta _{99}$.
Figure 9(a) summarises the variation of the perturbation amplitude ![]() $\tilde {U}_f$ with
$\tilde {U}_f$ with ![]() $\omega _f$ at different streamwise locations for
$\omega _f$ at different streamwise locations for ![]() $k_3=0.33$ and
$k_3=0.33$ and ![]() $\varTheta =45^\circ$. At
$\varTheta =45^\circ$. At ![]() $x=30$ and 60,
$x=30$ and 60, ![]() $\tilde {U}_f$ decreases monotonically with increase of
$\tilde {U}_f$ decreases monotonically with increase of ![]() $\omega _f$, and the amplitude is greater in a downstream position. As
$\omega _f$, and the amplitude is greater in a downstream position. As ![]() $x$ increases to 120, the amplitude shows a local peak at around
$x$ increases to 120, the amplitude shows a local peak at around ![]() $\omega _f=0.02$, and decreases with
$\omega _f=0.02$, and decreases with ![]() $\omega _f$ for higher frequencies. When
$\omega _f$ for higher frequencies. When ![]() $x=240$, the amplitude shows a local minimum (marked by a circle) at around
$x=240$, the amplitude shows a local minimum (marked by a circle) at around ![]() $\omega _f=0.03$, and for higher
$\omega _f=0.03$, and for higher ![]() $\omega _f$ values, the curve shows the same feature as that for
$\omega _f$ values, the curve shows the same feature as that for ![]() $x=120$. Comparing the
$x=120$. Comparing the ![]() $\tilde {U}_f$ curves with the LST predictions, we find that the local minimum point can be viewed as a critical frequency distinguishing the normal and non-modal growth of each perturbation at one location. Changing the wavenumbers
$\tilde {U}_f$ curves with the LST predictions, we find that the local minimum point can be viewed as a critical frequency distinguishing the normal and non-modal growth of each perturbation at one location. Changing the wavenumbers ![]() $k_3$ and keeping
$k_3$ and keeping ![]() $\varTheta =45^\circ$, we plot
$\varTheta =45^\circ$, we plot ![]() $\tilde {U}_f$ in figures 9(b), 9(c) and 9(d), and similar trend is observed. For the same
$\tilde {U}_f$ in figures 9(b), 9(c) and 9(d), and similar trend is observed. For the same ![]() $k_3$, the transition from non-modal to modal perturbations appears earlier as
$k_3$, the transition from non-modal to modal perturbations appears earlier as ![]() $\omega _f$ increases, and at the same position, the critical frequency increases with
$\omega _f$ increases, and at the same position, the critical frequency increases with ![]() $k_3$.
$k_3$.

Figure 9. Amplitudes of the excited perturbations with ![]() $\varTheta =45^\circ$ at different locations, where the circles mark the critical frequency distinguishing the non-modal and modal perturbations, for (a)
$\varTheta =45^\circ$ at different locations, where the circles mark the critical frequency distinguishing the non-modal and modal perturbations, for (a) ![]() $k_3=0.33$, (b)
$k_3=0.33$, (b) ![]() $k_3=1$, (c)
$k_3=1$, (c) ![]() $k_3=2$, (d)
$k_3=2$, (d) ![]() $k_3=2.67$.
$k_3=2.67$.
3.4. Linear response of a boundary layer to a spanwise vibration
For a weak wall vibration without any FSVD, the boundary-layer response ![]() $\tilde {\boldsymbol {\varphi }}_s$ is obtained by solving (2.4) numerically. Only
$\tilde {\boldsymbol {\varphi }}_s$ is obtained by solving (2.4) numerically. Only ![]() $\tilde {w}_s$ is non-zero, and its profiles for
$\tilde {w}_s$ is non-zero, and its profiles for ![]() $\omega _s = 0.05$ at different streamwise locations are shown in figure 10(a). As expected, the perturbation peaks at the wall, and attenuates as the boundary-layer edge is approached. Neglecting the non-parallelism, the lateral velocity perturbation can be approximated by the Stokes layer solution
$\omega _s = 0.05$ at different streamwise locations are shown in figure 10(a). As expected, the perturbation peaks at the wall, and attenuates as the boundary-layer edge is approached. Neglecting the non-parallelism, the lateral velocity perturbation can be approximated by the Stokes layer solution ![]() ${{\mathcal {E}}}_s W_{Stokes}\exp ({-\textrm {i}\omega _s t})+\textrm {c.c.}$, where
${{\mathcal {E}}}_s W_{Stokes}\exp ({-\textrm {i}\omega _s t})+\textrm {c.c.}$, where
with ![]() $-\textrm {i}$ chosen to be
$-\textrm {i}$ chosen to be ![]() $\exp ({3{\rm \pi} \textrm {i}/2})$. The comparison of
$\exp ({3{\rm \pi} \textrm {i}/2})$. The comparison of ![]() $\tilde {w}_{s}$ at
$\tilde {w}_{s}$ at ![]() $x=240$ with
$x=240$ with ![]() $W_{Stokes}$ is plotted in figure 10(b), showing a good agreement.
$W_{Stokes}$ is plotted in figure 10(b), showing a good agreement.

Figure 10. (a) Wall-normal profiles of ![]() $|\tilde {w}_s|$ at different stations with
$|\tilde {w}_s|$ at different stations with ![]() $\omega _s=0.05$. (b) Comparison of
$\omega _s=0.05$. (b) Comparison of ![]() $\tilde {w}_{s,r}$ for
$\tilde {w}_{s,r}$ for ![]() $x=240$ with
$x=240$ with ![]() $W_{Stokes}$.
$W_{Stokes}$.
3.5. The HWNNS calculations for the perturbations excited by FSVD–vibration interaction
As suggested by (2.3), the perturbation field for a vibration configuration includes ![]() $\tilde {\boldsymbol {\varphi }}_f$,
$\tilde {\boldsymbol {\varphi }}_f$, ![]() $\tilde {\boldsymbol {\varphi }}_s$ and
$\tilde {\boldsymbol {\varphi }}_s$ and ![]() $\tilde {\boldsymbol {\varphi }}_\pm$. The streamwise velocity and temperature amplitudes of
$\tilde {\boldsymbol {\varphi }}_\pm$. The streamwise velocity and temperature amplitudes of ![]() $\tilde {\boldsymbol {\varphi }}_\pm$ are denoted by
$\tilde {\boldsymbol {\varphi }}_\pm$ are denoted by ![]() $\tilde {U}_\pm (x)=\max _y(|\tilde {u}_\pm (x,y)|)$ and
$\tilde {U}_\pm (x)=\max _y(|\tilde {u}_\pm (x,y)|)$ and ![]() $\tilde {T}_\pm (x)=\max _y(|\tilde {T}_\pm (x,y)|)$, respectively. Actually, there would be more perturbations excited by the higher-order interactions, such as the perturbation with frequencies
$\tilde {T}_\pm (x)=\max _y(|\tilde {T}_\pm (x,y)|)$, respectively. Actually, there would be more perturbations excited by the higher-order interactions, such as the perturbation with frequencies ![]() $2\omega _s+\omega _f$ and
$2\omega _s+\omega _f$ and ![]() $\omega _s+2\omega _f$, which are neglected in this subsection due to their smaller magnitudes. The resolution study for the HWNNS calculations is provided in figure 29(a) in Appendix A, and its comparison with the DNS result is provided in figure 30 in Appendix B.
$\omega _s+2\omega _f$, which are neglected in this subsection due to their smaller magnitudes. The resolution study for the HWNNS calculations is provided in figure 29(a) in Appendix A, and its comparison with the DNS result is provided in figure 30 in Appendix B.
For different vibration amplitudes, figures 11(a) and 11(b) display the evolutions of ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {T}_f$ obtained by HWNNS, respectively. All the curves follow the same trend as the non-vibration curve (red solid lines), i.e. increase drastically in the close neighbourhood of the leading edge, followed by mild decreases, and then increase gently with
$\tilde {T}_f$ obtained by HWNNS, respectively. All the curves follow the same trend as the non-vibration curve (red solid lines), i.e. increase drastically in the close neighbourhood of the leading edge, followed by mild decreases, and then increase gently with ![]() $x$ until the end of the calculations. Again,
$x$ until the end of the calculations. Again, ![]() $\tilde {T}_f$ is more than one order of magnitude higher than
$\tilde {T}_f$ is more than one order of magnitude higher than ![]() $\tilde {U}_f$. Remarkably, inclusion of the surface vibration leads to a reduction of the amplitudes
$\tilde {U}_f$. Remarkably, inclusion of the surface vibration leads to a reduction of the amplitudes ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {T}_f$ in the downstream region, and such a reduction strengthens as the vibration intensifies, indicating a suppression of the FSVD-induced streak. Such an observation agrees with that of Hicks & Ricco (Reference Hicks and Ricco2015). Figures 11(c) and 11(d) show the evolution of
$\tilde {T}_f$ in the downstream region, and such a reduction strengthens as the vibration intensifies, indicating a suppression of the FSVD-induced streak. Such an observation agrees with that of Hicks & Ricco (Reference Hicks and Ricco2015). Figures 11(c) and 11(d) show the evolution of ![]() $\tilde {U}_\pm$ and
$\tilde {U}_\pm$ and ![]() $\tilde {T}_\pm$, respectively, and a monotonic increase with
$\tilde {T}_\pm$, respectively, and a monotonic increase with ![]() $x$ is observed for each curve. As expected, a stronger vibration results in a greater amplitude of
$x$ is observed for each curve. As expected, a stronger vibration results in a greater amplitude of ![]() $\tilde {U}_\pm$ or
$\tilde {U}_\pm$ or ![]() $\tilde {T}_\pm$ in the downstream region, and at the same location,
$\tilde {T}_\pm$ in the downstream region, and at the same location, ![]() $\tilde {U}_+$ (
$\tilde {U}_+$ (![]() $\tilde {T}_+$) is greater than
$\tilde {T}_+$) is greater than ![]() $\tilde {U}_-$ (
$\tilde {U}_-$ (![]() $\tilde {T}_-$). A clearer measurement of the excited mode
$\tilde {T}_-$). A clearer measurement of the excited mode ![]() $\tilde {U}_\pm$ is to normalise them by
$\tilde {U}_\pm$ is to normalise them by ![]() $\tilde {U}_f$ at each
$\tilde {U}_f$ at each ![]() $x$ location, namely,
$x$ location, namely,
The evolution of ![]() $\tilde {A}_\pm$ for different
$\tilde {A}_\pm$ for different ![]() ${{\mathcal {E}}}_s$ is shown in figures 11(e) and 11( f). Overall, each curve shows a sharp increase in the leading-edge vicinity (
${{\mathcal {E}}}_s$ is shown in figures 11(e) and 11( f). Overall, each curve shows a sharp increase in the leading-edge vicinity (![]() $x<40$), followed by a mild variation, including an almost constant state in the downstream limit of
$x<40$), followed by a mild variation, including an almost constant state in the downstream limit of ![]() $\tilde {A}_-$ and an increase with
$\tilde {A}_-$ and an increase with ![]() $x$ of
$x$ of ![]() $\tilde {A}_+$. Increase of
$\tilde {A}_+$. Increase of ![]() ${{\mathcal {E}}}_s$ leads to higher values of
${{\mathcal {E}}}_s$ leads to higher values of ![]() $\tilde {A}_\pm$, and for
$\tilde {A}_\pm$, and for ![]() ${{\mathcal {E}}}_s=0.03$, the value of
${{\mathcal {E}}}_s=0.03$, the value of ![]() $\tilde {A}_+$ can even reach unity, indicating a strong distortion of the FSVD-induced streaks. If the wave angle
$\tilde {A}_+$ can even reach unity, indicating a strong distortion of the FSVD-induced streaks. If the wave angle ![]() $\varTheta$ of the FSVD were changed from
$\varTheta$ of the FSVD were changed from ![]() $45^\circ$ to
$45^\circ$ to ![]() $-45^\circ$, then the evolution curves of
$-45^\circ$, then the evolution curves of ![]() $\tilde {U}_+$ and
$\tilde {U}_+$ and ![]() $\tilde {U}_-$ would swap.
$\tilde {U}_-$ would swap.

Figure 11. Streamwise evolution of (a) ![]() $\tilde {U}_f$, (b)
$\tilde {U}_f$, (b) ![]() $\tilde {T}_f$), (c)
$\tilde {T}_f$), (c) ![]() $\tilde {U}_\pm$, (d)
$\tilde {U}_\pm$, (d) ![]() $\tilde {T}_\pm$, and (e, f)
$\tilde {T}_\pm$, and (e, f) ![]() $\tilde {A}_\pm \equiv \tilde {U}_\pm /\tilde {U}_f$), where
$\tilde {A}_\pm \equiv \tilde {U}_\pm /\tilde {U}_f$), where ![]() $k_3=1$,
$k_3=1$, ![]() $\varTheta =45^\circ$,
$\varTheta =45^\circ$, ![]() $\omega _s=0.05$ and
$\omega _s=0.05$ and ![]() $\omega _f=0$.
$\omega _f=0$.
Increasing the frequency of the FSVD to ![]() $\omega _f=0.17$, the streamwise evolutions of the perturbations, shown in figure 12, are similar to those for the stationary-FSVD configuration shown in figure 11. However, the amplification rates of
$\omega _f=0.17$, the streamwise evolutions of the perturbations, shown in figure 12, are similar to those for the stationary-FSVD configuration shown in figure 11. However, the amplification rates of ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {U}_\pm$ in the downstream region are much greater, because the perturbations at these frequencies enter the unstable region of the Mack first mode. Comparing the results for different vibration amplitudes, it is found that in the region
$\tilde {U}_\pm$ in the downstream region are much greater, because the perturbations at these frequencies enter the unstable region of the Mack first mode. Comparing the results for different vibration amplitudes, it is found that in the region ![]() $x<100$, the vibration suppresses the FSVD-induced perturbation
$x<100$, the vibration suppresses the FSVD-induced perturbation ![]() $\tilde {U}_f$, but a destabilising effect is observed in further downstream locations where the perturbation is the Mack mode. The implication is that the lateral vibration would suppress the non-modal perturbations but enhance the modal perturbations.
$\tilde {U}_f$, but a destabilising effect is observed in further downstream locations where the perturbation is the Mack mode. The implication is that the lateral vibration would suppress the non-modal perturbations but enhance the modal perturbations.

Figure 12. Streamwise evolution of ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {U}_\pm$ for
$\tilde {U}_\pm$ for ![]() $k_3=1$,
$k_3=1$, ![]() $\varTheta =45^\circ$ and
$\varTheta =45^\circ$ and ![]() $\omega _f=0.17$.
$\omega _f=0.17$.
In figure 13, we probe the behaviours of ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {U}_+$ by varying
$\tilde {U}_+$ by varying ![]() $\omega _f$,
$\omega _f$, ![]() $k_3$ and
$k_3$ and ![]() $\varTheta$. Comparing figure 13(a) to figure 9(b), we find that the vibration impact on the FSVD-induced perturbation at this
$\varTheta$. Comparing figure 13(a) to figure 9(b), we find that the vibration impact on the FSVD-induced perturbation at this ![]() ${{\mathcal {E}}}_s$ value is rather limited, and again, the minima of the
${{\mathcal {E}}}_s$ value is rather limited, and again, the minima of the ![]() $\tilde {U}_f$ curves indicate the critical frequency distinguishing the non-modal and modal perturbations. In contrast, the amplitudes
$\tilde {U}_f$ curves indicate the critical frequency distinguishing the non-modal and modal perturbations. In contrast, the amplitudes ![]() $\tilde {U}_+$ in the downstream locations do not show local minima, as shown in figure 13(d). To avoid the transition of
$\tilde {U}_+$ in the downstream locations do not show local minima, as shown in figure 13(d). To avoid the transition of ![]() $\tilde {U}_f$ from non-modal to modal perturbations and perform a clear investigation of the bypass transition, we select
$\tilde {U}_f$ from non-modal to modal perturbations and perform a clear investigation of the bypass transition, we select ![]() $\omega _f=0$ in the following. It is seen from figures 13(b,e) that
$\omega _f=0$ in the following. It is seen from figures 13(b,e) that ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {U}_+$ show broad peaks at a downstream location
$\tilde {U}_+$ show broad peaks at a downstream location ![]() $x=240$ in a region around
$x=240$ in a region around ![]() $k_3=1.4$. Setting
$k_3=1.4$. Setting ![]() $k_3=1$ and
$k_3=1$ and ![]() $\omega _f=0$, we plot the dependence of
$\omega _f=0$, we plot the dependence of ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {U}_+$ on the wave angle
$\tilde {U}_+$ on the wave angle ![]() $\varTheta =\tan ^{-1}(k_2/k_3)$ in figures 13(c, f), respectively. It should be noted that an FSVD with both
$\varTheta =\tan ^{-1}(k_2/k_3)$ in figures 13(c, f), respectively. It should be noted that an FSVD with both ![]() $\omega _f=0$ and
$\omega _f=0$ and ![]() $k_2=0$ is not possible from the dispersion relation (2.9), so the curves in the close neighbourhood of
$k_2=0$ is not possible from the dispersion relation (2.9), so the curves in the close neighbourhood of ![]() $\varTheta =0$ are not shown. Apparently, the boundary-layer response to both FSVD and vibration is stronger when the wave angle
$\varTheta =0$ are not shown. Apparently, the boundary-layer response to both FSVD and vibration is stronger when the wave angle ![]() $\varTheta$ is small. Therefore, in order to achieve a relatively high boundary-layer response, we choose
$\varTheta$ is small. Therefore, in order to achieve a relatively high boundary-layer response, we choose ![]() $\omega _f=0$,
$\omega _f=0$, ![]() $k_3=1$ and
$k_3=1$ and ![]() $\varTheta =15^\circ$ in the following nonlinear calculations.
$\varTheta =15^\circ$ in the following nonlinear calculations.

Figure 13. Streamwise evolution of (a–c) ![]() $\tilde {U}_f$ and (d–f)
$\tilde {U}_f$ and (d–f) ![]() $\tilde {U}_\pm$ for
$\tilde {U}_\pm$ for ![]() $\omega _s=0.05$ and
$\omega _s=0.05$ and ![]() ${{\mathcal {E}}}_s=0.01$: (a,d) dependence on
${{\mathcal {E}}}_s=0.01$: (a,d) dependence on ![]() $\omega _f$ for
$\omega _f$ for ![]() $k_3=1$ and
$k_3=1$ and ![]() $\varTheta =45^\circ$; (b,e) dependence on
$\varTheta =45^\circ$; (b,e) dependence on ![]() $k_3$ for
$k_3$ for ![]() $\omega _f=0$ and
$\omega _f=0$ and ![]() $\varTheta =45^\circ$; (c, f) dependence on
$\varTheta =45^\circ$; (c, f) dependence on ![]() $\varTheta$ for
$\varTheta$ for ![]() $\omega _f=0$ and
$\omega _f=0$ and ![]() $k_3=1$.
$k_3=1$.
4. Nonlinear evolution of the streaks and their SI
4.1. Nonlinear evolution of the streaks excited by FSVD–vibration interaction
As shown in the previous section, the vibration modulates perturbations ![]() $\tilde {U}_\pm$ with frequencies different from that of the FSVD-induced streak, and influences the amplitude evolution of the latter. If the amplitude of the FSVD
$\tilde {U}_\pm$ with frequencies different from that of the FSVD-induced streak, and influences the amplitude evolution of the latter. If the amplitude of the FSVD ![]() ${{\mathcal {E}}}_f$ is moderate, then the nonlinearity would not be negligible in the downstream region. In this subsection, we will perform NPSE calculations for the nonlinear evolution of the boundary-layer perturbations. The NPSE calculation starts from
${{\mathcal {E}}}_f$ is moderate, then the nonlinearity would not be negligible in the downstream region. In this subsection, we will perform NPSE calculations for the nonlinear evolution of the boundary-layer perturbations. The NPSE calculation starts from ![]() $X_0=60$, where the base flow already satisfies the self-similarity solution, and the inflow perturbations are obtained by the aforementioned HWNNS calculations. In the physical situation, the vibration frequency is usually low, so the FSVDs with lower frequencies play a more important role. Thus, for demonstration, we choose the frequency of the FSVD
$X_0=60$, where the base flow already satisfies the self-similarity solution, and the inflow perturbations are obtained by the aforementioned HWNNS calculations. In the physical situation, the vibration frequency is usually low, so the FSVDs with lower frequencies play a more important role. Thus, for demonstration, we choose the frequency of the FSVD ![]() $\omega _f$ to be zero; the perturbation evolution with sufficiently low
$\omega _f$ to be zero; the perturbation evolution with sufficiently low ![]() ${{\mathcal {E}}}_f$ values was shown in figure 11. In NPSE calculations, we choose
${{\mathcal {E}}}_f$ values was shown in figure 11. In NPSE calculations, we choose ![]() $(\mathcal { M},\mathcal { N})=(5,5)$, which has been confirmed to be sufficiently accurate as compared with the results with
$(\mathcal { M},\mathcal { N})=(5,5)$, which has been confirmed to be sufficiently accurate as compared with the results with ![]() $(\mathcal {M},\mathcal {N})$ doubled. The mesh resolution study of the NPSE can be found in figure 29(b) in Appendix A, and the comparison of the
$(\mathcal {M},\mathcal {N})$ doubled. The mesh resolution study of the NPSE can be found in figure 29(b) in Appendix A, and the comparison of the ![]() $\textrm {HWNNS}+\textrm {NPSE}$ calculations with DNS is provided in figure 31 in Appendix B.
$\textrm {HWNNS}+\textrm {NPSE}$ calculations with DNS is provided in figure 31 in Appendix B.
We first choose ![]() ${{\mathcal {E}}}_f=10^{-8}$ and perform a comparison between the HWNNS and NPSE calculations to confirm the consistency of our numerical results. The controlling parameters are
${{\mathcal {E}}}_f=10^{-8}$ and perform a comparison between the HWNNS and NPSE calculations to confirm the consistency of our numerical results. The controlling parameters are ![]() $\omega _s=0.05$,
$\omega _s=0.05$, ![]() ${{\mathcal {E}}}_s=0.01$,
${{\mathcal {E}}}_s=0.01$, ![]() $\omega _f=0$,
$\omega _f=0$, ![]() $k_3=1$ and
$k_3=1$ and ![]() $\varTheta =15^\circ$. The amplitudes of
$\varTheta =15^\circ$. The amplitudes of ![]() $\tilde {U}_{m,n}(x)=\max _y({{\mathcal {E}}}_{m,n}\,|\tilde {u}_{m,n}(x,y)|)$ and
$\tilde {U}_{m,n}(x)=\max _y({{\mathcal {E}}}_{m,n}\,|\tilde {u}_{m,n}(x,y)|)$ and ![]() $\tilde {T}_{m,n}(x)=\max _y({{\mathcal {E}}}_{m,n}\,|\tilde {T}_{m,n}(x,y)|)$ obtained by the two approaches are shown in figure 14. Here,
$\tilde {T}_{m,n}(x)=\max _y({{\mathcal {E}}}_{m,n}\,|\tilde {T}_{m,n}(x,y)|)$ obtained by the two approaches are shown in figure 14. Here, ![]() $(m,n)$ denotes a Fourier mode with frequency
$(m,n)$ denotes a Fourier mode with frequency ![]() $m\omega _s$ and spanwise wavenumber
$m\omega _s$ and spanwise wavenumber ![]() $nk_3$. Both the entrainment of the vortical perturbation
$nk_3$. Both the entrainment of the vortical perturbation ![]() $(0,1)$ and its interaction with the surface vibration (
$(0,1)$ and its interaction with the surface vibration (![]() $\pm 1,1$) obtained by the two approaches agree perfectly. The agreement of the perturbation profiles at
$\pm 1,1$) obtained by the two approaches agree perfectly. The agreement of the perturbation profiles at ![]() $x=300$, as shown in figure 15, is also achieved.
$x=300$, as shown in figure 15, is also achieved.

Figure 14. Evolution of the perturbation amplitudes (a) ![]() $\tilde {U}_{m,n}$ and (b)
$\tilde {U}_{m,n}$ and (b) ![]() $\tilde {T}_{m,n}$ for
$\tilde {T}_{m,n}$ for ![]() $\omega _f=0$,
$\omega _f=0$, ![]() ${{\mathcal {E}}}_f=10^{-8}$,
${{\mathcal {E}}}_f=10^{-8}$, ![]() $k_3=1$,
$k_3=1$, ![]() $\varTheta =15^\circ$,
$\varTheta =15^\circ$, ![]() $\omega _s=0.05$ and
$\omega _s=0.05$ and ![]() ${{\mathcal {E}}}_s=0.01$.
${{\mathcal {E}}}_s=0.01$.

Figure 15. Wall-normal profiles of (a) ![]() $\tilde {u}_f$, (b)
$\tilde {u}_f$, (b) ![]() $\tilde {w}_s$, (c)
$\tilde {w}_s$, (c) ![]() $\tilde {u}_+$ and (d)
$\tilde {u}_+$ and (d) ![]() $\tilde {u}_-$ at
$\tilde {u}_-$ at ![]() $x=300$. The parameters are the same as in figure 14.
$x=300$. The parameters are the same as in figure 14.
For the calculations of the nonlinear evolution, we choose five groups of controlling parameters with different vibration amplitudes ![]() ${{\mathcal {E}}}_s$ as shown in table 2. In this subsection, we let the FSVDs consist of a pair of oblique stationary vortical perturbations with opposite wall-normal wavenumbers
${{\mathcal {E}}}_s$ as shown in table 2. In this subsection, we let the FSVDs consist of a pair of oblique stationary vortical perturbations with opposite wall-normal wavenumbers ![]() $\pm k_2$:
$\pm k_2$:
Such a selection ensures the symmetric feature about the ![]() $y=0$ plane. Note that although the FSVD amplitudes
$y=0$ plane. Note that although the FSVD amplitudes ![]() ${{\mathcal {E}}}_f$ in table 2 are chosen to be as small as 0.0005, the superposition of the four Fourier components (including
${{\mathcal {E}}}_f$ in table 2 are chosen to be as small as 0.0005, the superposition of the four Fourier components (including ![]() $\hat {\boldsymbol \varphi }_{f,+}$,
$\hat {\boldsymbol \varphi }_{f,+}$, ![]() $\hat {\boldsymbol \varphi }_{f,-}$ and their complex conjugates) determines the physical amplitude of the FSVD to be 0.002. The boundary-layer responses to the FSVDs are denoted by
$\hat {\boldsymbol \varphi }_{f,-}$ and their complex conjugates) determines the physical amplitude of the FSVD to be 0.002. The boundary-layer responses to the FSVDs are denoted by ![]() $\tilde {\boldsymbol {\varphi }}_{f,\pm }(x,y) \exp ({\textrm {i} k_3 z})$, and the two components together show a longitudinal streak feature in the boundary layer.
$\tilde {\boldsymbol {\varphi }}_{f,\pm }(x,y) \exp ({\textrm {i} k_3 z})$, and the two components together show a longitudinal streak feature in the boundary layer.
Table 2. Parameters for perturbations for the nonlinear calculations.

Figure 16(a) plots the streamwise evolution of ![]() $\tilde {U}_{m,n}$ of the representative Fourier modes excited by the FSVDs for case A. Because
$\tilde {U}_{m,n}$ of the representative Fourier modes excited by the FSVDs for case A. Because ![]() ${{\mathcal {E}}}_{f}$ is not large, the excited streak mode
${{\mathcal {E}}}_{f}$ is not large, the excited streak mode ![]() $(0,1)$ calculated by NPSE (marked by the red solid line) agrees well with the prediction given by the HWNNS approach (marked by the squares) or the LPSE (marked by the circles). However, the NPSE has the capability to predict the evolution of higher-order harmonics, such as
$(0,1)$ calculated by NPSE (marked by the red solid line) agrees well with the prediction given by the HWNNS approach (marked by the squares) or the LPSE (marked by the circles). However, the NPSE has the capability to predict the evolution of higher-order harmonics, such as ![]() $(0,2)$,
$(0,2)$, ![]() $(0,3)$, and so on, as well as the mean-flow distortion (MFD)
$(0,3)$, and so on, as well as the mean-flow distortion (MFD) ![]() $(0,0)$. Initially, these components are taken to be zero at the inlet due to their small magnitudes. However, they are excited in the downstream region due to nonlinearity. This mismatch results in a kink in each curve near the inlet, but it does not affect the downstream NPSE calculations since different choices of
$(0,0)$. Initially, these components are taken to be zero at the inlet due to their small magnitudes. However, they are excited in the downstream region due to nonlinearity. This mismatch results in a kink in each curve near the inlet, but it does not affect the downstream NPSE calculations since different choices of ![]() $X_0$ yield the same downstream perturbation field. The harmonic streak mode
$X_0$ yield the same downstream perturbation field. The harmonic streak mode ![]() $(0,2)$ and MFD
$(0,2)$ and MFD ![]() $(0,0)$ are generated through the self-interaction of
$(0,0)$ are generated through the self-interaction of ![]() $(0,1)$ or the mutual interaction of (
$(0,1)$ or the mutual interaction of (![]() $0,\pm 1$), which grow with a rate twice of that of
$0,\pm 1$), which grow with a rate twice of that of ![]() $(0,1)$ in the downstream region, with the former being stronger. For the vibrating wall cases, as shown in figures 16(b–e), because of the additionally introduced perturbation
$(0,1)$ in the downstream region, with the former being stronger. For the vibrating wall cases, as shown in figures 16(b–e), because of the additionally introduced perturbation ![]() $(1,0)$, the Fourier modes (
$(1,0)$, the Fourier modes (![]() ${\pm }1,1$) are exited. Overall, the NPSE calculations of (
${\pm }1,1$) are exited. Overall, the NPSE calculations of (![]() ${\pm }1,1$) and
${\pm }1,1$) and ![]() $(0,1)$ are smaller than the HWNNS calculations in the region
$(0,1)$ are smaller than the HWNNS calculations in the region ![]() $x>120$, and the discrepancy enlarges as the vibration intensifies. Comparing the evolution of the streak mode
$x>120$, and the discrepancy enlarges as the vibration intensifies. Comparing the evolution of the streak mode ![]() $(0,1)$ for different cases, it is again seen that the stabilising effect of the vibration on the streak mode is evident, and the amplitudes of
$(0,1)$ for different cases, it is again seen that the stabilising effect of the vibration on the streak mode is evident, and the amplitudes of ![]() $({\pm }1, 1)$ even exceed the FSVD-induced perturbation
$({\pm }1, 1)$ even exceed the FSVD-induced perturbation ![]() $(0,1)$ in downstream locations for case E. The implication is that for this case, the streaky structure is strongly influenced by the vibration, which could alter its SI. This phenomenon was also reported in many papers for incompressible flows (Hack & Zaki Reference Hack and Zaki2014, Reference Hack and Zaki2015; Hicks & Ricco Reference Hicks and Ricco2015). Since the amplitudes of the components
$(0,1)$ in downstream locations for case E. The implication is that for this case, the streaky structure is strongly influenced by the vibration, which could alter its SI. This phenomenon was also reported in many papers for incompressible flows (Hack & Zaki Reference Hack and Zaki2014, Reference Hack and Zaki2015; Hicks & Ricco Reference Hicks and Ricco2015). Since the amplitudes of the components ![]() $(0,2)$ and
$(0,2)$ and ![]() $(0,0)$ have reached
$(0,0)$ have reached ![]() $O(0.01)$ in the downstream region for all cases considered, their influence on the streaky structure and its instability property is non-negligible, as will be studied in the next subsection.
$O(0.01)$ in the downstream region for all cases considered, their influence on the streaky structure and its instability property is non-negligible, as will be studied in the next subsection.

Figure 16. Nonlinear evolution of the amplitude of the boundary-layer perturbations ![]() $\tilde {U}_{m,n}$ for
$\tilde {U}_{m,n}$ for ![]() $\omega _f=0$ and
$\omega _f=0$ and ![]() $\omega _s=0.05$, where (a–e) show cases A–E.
$\omega _s=0.05$, where (a–e) show cases A–E.
4.2. The SI analysis
The streaks do not break down themselves, but they could support SI modes with higher growth rates, which can be analysed by the numerical approach in ![]() $\S$ 2.6.
$\S$ 2.6.
4.2.1. The SI analysis for case A
For case A, the streak is stationary with a long streamwise length scale compared to the SI wavelength, and the SI analysis can be performed at a chosen ![]() $x$ position based on the parallel-flow assumption. Figure 17(a) shows the streaky structure obtained by the NPSE calculation, where the spanwise domain size is set to be one wavelength of the FSVD,
$x$ position based on the parallel-flow assumption. Figure 17(a) shows the streaky structure obtained by the NPSE calculation, where the spanwise domain size is set to be one wavelength of the FSVD, ![]() $2{\rm \pi} /k_3\approx 6.28$. As
$2{\rm \pi} /k_3\approx 6.28$. As ![]() $x$ approaches downstream, the mean velocity
$x$ approaches downstream, the mean velocity ![]() $\breve U_B$ shows stronger gradients in both the spanwise and wall-normal directions.
$\breve U_B$ shows stronger gradients in both the spanwise and wall-normal directions.

Figure 17. Contours of the streamwise velocity ![]() $\breve {U}_B$ of the streaky base flow: (a) case A; (b) case C with
$\breve {U}_B$ of the streaky base flow: (a) case A; (b) case C with ![]() $t=0$; (c) case E with
$t=0$; (c) case E with ![]() $t=0$.
$t=0$.
The temporal SI analysis is performed by solving (2.14) numerically. The growth rates ![]() $\omega _i$ of the most unstable varicose and sinuous modes at three streamwise locations are shown in figure 18(a). The perturbation profiles of representative varicose and sinuous modes at
$\omega _i$ of the most unstable varicose and sinuous modes at three streamwise locations are shown in figure 18(a). The perturbation profiles of representative varicose and sinuous modes at ![]() $x=300$ are displayed in figure 19, showing a symmetric and an anti-symmetric feature along the centreline, respectively. For the base-flow profiles concerned here, the varicose mode is overall more unstable. At each streamwise location, the unstable zone shows two peaks, and the one with a higher wavenumber is more unstable. This is a reminiscent of the Mack mode instability for a Blasius base flow, but the instability property is modified quantitatively by the streaks. As
$x=300$ are displayed in figure 19, showing a symmetric and an anti-symmetric feature along the centreline, respectively. For the base-flow profiles concerned here, the varicose mode is overall more unstable. At each streamwise location, the unstable zone shows two peaks, and the one with a higher wavenumber is more unstable. This is a reminiscent of the Mack mode instability for a Blasius base flow, but the instability property is modified quantitatively by the streaks. As ![]() $x$ approaches downstream, the peak value of the second lobe decreases mildly, with its corresponding wavenumber shifted to a lower value. The phase speeds
$x$ approaches downstream, the peak value of the second lobe decreases mildly, with its corresponding wavenumber shifted to a lower value. The phase speeds ![]() $C_r$, shown in figure 18(b), vary in the region
$C_r$, shown in figure 18(b), vary in the region ![]() $(0.88,0.94)$, indicating an inviscid nature of the SI modes. Using the Gaster transformation (Gaster Reference Gaster1962; Xu et al. Reference Xu, Liu, Zhang and Wu2023), we are able to convert the temporal growth rate to the spatial growth rate via
$(0.88,0.94)$, indicating an inviscid nature of the SI modes. Using the Gaster transformation (Gaster Reference Gaster1962; Xu et al. Reference Xu, Liu, Zhang and Wu2023), we are able to convert the temporal growth rate to the spatial growth rate via
where ![]() $C_g \equiv |C_g|\exp ({\textrm {i} \vartheta }) \equiv \partial \omega / \partial \alpha$ is the complex group velocity. The spatial growth rate
$C_g \equiv |C_g|\exp ({\textrm {i} \vartheta }) \equiv \partial \omega / \partial \alpha$ is the complex group velocity. The spatial growth rate ![]() $-\tilde {\alpha }_i$ is plotted in figure 18(c), and a feature similar to that in figure 18(a) is evident.
$-\tilde {\alpha }_i$ is plotted in figure 18(c), and a feature similar to that in figure 18(a) is evident.

Figure 18. Dependence on ![]() $\alpha$ of (a) the temporal growth rate
$\alpha$ of (a) the temporal growth rate ![]() $\omega _i$, (b) the phase speed
$\omega _i$, (b) the phase speed ![]() $C_r$ and (c) the spatial growth rate
$C_r$ and (c) the spatial growth rate ![]() $-\tilde {\alpha }_i$, of representative unstable SI modes for case A.
$-\tilde {\alpha }_i$, of representative unstable SI modes for case A.

Figure 19. Eigenfunctions of ![]() $|\tilde {u}_{SI}|$ at
$|\tilde {u}_{SI}|$ at ![]() $x=300$ for case A: (a) mode I for
$x=300$ for case A: (a) mode I for ![]() $\alpha =1.4$; (b) mode II for
$\alpha =1.4$; (b) mode II for ![]() $\alpha =1.57$. The dashed lines represent the contours of the base flow
$\alpha =1.57$. The dashed lines represent the contours of the base flow ![]() $\breve {U}_B$.
$\breve {U}_B$.
The accumulated amplitude of a linear perturbation can be predicted by an ![]() $N$-factor, defined by
$N$-factor, defined by
where ![]() $x_1$ denotes the neutral location of the unstable perturbation. Based on the spatial growth rates
$x_1$ denotes the neutral location of the unstable perturbation. Based on the spatial growth rates ![]() $-\tilde {\alpha }_i$ shown in figure 20(a), the
$-\tilde {\alpha }_i$ shown in figure 20(a), the ![]() $N$-factors for different
$N$-factors for different ![]() $\omega$ values are shown in figure 20(b). For
$\omega$ values are shown in figure 20(b). For ![]() $\omega \leqslant 1.17$, the
$\omega \leqslant 1.17$, the ![]() $N$-factor grows gently with
$N$-factor grows gently with ![]() $x$ until the end of the calculation, and the greatest value of the
$x$ until the end of the calculation, and the greatest value of the ![]() $N$-factor is 4.49, appearing for
$N$-factor is 4.49, appearing for ![]() $\omega =0.5$ at
$\omega =0.5$ at ![]() $x=300$. For a higher
$x=300$. For a higher ![]() $\omega$ value, the
$\omega$ value, the ![]() $N$ curve undergoes a sharp increase at a certain position, due to the appearance of the second peak of the varicose mode. This sharp increase moves upstream as
$N$ curve undergoes a sharp increase at a certain position, due to the appearance of the second peak of the varicose mode. This sharp increase moves upstream as ![]() $\omega$ increases, agreeing with the peaks of the growth rates shown in figure 20(a).
$\omega$ increases, agreeing with the peaks of the growth rates shown in figure 20(a).

Figure 20. Evolution of (a) spatial growth rates ![]() $-\tilde {\alpha }_i$ and (b)
$-\tilde {\alpha }_i$ and (b) ![]() $N$-factors of the SI modes for different frequencies predicted by SI analysis for case A. The dash-dot-dotted lines denote the zero value.
$N$-factors of the SI modes for different frequencies predicted by SI analysis for case A. The dash-dot-dotted lines denote the zero value.
4.2.2. The SI analysis for cases with vibrating walls
For a vibrating wall, because the excited streaks vary with longer time and length scales than the SI modes, the SI analysis should be performed at a frozen time and streamwise location. Two snapshots of the streaky structure of cases C and E at ![]() $t=0$ are shown in figures 17(b) and 17(c), respectively. Figures 21(b), 21(c) and 21(d) further show the contours of the unsteady streaky base flow at
$t=0$ are shown in figures 17(b) and 17(c), respectively. Figures 21(b), 21(c) and 21(d) further show the contours of the unsteady streaky base flow at ![]() $x=300$ at different time instants for case C, and the base flow for case A is also shown in figure 21(a) for comparison. Here, the vibrating period is defined as
$x=300$ at different time instants for case C, and the base flow for case A is also shown in figure 21(a) for comparison. Here, the vibrating period is defined as ![]() $T_s=2{\rm \pi} /\omega _s$. The distortion of the streaky base flow by the unsteady vibration at different phases is clearly displayed.
$T_s=2{\rm \pi} /\omega _s$. The distortion of the streaky base flow by the unsteady vibration at different phases is clearly displayed.

Figure 21. Contours of the instant streamwise velocity ![]() $\breve {U}_B$ of the unsteady streaky base flow at
$\breve {U}_B$ of the unsteady streaky base flow at ![]() $x=300$: (a) case A; (b) case C at
$x=300$: (a) case A; (b) case C at ![]() $t=0$; (c) case C at
$t=0$; (c) case C at ![]() $t=T_s/4$; (d) case C at
$t=T_s/4$; (d) case C at ![]() $t=T_s/2$.
$t=T_s/2$.
The growth rates of the SI modes for the base flow shown in figure 21 are compared in figure 22. Because the base flow for case C is not symmetric along the centreline, it is not possible to define the varicose and sinuous modes. Therefore, we denote the two most unstable modes by mode I and mode II. For case C at ![]() $t=T_s/4$, the growth rate for the most unstable wavenumber band is quite close to that for case A. The vibration leads to a slight destabilising and stabilising effects on modes I and II, respectively. The instability properties for
$t=T_s/4$, the growth rate for the most unstable wavenumber band is quite close to that for case A. The vibration leads to a slight destabilising and stabilising effects on modes I and II, respectively. The instability properties for ![]() $t=0$ and
$t=0$ and ![]() $T_s/2$ are almost the same, for which both modes I and II have greater growth rates in a wide wavenumber band, except in a local region around the peaks of the two modes for case A. For case E, because the streaky structure is severely influenced by the vibration, more SI modes with greater growth rates can be found, and figure 23 displays only the most unstable two or three modes at different time instants. The destabilising effect due to the vibration is evident for the whole wavenumber band. The eigenfunctions of the most unstable modes for cases C and E are show in figure 24. For all the plots, the eigenfunctions peak at the localised region around the shoulder of the skewed mushroom-type structure where the shear is strong.
$T_s/2$ are almost the same, for which both modes I and II have greater growth rates in a wide wavenumber band, except in a local region around the peaks of the two modes for case A. For case E, because the streaky structure is severely influenced by the vibration, more SI modes with greater growth rates can be found, and figure 23 displays only the most unstable two or three modes at different time instants. The destabilising effect due to the vibration is evident for the whole wavenumber band. The eigenfunctions of the most unstable modes for cases C and E are show in figure 24. For all the plots, the eigenfunctions peak at the localised region around the shoulder of the skewed mushroom-type structure where the shear is strong.

Figure 22. Dependence on ![]() $\alpha$ of the temporal growth rate
$\alpha$ of the temporal growth rate ![]() $\omega _i$ of representative unstable SI modes for case C at
$\omega _i$ of representative unstable SI modes for case C at ![]() $x=300$, where the result for case A is also shown for reference: (a) mode I, (b) mode II.
$x=300$, where the result for case A is also shown for reference: (a) mode I, (b) mode II.

Figure 23. Dependence on ![]() $\alpha$ of the temporal growth rate
$\alpha$ of the temporal growth rate ![]() $\omega _i$ of representative unstable SI modes for case E at
$\omega _i$ of representative unstable SI modes for case E at ![]() $x=300$, where the result for case A is also shown for reference: (a)
$x=300$, where the result for case A is also shown for reference: (a) ![]() $t=0$ and
$t=0$ and ![]() $T_s/2$; (b)
$T_s/2$; (b) ![]() $t=T_s/4$.
$t=T_s/4$.

Figure 24. Eigenfunctions of ![]() $|\tilde {u}_{SI}|$ of mode I for cases C and E at
$|\tilde {u}_{SI}|$ of mode I for cases C and E at ![]() $x=300$: (a,b)
$x=300$: (a,b) ![]() $t=0$; (c,d)
$t=0$; (c,d) ![]() $t=T_s/4$; (e, f)
$t=T_s/4$; (e, f) ![]() $t=T_s/2$. The dashed lines represent the contours of the base flow
$t=T_s/2$. The dashed lines represent the contours of the base flow ![]() $\breve {U}_B$.
$\breve {U}_B$.
For an unsteady streaky base flow, the amplitude accumulation should be calculated in a more complicated way than that for case A, i.e. the integral path of the ![]() $N$-factor should follow the trajectory of the group velocity of the SI mode. The premise is that the variation of the streaky base flow is in a much longer length scale than the wavelength of the SI mode. Denote the SI growth rate at a time
$N$-factor should follow the trajectory of the group velocity of the SI mode. The premise is that the variation of the streaky base flow is in a much longer length scale than the wavelength of the SI mode. Denote the SI growth rate at a time ![]() $t$ and a location
$t$ and a location ![]() $x$ as
$x$ as ![]() $-\tilde {\alpha }_i(x, t)$. Then the
$-\tilde {\alpha }_i(x, t)$. Then the ![]() $N$-factor can be calculated by
$N$-factor can be calculated by
where ![]() $C_{g,r}$ denotes the group velocity of the SI mode, and
$C_{g,r}$ denotes the group velocity of the SI mode, and ![]() $\bar {t}_0$ is the initial time instant
$\bar {t}_0$ is the initial time instant ![]() $t_0$ distinguishing the initial phase. In figure 25, we show one example of the calculation strategy for
$t_0$ distinguishing the initial phase. In figure 25, we show one example of the calculation strategy for ![]() $\omega =1.33$ for case C. First, we plot the growth rate contours in the
$\omega =1.33$ for case C. First, we plot the growth rate contours in the ![]() $x{-}t$ plane in figure 25(a), where the results for five time periods are shown. The growth rate shows an appreciable variation for different phases within one period, but overall it shows a broad peak in the interval
$x{-}t$ plane in figure 25(a), where the results for five time periods are shown. The growth rate shows an appreciable variation for different phases within one period, but overall it shows a broad peak in the interval ![]() $x\in (210,300)$. The contours of the group velocity are shown in figure 25(b), which shows a peak at
$x\in (210,300)$. The contours of the group velocity are shown in figure 25(b), which shows a peak at ![]() $x\approx 195$, but becomes smaller at further downstream positions. The propagation trajectories of the SI modes in the
$x\approx 195$, but becomes smaller at further downstream positions. The propagation trajectories of the SI modes in the ![]() $x{-}t$ plane are shown by the arrowed lines, which are the basis for the calculations of the
$x{-}t$ plane are shown by the arrowed lines, which are the basis for the calculations of the ![]() $N$-factor starting from different phases. In figure 25(c), the dependence on
$N$-factor starting from different phases. In figure 25(c), the dependence on ![]() $x$ of the growth rates along the 16 trajectories is shown by the grey curves, with their averaged value marked by the red dashed line. For comparison, the result for case A is plotted by the black line. For
$x$ of the growth rates along the 16 trajectories is shown by the grey curves, with their averaged value marked by the red dashed line. For comparison, the result for case A is plotted by the black line. For ![]() $x<180$, the effect of vibration on the SI growth is a little stabilising, but a destabilising effect is evident for most of the initial phases in further downstream locations. Integrating
$x<180$, the effect of vibration on the SI growth is a little stabilising, but a destabilising effect is evident for most of the initial phases in further downstream locations. Integrating ![]() $-\tilde {\alpha }_i(\bar {x},\bar {t}_0+\bar {x}/C_{g,r})$ along
$-\tilde {\alpha }_i(\bar {x},\bar {t}_0+\bar {x}/C_{g,r})$ along ![]() $\bar {x}$, we obtain the
$\bar {x}$, we obtain the ![]() $N$-factor for different initial phases
$N$-factor for different initial phases ![]() $\bar {t}_0$, as shown in figure 25(d). It is seen that at this frequency, the evolution of the averaged
$\bar {t}_0$, as shown in figure 25(d). It is seen that at this frequency, the evolution of the averaged ![]() $N$-factor for case C is rather close to that of case A, but the instantaneous
$N$-factor for case C is rather close to that of case A, but the instantaneous ![]() $N$-factor scatters in the downstream region, showing an intermittent feature of the accumulated SI perturbations.
$N$-factor scatters in the downstream region, showing an intermittent feature of the accumulated SI perturbations.

Figure 25. Contours of (a) the growth rate ![]() $-\tilde {\alpha }_i(x,t)$ and (b) the group velocity
$-\tilde {\alpha }_i(x,t)$ and (b) the group velocity ![]() $C_{g,r}$ in the
$C_{g,r}$ in the ![]() $x{-}t$ plane for
$x{-}t$ plane for ![]() $\omega =1.33$ for case C. The arrowed lines denote the trajectories of the SI propagation for different initial phases. (c,d) The growth rate and the
$\omega =1.33$ for case C. The arrowed lines denote the trajectories of the SI propagation for different initial phases. (c,d) The growth rate and the ![]() $N$-factor for different initial phases, respectively, where the results for their averaged value and those for case A are shown by the red and black lines, respectively.
$N$-factor for different initial phases, respectively, where the results for their averaged value and those for case A are shown by the red and black lines, respectively.
In figure 26, we examine the evolution of the growth rate and the ![]() $N$-factor for two other representative frequencies. Among the 16 curves starting from different initial instants, we choose the two with the maximum and minimum values of the
$N$-factor for two other representative frequencies. Among the 16 curves starting from different initial instants, we choose the two with the maximum and minimum values of the ![]() $N$-factor at
$N$-factor at ![]() $x=300$, shown by the dashed and dotted lines. The time-averaged values are also shown by the curves with circles. As shown in figures 26(a,c), the growth rate for each case fluctuates in the downstream region, but the averaged growth rate is overall suppressed by the vibration for
$x=300$, shown by the dashed and dotted lines. The time-averaged values are also shown by the curves with circles. As shown in figures 26(a,c), the growth rate for each case fluctuates in the downstream region, but the averaged growth rate is overall suppressed by the vibration for ![]() $\omega =0.83$, and enhanced by the vibration for
$\omega =0.83$, and enhanced by the vibration for ![]() $\omega =2$. Integrating the growth rate along
$\omega =2$. Integrating the growth rate along ![]() $x$, we obtain the evolution of the
$x$, we obtain the evolution of the ![]() $N$-factors in figures 26(b,d). As expected, the averaged
$N$-factors in figures 26(b,d). As expected, the averaged ![]() $N$-factor in the downstream region is reduced for a low frequency, but is increased for a high frequency. Since the high-frequency perturbations attain a greater
$N$-factor in the downstream region is reduced for a low frequency, but is increased for a high frequency. Since the high-frequency perturbations attain a greater ![]() $N$-factor, they may be more important on triggering the bypass transition. Additionally, comparing the results for cases C and D, it is found that a stronger vibration leads to a greater increment of the
$N$-factor, they may be more important on triggering the bypass transition. Additionally, comparing the results for cases C and D, it is found that a stronger vibration leads to a greater increment of the ![]() $N$-factor. Meanwhile, at different initial time instants, the
$N$-factor. Meanwhile, at different initial time instants, the ![]() $N$-factor at
$N$-factor at ![]() $x=300$ and
$x=300$ and ![]() $\omega =2$ for case D can vary from 5 to 11, indicating the intermittent emergence of high-amplitude perturbations.
$\omega =2$ for case D can vary from 5 to 11, indicating the intermittent emergence of high-amplitude perturbations.

Figure 26. Streamwise evolution of (a,c) growth rate ![]() $-\tilde {\alpha }_i$ and (b,d) the
$-\tilde {\alpha }_i$ and (b,d) the ![]() $N$-factor of the SI modes, for (a,b)
$N$-factor of the SI modes, for (a,b) ![]() $\omega =0.83$ and (c,d)
$\omega =0.83$ and (c,d) ![]() $\omega =2.0$. Black curves indicate case A; red curves indicate case C; blue curves indicate case D. Solid lines with circles indicate time-averaged value; dashed (dotted) lines indicate a phase where the growth rate at
$\omega =2.0$. Black curves indicate case A; red curves indicate case C; blue curves indicate case D. Solid lines with circles indicate time-averaged value; dashed (dotted) lines indicate a phase where the growth rate at ![]() $x=300$ reaches the maximum (minimum) value.
$x=300$ reaches the maximum (minimum) value.
The dependence of the ![]() $N$-factors on
$N$-factors on ![]() $\omega$ for
$\omega$ for ![]() $x= 240$ and 300 is summarised in figures 27(a) and 27(b), respectively, where the shaded areas represent the results for the 16 initial phases. To better quantify the impact of the wall vibration on the amplitude accumulation, we introduce
$x= 240$ and 300 is summarised in figures 27(a) and 27(b), respectively, where the shaded areas represent the results for the 16 initial phases. To better quantify the impact of the wall vibration on the amplitude accumulation, we introduce
to denote the change of the ![]() $N$-factor relative to the no-vibration case. The results of
$N$-factor relative to the no-vibration case. The results of ![]() $\Delta N(240,\bar {t}_0)$ and
$\Delta N(240,\bar {t}_0)$ and ![]() $\Delta N(300,\bar {t}_0)$ are shown in figures 27(c) and 27(d), respectively. It is clearly seen that for
$\Delta N(300,\bar {t}_0)$ are shown in figures 27(c) and 27(d), respectively. It is clearly seen that for ![]() $\omega <1.33$, the majority of the SI perturbations are suppressed by the vibration (
$\omega <1.33$, the majority of the SI perturbations are suppressed by the vibration (![]() $\Delta N<0$), whereas for
$\Delta N<0$), whereas for ![]() $\omega >1.33$, an overall enhancement is observed (
$\omega >1.33$, an overall enhancement is observed (![]() $\Delta N>0$). Additionally, the shaded areas are wider for a higher-frequency band. The implication is that although on average the perturbations are enhanced by the vibration with
$\Delta N>0$). Additionally, the shaded areas are wider for a higher-frequency band. The implication is that although on average the perturbations are enhanced by the vibration with ![]() $\omega >1.33$, a postponement of transition can also be observed at some time instants, showing a strong intermittent feature.
$\omega >1.33$, a postponement of transition can also be observed at some time instants, showing a strong intermittent feature.

Figure 27. Dependence on ![]() $\omega$ of (a,b) the
$\omega$ of (a,b) the ![]() $N$-factor and (c,d)
$N$-factor and (c,d) ![]() $\Delta N$ at
$\Delta N$ at ![]() $x= 240$ and 300 for cases A, B, C and D. Lines indicate time-averaged solutions; shaded areas indicate 16-instants solutions.
$x= 240$ and 300 for cases A, B, C and D. Lines indicate time-averaged solutions; shaded areas indicate 16-instants solutions.
In figure 28(a), we further summarise the dependence of ![]() $\Delta N$ at
$\Delta N$ at ![]() $x=300$ on initial phases for
$x=300$ on initial phases for ![]() $\omega =0.83$. The values of
$\omega =0.83$. The values of ![]() $\Delta N$ for both cases B and C are less than
$\Delta N$ for both cases B and C are less than ![]() $-0.18$, indicating a stabilising effect of the vibration for the relatively low-frequency SI modes. The
$-0.18$, indicating a stabilising effect of the vibration for the relatively low-frequency SI modes. The ![]() $\Delta N$ values vary remarkably as the initial phase changes, and the averaged stabilising effect, shown by the dashed lines, is stronger when the vibration amplitude
$\Delta N$ values vary remarkably as the initial phase changes, and the averaged stabilising effect, shown by the dashed lines, is stronger when the vibration amplitude ![]() ${{\mathcal {E}}}_s$ is larger. However, for higher frequencies,
${{\mathcal {E}}}_s$ is larger. However, for higher frequencies, ![]() $\omega =1.33$ or 1.67, as shown in figures 28(b,c), an overall strong destabilising effect of the vibration is observed, except at some particular initial phases. The implication is that premature transition may appear if the high-frequency SI modes are the key factor to trigger the bypass transition.
$\omega =1.33$ or 1.67, as shown in figures 28(b,c), an overall strong destabilising effect of the vibration is observed, except at some particular initial phases. The implication is that premature transition may appear if the high-frequency SI modes are the key factor to trigger the bypass transition.

Figure 28. Dependence on ![]() $\bar {t}_0/T_s$ of
$\bar {t}_0/T_s$ of ![]() $\Delta N$ for the SI modes with (a)
$\Delta N$ for the SI modes with (a) ![]() $\omega =0.83$, (b)
$\omega =0.83$, (b) ![]() $\omega =1.33$ and (c)
$\omega =1.33$ and (c) ![]() $\omega =1.83$ at
$\omega =1.83$ at ![]() $x=300$. Lines with symbols indicate results with different initial phases; dashed lines indicate time-averaged results; yellow-shaded areas indicate
$x=300$. Lines with symbols indicate results with different initial phases; dashed lines indicate time-averaged results; yellow-shaded areas indicate ![]() $\Delta N<0$.
$\Delta N<0$.
5. Concluding remarks and discussion
For cruising high-speed vehicles, the surface vibration frequently appears as wall perturbations, which may induce an appreciative effect on the laminar–turbulent transition, leading to a remarkable change of the aerodynamic performance of the flights. However, this mechanism is not well understood. To address this issue, in this paper we develop a novel, high-efficiency numerical approach, namely (i) calculating the entrainment of the freestream vortical disturbances (FSVDs) and the excitation of the non-modal streaks using the harmonic weakly nonlinear Navier–Stokes (HWNNS) approach to accommodate the rapid distortion of the perturbation in the leading-edge vicinity, and (ii) calculating the nonlinear evolution of the streaks in the downstream region to accommodate the nonlinear interaction of finite-amplitude Fourier modes. From comparison with the DNS results for a number of representative case studies, the ![]() $\textrm {HWNNS}+\textrm {NPSE}$ approach (where NPSE means nonlinear parabolised stability equation) is confirmed to be reliable and accurate for calculating the evolution of boundary-layer perturbations excited by the FSVD–vibration interaction in hypersonic boundary layers.
$\textrm {HWNNS}+\textrm {NPSE}$ approach (where NPSE means nonlinear parabolised stability equation) is confirmed to be reliable and accurate for calculating the evolution of boundary-layer perturbations excited by the FSVD–vibration interaction in hypersonic boundary layers.
It is found that the entrainment of low-frequency FSVDs would lead to excitation and amplification of non-modal perturbations in hypersonic boundary layers, appearing as longitudinal streaks in both the streamwise velocity and temperature components, and the temperature perturbation is much greater due to the compressibility effect. If the perturbation frequency is above a certain value, then the non-modal perturbation would evolve into the Mack first mode in a downstream position. For a higher frequency, the transition from non-modal perturbation to the Mack mode appears earlier, and at the same position, the critical frequency distinguishing the non-modal and modal perturbations increases with the spanwise wavenumber.
When the lateral vibration is considered, the perturbations with frequencies ![]() $\omega _f\pm \omega _s$ would be excited in the boundary layer. It is found from a scaling estimate that even if the vibration amplitude is as small as
$\omega _f\pm \omega _s$ would be excited in the boundary layer. It is found from a scaling estimate that even if the vibration amplitude is as small as ![]() $R^{-1}$, it would have a leading-order impact on the FSVD-induced perturbation (with frequency
$R^{-1}$, it would have a leading-order impact on the FSVD-induced perturbation (with frequency ![]() $\omega _f$), because the streamwise gradient of the FSVD-induced perturbation is also of that magnitude. Therefore, the governing equations of
$\omega _f$), because the streamwise gradient of the FSVD-induced perturbation is also of that magnitude. Therefore, the governing equations of ![]() $\tilde {u}_f$ and
$\tilde {u}_f$ and ![]() $\tilde {u}_\pm$ form a coupled linear system, which is in principle the same as in the asymptotic study conducted by Hicks & Ricco (Reference Hicks and Ricco2015). These equations are solved using an iterative scheme. Because in each iterative step the governing equations appear as a weakly nonlinear form, the HWNNS approach is so-named. A parametric study of the response to the interaction of the FSVD and the surface vibration based on the HWNNS approach is carried out. The FSVD-induced non-modal perturbation
$\tilde {u}_\pm$ form a coupled linear system, which is in principle the same as in the asymptotic study conducted by Hicks & Ricco (Reference Hicks and Ricco2015). These equations are solved using an iterative scheme. Because in each iterative step the governing equations appear as a weakly nonlinear form, the HWNNS approach is so-named. A parametric study of the response to the interaction of the FSVD and the surface vibration based on the HWNNS approach is carried out. The FSVD-induced non-modal perturbation ![]() $\tilde {u}_f$ is suppressed by the lateral vibration, but the Mack first mode in the downstream region is enhanced. Such an effect becomes more evident as the vibration intensifies. The perturbation excited by the FSVD–vibration interaction
$\tilde {u}_f$ is suppressed by the lateral vibration, but the Mack first mode in the downstream region is enhanced. Such an effect becomes more evident as the vibration intensifies. The perturbation excited by the FSVD–vibration interaction ![]() $\tilde {u}_\pm$ increases monotonically with the vibration amplitude. Therefore, when the vibration is relatively strong, say
$\tilde {u}_\pm$ increases monotonically with the vibration amplitude. Therefore, when the vibration is relatively strong, say ![]() ${{\mathcal {E}}}_s=0.03$, the amplitude of
${{\mathcal {E}}}_s=0.03$, the amplitude of ![]() $\tilde {u}_\pm$ could become comparable with that of
$\tilde {u}_\pm$ could become comparable with that of ![]() $\tilde {u}_f$ in a downstream location, indicating a severe distortion of the FSVD-induced streaks.
$\tilde {u}_f$ in a downstream location, indicating a severe distortion of the FSVD-induced streaks.
Choosing ![]() ${{\mathcal {E}}}_f=0.0005$, the FSVD-induced streaks may accumulate to finite amplitudes in the downstream region. Therefore, the NPSE approach is employed for calculations of the nonlinear interactions among different Fourier modes. The NPSE calculations start from
${{\mathcal {E}}}_f=0.0005$, the FSVD-induced streaks may accumulate to finite amplitudes in the downstream region. Therefore, the NPSE approach is employed for calculations of the nonlinear interactions among different Fourier modes. The NPSE calculations start from ![]() $x=60$, where the nonlinearity is rather weak, and the inflow perturbations are obtained by the HWNNS calculations. The lateral vibration induces a Stokes layer of the spanwise velocity perturbation, which distorts the FSVD-induced streaks remarkably if the vibration intensity is moderate. Performing the secondary instability (SI) analysis, it is found that the instability property of the streaky base flow depends strongly on time, and the SI modes may be promoted or suppressed depending on their initial phases. Overall, the averaged effect is that the low-frequency and high-frequency SI modes are stabilised and destabilised by the vibration, respectively. Integrating the growth rates along the streamwise direction, we find that the high-frequency SI modes can be amplified by a greater factor, indicating their dominant role in triggering the bypass transition to turbulence. Therefore, the vibration is likely to promote the bypass transition for our cases, and a stronger vibration enhances the promotion effect.
$x=60$, where the nonlinearity is rather weak, and the inflow perturbations are obtained by the HWNNS calculations. The lateral vibration induces a Stokes layer of the spanwise velocity perturbation, which distorts the FSVD-induced streaks remarkably if the vibration intensity is moderate. Performing the secondary instability (SI) analysis, it is found that the instability property of the streaky base flow depends strongly on time, and the SI modes may be promoted or suppressed depending on their initial phases. Overall, the averaged effect is that the low-frequency and high-frequency SI modes are stabilised and destabilised by the vibration, respectively. Integrating the growth rates along the streamwise direction, we find that the high-frequency SI modes can be amplified by a greater factor, indicating their dominant role in triggering the bypass transition to turbulence. Therefore, the vibration is likely to promote the bypass transition for our cases, and a stronger vibration enhances the promotion effect.
It should be noted that our observation is a little different from the incompressible configurations studied by Hack & Zaki (Reference Hack and Zaki2014), for which a severe suppression of the non-modal streaks is observed for the properly chosen parameter set. This may be attributed to the following reason. The dimensionless amplitude of the vibration velocity for incompressible boundary layers could reach magnitudes of ![]() $O(0.1)$, but such values are unrealistic for a hypersonic configuration. In the case of weak vibrations with
$O(0.1)$, but such values are unrealistic for a hypersonic configuration. In the case of weak vibrations with ![]() ${{\mathcal {E}}}_s=O(0.01)$, the suppression effect of the FSVD-induced streaks with frequency
${{\mathcal {E}}}_s=O(0.01)$, the suppression effect of the FSVD-induced streaks with frequency ![]() $\omega _f$ is limited. However, the vibration induces an additional perturbation with a different frequency
$\omega _f$ is limited. However, the vibration induces an additional perturbation with a different frequency ![]() $\omega _f\pm \omega _s$, which has the potential to be amplified to the nonlinear phase in the downstream region. Indeed, whether the SI of the streaks is enhanced or suppressed depends on the combined effect of the aforementioned two factors.
$\omega _f\pm \omega _s$, which has the potential to be amplified to the nonlinear phase in the downstream region. Indeed, whether the SI of the streaks is enhanced or suppressed depends on the combined effect of the aforementioned two factors.
Actually, for hypersonic flights, the flat-plate model with a sharp leading edge studied in this paper is only idealised, and a blunt leading edge is usually employed to avoid extreme heat load. In such situations, the bow-shaped leading-edge shock appears, and the numerical scheme for the HLNS/HWNNS approach employed in this paper is not adequate to deal with the sharp jump of perturbations. Thus future work may be directed to the development of the shock-fitting scheme for the HLNS/HWNNS approach, to cope with the hypersonic transition for more complex geometries. Nevertheless, the present paper is still a significant step for developing an efficient means for the study of hypersonic transition.
Funding
This work is supported by the National Science Foundation of China (grant nos U20B2003, 92371104, 12372222, 12002235 and 11988102) and the Strategic Priority Research Program, CAS (no. XDB22040104).
Declaration of interests
The authors report no conflict of interest.
Appendix A. Resolution studies for HWNNS and NPSE
In figures 29(a) and 29(b), we show the resolution studies for the HWNNS and NPSE approaches, respectively, where ![]() $\omega _f=0$,
$\omega _f=0$, ![]() $k_3=1$,
$k_3=1$, ![]() $\varTheta =45^\circ$ and
$\varTheta =45^\circ$ and ![]() $\omega _s=0.05$. For the NPSE calculation, the amplitudes of the FSVD and vibration are chosen from case C. The results for three mesh scales are compared, where the standard mesh includes
$\omega _s=0.05$. For the NPSE calculation, the amplitudes of the FSVD and vibration are chosen from case C. The results for three mesh scales are compared, where the standard mesh includes ![]() $401\times 301$ for the HWNNS approach and
$401\times 301$ for the HWNNS approach and ![]() $230\times 301$ for the NPSE, and the other two mesh scales include doubled grid points in the
$230\times 301$ for the NPSE, and the other two mesh scales include doubled grid points in the ![]() $x$- and
$x$- and ![]() $y$-directions, respectively. Perfect agreement is achieved, confirming the reliability of our calculations.
$y$-directions, respectively. Perfect agreement is achieved, confirming the reliability of our calculations.

Figure 29. Resolution studies for HWNNS and NPSE obtained by different mesh scales. Lines indicate the standard mesh; squares indicate the mesh refined in the ![]() $x$-direction; circles indicate the mesh refined in the
$x$-direction; circles indicate the mesh refined in the ![]() $y$-direction.
$y$-direction.
Appendix B. Verification of HWNNS and NPSE calculations by DNS
As a newly-developed approach combining the HWNNS and NPSE approaches, the central issue is to confirm its reliability and accuracy by the well-accepted tool (DNS). The DNS code is the same as that used in § 2.3, but we need to introduce a low-frequency FSVD at both the inlet and upper boundaries and a lateral vibration at the wall, and extend the calculations to be 3-D; the detailed introduction of the DNS approach can be found in Song, Zhao & Huang (Reference Song, Zhao and Huang2020) and Song et al. (Reference Song, Zhao and Dong2023a).
The first group of case studies for verification is selected by putting the amplitude of FSVDs to be small, ![]() ${{\mathcal {E}}}_f=10^{-8}$, for which the high-order harmonics in (2.3) are indeed negligible. For these cases, the DNS results can be compared directly to the HWNNS calculations. Figures 30(a) and 30(b) display the streamwise evolution of the amplitude of
${{\mathcal {E}}}_f=10^{-8}$, for which the high-order harmonics in (2.3) are indeed negligible. For these cases, the DNS results can be compared directly to the HWNNS calculations. Figures 30(a) and 30(b) display the streamwise evolution of the amplitude of ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {U}_\pm$ for
$\tilde {U}_\pm$ for ![]() ${{\mathcal {E}}}_s=0.0001$ and 0.01, respectively, and the perturbation profiles at representative
${{\mathcal {E}}}_s=0.0001$ and 0.01, respectively, and the perturbation profiles at representative ![]() $x$ locations are shown in figures 30(c–h). Although a slight discrepancy appears for
$x$ locations are shown in figures 30(c–h). Although a slight discrepancy appears for ![]() $\tilde {u}_\pm$ in the downstream locations, the overall agreement between the results obtained by the two approaches is quite satisfactory.
$\tilde {u}_\pm$ in the downstream locations, the overall agreement between the results obtained by the two approaches is quite satisfactory.

Figure 30. Comparison of the evolution of perturbations between HWNNS and DNS for ![]() ${{\mathcal {E}}}_f=10^{-8}$, where
${{\mathcal {E}}}_f=10^{-8}$, where ![]() $\omega _f=0$,
$\omega _f=0$, ![]() $k_3=1$ and
$k_3=1$ and ![]() $\varTheta =15^\circ$, for (a,c,e,g)
$\varTheta =15^\circ$, for (a,c,e,g) ![]() ${{\mathcal {E}}}_s=0.0001$, (b,d, f,h)
${{\mathcal {E}}}_s=0.0001$, (b,d, f,h) ![]() ${{\mathcal {E}}}_s=0.01$. (a,b) Streamwise evolution of
${{\mathcal {E}}}_s=0.01$. (a,b) Streamwise evolution of ![]() $\tilde {U}_f$ and
$\tilde {U}_f$ and ![]() $\tilde {U}_\pm$. (c–h) Wall-normal profiles at
$\tilde {U}_\pm$. (c–h) Wall-normal profiles at ![]() $x=15$, 30 and 60, in which the horizontal dashed lines denote the nominal boundary-layer thickness.
$x=15$, 30 and 60, in which the horizontal dashed lines denote the nominal boundary-layer thickness.
In figure 31, we show the results by increasing the FSVD amplitude to ![]() ${{\mathcal {E}}}_f=0.0005$, and the parameters are chosen from cases C and E. It needs to be emphasised that for a clean comparison, only one Fourier component in (4.1),
${{\mathcal {E}}}_f=0.0005$, and the parameters are chosen from cases C and E. It needs to be emphasised that for a clean comparison, only one Fourier component in (4.1), ![]() ${{\mathcal {E}}}_f\hat {\boldsymbol \varphi }_{f,+}\exp ({\textrm {i} (k_2 y+ k_3 z)})+\textrm {c.c.}$, is considered here. Therefore, the numerical results in this figure are different from the NPSE calculations in figure 16. Although the HWNNS calculations for
${{\mathcal {E}}}_f\hat {\boldsymbol \varphi }_{f,+}\exp ({\textrm {i} (k_2 y+ k_3 z)})+\textrm {c.c.}$, is considered here. Therefore, the numerical results in this figure are different from the NPSE calculations in figure 16. Although the HWNNS calculations for ![]() $\tilde {u}_f$ (or
$\tilde {u}_f$ (or ![]() $\tilde {U}_{0,1}$) and
$\tilde {U}_{0,1}$) and ![]() $\tilde {u}_\pm$ (or
$\tilde {u}_\pm$ (or ![]() $\tilde {U}_{\pm 1,1}$) agree overall with the DNS results with a limited discrepancy, they do not include the evolution of the MFD, component
$\tilde {U}_{\pm 1,1}$) agree overall with the DNS results with a limited discrepancy, they do not include the evolution of the MFD, component ![]() $(0,0)$, and the harmonics shown by the black and brown curves in the DNS results. Since the MFD has reached amplitude 0.006 at
$(0,0)$, and the harmonics shown by the black and brown curves in the DNS results. Since the MFD has reached amplitude 0.006 at ![]() $x=300$ for both cases, its impact on the SI would not be neglected. Therefore, the NPSE calculation is employed to take into account the nonlinear interaction among different Fourier components, starting from
$x=300$ for both cases, its impact on the SI would not be neglected. Therefore, the NPSE calculation is employed to take into account the nonlinear interaction among different Fourier components, starting from ![]() $x=60$. After a short adjustment (
$x=60$. After a short adjustment (![]() $x\in (60,100)$), the agreement between the NPSE and DNS results is quite satisfactory. Additionally, as expected, the NPSE calculations of
$x\in (60,100)$), the agreement between the NPSE and DNS results is quite satisfactory. Additionally, as expected, the NPSE calculations of ![]() $\tilde {U}_{0,1}$ and
$\tilde {U}_{0,1}$ and ![]() $\tilde {U}_{\pm 1, 1}$ are a little closer to the DNS results in comparison with the HWNNS calculations in downstream regions.
$\tilde {U}_{\pm 1, 1}$ are a little closer to the DNS results in comparison with the HWNNS calculations in downstream regions.

Figure 31. Comparison of the evolution of perturbations between HWNNS, NPSE and DNS for (a,c,e,g) case C, and (b,d, f,h) case E. (a,b) Streamwise evolution of ![]() $\tilde {U}_{m,n}$. (c–h) Wall-normal profiles at
$\tilde {U}_{m,n}$. (c–h) Wall-normal profiles at ![]() $x=180$ and 300, in which the horizontal dashed lines denote the nominal boundary-layer thickness.
$x=180$ and 300, in which the horizontal dashed lines denote the nominal boundary-layer thickness.
To conclude, the above comparison confirms that our ![]() $\textrm {HWNNS}+\textrm {NPSE}$ approach provides reliable and accurate results in comparison with those obtained by DNS, although there exists a small discrepancy in the downstream region for both low and high
$\textrm {HWNNS}+\textrm {NPSE}$ approach provides reliable and accurate results in comparison with those obtained by DNS, although there exists a small discrepancy in the downstream region for both low and high ![]() ${{\mathcal {E}}}_f$ values. The reason may be attributed to the difference between the numerical schemes, i.e. the central scheme is employed for HWNNS, and the flux-splitting method with the WENO scheme is employed for DNS. The existence of the sharp leading edge challenges the numerical approach, and a small discrepancy computed by different numerical schemes may occur in the leading-edge vicinity, which will not be diminished by increasing the grid resolution. Such a discrepancy may be removed if the sharp leading edge is replaced by a blunt leading edge, which will be considered in our future study.
${{\mathcal {E}}}_f$ values. The reason may be attributed to the difference between the numerical schemes, i.e. the central scheme is employed for HWNNS, and the flux-splitting method with the WENO scheme is employed for DNS. The existence of the sharp leading edge challenges the numerical approach, and a small discrepancy computed by different numerical schemes may occur in the leading-edge vicinity, which will not be diminished by increasing the grid resolution. Such a discrepancy may be removed if the sharp leading edge is replaced by a blunt leading edge, which will be considered in our future study.