No CrossRef data available.
Article contents
Teichmüller displacement theorem on Gromov hyperbolic spaces
Part of:
Analysis on metric spaces
Published online by Cambridge University Press: 06 November 2024
Abstract
Given a Gromov hyperbolic domain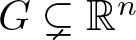 
$G\subsetneq \mathbb{R}^n$ with uniformly perfect Gromov boundary, Zhou and Rasila recently proved that for all quasiconformal homeomorphisms 
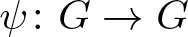$\psi\colon G\to G$ with identity value on the Gromov boundary, the quasihyperbolic displacement 
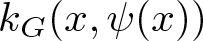$k_G(x,\psi(x))$ for all 
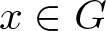$x\in G$ is bounded above. In this paper, we generalize this result and establish Teichmüller displacement theorem for quasi-isometries of Gromov hyperbolic spaces in a quantitative way. As applications, we obtain its connections to bilipschitz extensions of certain Gromov hyperbolic spaces.
Keywords
MSC classification
Secondary:
30L05: Geometric embeddings of metric spaces
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 67 , Issue 4 , November 2024 , pp. 1148 - 1166
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society.
References
Bonfert–Taylor, P., Canary, R. D., Martin, G. and Taylor, E., Quasiconformal homogeneity of hyperbolic manifolds, Math. Ann. 331(2) (2005), 281–295.CrossRefGoogle Scholar
Bonk, M., Heinonen, J. and Koskela, P., Uniformizing Gromov hyperbolic spaces, Astérisque 270 (2001), .Google Scholar
Bonk, M. and Schramm, O., Embeddings of Gromov hyperbolic spaces, Geom. Funct. Anal. 10(2) (2000), 266–306.CrossRefGoogle Scholar
Bridson, M. and Haefliger, A., Metric Spaces of Non-Positive Curvature, Grundlehren der Mathematischen Wissenschaften, Volume 319, (Springer-Verlag, Berlin, 1999).CrossRefGoogle Scholar
Buyalo, S. and Schroeder, V., Elements of asymptotic geometry (EMS Monographs in Mathematics: Zürich, xii+200 pp. 2007).CrossRefGoogle Scholar
Gehring, F. W. and Osgood, B. G., Uniform domains and the quasi-hyperbolic metric, J. Anal. Math. 36 (1979), 50–74.CrossRefGoogle Scholar
Hästö, P., Gromov hyperbolicity of the jG and 
$\widetilde{j}_G$ metrics, Proc. Amer. Math. Soc. 134(4) (2006), 1137–1142.CrossRefGoogle Scholar
$\widetilde{j}_G$ metrics, Proc. Amer. Math. Soc. 134(4) (2006), 1137–1142.CrossRefGoogle ScholarHariri, P., Klén, R. and Vuorinen, M., Conformally invariant metrics and quasiconformal mappings. Springer Monographs in Mathematics, . (Springer, Cham, 2020).CrossRefGoogle Scholar
Heinonen, J., Lectures on Analysis on Metric Spaces (Springer-Verlag, Berlin-Heidelberg-New York, 2001).CrossRefGoogle Scholar
Herron, D., Shanmugalingam, N. and Xie, X., Uniformity from Gromov hyperbolicity, Illinois J. Math. 52(4) (2008), 1065–1109.CrossRefGoogle Scholar
Klén, R., Todorčević, V. and Vuorinen, M., Teichmüller’s problem in space, J. Math. Anal. Appl. 455(2) (2017), 1297–1316.CrossRefGoogle Scholar
Li, Y., Vuorinen, M. and Wang, X., Quasiconformal maps with bilipschitz or identity boundary values in Banach spaces, Ann. Acad. Sci. Fenn. Math. 39(2) (2014), 905–917.Google Scholar
Lukyanenko, A., Bi-Lipschitz extension from boundaries of certain hyperbolic spaces, Geom. Dedicata 164 (2013), 47–71.CrossRefGoogle Scholar
Manojlović, V. and Vuorinen, M., On quasiconformal maps with identity boundary values, Trans. Amer. Math. Soc. 363(4) (2011), 2467–2479.CrossRefGoogle Scholar
Rainio, O., Sugawa, T. and Vuorinen, M., Uniformly perfect sets, Hausdorff dimension, and conformal capacity, J. Geom. Anal. 34(6) (2024), , 10.1007/s12220-024-01599-5.CrossRefGoogle Scholar
Shanmugalingam, N. and Xie, X., A rigidity property of some negatively curved solvable Lie groups, Comment. Math. Helv. 87(4) (2012), 805–823.CrossRefGoogle Scholar
Sugawa, T., Uniformly perfect sets–analytic and geometric aspects, Sugaku 53(4) (2001), 387–402.Google Scholar
Sugawa, T., Vuorinen, M. and Zhang, T., Conformally invariant complete metrics, Math. Proc. Cambridge Philos. Soc. 174(2) (2023), 273–300.CrossRefGoogle Scholar
Teichmüller, O., Ein Verschiebungssatz der quasikonformen Abbildung, Deutsche Math, 7 (1944), 336–343.Google Scholar
Väisälä, J., Hyperbolic and uniform domains in Banach spaces, Ann. Acad. Sci. Fenn. Ser. A I Math. 30(2) (2005), 261–302.Google Scholar
Vuorinen, M. and Zhang, X., Distortion of quasiconformal mappings with identity boundary values, J. Lond. Math. Soc. 90(3) (2014), 637–653.CrossRefGoogle Scholar
Wang, X. and Zhou, Q., Quasimöbius maps, weakly quasimöbius maps and uniform perfectness in quasi-metric spaces, Ann. Acad. Sci. Fenn. Ser. AI Math. 42(1) (2017), 257–284.CrossRefGoogle Scholar
Xie, X., Quasiisometries between negatively curved Hadamard manifolds, J. Lond. Math. Soc. 79(1) (2009), 15–32.CrossRefGoogle Scholar
Xie, X., Quasisymmetric maps on the boundary of a negatively curved solvable Lie group, Math. Ann. 353(3) (2012), 727–746.CrossRefGoogle Scholar
Zhou, Q., Li, Y. and Rasila, A., Gromov hyperbolicity, John spaces, and quasihyperbolic geodesics, J. Geom. Anal. 32(9) (2022), .CrossRefGoogle Scholar
Zhou, Q., Ponnusamy, S. and Guan, T., Gromov hyperbolicity of the $\widetilde{j}_G$ metric and boundary correspondence, Proc. Amer. Math. Soc. 150(7) (2022), 2839–2847.CrossRefGoogle Scholar
$\widetilde{j}_G$ metric and boundary correspondence, Proc. Amer. Math. Soc. 150(7) (2022), 2839–2847.CrossRefGoogle ScholarZhou, Q., Ponnusamy, S. and Rasila, A., Busemann functions and uniformization of Gromov hyperbolic spaces, https://arxiv.org/pdf/2008.01399v2.pdf.Google Scholar
Zhou, Q. and Rasila, A., Teichmüller’s problem for Gromov hyperbolic domains, Israel J. Math. 252(1) (2022), 399–427.CrossRefGoogle Scholar