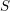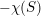Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ballmann, Werner
Matthiesen, Henrik
and
Mondal, Sugata
2017.
On the analytic systole of Riemannian surfaces of finite type.
Geometric and Functional Analysis,
Vol. 27,
Issue. 5,
p.
1070.
Mondal, Sugata
2019.
Topological properties of eigenfunctions of Riemannian surfaces.
Annales de la Faculté des sciences de Toulouse : Mathématiques,
Vol. 28,
Issue. 3,
p.
593.
Magee, Michael
and
Naud, Frédéric
2020.
Explicit spectral gaps for random covers of Riemann surfaces.
Publications mathématiques de l'IHÉS,
Vol. 132,
Issue. 1,
p.
137.
De Luca, G. Bruno
De Ponti, Nicolò
Mondino, Andrea
and
Tomasiello, Alessandro
2021.
Cheeger bounds on spin-two fields.
Journal of High Energy Physics,
Vol. 2021,
Issue. 12,
Soares, Louis
2022.
Hecke Triangle Groups, Transfer Operators and Hausdorff Dimension.
Annales Henri Poincaré,
Vol. 23,
Issue. 4,
p.
1239.
Falliero, Thérèse
2024.
Selberg Zeta function and hyperbolic Eisenstein series.
Communications in Contemporary Mathematics,
Vol. 26,
Issue. 05,
Ballmann, Werner
and
Mondal, Sugata
2025.
Spectral Instability of Coverings.
International Mathematics Research Notices,
Vol. 2025,
Issue. 1,