Hostname: page-component-6bf8c574d5-vmclg Total loading time: 0 Render date: 2025-02-16T21:01:04.064Z Has data issue: false hasContentIssue false
  • English
  • Français

Return-time 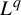$L^q$-spectrum for equilibrium states with potentials of summable variation

Part of: Ergodic theory Topological dynamics Dynamical systems with hyperbolic behavior

Published online by Cambridge University Press:  06 June 2022

M. ABADI ,
V. AMORIM ,
J.-R. CHAZOTTES Open the ORCID record for J.-R. CHAZOTTES [Opens in a new window]  and
S. GALLO
M. ABADI
Affiliation:
Instituto de Matemática e Estatística, Universidade de São Paulo, São Paulo, Brasil (e-mail: [email protected])
V. AMORIM
Affiliation:
Instituto Federal de São Paulo, São Paulo, Brasil (e-mail: [email protected])
J.-R. CHAZOTTES*
Affiliation:
CPHT, CNRS, IP Paris, Palaiseau, France
S. GALLO
Affiliation:
Departamento de Estatística, Universidade Federal de São Carlos, São Carlos, Brasil (e-mail: [email protected])
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 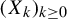$(X_k)_{k\geq 0}$ be a stationary and ergodic process with joint distribution 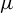$\mu $ , where the random variables 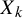$X_k$ take values in a finite set 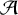$\mathcal {A}$ . Let 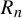$R_n$ be the first time this process repeats its first n symbols of output. It is well known that 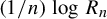$({1}/{n})\log R_n$ converges almost surely to the entropy of the process. Refined properties of $R_n$ (large deviations, multifractality, etc) are encoded in the return-time $L^q$ -spectrum defined as

provided the limit exists. We consider the case where 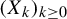$(X_k)_{k\geq 0}$ is distributed according to the equilibrium state of a potential 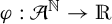with summable variation, and we prove that

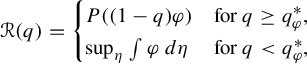
where $P((1-q)\varphi )$ is the topological pressure of $(1-q)\varphi $ , the supremum is taken over all shift-invariant measures, and 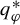$q_\varphi ^*$ is the unique solution of $P((1-q)\varphi ) =\sup _\eta \int \varphi \,d\eta $ . Unexpectedly, this spectrum does not coincide with the 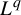$L^q$ -spectrum of 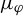$\mu _\varphi $ , which is $P((1-q)\varphi )$ , and it does not coincide with the waiting-time $L^q$ -spectrum in general. 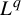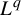In fact, the return-time $L^q$ -spectrum coincides with the waiting-time $L^q$ -spectrum if and only if the equilibrium state of 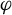$\varphi $ is the measure of maximal entropy. As a by-product, we also improve the large deviation asymptotics of 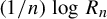$({1}/{n})\log R_n$ .

Keywords

Poincaré recurrenceentropyGibbs measuresφ-mixing processlarge deviations

MSC classification

Primary: 37B20: Notions of recurrence 37D35: Thermodynamic formalism, variational principles, equilibrium states
Secondary: 37A25: Ergodicity, mixing, rates of mixing 37A50: Relations with probability theory and stochastic processes
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 43 , Issue 8 , August 2023 , pp. 2489 - 2515
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abadi, M., Amorim, V. and Gallo, S.. Potential well in Poincaré recurrence. Entropy 23(3) (2021), Paper no. 379, 26 pp.10.3390/e23030379CrossRefGoogle ScholarPubMed
Abadi, M., Cardeño, L. and Gallo, S.. Potential well spectrum and hitting time in renewal processes. J. Stat. Phys. 159(5) (2015), 1087–1106.10.1007/s10955-015-1216-yCrossRefGoogle Scholar
Abadi, M. and Vergne, N.. Sharp error terms for return time statistics under mixing conditions. J. Theoret. Probab. 22(1) (2009), 18–37.10.1007/s10959-008-0199-xCrossRefGoogle Scholar
Bowen, R.. Equilibrium States and the Ergodic Theory of Anosov Diffeomorphisms (Lecture Notes in Mathematics, 470), revised edition. Ed. Jean-René Chazottes. Springer-Verlag, Berlin, 2008. With a preface by D. Ruelle.10.1007/978-3-540-77695-6CrossRefGoogle Scholar
Bradley, R. C.. Basic properties of strong mixing conditions. A survey and some open questions. Probab. Surv. 2 (2005), 107–144. Update of, and a supplement to, the 1986 original.CrossRefGoogle Scholar
Caby, T., Faranda, D., Mantica, G., Vaienti, S. and Yiou, P.. Generalized dimensions, large deviations and the distribution of rare events. Phys. D 400 (2019), 132143, 15 pp.CrossRefGoogle Scholar
Chazottes, J.-R. and Ugalde, E.. Entropy estimation and fluctuations of hitting and recurrence times for Gibbsian sources. Discrete Contin. Dyn. Syst. Ser. B 5(3) (2005), 565–586.Google Scholar
Collet, P., Galves, A. and Schmitt, B.. Repetition times for Gibbsian sources. Nonlinearity 12(4) (1999), 1225–1237.CrossRefGoogle Scholar
Coutinho, A., Rousseau, J. and Saussol, B.. Large deviation for return times. Nonlinearity 31(11) (2018), 5162.CrossRefGoogle Scholar
Dembo, A. and Zeitouni, O.. Large Deviations Techniques and Applications (Stochastic Modelling and Applied Probability, 38). Springer-Verlag, Berlin, 2010. Corrected reprint of the second (1998) edition.10.1007/978-3-642-03311-7CrossRefGoogle Scholar
Hadyn, N., Luevano, J., Mantica, G. and Vaienti, S.. Multifractal properties of return time statistics. Phys. Rev. Lett. 88 (2002), 224502.CrossRefGoogle ScholarPubMed
Jain, S. and Bansal, R. K.. On large deviation property of recurrence times. Proc. 2013 IEEE International Symposium on Information Theory. IEEE, Piscataway, NJ, 2013, pp. 2880–2884.CrossRefGoogle Scholar
Jenkinson, O.. Ergodic optimization. Discrete Contin. Dyn. Syst. 15(1) (2006), 197–224.10.3934/dcds.2006.15.197CrossRefGoogle Scholar
Kamath, S. and Verdú, S.. Estimation of entropy rate and Rényi entropy rate for Markov chains. Proc. 2016 IEEE International Symposium on Information Theory (ISIT). IEEE, Piscataway, NJ, 2016, pp. 685–689.CrossRefGoogle Scholar
Ornstein, D. S. and Weiss, B.. Entropy and data compression schemes. IEEE Trans. Inform. Theory 39(1) (1993), 78–83.10.1109/18.179344CrossRefGoogle Scholar
Parry, W. and Pollicott, M.. Zeta functions and the periodic orbit structure of hyperbolic dynamics. Astérisque 187–188 (1990), 268.Google Scholar
Plachky, D. and Steinebach, J.. A theorem about probabilities of large deviations with an application to queuing theory. Period. Math. Hungar. 6(4) (1975), 343–345.CrossRefGoogle Scholar
Shields, P. C.. The Ergodic Theory of Discrete Sample Paths (Graduate Studies in Mathematics, 13). American Mathematical Society, Providence, RI, 1996.CrossRefGoogle Scholar
Szpankowski, W.. A generalized suffix tree and its (un) expected asymptotic behaviors. SIAM J. Comput. 22(6) (1993), 1176–1198.CrossRefGoogle Scholar
Szpankowski, W.. Average Case Analysis of Algorithms on Sequences (Wiley-Interscience Series in Discrete Mathematics and Optimization). Wiley-Interscience, New York, 2001. With a foreword by P. Flajolet.10.1002/9781118032770CrossRefGoogle Scholar
Takens, F. and Verbitski, E.. Multifractal analysis of local entropies for expansive homeomorphisms with specification. Comm. Math. Phys. 203(3) (1999), 593–612.CrossRefGoogle Scholar
Walters, P.. Ruelle’s operator theorem and $g$ -measures. Trans. Amer. Math. Soc. 214 (1975), 375–387.Google Scholar