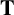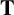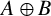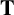1 Introduction
Throughout the paper, all Tannakian categories are neutral. We will freely use the language of Tannakian categories (see [Reference Deligne, Milne, Deligne, Milne, Ogus and Shih3] for further details).
Let K be a field of characteristic zero and
![]() $\mathbf {T}$
a Tannakian category over K. Given any object X of
$\mathbf {T}$
a Tannakian category over K. Given any object X of
![]() $\mathbf {T}$
, let
$\mathbf {T}$
, let
![]() $\mathrm{End}_{\mathbf {T}}(X)$
be the endomorphism algebra of X. Given a subobject Y of X, denote the subalgebra of
$\mathrm{End}_{\mathbf {T}}(X)$
be the endomorphism algebra of X. Given a subobject Y of X, denote the subalgebra of
![]() $\mathrm{End}_{\mathbf {T}}(X)$
consisting of the endomorphisms that restrict to an endomorphism of Y (that is, those mapping Y to Y) by
$\mathrm{End}_{\mathbf {T}}(X)$
consisting of the endomorphisms that restrict to an endomorphism of Y (that is, those mapping Y to Y) by
![]() $\mathrm{End}_{\mathbf {T}}(X;Y)$
.
$\mathrm{End}_{\mathbf {T}}(X;Y)$
.
Let A and B be nonzero objects of
![]() $\mathbf {T}$
. Fix an extension of A by B:
$\mathbf {T}$
. Fix an extension of A by B:
In this note, we prove some analogues of Schur’s lemma for
![]() $\mathrm{End}_{\mathbf {T}}(E;B)$
.
$\mathrm{End}_{\mathbf {T}}(E;B)$
.
The extension (1.1) induces a homomorphism of algebras
where given
![]() $\phi \in \mathrm{End}_{\mathbf {T}}(E;B)$
, its image
$\phi \in \mathrm{End}_{\mathbf {T}}(E;B)$
, its image
![]() $(\phi _B,\phi _A)$
is characterised by the commutativity of
$(\phi _B,\phi _A)$
is characterised by the commutativity of

The image of
![]() $\Omega $
always contains the diagonal copy of K in
$\Omega $
always contains the diagonal copy of K in
![]() $\mathrm{End}_{\mathbf {T}}(B)\times \mathrm{End}_{\mathbf {T}}(A)$
(as the image of scalar endomorphisms of E). Roughly speaking, it is natural to expect that the further away (1.1) is from splitting, the smaller the image of
$\mathrm{End}_{\mathbf {T}}(B)\times \mathrm{End}_{\mathbf {T}}(A)$
(as the image of scalar endomorphisms of E). Roughly speaking, it is natural to expect that the further away (1.1) is from splitting, the smaller the image of
![]() $\Omega $
should be. We shall prove two results in this spirit. The first is the following theorem.
$\Omega $
should be. We shall prove two results in this spirit. The first is the following theorem.
Theorem 1.1. Let
![]() ${\mathfrak {u}}(E)$
be the Lie algebra of the kernel of the homomorphism from the Tannakian group of E to the Tannakian group of
${\mathfrak {u}}(E)$
be the Lie algebra of the kernel of the homomorphism from the Tannakian group of E to the Tannakian group of
![]() $A\oplus B$
, naturally considered as a subobject of the internal Hom object
$A\oplus B$
, naturally considered as a subobject of the internal Hom object
![]() $\mathrm{Hom}(A,B)$
(see Section 2 for details). Assume that
$\mathrm{Hom}(A,B)$
(see Section 2 for details). Assume that
![]() ${\mathfrak {u}}(E)=\mathrm{Hom}(A,B)$
. Then the image of
${\mathfrak {u}}(E)=\mathrm{Hom}(A,B)$
. Then the image of
![]() $\Omega $
is equal to the diagonal copy of K.
$\Omega $
is equal to the diagonal copy of K.
Hardouin [Reference Hardouin8, Reference Hardouin, Di Vizio and Rivoal9] (in the case where A and B are semisimple) and the author and Kumar Murty [Reference Eskandari and Kumar Murty5] (for arbitrary possibly nonsemisimple A and B) give a characterisation of the subobject
![]() ${\mathfrak {u}}(E)$
of
${\mathfrak {u}}(E)$
of
![]() $\mathrm{Hom}(A,B)$
. A summary of this characterisation is recalled in Section 2. It follows from this characterisation that the condition
$\mathrm{Hom}(A,B)$
. A summary of this characterisation is recalled in Section 2. It follows from this characterisation that the condition
![]() ${\mathfrak {u}}(E)=\mathrm{Hom}(A,B)$
, which we refer to as the maximality of
${\mathfrak {u}}(E)=\mathrm{Hom}(A,B)$
, which we refer to as the maximality of
![]() ${\mathfrak {u}}(E)$
, implies that the extension class
${\mathfrak {u}}(E)$
, implies that the extension class
(where
![]() $\mathrm{Ext}^1_{\mathbf {T}}$
is the
$\mathrm{Ext}^1_{\mathbf {T}}$
is the
![]() $\mathrm{Ext}^1$
group in
$\mathrm{Ext}^1$
group in
![]() $\mathbf {T}$
and
$\mathbf {T}$
and
is the unit object) corresponding to (1.1) under the canonical isomorphism
is totally nonsplit, that is, for any proper subobject C of
![]() $\mathrm{Hom}(A,B)$
, the pushforward of
$\mathrm{Hom}(A,B)$
, the pushforward of
![]() $\mathcal {E}$
along the quotient
$\mathcal {E}$
along the quotient
![]() $\mathrm{Hom}(A,B)\rightarrow \mathrm{Hom}(A,B)/C$
is nonsplit. (Equivalently, an extension
$\mathrm{Hom}(A,B)\rightarrow \mathrm{Hom}(A,B)/C$
is nonsplit. (Equivalently, an extension
is totally nonsplit if the only subobject of Y that is mapped onto
is Y.)
In the case where A and B are semisimple, the maximality of
![]() ${\mathfrak {u}}(E)$
is equivalent to the total nonsplitting of
${\mathfrak {u}}(E)$
is equivalent to the total nonsplitting of
![]() $\mathcal {E}$
. However, in general, the two conditions are not equivalent, as the examples in Section 5 illustrate. The second result of the paper asserts that in some important settings, one can relax the hypothesis of Theorem 1.1 from assuming maximality of
$\mathcal {E}$
. However, in general, the two conditions are not equivalent, as the examples in Section 5 illustrate. The second result of the paper asserts that in some important settings, one can relax the hypothesis of Theorem 1.1 from assuming maximality of
![]() ${\mathfrak {u}}(E)$
to assuming total nonsplitting of
${\mathfrak {u}}(E)$
to assuming total nonsplitting of
![]() $\mathcal {E}$
.
$\mathcal {E}$
.
A Tannakian category
![]() $\mathbf {T}$
is said to be filtered if it is equipped with a filtration
$\mathbf {T}$
is said to be filtered if it is equipped with a filtration
![]() $W_\bullet $
satisfying similar properties to the weight filtration on mixed Hodge structures, that is,
$W_\bullet $
satisfying similar properties to the weight filtration on mixed Hodge structures, that is,
![]() $W_\bullet $
is indexed by
$W_\bullet $
is indexed by
![]() ${\mathbb Z}$
, functorial, exact, increasing, finite on every object and respects the tensor structure. This means that for every integer n, we have an exact linear functor
${\mathbb Z}$
, functorial, exact, increasing, finite on every object and respects the tensor structure. This means that for every integer n, we have an exact linear functor
![]() $W_n:\mathbf {T}\rightarrow \mathbf {T}$
such that for every object X of
$W_n:\mathbf {T}\rightarrow \mathbf {T}$
such that for every object X of
![]() $\mathbf {T}$
,
$\mathbf {T}$
,
 $$ \begin{align*} W_{n-1}X \ &\subset \ W_nX \quad (\mbox{for all } n),\\ W_nX \ &= \ 0 \quad (\mbox{for all } n\ll0),\\ W_nX \ &= \ X \quad (\mbox{for all } n\gg 0), \end{align*} $$
$$ \begin{align*} W_{n-1}X \ &\subset \ W_nX \quad (\mbox{for all } n),\\ W_nX \ &= \ 0 \quad (\mbox{for all } n\ll0),\\ W_nX \ &= \ X \quad (\mbox{for all } n\gg 0), \end{align*} $$
and such that the inclusions
![]() $W_nX\subset X$
for various X give a morphism of functors from
$W_nX\subset X$
for various X give a morphism of functors from
![]() $W_n$
to the identity. Compatibility with the tensor structure means that for all objects X and Y and every n,
$W_n$
to the identity. Compatibility with the tensor structure means that for all objects X and Y and every n,
 $$ \begin{align*} W_n(X\otimes Y) = \sum\limits_{p+q=n}W_p(X)\otimes W_q(Y). \end{align*} $$
$$ \begin{align*} W_n(X\otimes Y) = \sum\limits_{p+q=n}W_p(X)\otimes W_q(Y). \end{align*} $$
We will refer to
![]() $W_\bullet $
as the weight filtration. By the weights of an object X, we mean the integers n such that
$W_\bullet $
as the weight filtration. By the weights of an object X, we mean the integers n such that
![]() $W_nX/W_{n-1}X$
is not zero. The associated grading of X is defined to be
$W_nX/W_{n-1}X$
is not zero. The associated grading of X is defined to be
![]() $Gr^W X:=\bigoplus _n W_nX/W_{n-1}X$
. The prototype examples of filtered Tannakian categories are various Tannakian categories of mixed motives and the category of mixed Hodge structures.
$Gr^W X:=\bigoplus _n W_nX/W_{n-1}X$
. The prototype examples of filtered Tannakian categories are various Tannakian categories of mixed motives and the category of mixed Hodge structures.
We can now state the second result of the paper.
Theorem 1.2. Suppose that
![]() $\mathbf {T}$
is a filtered Tannakian category with the weight filtration denoted by
$\mathbf {T}$
is a filtered Tannakian category with the weight filtration denoted by
![]() $W_\bullet $
. Suppose moreover that condition (i) or (ii) below holds:
$W_\bullet $
. Suppose moreover that condition (i) or (ii) below holds:
-
(i) the associated graded
 $Gr^W E$
is semisimple and there are no nonzero morphisms
$Gr^W E$
is semisimple and there are no nonzero morphisms
 $Gr^W A\rightarrow Gr^W B$
;
$Gr^W A\rightarrow Gr^W B$
; -
(ii) the sets of weights of A and B are disjoint.
Then if
![]() $\mathcal {E}$
(defined as above) is totally nonsplit, the image of
$\mathcal {E}$
(defined as above) is totally nonsplit, the image of
![]() $\Omega $
will be equal to the diagonal copy of K.
$\Omega $
will be equal to the diagonal copy of K.
In any reasonable category of mixed motives,
![]() $Gr^WE$
is always semisimple. In the category of mixed Hodge structures,
$Gr^WE$
is always semisimple. In the category of mixed Hodge structures,
![]() $Gr^WE$
is semisimple if E is graded polarisable. Of course, it is only useful to include condition (ii) as a separate condition in the statement if
$Gr^WE$
is semisimple if E is graded polarisable. Of course, it is only useful to include condition (ii) as a separate condition in the statement if
![]() $Gr^WE$
is not known to be semisimple.
$Gr^WE$
is not known to be semisimple.
Theorem 1.2 is used crucially in [Reference Eskandari4], where we give a classification result for mixed motives with maximal unipotent radicals of motivic Galois groups and a given associated grading with respect to the weight filtration. Note that the assertion of Theorem 1.2 can be equivalently replaced by
that is, every element of
![]() $\mathrm{End}_{\mathbf {T}}(E;B)$
is a scalar endomorphisms of E. Indeed, the kernel of
$\mathrm{End}_{\mathbf {T}}(E;B)$
is a scalar endomorphisms of E. Indeed, the kernel of
![]() $\Omega $
is canonically isomorphic to
$\Omega $
is canonically isomorphic to
![]() $\mathrm{Hom}_{\mathbf {T}}(A,B)$
, where
$\mathrm{Hom}_{\mathbf {T}}(A,B)$
, where
![]() $\mathrm{Hom}_{\mathbf {T}}$
is the Hom group in
$\mathrm{Hom}_{\mathbf {T}}$
is the Hom group in
![]() $\mathbf {T}$
. Since the functor that sends an object X to
$\mathbf {T}$
. Since the functor that sends an object X to
![]() $Gr^WX$
is faithful, under condition (i) or (ii) of Theorem 1.2,
$Gr^WX$
is faithful, under condition (i) or (ii) of Theorem 1.2,
![]() $\mathrm{Hom}_{\mathbf {T}}(A,B)$
will be zero.
$\mathrm{Hom}_{\mathbf {T}}(A,B)$
will be zero.
Below, we first recall the characterisation of
![]() ${\mathfrak {u}}(E)$
mentioned above and then prove Theorems 1.1 and 1.2. The final section of the note includes some further remarks. In particular, we give an example that shows that in the general setting of Theorem 1.1, one cannot relax the maximality condition to total nonsplitting. Also, we discuss an example involving 1-motives that shows that in the setting where
${\mathfrak {u}}(E)$
mentioned above and then prove Theorems 1.1 and 1.2. The final section of the note includes some further remarks. In particular, we give an example that shows that in the general setting of Theorem 1.1, one cannot relax the maximality condition to total nonsplitting. Also, we discuss an example involving 1-motives that shows that in the setting where
![]() $\mathbf {T}$
is filtered and the sets of weights of A and B are disjoint, the total nonsplitting of
$\mathbf {T}$
is filtered and the sets of weights of A and B are disjoint, the total nonsplitting of
![]() $\mathcal {E}$
does not imply maximality of
$\mathcal {E}$
does not imply maximality of
![]() ${\mathfrak {u}}(E)$
, so that in this setting, the second theorem is indeed stronger than the first one. We also discuss a generalisation of Theorem 1.2 (see Section 5).
${\mathfrak {u}}(E)$
, so that in this setting, the second theorem is indeed stronger than the first one. We also discuss a generalisation of Theorem 1.2 (see Section 5).
2 Recollections on Tannakian groups of extensions
To simplify the notation, we fix a choice of fibre functor and identify
![]() $\mathbf {T}$
with the category of finite dimensional (algebraic) representations of an affine group scheme
$\mathbf {T}$
with the category of finite dimensional (algebraic) representations of an affine group scheme
![]() $\mathcal {G}$
over K (with
$\mathcal {G}$
over K (with
![]() $\mathcal {G}$
= the Tannakian group of
$\mathcal {G}$
= the Tannakian group of
![]() $\mathbf {T}$
with respect to the fibre functor). We will use the same symbol for an object of
$\mathbf {T}$
with respect to the fibre functor). We will use the same symbol for an object of
![]() $\mathbf {T}$
and its underlying vector space. For any object X of
$\mathbf {T}$
and its underlying vector space. For any object X of
![]() $\mathbf {T}$
and any
$\mathbf {T}$
and any
![]() $g\in \mathcal {G}$
, we denote the image of g in
$g\in \mathcal {G}$
, we denote the image of g in
![]() $\mathrm{GL}(X)$
by
$\mathrm{GL}(X)$
by
![]() $g_X$
. The image of
$g_X$
. The image of
![]() $\mathcal {G}$
in
$\mathcal {G}$
in
![]() $\mathrm{GL}(X)$
is denoted by
$\mathrm{GL}(X)$
is denoted by
![]() $\mathcal {G}(X)$
; this is the Tannakian group of the Tannakian subcategory
$\mathcal {G}(X)$
; this is the Tannakian group of the Tannakian subcategory
![]() $\langle X\rangle $
generated by X. (Recall that
$\langle X\rangle $
generated by X. (Recall that
![]() $\langle X\rangle $
is the smallest full Tannakian subcategory of
$\langle X\rangle $
is the smallest full Tannakian subcategory of
![]() $\mathbf {T}$
that contains X and is closed under taking subquotients.)
$\mathbf {T}$
that contains X and is closed under taking subquotients.)
We should point out that even though we think of
![]() $\mathbf {T}$
as the category of representations of
$\mathbf {T}$
as the category of representations of
![]() $\mathcal {G}$
, all the objects in
$\mathcal {G}$
, all the objects in
![]() $\mathbf {T}$
that appear in the following text (in particular, the object
$\mathbf {T}$
that appear in the following text (in particular, the object
![]() ${\mathfrak {u}}(E)$
introduced below) will be intrinsic to the Tannakian category
${\mathfrak {u}}(E)$
introduced below) will be intrinsic to the Tannakian category
![]() $\mathbf {T}$
. For this reason, we often prefer to use the terms object and subobject (
$\mathbf {T}$
. For this reason, we often prefer to use the terms object and subobject (
![]() $=$
object and subobject in
$=$
object and subobject in
![]() $\mathbf {T}$
) instead of the terms
$\mathbf {T}$
) instead of the terms
![]() $\mathcal {G}$
-representation and
$\mathcal {G}$
-representation and
![]() $\mathcal {G}$
-subrepresentation.
$\mathcal {G}$
-subrepresentation.
As they were in Section 1, the Ext and Hom groups in
![]() $\mathbf {T}$
are denoted by
$\mathbf {T}$
are denoted by
![]() $\mathrm{Ext}_{\mathbf {T}}$
and
$\mathrm{Ext}_{\mathbf {T}}$
and
![]() $\mathrm{Hom}_{\mathbf {T}}$
. We use the notation
$\mathrm{Hom}_{\mathbf {T}}$
. We use the notation
![]() $\mathrm{Hom}$
and
$\mathrm{Hom}$
and
![]() $\mathrm{End}$
(without any decorations) to refer to the Hom and End groups in the category of vector spaces. As we have adopted the convention of denoting an object of
$\mathrm{End}$
(without any decorations) to refer to the Hom and End groups in the category of vector spaces. As we have adopted the convention of denoting an object of
![]() $\mathbf {T}$
and its underlying vector space by the same symbol, for any objects X and Y of
$\mathbf {T}$
and its underlying vector space by the same symbol, for any objects X and Y of
![]() $\mathbf {T}$
, the notation
$\mathbf {T}$
, the notation
![]() $\mathrm{Hom}(X,Y)$
will refer to both the internal Hom (which is an object of
$\mathrm{Hom}(X,Y)$
will refer to both the internal Hom (which is an object of
![]() $\mathbf {T}$
) and the Hom space in the category of vector spaces between the underlying vector spaces. This should not lead to confusion as the relevant interpretation will be clear from the context.
$\mathbf {T}$
) and the Hom space in the category of vector spaces between the underlying vector spaces. This should not lead to confusion as the relevant interpretation will be clear from the context.
Given a vector space X and a subspace Y of X, denote the subalgebra of
![]() $\mathrm{End}(X)$
consisting of linear maps on X which map Y to Y by
$\mathrm{End}(X)$
consisting of linear maps on X which map Y to Y by
![]() $\mathrm{End}(X;Y)$
. Similarly, the subgroup of
$\mathrm{End}(X;Y)$
. Similarly, the subgroup of
![]() $\mathrm{GL}(X)$
consisting of the elements which map Y to itself is denoted by
$\mathrm{GL}(X)$
consisting of the elements which map Y to itself is denoted by
![]() $\mathrm{GL}(X;Y)$
. Given an object X of any category, the identity map on X is denoted by
$\mathrm{GL}(X;Y)$
. Given an object X of any category, the identity map on X is denoted by
![]() $\mathrm{Id}_X$
. We will sometimes simply write
$\mathrm{Id}_X$
. We will sometimes simply write
![]() $\mathrm{Id}$
if X is clear from the context.
$\mathrm{Id}$
if X is clear from the context.
Fix objects A, B and E of
![]() $\mathbf {T}$
and the exact sequence (1.1). Let
$\mathbf {T}$
and the exact sequence (1.1). Let
![]() $\mathcal {U}(E)$
be the kernel of the natural map
$\mathcal {U}(E)$
be the kernel of the natural map
Choosing a section of
![]() $E\twoheadrightarrow B$
in the category of vector spaces to identify
$E\twoheadrightarrow B$
in the category of vector spaces to identify
as vector spaces, we have an embedding
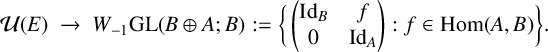 $$ \begin{align*} \mathcal{U}(E) \ \rightarrow \ W_{-1}\mathrm{GL}(B\oplus A;B):=\bigg\{\begin{pmatrix} \mathrm{Id}_B & f \\ 0 &\mathrm{Id}_A\end{pmatrix}: f\in \mathrm{Hom}(A,B)\bigg\}. \end{align*} $$
$$ \begin{align*} \mathcal{U}(E) \ \rightarrow \ W_{-1}\mathrm{GL}(B\oplus A;B):=\bigg\{\begin{pmatrix} \mathrm{Id}_B & f \\ 0 &\mathrm{Id}_A\end{pmatrix}: f\in \mathrm{Hom}(A,B)\bigg\}. \end{align*} $$
The group
![]() $W_{-1}\mathrm{GL}(B\oplus A;B)$
is unipotent and abelian, and hence so is
$W_{-1}\mathrm{GL}(B\oplus A;B)$
is unipotent and abelian, and hence so is
![]() $\mathcal {U}(E)$
. Since
$\mathcal {U}(E)$
. Since
![]() $W_{-1}\mathrm{GL}(B\oplus A;B)$
is abelian, the embedding above is actually canonical, that is, it does not depend on the choice of the section of
$W_{-1}\mathrm{GL}(B\oplus A;B)$
is abelian, the embedding above is actually canonical, that is, it does not depend on the choice of the section of
![]() $E \twoheadrightarrow A$
used to identify
$E \twoheadrightarrow A$
used to identify
![]() $E=B\oplus A$
.
$E=B\oplus A$
.
Let
![]() ${\mathfrak {u}}(E)$
be the Lie algebra of
${\mathfrak {u}}(E)$
be the Lie algebra of
![]() $\mathcal {U}(E)$
. Then
$\mathcal {U}(E)$
. Then
![]() ${\mathfrak {u}}(E)$
can be identified as a subspace of
${\mathfrak {u}}(E)$
can be identified as a subspace of
![]() $\mathrm{Hom}(A,B)$
with the exponential map
$\mathrm{Hom}(A,B)$
with the exponential map
![]() ${\mathfrak {u}}(E) \rightarrow \mathcal {U}(E)$
simply sending
${\mathfrak {u}}(E) \rightarrow \mathcal {U}(E)$
simply sending
 $$ \begin{align*} f\in {\mathfrak{u}}(E)\subset \mathrm{Hom}(A,B) \quad \mathrm{to} \quad \begin{pmatrix} \mathrm{Id} & f \\ 0 &\mathrm{Id}\end{pmatrix}. \end{align*} $$
$$ \begin{align*} f\in {\mathfrak{u}}(E)\subset \mathrm{Hom}(A,B) \quad \mathrm{to} \quad \begin{pmatrix} \mathrm{Id} & f \\ 0 &\mathrm{Id}\end{pmatrix}. \end{align*} $$
Through the adjoint representation of
![]() $\mathcal {G}(E)$
on
$\mathcal {G}(E)$
on
![]() ${\mathfrak {u}}(E)$
, the Lie algebra
${\mathfrak {u}}(E)$
, the Lie algebra
![]() ${\mathfrak {u}}(E)$
is naturally equipped with a
${\mathfrak {u}}(E)$
is naturally equipped with a
![]() $\mathcal {G}$
-action. The inclusion
$\mathcal {G}$
-action. The inclusion
![]() ${\mathfrak {u}}(E)\subset \mathrm{Hom}(A,B)$
is compatible with the
${\mathfrak {u}}(E)\subset \mathrm{Hom}(A,B)$
is compatible with the
![]() $\mathcal {G}$
-actions, making
$\mathcal {G}$
-actions, making
![]() ${\mathfrak {u}}(E)$
a subobject of the internal Hom,
${\mathfrak {u}}(E)$
a subobject of the internal Hom,
![]() $\mathrm{Hom}(A,B)$
(see [Reference Eskandari and Kumar Murty5, Section 3.1], for instance). This subobject has a nice description, which we recall now.
$\mathrm{Hom}(A,B)$
(see [Reference Eskandari and Kumar Murty5, Section 3.1], for instance). This subobject has a nice description, which we recall now.
As in Section 1, let
be the element corresponding to the class of (1.1) under the canonical isomorphism
For any subobject
![]() $C\subset \mathrm{Hom}(A,B)$
, the pushforward of
$C\subset \mathrm{Hom}(A,B)$
, the pushforward of
![]() $\mathcal {E}$
along the quotient map
$\mathcal {E}$
along the quotient map
is denoted by
![]() $\mathcal {E}/C$
. The following characterisation of
$\mathcal {E}/C$
. The following characterisation of
![]() ${\mathfrak {u}}(E)$
was proved in [Reference Eskandari and Kumar Murty5].
${\mathfrak {u}}(E)$
was proved in [Reference Eskandari and Kumar Murty5].
Theorem 2.1 ([Reference Eskandari and Kumar Murty5, Theorem 3.3.1]; see also [Reference Eskandari and Kumar Murty6, Lemma 3.4.3]).
Given any subobject C of
![]() $\mathrm{Hom}(A,B)$
, we have
$\mathrm{Hom}(A,B)$
, we have
![]() ${\mathfrak {u}}(E)\subset C$
if and only if the pushforward
${\mathfrak {u}}(E)\subset C$
if and only if the pushforward
is in the image of the natural injection
where
![]() $\mathrm{Ext}^1_{\langle A\oplus B\rangle }$
is the
$\mathrm{Ext}^1_{\langle A\oplus B\rangle }$
is the
![]() $\mathrm{Ext}^1$
group in the Tannakian subcategory
$\mathrm{Ext}^1$
group in the Tannakian subcategory
![]() $\langle A\oplus B\rangle $
of
$\langle A\oplus B\rangle $
of
![]() $\mathbf {T}$
generated by
$\mathbf {T}$
generated by
![]() $A\oplus B$
. (Thus,
$A\oplus B$
. (Thus,
![]() ${\mathfrak {u}}(E)$
is the smallest subobject of
${\mathfrak {u}}(E)$
is the smallest subobject of
![]() $\mathrm{Hom}(A,B)$
with this property.)
$\mathrm{Hom}(A,B)$
with this property.)
In the case where A and B are semisimple, this was earlier proved by Bertrand [Reference Bertrand2] in the setting of D-modules and by Hardouin [Reference Hardouin8, Reference Hardouin, Di Vizio and Rivoal9] in the setting of arbitrary Tannakian categories. In this case, the statement simplifies to the following theorem.
Theorem 2.2 [Reference Hardouin, Di Vizio and Rivoal9, Theorem 2].
Suppose A and B are semisimple. Let
![]() $\mathcal {E}$
be as above. Then given any subobject C of
$\mathcal {E}$
be as above. Then given any subobject C of
![]() $\mathrm{Hom}(A,B)$
, we have
$\mathrm{Hom}(A,B)$
, we have
![]() ${\mathfrak {u}}(E)\subset C$
if and only if the pushforward
${\mathfrak {u}}(E)\subset C$
if and only if the pushforward
![]() $\mathcal {E}/C$
splits.
$\mathcal {E}/C$
splits.
In the general case (where A or B may not be semisimple), by Theorem 2.1, if C is any subobject of
![]() $\mathrm{Hom}(A,B)$
such that
$\mathrm{Hom}(A,B)$
such that
![]() $\mathcal {E}/C$
splits, then C contains
$\mathcal {E}/C$
splits, then C contains
![]() ${\mathfrak {u}}(E)$
. The pushforward
${\mathfrak {u}}(E)$
. The pushforward
![]() $\mathcal {E}/{\mathfrak {u}}(E)$
however may not split (see the examples in Section 5).
$\mathcal {E}/{\mathfrak {u}}(E)$
however may not split (see the examples in Section 5).
We also recall an explicit description of
![]() $\mathcal {E}$
(see [Reference Eskandari and Kumar Murty5, Section 3.2] for details). Let
$\mathcal {E}$
(see [Reference Eskandari and Kumar Murty5, Section 3.2] for details). Let
 $$ \begin{align*} \mathrm{Hom}(A,E)^\dagger := \bigg\{f\in \mathrm{Hom}(A,E): \begin{array}{ll} \mathrm{the\ composition}\ {A\xrightarrow{f}E\twoheadrightarrow A}\\ \mathrm{is\ a\ scalar\ multiple\ of}\ {\mathrm{Id}_A}\end{array}\!\!\bigg\}. \end{align*} $$
$$ \begin{align*} \mathrm{Hom}(A,E)^\dagger := \bigg\{f\in \mathrm{Hom}(A,E): \begin{array}{ll} \mathrm{the\ composition}\ {A\xrightarrow{f}E\twoheadrightarrow A}\\ \mathrm{is\ a\ scalar\ multiple\ of}\ {\mathrm{Id}_A}\end{array}\!\!\bigg\}. \end{align*} $$
It is easy to see that this is a subobject of
![]() $\mathrm{Hom}(A,E)$
. The element
$\mathrm{Hom}(A,E)$
. The element
![]() $\mathcal {E}$
is the class of the extension
$\mathcal {E}$
is the class of the extension
Here, the injective map is simply the obvious embedding, sending
![]() $f\in \mathrm{Hom}(A,B)$
to
$f\in \mathrm{Hom}(A,B)$
to
The surjective map in (2.2) is the map that sends
![]() $f\in \mathrm{Hom}(A,E)^\dagger $
to
$f\in \mathrm{Hom}(A,E)^\dagger $
to
![]() $a\in K$
, where
$a\in K$
, where
is
![]() $a\cdot \mathrm{Id}_A$
.
$a\cdot \mathrm{Id}_A$
.
3 Proofs of Theorems 1.1 and 1.2 for
 $A=\mathbb{1}$
$A=\mathbb{1}$
The goal of this section is to prove Theorems 1.1 and 1.2 in the case where
![]() ; the general case will be deduced from this in the next section. In this case, identifying
; the general case will be deduced from this in the next section. In this case, identifying
![]() , the extension
, the extension
![]() $\mathcal {E}$
is simply given by (1.1). Theorem 2.1 asserts that
$\mathcal {E}$
is simply given by (1.1). Theorem 2.1 asserts that
![]() ${\mathfrak {u}}(E)$
is the smallest subobject of B such that
${\mathfrak {u}}(E)$
is the smallest subobject of B such that
![]() $\mathcal {E}/{\mathfrak {u}}(E)$
is an extension of
$\mathcal {E}/{\mathfrak {u}}(E)$
is an extension of
![]() by
by
![]() $B/{\mathfrak {u}}(E)$
in the subcategory
$B/{\mathfrak {u}}(E)$
in the subcategory
![]() $\langle B\rangle $
. If B is semisimple,
$\langle B\rangle $
. If B is semisimple,
![]() ${\mathfrak {u}}(E)$
is the smallest subobject of B such that
${\mathfrak {u}}(E)$
is the smallest subobject of B such that
![]() $\mathcal {E}/{\mathfrak {u}}(E)$
splits.
$\mathcal {E}/{\mathfrak {u}}(E)$
splits.
We first establish a lemma.
Lemma 3.1. Assume
![]() . Let
. Let
![]() $\lambda : E\rightarrow B_0$
be a morphism from E to an object
$\lambda : E\rightarrow B_0$
be a morphism from E to an object
![]() $B_0$
, such that
$B_0$
, such that
![]() $B_0$
belongs to the subcategory
$B_0$
belongs to the subcategory
![]() $\langle B\rangle $
. Then
$\langle B\rangle $
. Then
![]() ${\mathfrak {u}}(E)\subset B\cap \ker (\lambda )$
.
${\mathfrak {u}}(E)\subset B\cap \ker (\lambda )$
.
Proof. Set
![]() $B':=B\cap \ker (\lambda )$
. Consider the commutative diagram
$B':=B\cap \ker (\lambda )$
. Consider the commutative diagram

where the maps are inclusions and quotient maps. The rows and columns are exact.
Case I. Suppose
![]() $\ker (\lambda )\not \subset B$
, so that
$\ker (\lambda )\not \subset B$
, so that
![]() $B'$
is a proper subobject of
$B'$
is a proper subobject of
![]() $\ker (\lambda )$
. Being a nonzero subobject of the unit object,
$\ker (\lambda )$
. Being a nonzero subobject of the unit object,
![]() $\ker (\lambda )/B'$
must be isomorphic to
$\ker (\lambda )/B'$
must be isomorphic to
![]() . Thus,
. Thus,
![]() $\mathcal {E}$
(the second row) is the pushforward of an extension of
$\mathcal {E}$
(the second row) is the pushforward of an extension of
![]() by
by
![]() $B'$
(the first row). It follows that
$B'$
(the first row). It follows that
![]() $\mathcal {E}/B'$
splits and
$\mathcal {E}/B'$
splits and
![]() ${\mathfrak {u}}(E)\subset B'$
by Theorem 2.1.
${\mathfrak {u}}(E)\subset B'$
by Theorem 2.1.
Case II. Suppose
![]() $\ker (\lambda )\subset B$
, so that
$\ker (\lambda )\subset B$
, so that
![]() $B'=\ker (\lambda )$
. Then the third row of the diagram is the pushforward
$B'=\ker (\lambda )$
. Then the third row of the diagram is the pushforward
![]() $\mathcal {E}/B'$
. By assumption,
$\mathcal {E}/B'$
. By assumption,
![]() $E/\ker (\lambda )$
is in the subcategory generated by B. Again
$E/\ker (\lambda )$
is in the subcategory generated by B. Again
![]() ${\mathfrak {u}}(E)\subset B'$
by Theorem 2.1.
${\mathfrak {u}}(E)\subset B'$
by Theorem 2.1.
We can now establish Theorems 1.1 and 1.2 in the case that
![]() . Let
. Let
![]() $\phi \in \mathrm{End}_{\mathbf {T}}(E;B)$
. Then
$\phi \in \mathrm{End}_{\mathbf {T}}(E;B)$
. Then
![]() (= the induced map on
(= the induced map on
![]() by
by
![]() $\phi $
) is equal to
$\phi $
) is equal to
![]() for some
for some
![]() $a\in K$
. The endomorphism
$a\in K$
. The endomorphism
![]() $\lambda :=\phi -a \cdot \mathrm{Id}_E$
of E then factors through B, that is, it is the composition with the inclusion
$\lambda :=\phi -a \cdot \mathrm{Id}_E$
of E then factors through B, that is, it is the composition with the inclusion
![]() $B\hookrightarrow E$
of a morphism
$B\hookrightarrow E$
of a morphism
![]() $E\rightarrow B$
, which we also denote by
$E\rightarrow B$
, which we also denote by
![]() $\lambda $
.
$\lambda $
.
To obtain Theorem 1.1, apply the previous lemma to
![]() $\lambda $
. We get
$\lambda $
. We get
![]() ${\mathfrak {u}}(E)\subset B\cap \ker (\lambda )$
. The assumption that
${\mathfrak {u}}(E)\subset B\cap \ker (\lambda )$
. The assumption that
![]() ${\mathfrak {u}}(E)=B$
gives
${\mathfrak {u}}(E)=B$
gives
![]() $B\subset \ker (\lambda )$
, that is,
$B\subset \ker (\lambda )$
, that is,
![]() $\phi =a\cdot \mathrm{Id}$
on B, as desired.
$\phi =a\cdot \mathrm{Id}$
on B, as desired.
We now turn our attention to Theorem 1.2. Thus, we assume that
![]() $\mathbf {T}$
is a filtered Tannakian category and that either (i)
$\mathbf {T}$
is a filtered Tannakian category and that either (i)
![]() $Gr^WE$
is semisimple and
$Gr^WE$
is semisimple and
or (ii) 0 is not a weight of B (note that
is pure of weight 0). Both conditions guarantee that the kernel of
![]() $\lambda : E\rightarrow B$
cannot be contained in B. Indeed, this follows simply by weight considerations if item (ii) holds. On the other hand, if item (i) holds, after applying the associated graded functor, the sequence (1.1) splits. Choosing a section for the sequence (which will be unique because
$\lambda : E\rightarrow B$
cannot be contained in B. Indeed, this follows simply by weight considerations if item (ii) holds. On the other hand, if item (i) holds, after applying the associated graded functor, the sequence (1.1) splits. Choosing a section for the sequence (which will be unique because
vanishes), if
![]() $\ker (\lambda )\subset B$
, we have a diagram
$\ker (\lambda )\subset B$
, we have a diagram

(with obvious maps and the row being exact). We thus get a nonzero morphism
.
Thus,
![]() $B':=B\cap \ker (\lambda )$
is a proper subobject of
$B':=B\cap \ker (\lambda )$
is a proper subobject of
![]() $\ker (\lambda )$
. Considering the diagram (3.1) with
$\ker (\lambda )$
. Considering the diagram (3.1) with
![]() $B'$
and
$B'$
and
![]() $\lambda :E\rightarrow B$
as described, the extension
$\lambda :E\rightarrow B$
as described, the extension
![]() $\mathcal {E}$
is the pushforward of the top row along the inclusion
$\mathcal {E}$
is the pushforward of the top row along the inclusion
![]() $B'\hookrightarrow B$
, so that
$B'\hookrightarrow B$
, so that
![]() $\mathcal {E}/B'$
splits. If
$\mathcal {E}/B'$
splits. If
![]() $\mathcal {E}$
is totally nonsplit, we get
$\mathcal {E}$
is totally nonsplit, we get
![]() $B'=B$
and
$B'=B$
and
![]() $B\subset \ker (\lambda )$
. Thus, we have also established Theorem 1.2 when
$B\subset \ker (\lambda )$
. Thus, we have also established Theorem 1.2 when
![]() .
.
4 Proofs of Theorems 1.1 and 1.2 for arbitrary A
We now assume that A is arbitrary. The extension
![]() $\mathcal {E}$
is now given by (2.2). Assume the hypotheses of Theorems 1.1 or 1.2 for the extension given in (1.1). Then the hypotheses also hold for the extension given in (2.2), that is, if (2.2) is taken as our original (1.1). To see this for Theorem 1.1, note that in view of Theorem 2.1, we have
$\mathcal {E}$
is now given by (2.2). Assume the hypotheses of Theorems 1.1 or 1.2 for the extension given in (1.1). Then the hypotheses also hold for the extension given in (2.2), that is, if (2.2) is taken as our original (1.1). To see this for Theorem 1.1, note that in view of Theorem 2.1, we have
![]() ${\mathfrak {u}}(E)\subset {\mathfrak {u}}(\mathrm{Hom}(A,E)^\dagger )$
, as the subcategory
${\mathfrak {u}}(E)\subset {\mathfrak {u}}(\mathrm{Hom}(A,E)^\dagger )$
, as the subcategory
![]() $\langle \mathrm{Hom}(A,B)\rangle $
is contained in
$\langle \mathrm{Hom}(A,B)\rangle $
is contained in
![]() $\langle A\oplus B\rangle $
; to see it for Theorem 1.2, note that
$\langle A\oplus B\rangle $
; to see it for Theorem 1.2, note that
Thus, by the special case already proved, the image of the map
induced by (2.2) is the diagonal copy of K. Hence, the general case of the results will be established if we prove the following lemma.
Proof. Let
![]() $\phi \in \mathrm{End}_{\mathbf {T}}(E;B)$
. We will show that
$\phi \in \mathrm{End}_{\mathbf {T}}(E;B)$
. We will show that
![]() $(\phi _B,\phi _A)$
is in the diagonal copy of K. Adding a suitable scalar multiple of
$(\phi _B,\phi _A)$
is in the diagonal copy of K. Adding a suitable scalar multiple of
![]() $\mathrm{Id}_E$
to
$\mathrm{Id}_E$
to
![]() $\phi $
if necessary, we may assume that
$\phi $
if necessary, we may assume that
![]() $\phi $
is an automorphism (recall that K is of characteristic zero). Let
$\phi $
is an automorphism (recall that K is of characteristic zero). Let
![]() $\phi ^\dagger \in \mathrm{End}(\mathrm{Hom}(A,E))$
be the map that sends any
$\phi ^\dagger \in \mathrm{End}(\mathrm{Hom}(A,E))$
be the map that sends any
![]() $f\in \mathrm{Hom}(A,E)$
to the composition
$f\in \mathrm{Hom}(A,E)$
to the composition
Since
![]() $\phi _A$
and
$\phi _A$
and
![]() $\phi $
are morphisms in
$\phi $
are morphisms in
![]() $\mathbf {T}$
, so is
$\mathbf {T}$
, so is
![]() $\phi ^\dagger $
. Since B is stable under
$\phi ^\dagger $
. Since B is stable under
![]() $\phi $
, the map
$\phi $
, the map
![]() $\phi ^\dagger $
stabilises
$\phi ^\dagger $
stabilises
![]() $\mathrm{Hom}(A,B)$
. Moreover, if f is in
$\mathrm{Hom}(A,B)$
. Moreover, if f is in
![]() $\mathrm{Hom}(A,E)^\dagger $
with
$\mathrm{Hom}(A,E)^\dagger $
with
![]() $f\pmod {B}=\mathrm{Id}_A$
, then we have a commutative diagram
$f\pmod {B}=\mathrm{Id}_A$
, then we have a commutative diagram

so that
![]() $\phi ^\dagger (f)$
is also in
$\phi ^\dagger (f)$
is also in
![]() $\mathrm{Hom}(A,E)^\dagger $
with
$\mathrm{Hom}(A,E)^\dagger $
with
![]() $\phi ^\dagger (f) \pmod {B} $
being the identity map on A. We conclude that:
$\phi ^\dagger (f) \pmod {B} $
being the identity map on A. We conclude that:
-
(i)
 $\phi ^\dagger $
restricts to an element of
$\phi ^\dagger $
restricts to an element of
 $\mathrm{End}_{\mathbf {T}}(\mathrm{Hom}(A,E)^\dagger ;\mathrm{Hom}(A,B))$
and
$\mathrm{End}_{\mathbf {T}}(\mathrm{Hom}(A,E)^\dagger ;\mathrm{Hom}(A,B))$
and -
(ii) denoting this restriction also by
 $\phi ^\dagger $
, we have
$\phi ^\dagger $
, we have
 (where
(where
 is the map induced on
is the map induced on
 by
by
 $\phi ^\dagger \in \mathrm{End}_{\mathbf {T}}(\mathrm{Hom}(A,E)^\dagger )$
).
$\phi ^\dagger \in \mathrm{End}_{\mathbf {T}}(\mathrm{Hom}(A,E)^\dagger )$
).
Since the image of (4.1) is the diagonal copy of K, it follows that the restriction of
![]() $\phi ^\dagger $
to
$\phi ^\dagger $
to
![]() $\mathrm{Hom}(A,B)$
is also the identity map. That is, for every linear map
$\mathrm{Hom}(A,B)$
is also the identity map. That is, for every linear map
![]() $f: A\rightarrow B$
,
$f: A\rightarrow B$
,
Since A and B are nonzero, by elementary linear algebra,
![]() $\phi _A$
and
$\phi _A$
and
![]() $\phi _B$
are both scalar maps and they are given by multiplication with the same element of K.
$\phi _B$
are both scalar maps and they are given by multiplication with the same element of K.
5 Further remarks
5.1 Sharpness of Theorem 1.1
If (1.1) is an arbitrary extension in a general Tannakian category
![]() $\mathbf {T}$
(with no extra assumptions on (1.1) or
$\mathbf {T}$
(with no extra assumptions on (1.1) or
![]() $\mathbf {T}$
), total nonsplitting of
$\mathbf {T}$
), total nonsplitting of
![]() $\mathcal {E}$
(= the extension of
$\mathcal {E}$
(= the extension of
![]() by
by
![]() $\mathrm{Hom}(A,B)$
corresponding to (1.1) under the canonical isomorphism) does not guarantee that the image of
$\mathrm{Hom}(A,B)$
corresponding to (1.1) under the canonical isomorphism) does not guarantee that the image of
![]() $\Omega $
is K. Thus, the hypothesis of maximality of
$\Omega $
is K. Thus, the hypothesis of maximality of
![]() ${\mathfrak {u}}(E)$
in Theorem 1.1 cannot be relaxed to total nonsplitting.
${\mathfrak {u}}(E)$
in Theorem 1.1 cannot be relaxed to total nonsplitting.
For example, given any field K of characteristic zero, let
![]() $\mathbf {T}$
be the category of finite dimensional algebraic representations of the subgroup
$\mathbf {T}$
be the category of finite dimensional algebraic representations of the subgroup
![]() $\mathcal {G}$
of
$\mathcal {G}$
of
![]() $\mathrm{GL}_3$
(over K) consisting of all the matrices of the form
$\mathrm{GL}_3$
(over K) consisting of all the matrices of the form
 $$ \begin{align*} \begin{pmatrix} 1&a&b\\ &1&a\\ &&1 \end{pmatrix}, \end{align*} $$
$$ \begin{align*} \begin{pmatrix} 1&a&b\\ &1&a\\ &&1 \end{pmatrix}, \end{align*} $$
where the missing entries are zero. Let B be
![]() $K^2$
with the action of
$K^2$
with the action of
![]() $\mathcal {G}$
given by left multiplication by the top left
$\mathcal {G}$
given by left multiplication by the top left
![]() $2\times 2$
submatrix, and let E be
$2\times 2$
submatrix, and let E be
![]() $K^3$
with the canonical action of
$K^3$
with the canonical action of
![]() $\mathcal {G}$
through left multiplication. We have an embedding
$\mathcal {G}$
through left multiplication. We have an embedding
![]() $B\hookrightarrow E$
given by
$B\hookrightarrow E$
given by
![]() $(x_1,x_2)\mapsto (x_1,x_2,0)$
, fitting into a short exact sequence
$(x_1,x_2)\mapsto (x_1,x_2,0)$
, fitting into a short exact sequence
with the map
being projection onto the third coordinate. It is easy to see that the extension above is totally nonsplit. However, E has an endomorphism
which stabilises B but its restriction to B is not a scalar multiple of the identity.
It is worth mentioning that here, by Theorem 1.1,
![]() ${\mathfrak {u}}(E)$
is not maximal, so this example also shows that, in general, total nonsplitting of
${\mathfrak {u}}(E)$
is not maximal, so this example also shows that, in general, total nonsplitting of
![]() $\mathcal {E}$
does not imply that
$\mathcal {E}$
does not imply that
![]() ${\mathfrak {u}}(E)$
must be maximal (in particular, in general,
${\mathfrak {u}}(E)$
must be maximal (in particular, in general,
![]() $\mathcal {E}/{\mathfrak {u}}(E)$
does not have to split). See the next subsection for a more interesting example that also illustrates this.
$\mathcal {E}/{\mathfrak {u}}(E)$
does not have to split). See the next subsection for a more interesting example that also illustrates this.
5.2 Sharpness of Theorem 1.2
Assume that
![]() $\mathbf {T}$
is filtered, and that A and B have disjoint sets of weights. Then total nonsplitting of
$\mathbf {T}$
is filtered, and that A and B have disjoint sets of weights. Then total nonsplitting of
![]() $\mathcal {E}$
still does not imply maximality of
$\mathcal {E}$
still does not imply maximality of
![]() ${\mathfrak {u}}(E)$
, so that Theorem 1.2 is indeed stronger than Theorem 1.1 in this setting. The example in [Reference Eskandari and Kumar Murty6, Section 6.10] using the work of Jacquinot and Ribet [Reference Jacquinot and Ribet10] on deficient points on semiabelian varieties illustrates this. If we take
${\mathfrak {u}}(E)$
, so that Theorem 1.2 is indeed stronger than Theorem 1.1 in this setting. The example in [Reference Eskandari and Kumar Murty6, Section 6.10] using the work of Jacquinot and Ribet [Reference Jacquinot and Ribet10] on deficient points on semiabelian varieties illustrates this. If we take
![]() $\mathbf {T}$
to be the category of mixed Hodge structures, E to be the 1-motive denoted by M in [Reference Eskandari and Kumar Murty6, Section 6.10], and
$\mathbf {T}$
to be the category of mixed Hodge structures, E to be the 1-motive denoted by M in [Reference Eskandari and Kumar Murty6, Section 6.10], and
![]() $B=W_{-1}M$
and
$B=W_{-1}M$
and
![]() , then the sequence (1.1) (given by the natural inclusion and quotient maps) is totally nonsplit, the weights of A and B are disjoint, and
, then the sequence (1.1) (given by the natural inclusion and quotient maps) is totally nonsplit, the weights of A and B are disjoint, and
![]() ${\mathfrak {u}}(E)$
(which is the same as
${\mathfrak {u}}(E)$
(which is the same as
![]() ${\mathfrak {u}}_{-1}(M)$
in [Reference Eskandari and Kumar Murty6, Section 6.10]) is not maximal. In fact,
${\mathfrak {u}}_{-1}(M)$
in [Reference Eskandari and Kumar Murty6, Section 6.10]) is not maximal. In fact,
![]() ${\mathfrak {u}}(E)=0$
(see [Reference Eskandari and Kumar Murty6]).
${\mathfrak {u}}(E)=0$
(see [Reference Eskandari and Kumar Murty6]).
Continuing to work in the category of mixed Hodge structures, here we include a somewhat simpler example which avoids using deficient points. Let J be a simple complex abelian variety of positive dimension. Let N be a nonsplit extension of
by
![]() $H_1(J)$
. Then
$H_1(J)$
. Then
is a nonsplit extension of
![]() $H^1(J)(1)$
by
$H^1(J)(1)$
by
![]() ${\mathbb Q}(1)$
, which after a choice of polarisation can be thought of as a nonsplit extension of
${\mathbb Q}(1)$
, which after a choice of polarisation can be thought of as a nonsplit extension of
![]() $H_1(J)$
by
$H_1(J)$
by
![]() ${\mathbb Q}(1)$
. Since the
${\mathbb Q}(1)$
. Since the
![]() $\mathrm{Ext}^2$
groups vanish in the category of mixed Hodge structures (see [Reference Beilinson, Bloch, Dennis, Friedlander and Stein1], for example), there is an object E fitting into the diagram
$\mathrm{Ext}^2$
groups vanish in the category of mixed Hodge structures (see [Reference Beilinson, Bloch, Dennis, Friedlander and Stein1], for example), there is an object E fitting into the diagram

in which the rows and columns are exact and the top row and the right column are our nonsplit extensions. (See [Reference Grothendieck, Deligne, Raynaud, Rim, Grothendieck and Raynaud7, Lemma 9.3.8] or [Reference Eskandari and Kumar Murty6, Lemma 6.4.1].)
Take the first vertical extension of the diagram to play the role of our (1.1); it will also be our
![]() $\mathcal {E}$
. Then
$\mathcal {E}$
. Then
![]() $\mathcal {E}$
is totally nonsplit, as
$\mathcal {E}$
is totally nonsplit, as
![]() ${\mathbb Q}(1)$
is the unique maximal proper subobject of
${\mathbb Q}(1)$
is the unique maximal proper subobject of
![]() and the pushforward
and the pushforward
![]() $\mathcal {E}/{\mathbb Q}(1)$
(= the right column) is nonsplit. However,
$\mathcal {E}/{\mathbb Q}(1)$
(= the right column) is nonsplit. However,
![]() $\mathcal {E}/{\mathbb Q}(1)$
is an extension in the subcategory generated by
$\mathcal {E}/{\mathbb Q}(1)$
is an extension in the subcategory generated by
![]() , hence by Theorem 2.1, we have
, hence by Theorem 2.1, we have
![]() ${\mathfrak {u}}(E)\subset {\mathbb Q}(1)$
. In particular,
${\mathfrak {u}}(E)\subset {\mathbb Q}(1)$
. In particular,
![]() ${\mathfrak {u}}(E)$
is not maximal.
${\mathfrak {u}}(E)$
is not maximal.
5.3 Generalisation of Theorem 1.2
In the proof of the case
![]() of Theorem 1.2, the only place where the filtration on
of Theorem 1.2, the only place where the filtration on
![]() $\mathbf {T}$
and condition (i) or (ii) played a part is when we concluded that the kernel of
$\mathbf {T}$
and condition (i) or (ii) played a part is when we concluded that the kernel of
![]() $\lambda :E\rightarrow B$
(with
$\lambda :E\rightarrow B$
(with
![]() $\lambda $
as in the proof) is not contained in B. Combining this remark with Lemma 4.1, we obtain the following generalisation of Theorem 1.2.
$\lambda $
as in the proof) is not contained in B. Combining this remark with Lemma 4.1, we obtain the following generalisation of Theorem 1.2.
Theorem 5.1. Let (1.1) be an extension in any Tannakian category
![]() $\mathbf {T}$
over a field of characteristic 0. Suppose that the kernel of any morphism
$\mathbf {T}$
over a field of characteristic 0. Suppose that the kernel of any morphism
is not contained in
![]() $\mathrm{Hom}(A,B)$
. Then if
$\mathrm{Hom}(A,B)$
. Then if
![]() $\mathcal {E}$
(that is, the extension of
$\mathcal {E}$
(that is, the extension of
![]() by
by
![]() $\mathrm{Hom}(A,B)$
corresponding to (1.1), as before) is totally nonsplit, the image of
$\mathrm{Hom}(A,B)$
corresponding to (1.1), as before) is totally nonsplit, the image of
![]() $\Omega $
will be the diagonal copy of K.
$\Omega $
will be the diagonal copy of K.
In particular, this can be applied in the following situation. Suppose
![]() $\mathcal {R}$
is a reductive subgroup of the group
$\mathcal {R}$
is a reductive subgroup of the group
![]() $\mathcal {G}(E)$
(= the Tannakian group of
$\mathcal {G}(E)$
(= the Tannakian group of
![]() $\langle E\rangle $
). Every object of
$\langle E\rangle $
). Every object of
![]() $\langle E\rangle $
can also be considered as an
$\langle E\rangle $
can also be considered as an
![]() $\mathcal {R}$
-representation. In the (semisimple) category of
$\mathcal {R}$
-representation. In the (semisimple) category of
![]() $\mathcal {R}$
-representations, we can choose a splitting of
$\mathcal {R}$
-representations, we can choose a splitting of
![]() $\mathcal {E}$
to decompose
$\mathcal {E}$
to decompose
Suppose that there are no nonzero
![]() $\mathcal {R}$
-equivariant maps
$\mathcal {R}$
-equivariant maps
![]() $A\rightarrow B$
, or equivalently
$A\rightarrow B$
, or equivalently
Then the kernel of any morphism (5.1) in
![]() $\mathbf {T}$
cannot be contained in
$\mathbf {T}$
cannot be contained in
![]() $\mathrm{Hom}(A,B)$
, and hence the image of
$\mathrm{Hom}(A,B)$
, and hence the image of
![]() $\Omega $
will be the diagonal copy of K. In fact, since
$\Omega $
will be the diagonal copy of K. In fact, since
![]() $\mathrm{Hom}_{\mathbf {T}}(A,B)$
is zero, we get
$\mathrm{Hom}_{\mathbf {T}}(A,B)$
is zero, we get
![]() $\mathrm{End}_{\mathbf {T}}(E;B)\cong K$
.
$\mathrm{End}_{\mathbf {T}}(E;B)\cong K$
.
Note that this scenario directly generalises the situation of Theorem 1.2. If
![]() $\mathbf {T}$
is filtered, taking
$\mathbf {T}$
is filtered, taking
![]() $\mathcal {R}$
to be
$\mathcal {R}$
to be
![]() $\mathcal {G}(Gr^WE)$
embedded in
$\mathcal {G}(Gr^WE)$
embedded in
![]() $\mathcal {G}(E)$
via the section of
$\mathcal {G}(E)$
via the section of
![]() $\mathcal {G}(E)\twoheadrightarrow \mathcal {G}(Gr^WE)$
induced by
$\mathcal {G}(E)\twoheadrightarrow \mathcal {G}(Gr^WE)$
induced by
![]() $Gr^W: \langle E\rangle \rightarrow \langle Gr^WE\rangle $
, we recover case (i) of Theorem 1.2. Taking
$Gr^W: \langle E\rangle \rightarrow \langle Gr^WE\rangle $
, we recover case (i) of Theorem 1.2. Taking
![]() $\mathcal {R}$
to be the multiplicative group
$\mathcal {R}$
to be the multiplicative group
![]() $\mathbb {G}_m$
embedded in
$\mathbb {G}_m$
embedded in
![]() $\mathcal {G}(E)$
through a (possibly noncentral) cocharacter
$\mathcal {G}(E)$
through a (possibly noncentral) cocharacter
![]() $\mathbb {G}_m \rightarrow \mathcal {G}(E)$
that induces the weight grading on the associated graded objects, we recover case (ii) of the result.
$\mathbb {G}_m \rightarrow \mathcal {G}(E)$
that induces the weight grading on the associated graded objects, we recover case (ii) of the result.
5.4 Final remark
We have
where
![]() $\mathrm{Hom}_{\mathbf {T}}(A,B)$
is considered as a subset of
$\mathrm{Hom}_{\mathbf {T}}(A,B)$
is considered as a subset of
![]() $\mathrm{End}_{\mathbf {T}}(E)$
via
$\mathrm{End}_{\mathbf {T}}(E)$
via
Whenever
![]() ${\text {Im}}(\Omega )=K$
, the natural embedding of K into
${\text {Im}}(\Omega )=K$
, the natural embedding of K into
![]() $\mathrm{End}_{\mathbf {T}}(E;B)$
as the space of scalar maps provides a section for the short exact sequence
$\mathrm{End}_{\mathbf {T}}(E;B)$
as the space of scalar maps provides a section for the short exact sequence
This gives an isomorphism
The isomorphism is initially of vector spaces only, but transferring the multiplication on
![]() $\mathrm{End}_{\mathbf {T}}(E;B)$
to the right-hand side, it becomes an isomorphism of algebras. The multiplication on the right is given by
$\mathrm{End}_{\mathbf {T}}(E;B)$
to the right-hand side, it becomes an isomorphism of algebras. The multiplication on the right is given by
and the embedding of K is through the first factor. In particular,
![]() $\mathrm{End}_{\mathbf {T}}(E;B)$
is commutative if
$\mathrm{End}_{\mathbf {T}}(E;B)$
is commutative if
![]() ${\text {Im}}(\Omega )=K$
.
${\text {Im}}(\Omega )=K$
.
Acknowledgements
I would like to thank Kumar Murty for many helpful discussions. I would also like to thank the anonymous referee for a careful reading of the paper and several suggestions that helped improve the exposition of the paper.