Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-22T20:30:08.707Z Has data issue: false hasContentIssue false
  • English
  • Français

Multiplicity-Free Schubert Calculus

Published online by Cambridge University Press:  20 November 2018

Hugh Thomas  and
Alexander Yong
Hugh Thomas
Affiliation:
Department of Mathematics and Statistics, University of New Brunswick, Fredericton, New Brunswick E3B 5A3 e-mail: [email protected]
Alexander Yong
Affiliation:
Department of Mathematics, University of Illinois at Urbana-Champaign, Urbana, IL 61801, USA e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

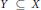Multiplicity-free algebraic geometry is the study of subvarieties $Y\,\subseteq \,X$ with the “smallest invariants” as witnessed by a multiplicity-free Chow ring decomposition of 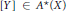$\left[ Y \right]\,\in \,{{A}^{*}}\left( X \right)$ into a predetermined linear basis.

This paper concerns the case of Richardson subvarieties of the Grassmannian in terms of the Schubert basis. We give a nonrecursive combinatorial classification of multiplicity-free Richardson varieties, i.e., we classify multiplicity-free products of Schubert classes. This answers a question of W. Fulton.

Keywords

14M1514M0505E99
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 53 , Issue 1 , 01 March 2010 , pp. 171 - 186
Copyright
Copyright © Canadian Mathematical Society 2010

References

[1] Bessenrodt, C., On multiplicity-free products of Schur P-functions. Ann. Comb. 6(2002), no. 2, 119–124. doi:10.1007/PL00012579Google Scholar
[2] Brion, M., Multiplicity-free subvarieties of flag varieties. In: Commutative algebra (Grenoble/Lyon, 2001), Contemp. Math. 331, American Mathematical Society, Providence, RI, 2003, pp. 13–23.Google Scholar
[3] Fulton, W., Young tableaux. With applications to representation theory and geometry. London Mathematical Society Student Texts 35, Cambridge University Press, Cambridge, 1997.Google Scholar
[4] Gutschwager, C., On multiplicity-free skew characters and Schubert calculus. Ann. Comb., to appear.Google Scholar
[5] Hiller, H. and Boe, B., Pieri formula for SO2 n +1 /U n and Sp n/U n . Adv. in Math. 62(1986), no. 1, 49–67. doi:10.1016/0001-8708(86)90087-3Google Scholar
[6] Pragacz, P., Algebro-geometric applications of Schur S- and Q-polynomials. In: Topics in Invariant Theory (Paris, 1989/1990), Lecture Notes in Math. 1478, Springer, Berlin, 1991, pp. 130–191.Google Scholar
[7] Stanley, R. P., Enumerative Combinatorics. Volume 2, Cambridge Studies in Advanced Mathematics 62, Cambridge University Press, Cambridge, 1999.Google Scholar
[8] Stembridge, J. R., Shifted tableaux and the projective representations of symmetric groups. Adv. Math. 74(1989), no. 1, 87–134. doi:10.1016/0001-8708(89)90005-4Google Scholar
[9] Stembridge, J. R., On multiplicity-free products of Schur functions. Ann. Comb. 5(2001), no. 2, 113–121. doi:10.1007/s00026-001-8008-6Google Scholar
[10] Stembridge, J. R., Multiplicity-free products and restrictions of Weyl characters. Represent. Theory 7(2003), 404–439. doi:10.1090/S1088-4165-03-00150-XGoogle Scholar
[11] Stembridge, J. R., The SF package. Available at http://www.math.lsa.umich.edu/∼jrs/maple.html#SF.Google Scholar
[12] Thomas, H. and Yong, A., A combinatorial rule for (co)minuscule Schubert calculus. To appear in Adv. Math. doi:10.1016/j.aim.2009.05.008Google Scholar