Hostname: page-component-6bf8c574d5-gr6zb Total loading time: 0 Render date: 2025-02-16T23:35:38.272Z Has data issue: false hasContentIssue false
  • English
  • Français

Sur les 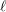$\ell$ -blocs de niveau zéro des groupes $p$ -adiques

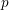Part of: Lie groups Algebraic number theory: local and $p$-adic fields

Published online by Cambridge University Press:  24 May 2018

Thomas Lanard
Thomas Lanard*
Affiliation:
Institut de Mathématiques de Jussieu - Paris Rive Gauche, 4 place Jussieu, 75005 Paris, France email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 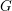$G$ be a 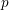$p$ -adic group that splits over an unramified extension. We decompose 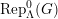$\text{Rep}_{\unicode[STIX]{x1D6EC}}^{0}(G)$ , the abelian category of smooth level $0$ representations of 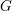$G$ with coefficients in 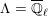$\unicode[STIX]{x1D6EC}=\overline{\mathbb{Q}}_{\ell }$ or $\overline{\mathbb{Z}}_{\ell }$ , into a product of subcategories indexed by inertial Langlands parameters. We construct these categories via systems of idempotents on the Bruhat–Tits building and Deligne–Lusztig theory. Then, we prove compatibilities with parabolic induction and restriction functors and the local Langlands correspondence.

Keywords

representationlocal Langlands correspondencep-adic groupsl-block

MSC classification

Primary: 22E50: Representations of Lie and linear algebraic groups over local fields 11S37: Langlands-Weil conjectures, nonabelian class field theory
Type
Research Article
Information
Compositio Mathematica , Volume 154 , Issue 7 , July 2018 , pp. 1473 - 1507
Copyright
© The Author 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arthur, J., The endoscopic classification of representations: orthogonal and symplectic groups, American Mathematical Society Colloquium Publications, vol. 61 (American Mathematical Society, Providence, RI, 2013); MR 3135650.Google Scholar
Bonnafé, C. and Rouquier, R., Catégories dérivées et variétés de Deligne–Lusztig , Publ. Math. Inst. Hautes Études Sci. 97 (2003), 1–59; MR 2010739 (2004i:20079).Google Scholar
Borel, A., Automorphic L-functions , in Automorphic forms, representations and L-functions (Proc. Sympos. Pure Math., Oregon State Univ., Corvallis, OR, 1977), Part 2, Proceedings of Symposia in Pure Mathematics, vol. XXXIII (American Mathematical Society, Providence, RI, 1979), 27–61; MR 546608.Google Scholar
Bruhat, F. and Tits, J., Groupes réductifs sur un corps local. II. Schémas en groupes. Existence d’une donnée radicielle valuée , Publ. Math. Inst. Hautes Études Sci. 60 (1984), 197–376; MR 756316 (86c:20042).Google Scholar
Cabanes, M. and Enguehard, M., Representation theory of finite reductive groups, New Mathematical Monographs, vol. 1 (Cambridge University Press, Cambridge, 2004), MR 2057756 (2005g:20067).Google Scholar
Chinello, G., Blocks of the category of smooth  $\ell$ -modular representations of  $\text{GL}(n,F)$ and its inner forms: reduction to level- $0$ , Preprint (2017), arXiv:1705.05261.Google Scholar
Dat, J.-F., Simple subquotients of big parabolically induced representations of $p$ -adic groups, https://webusers.imj-prg.fr/∼jean-francois.dat/recherche/publis/subquotient.pdf.Google Scholar
Dat, J.-F., v-tempered representations of p-adic groups. I. l-adic case , Duke Math. J. 126 (2005), 397–469; MR 2120114.Google Scholar
Dat, J.-F., Finitude pour les représentations lisses de groupes p-adiques , J. Inst. Math. Jussieu 8 (2009), 261–333; MR 2485794.Google Scholar
Dat, J.-F., Equivalences of tame blocks for p-adic linear groups, Preprint (2016), arXiv:1603.07226.Google Scholar
Dat, J.-F., A functoriality principle for blocks of p-adic linear groups , in Around Langlands correspondences, Contemporary Mathematics, vol. 691 (American Mathematical Society, Providence, RI, 2017), 103–131.Google Scholar
Digne, F. and Michel, J., Groupes réductifs non connexes , Ann. Sci. Éc. Norm. Supér. (4) 27 (1994), 345–406; MR 1272294.Google Scholar
Haines, T. J., The stable Bernstein center and test functions for Shimura varieties , in Automorphic forms and Galois representations, Vol. 2, London Mathematical Society Lecture Note Series, vol. 415 (Cambridge University Press, Cambridge, 2014), 118–186; MR 3444233.Google Scholar
Harris, M. and Taylor, R., The geometry and cohomology of some simple Shimura varieties, Annals of Mathematics Studies, vol. 151 (Princeton University Press, Princeton, NJ, 2001); With an appendix by Vladimir G. Berkovich. MR 1876802.Google Scholar
Helm, D., The Bernstein center of the category of smooth W (k)[GL n (F)]-modules , Forum Math. Sigma 4 (2016), e11; 98 MR 3508741.Google Scholar
Henniart, G., Une preuve simple des conjectures de Langlands pour GL(n) sur un corps p-adique , Invent. Math. 139 (2000), 439–455; MR 1738446.Google Scholar
Kaletha, T., Regular supercuspidal representations, Preprint (2016), arXiv:1602.03144.Google Scholar
Kaletha, T., Minguez, A., Shin, S. W. and White, P.-J., Endoscopic classification of representations: inner forms of unitary groups, Preprint (2014), arXiv:1409.3731.Google Scholar
Lust, J. and Stevens, S., On depth zero L-packets for classical groups, Preprint (2016), arXiv:1611.08421.Google Scholar
Lusztig, G., Classification of unipotent representations of simple p-adic groups , Int. Math. Res. Not. IMRN 1995 (1995), 517–589; MR 1369407.Google Scholar
Lusztig, G., Classification of unipotent representations of simple p-adic groups. II , Represent. Theory 6 (2002), 243–289; MR 1927955.Google Scholar
Meyer, R. and Solleveld, M., Resolutions for representations of reductive p-adic groups via their buildings , J. Reine Angew. Math. 647 (2010), 115–150; MR 2729360 (2011m:22031).Google Scholar
Moeglin, C., Paquets stables des séries discrètes accessibles par endoscopie tordue; leur paramètre de Langlands , in Automorphic forms and related geometry: assessing the legacy of I. I. Piatetski-Shapiro, Contemporary Mathematics, vol. 614 (American Mathematical Society, Providence, RI, 2014), 295–336; MR 3220932.Google Scholar
Mok, C. P., Endoscopic classification of representations of quasi-split unitary groups , Mem. Amer. Math. Soc. 235 (2015); MR 3338302.Google Scholar
Moussaoui, A., Centre de Bernstein dual pour les groupes classiques , Represent. Theory 21 (2017), 172–246; MR 3694312.Google Scholar
Moussaoui, A., Proof of the Aubert–Baum–Plymen–Solleveld conjecture for split classical groups , in Around Langlands correspondences, Contemporary Mathematics, vol. 691 (American Mathematical Society, Providence, RI, 2017), 257–281; MR 3666057.CrossRefGoogle Scholar
Moy, A. and Prasad, G., Jacquet functors and unrefined minimal k-types , Comment. Math. Helv. 71 (1996), 98–121.CrossRefGoogle Scholar
Sécherre, V. and Stevens, S., Block decomposition of the category of ℓ-modular smooth representations of GL n (F) and its inner forms , Ann. Sci. Éc. Norm. Supér. (4) 49 (2016), 669–709; MR 3503829.Google Scholar
Silberger, A. J., Special representations of reductive p-adic groups are not integrable , Ann. of Math. (2) 111 (1980), 571–587.Google Scholar
Springer, T. A., Regular elements of finite reflection groups , Invent. Math. 25 (1974), 159–198; MR 0354894.Google Scholar
Springer, T. A., Reductive groups , in Automorphic forms, representations and L-functions (Proc. Sympos. Pure Math., Oregon State Univ., Corvallis, OR, 1977), Part 1, Proceedings of Symposia in Pure Mathematics, vol. XXXIII (American Mathematical Society, Providence, RI, 1979), 3–27; MR 546587.Google Scholar
Steinberg, R., Endomorphisms of linear algebraic groups, Memoirs of the American Mathematical Society, No. 80 (American Mathematical Society, Providence, RI, 1968); MR 0230728.Google Scholar
Tits, J., Reductive groups over local fields , in Automorphic forms, representations and L-functions (Proc. Sympos. Pure Math., Oregon State Univ., Corvallis, OR, 1977), Part 1, Proceedings of Symposia in Pure Mathematics, vol. XXXIII (American Mathematical Society, Providence, RI, 1979), 29–69; MR 546588 (80h:20064).Google Scholar
Vignéras, M.-F., Induced R-representations of p-adic reductive groups , Selecta Math. (N.S.) 4 (1998), 549–623; MR 1668044.Google Scholar