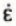Introduction
The closure rates of bore holes give useful information on the dynamics of glaciers and ice sheets. Extensive studies on bore-hole closure have recently been carried out with reference to some of the properties of ice, e.g. crystal fabrics at Cape Folger, East Antarctica (Reference Thwaites, Wilson and McCrayThwaites and others 1984), dust content at Dye 3 in south Greenland (Reference Gundestrup and HansenGundestrup and Hansen 1984), and microparticle concentrations, grain-size, and other parameters at Agassiz Ice Cap in Ellesmere Island (Reference Fisher and KoernerFisher and Koerner 1986).
Provided that the strain-rates are obtained from frequent measurements of bore-hole diameters over a wide range of depth, a stress–strain-rate relation can be deduced. Only a few studies have been done in this area: Reference NyeNye (1953) analysed the Jungfraufirn bore hole, and Reference GowGow (1963)studied the Byrd Station bore hole. Reference PatersonPaterson (1977) derived a power law for ice creep based on the data from Byrd Station, Site 2 in Greenland, and Meighan and Devon Island ice caps. However, Paterson’s successful analysis was restricted to the low-stress range from 0.15 to 0.8 MN m−2, due to the difficulty of distinguishing the secondary (steady) creep stage in deep layers.
An ice-core drilling project was conducted in 1983–84 at Mizuho Station (70°42′03″S, 44°17′36″E; 2247 m a.s.l.), East Antarctica, by the 24th and 25th Japanese Antarctic Research Expeditions (JARE-24 and JARE-25). A 411 m deep bore hole was drilled in the austral winter of 1983 by JARE-24. A preliminary study of the bore-hole closure was made by Reference Kawada, Yoshida and NaruseKawada and others (1986). JARE-25 drilled a new bore hole from 140 to 700.63 m depth during the period 2 June – 1 August 1984. A thermal drill 168 mm in diameter was used down to a depth of 626 m, and one 138 mm in diameter was used for the deeper part. The bore hole was not filled with liquid during or after drilling.
By analysing the results of measurements of closure rates in this bore hole, we can present a power law for ice creep in a relatively high stress range, from 0.8 to 1.65 MN m−2, at a temperature of −35°C.
Instruments and Methods
The diameter gauge used for the Mizuho bore hole was a tandem one developed by the Institute of Low Temperature Science, Hokkaido University. Figure 1 is a schematic illustration of the gauge (Reference Naruse, Shimbori, Akitaya and SuzukiNaruse and others 1985). It has two separate calipers 0.53 m apart, each having three contacting steel wheels (W) which are pressed against the wall of the bore hole by a spring (S). Two bars (F) are a device for releasing the gauge when it is stuck. Because of the use of two calipers in tandem, the gauge has such advantages as better alignment with the axis of the bore hole, and back-up in case one of the calipers malfunctions. The potentiometer of one of the calipers did not work well and only the other calipers were used. Change in diameter is converted into change in the output voltage by a rotational potentiometer (P) mounted on the axle of each of the calipers. The total length of the gauge is 1.4 m, the power supply is 12 V d.c, and the range of measurement is 90–190 mm.
Another gauge was used for the narrow bore hole — a Geologger 300 manufactured by the Oyo Corporation, Japan.

Fig. 1. Schematic illustration of a diameter gauge with two calipers in tandem. S: compressive spring; W: wheel; P: rotational potentiometer; F: rod for release.
Its caliper probe (model 3973) has two arms which are pressed against the wall of the bore hole by a spring. The gauge measures diameters from 65 to 100 mm.
Calibrations were made before and after every measurement at Mizuho Station by using six plastic pipes whose diameters ranged from 67 to 158 mm. Judging from these results, the accuracy of the gauges is about ²1 mm for the tandem gauge and ²2 mm for the Geologger calipers, provided the tips of the calipers are in good contact with the wall of the bore hole.
A power supply and recorder were placed outside the bore hole. Measurements were carried out continuously with depth, and the running speed of the gauge in the bore hole ranged from 0.2 to 0.25 m/s. Diameter at depth was obtained by averaging data measured while the gauge moved both downward and upward. The depth in the bore hole was determined by reading the length of winch cable released.
A profile of the bore-hole diameter was first measured to a depth of 700 m on 3 August 1984, 2 d after the completion of drilling. This survey was made with calipers which also had three contact points. Then, over a period of 2 years, measurements were carried out on a total of ten occasions. For diameters less than about 95 mm, the Geologger calipers were used.
The temperature of ice in the bore hole was also measured, with a thermistor thermometer whose sensor was pressed against the wall of the bore hole by a spring. The resolution of the thermometer was 0.01°C.
Results of the Survey
From the continuous records of diameter with depth, a mean diameter over 2 m depth intervals was estimated every 10 m (Reference Okuhira, Naruse, Nakawo and KawadaOkuhira and others 1987). Ten profiles of the bore-hole diameter are shown in Figure 2. It is noted that the diameter measurements fluctuate considerably: the widening of the diameter is the result of melting by the thermal drill. Observed diameters larger than 158 mm in the shallow layer were eliminated here, with the exception of data from the first survey, because of possible instrumental error in the large-diameter range. Due to the intensive contraction of the bore hole in the deep layer, measurements could only be made down to about 400 m depth in 1986. Based on the results of test drillings with the same types of thermal drill, the initial diameter of the bore hole is assumed to be 175 mm in the layer from 150 to 300 m depth, and to be 180 mm from 300 to 625 m depth. These values are used for calculations of strain.

Fig. 2. Vertical distribution of the diameter in a 700 m deep bore hole at Mizuho Station. Numbers from 1 to 10 indicate the date of measurement: 1: 3 August 1984; 2: 23 February 1985; 3: 30 April 1985; 4: 6 June 1985; 5: 1 July 1985; 6: 2 August 1985; 7: 12 September 1985; 8: 17 January 1986; 9: 9 May 1986; and 10: 7 July 1986.
Ice temperatures measured on 27–31 March 1985 (Okuhira, unpublished report) are listed in Table I for the layer from 280 to 540 m depth, and a stress–strain relation is analysed. Almost uniform temperature, from −35.2° to −35.5°C, is noted.
TABLE I. Data obtained from the mizuho station bore hole


Fig. 3. Strain ε versus time t after drilling, shown at every 40 m depth. On 3 August 1984, when the first measurement was made, t = 49 d at 260 m depth, and t = 16 d at 540 m depth. Thick segments in the broken lines represent the ranges of ε and t used to estimate the minimum strain-rates.
Secondary (Minimum) Creep Rate
Although the creep rate of ice is not homogeneous around a bore hole, i.e. the strain-rate is maximum at the wall of the bore hole and decreases with distance from the bore hole,Reference Paterson Paterson (1977) showed that an appropriate measure of the strain ε for the closure of a cylindrical hole was
where a0 is the initial diameter of the hole and a is the diameter at the time of measurement.
Using the depth profiles of the diameter shown in Figure 2, a creep curve can be obtained at each depth where many measurements were made. Figure 3 shows the total strain ε plotted against the time t after drilling for every 40 m depth. Some deviations from smoothed curves are noted (shown by broken lines); they are considered to be caused either by accidental loose contacts of the calipers with the bore hole or by the decrease in sensitivity of the instrument at such a low temperature.
As mentioned by Reference GowGow (1963), Reference PatersonPaterson (1977), and other investigators, secondary (steady) creep often cannot be recognized clearly in the deformation of a bore hole, but tertiary (accelerating) creep appears just after transient (decelerating) creep. It is also considered that tertiary creep, due to recrystallization of ice, starts in the deep layer when the ice in the shallow layer is still in a state of transient creep. Therefore we determine the minimum creep rate
Data for the minimum strain-rate were obtained at successive intervals of 10 m from 280 to 540 m depth and are listed in Table I.
Stress-Strain-Rate Relation
If the ice is assumed to be isotropic and incompressible, the relation between the effective strain-rate
and the effective shear stress τ is generally expressed as
where Q is the activation energy for the creep of ice, R is the gas constant, Τ is the temperature of ice, and A is a parameter which may depend on impurities (soluble and insoluble), crystal size, and other parameters as yet unstudied. Then, according to the plastic theory, the effective shear stress at the wall of a cylindrical hole submerged in pressure P, as shown by Nye (1953) and used by Paterson (1977) and other workers, can be given as
If the bore hole is filled with liquid, Ρ is the net hydrostatic pressure between ice and liquid. In the present study, Ρ was calculated (Table I) by the density profile which was obtained from in-situ measurements of ice cores at Mizuho Station (Reference Nakawo and NaritaNakawo and Narita 1985; Fujii and others, unpublished report). From the relation between
and Ρ (i.e. Equation (equ.2) and (equ.3)), and the data in Table I, n was calculated by regression to have the value 2.87. Then the values of τ were calculated and are also shown in Table I.

Fig. 4. Relation between the effective strain-rate
Figure 4 shows the relation between
and τ, which can be expressed as
where
is measured in s−1 and τ in MN m−1. Also illustrated (by dotted lines) are Paterson’s result at −22°C and the same result adjusted to −35°C by using his mean Q value of 54 kJ mol−1. Although the range of effective stress is different for these two studies, Equation (4) indicates strain-rates approximately twice those of Paterson at the same temperature and stress. Reference Fisher and KoernerFisher and Koerner (1986) concluded that the closure strain-rates in Holocene ice fit the Paterson line, but the rates in late Wisconsin ice show nearly three times the Holocene value in Agassiz Ice Cap. The detailed mechanism and reasons for this difference have not been made clear to date.
Strictly, we cannot compare the results of the bore-hole study with those of laboratory experiments, not only because of the difference in dimensions and properties of the ice but also because of the difference in the method of evaluation of the stress and strain-rate. However, an experimental relation obtained by Reference Barnes, Tabor and WalkerBarnes and others (1971), which is for stress lower than 3 MN m−2 and after the temperature had been adjusted to −35°C, is also shown by a broken line in Figure 4. The results of the present study show rheological properties of ice intermediate between those deduced in the laboratory and those obtained by Paterson.
Concluding Remarks
Closure rates of a 700 m deep bore hole were obtained at Mizuho Station. Due to a scarcity of measurements in the early stage after drilling, we could not clearly recognize the secondary (steady) creep rate. It seems, from the creep curves of closure, that transient (decelerating) creep is followed by tertiary (accelerating) creep. Hence we determined the minimum rate of strain, which should correspond to the conventional secondary creep rate. Although the estimated minimum strain-rates involve some degree of error, caused by fitting smoothed curves to data, the strain-rates correlate well with the stresses in the form of a power law in which n = 2.87 in the depth range from 280 to 540 m.
Closure rates at Mizuho Station are found to be much higher than those obtained from other ice sheets and ice caps (Reference PatersonPaterson 1977).Reference Narita, Nakawo and Fujii Narita and others (1986) showed that at Mizuho Station mean grain-size (area) is 8–10 mm2 in the layer from 300 to 700 m depth, and that the c-axis alignment, which is random at shallow depths, becomes a great-circle girdle at 600 m depth. The c-axis concentration (e.g. single-maximum) favors the bore-hole tilting, i.e. shear deformation, but it tends to hamper the bore-hole closure, as pointed out by Reference Thwaites, Wilson and McCrayThwaites and others (1984) and Reference Fisher and KoernerFisher and Koerner (1986).
At present we cannot account for the higher closure strain-rates in the bore hole at Mizuho Station. Extensive thinning (Reference NaruseNaruse 1979) or the recent variation in the ice sheet (Nishio, in preparation) in Mizuho Plateau might be elucidated by this flow enhancement.
More field research on bore-hole tilt and closure is necessary, as well as laboratory studies on the dependence of ice textures, c-axis fabrics, and impurities upon ice deformation.
Acknowledgements
We are grateful to Dr H. Narita of the Institute of Low Temperature Science (ILTS), Dr Y. Fujii and Dr F. Nishio of the National Institute of Polar Research (NIPR), Mr M. Yoshida of the Hakusan Corporation, Dr Y. Ageta of Nagoya University, and other wintering members of JARE-25, JARE-26, and JARE-27 for their assistance with field measurements. We are also indebted to Professor A. Higashi of the International Christian University for his generous supervision of this project, and to Professor N. Maeno of ILTS for valuable comments on this paper.