1.1 Introduction

The risk margin is part of the Solvency II regime which came into force in the European Economic Area (EEA) in 2016. It has proved to be controversial in the United Kingdom (UK), where it has been criticised for being too large and too sensitive to interest rate movements, particularly in relation to business subject to long-term guarantees, such as annuities.

This paper examines the need for the risk margin and considers a range of possible alternatives, ranging from amendments to the calibration of the existing methodology to more radical changes. The working party hopes that the paper will inform the debate both in the context of reviews of Solvency II and more particularly within the UK, if changes to the solvency regime are under consideration following Brexit – the withdrawal of the UK from the European Union (EU).

1.2 The Risk Margin and Its Problems

1.3 The Working Party

In the light of the problems that had been identified and the political background, our working party was established in September 2017.

Our brief was to address two key objectives, in the context of life insurance business:

• What can be done to fix known issues with the risk margin, either within Solvency II or using potential post-Brexit flexibility?

• What should be the purpose of the risk margin and how can that purpose best be fulfilled?

We aim to address both of these objectives through the production and presentation of this paper.

In order to inform our conclusions, we carried out a survey of life insurers. The results of the survey are given in sections 4 and 6 of the paper, with a list of the questions in Appendix C.

We have also developed a simple model to illustrate features of the risk margin and assess the impact of various alternatives – the model is described in section 5 of the paper, with more detail in Appendix D. The main results of the model are presented throughout the paper.

In addition to the above, we have

• contributed to the response from the IFoA to a consultation by the European Insurance and Occupational Pensions Authority (EIOPA) reviewing Solvency II;

• presented at Current Issues in Life Insurance (CILA) in the form of a debate and at the IFoA Life Conference; and

• engaged in a debate on the risk margin in The Actuary magazine.

1.4 Potential Amendments to the Risk Margin

In section 6 of the paper, we analyse a number of alternatives for re-calibration within the framework of the existing Solvency II Directive, retaining the cost-of-capital methodology.

These include

• reducing the assumed cost-of-capital rate;

• allowing the cost-of-capital rate to vary with interest rates; and

• amending the discount rate from the risk-free rate.

The working party’s view is that more fundamental changes should be considered. In section 7 of the paper, we examine the following alternative methodologies:

• a run-off percentile approach originally considered for Solvency II, for example, the risk margin might be defined as the difference between the 75th percentile of the probability distribution of the liabilities and the BEL;

• a Provision for Adverse Deviation (PAD), for example, the impact of a specified adverse scenario;

• a Value-at-Risk (VaR)-based approach;

• a run-off capitalisation approach;

• market reinsurance pricing in relation to longevity risk; and

• removal of the risk margin altogether or replacing it with an increase in the solvency capital requirement (SCR).

In addition, section 7 includes a description of the margin over current estimate (MOCE), which is under consideration for the framework for International Capital Standards (ICS) being developed by the International Association of Insurance Supervisors (IAIS). To supplement this, Appendix E includes an overview of measures similar to the risk margin used in various solvency regimes outside the EEA.

In section 8 of the paper, we consider the properties of a desirable risk margin. We also evaluate the alternatives considered in sections 6 and 7 against these properties.

1.5 Practical Issues

Appendix A considers briefly a number of practical issues relating to the risk margin.

In section A1 of this Appendix, we consider the calculation of the risk margin and describe some of the simplifications which are necessary to carry out the calculations in practice.

In section A2 of this Appendix, we consider the question of stressing the risk margin in calculating the SCR.

An analysis of whether and how the risk margin can be hedged is given in section A3 of this Appendix.

1.6 Impact of Brexit

The future of the risk margin in the UK is likely to be highly dependent on Brexit.

At the time of writing, it remains unclear when and in what form Brexit will take place, and indeed even whether it will happen at all. As indicated in section 1.2.3, uncertainty over Brexit is already inhibiting the PRA from implementing changes considered to be desirable.

However, one of the working party’s aims in producing this paper is to inform any future debate on the development of the risk margin, and so we have to consider all possible outcomes.

One outcome is that following Brexit, the UK will have a relatively free hand in determining the solvency regime that is considered appropriate for UK insurers. In that situation, given the consensus in the UK that the risk margin is flawed, the alternative methodologies described in section 7 of the paper should be considered.

It is possible that following Brexit, the UK wishes to obtain Equivalence with Solvency II. Although Equivalence is likely to be more relevant to general insurance business than life business, a major change to the risk margin for life business may be a significant barrier to this. This could constrain potential changes to the risk margin in the UK.

Furthermore, the outcome of Brexit may be that the UK is constrained to be subject to Solvency II, either through a UK–EU agreement covering insurance business or through the UK remaining a member of the EEA. In this case, the UK would have no direct influence on the future of Solvency II, including the risk margin, and it is likely that changes to the risk margin to resolve problems specific to the UK would be difficult to achieve.

Nevertheless, irrespective of whether or not the UK remains a member of the EU, the working party hopes that this paper will be of direct use to EIOPA in reviewing the risk margin within the forthcoming review of Solvency II. We also expect the paper to inform the profession’s response to the consultation for the review.

1.7 Conclusions

The working party’s analysis has not reached a firm conclusion on one specific alternative method. Indeed, the different objectives are at times in conflict, so there is no one solution that meets all of them. Furthermore, the range of possible changes will be dependent on the outcome of Brexit.

Nevertheless, having regard to our evaluations of the various alternatives, the working party sees merit in considering the following changes:

• to allow for an automatic change in the assumed cost-of-capital rate when risk-free rates change;

• to allow a prudent illiquidity premium to be used in the calculations of the projected future SCRs and in the risk-free rate used in discounting the future costs of capital;

• to allow certain longevity risk to be treated as hedgeable and the relevant part of the risk margin to be replaced by the cost of the hedge; or

• to move to, or to allow as an alternative, the Percentile-MOCE, which is being considered under ICS.

More details are given in section 8.4, including our reasoning for selecting these alternatives.

The working party believes that a risk margin in some form is necessary. However, our view is that striking the balance between protecting policyholders and ensuring value for money is primarily a political choice, which affects the definition of both the risk margin and the SCR. This choice can be reflected in the calibration of any method that may be chosen. We hope that this paper will be useful in informing any deliberations regarding this balance.

1.8 Literature Review

A brief literature review is given in Appendix G.

2.1 The Case for a Margin

The idea that people are risk-averse is a well-known concept in many branches of economics and finance. Given a choice between receiving a guaranteed £50 and spinning a coin to decide whether they receive £100 or nothing at all, we expect the majority of people to choose the guaranteed amount.

We similarly expect firms to prefer liabilities where the value is known with certainty, rather than liabilities where the value cannot be known in advance. It seems sensible to reflect this preference in the value placed on the liabilities, with this value reflecting not only the expected cost, but also the uncertainty around this cost.

In the Solvency II framework, the starting point for determining technical provisions is to project a set of best estimate cash flows, each of which represents the mean outcome. Uncertainty around market risks is allowed for using a risk-free discount rate to value these cash flows, albeit subject to adjustments. The risk margin is then intended to reflect the “price” that is placed on the uncertainty relating to non-hedgeable risks.

We believe, therefore, that the underlying purpose of the risk margin is to ensure that technical provisions are struck on a market-consistent basis, that is, that a market price of risk is incorporated in respect of all risks, not only those risks for which deep and liquid markets exist. The hypothetical construct of the reference undertaking (see section 2.3), under this interpretation, is simply a means to arriving at a transfer value for the firm’s liabilities, rather than an end in itself. We now consider market consistency in more depth.

2.2 Market Consistency of Technical Provisions

One potential way in which the price of uncertainty can be included in the liability value is by setting the liability value equal to the amount required to settle the liability in the financial markets. If market participants are indeed risk-averse, then the amount for which they are prepared to settle the liability will presumably include the price of risk.

This is the approach taken by Solvency II, with Article 75, paragraph 1.(b) of the Directive stating that:

One key argument in favour of market-consistent valuation is that it results in liability values that reflect the cost of hedging the liabilities. If this is the case, and the insurer covers its liabilities with assets, then the insurer should be able to remove risk from its balance sheet should this become necessary, and thereby ensure that it does not default on its obligations.

An insurer’s solvency capital requirement (SCR) represents the 99.5% 1-year VaR in “basic own funds” (broadly equal to the excess of assets over liabilities). If an insurer holds own funds equal to its SCR then, in theory at least, there is a 99.5% chance of it surviving the next year. After 1 year has passed, if the insurer no longer covers its SCR it should still have sufficient assets to de-risk its balance sheet and therefore have a reasonable certainty that it will discharge its obligations in full.

This description of the Solvency II regime relies on the risk margin acting as a bridge between the SCR – which provides protection for policyholders over the next year – and the term of life insurance policies – which is typically much longer than a year.

While this description of the protection afforded by the Solvency II regime has a certain logic to it, it should be noted that this is only one interpretation of the intention behind the regime and this objective is not stated in the Solvency II Directive.

As indicated in Appendix B section B1.2, in developing the risk margin, the Committee of European Insurance and Occupational Pensions Supervisors (CEIOPS) – the forerunner of EIOPA – stated that it should have regard to a number of criteria including “achieving an appropriate level of policyholder protection over the run-off period of the liabilities” (CEIOPS, 2007, page 13). We should therefore also regard policyholder protection as an objective of the risk margin.

2.3 The Risk Margin Calculation

While a market-consistent valuation may look like an attractive option, the challenge it faces is that the cost of settling insurance liabilities cannot be observed directly from the financial markets.

This has required the architects of Solvency II to attempt to model this cost, and this modelled cost is necessarily dependent on a number of assumptions. Key features of the model are

• the transfer takes place to a “reference undertaking” which has no assets or liabilities before the transfer, and which remains closed to new business after the transfer;

• the reference undertaking will de-risk its assets so as to minimise market risk;

• the reference undertaking is subject to Solvency II on an ongoing basis. It is required to hold technical provisions plus an SCR in respect of its remaining risks at all times – it therefore raises sufficient capital at the beginning of each year to cover this SCR for that year;

• the amounts set aside to raise future capital are assumed to earn the basic risk-free rate of return inside of the reference undertaking;

• however, the providers of capital require a return equal to 6% above the basic risk-free rate, since the capital is at risk (e.g. if assumptions are worse than best estimate).

These assumptions give rise to the following formula for calculating the risk margin:

(1) $$Risk\;Margin = CoC.\sum\limits_{t \ge 0} {{{SCR\left( t \right)} \over {{{\left( {1 + r\left( {t + 1} \right)} \right)}^{t + 1}}}}} {\rm{ }}$$

where

• CoC denotes the cost-of-capital rate – for which a value of 6% is prescribed;

• SCR(t) denotes the projected SCR after t years;

• r(t + 1) denotes the basic risk-free interest rate for maturity t + 1 years.

It should be noted that the Solvency II Delegated Regulation prescribes the risks that insurers should assume will be included in the reference undertaking’s SCR. The only exception to this is where the insurer has actually hedged the risk – in which case it is assumed that the reference undertaking will maintain the hedge. The prescribed risks are

• underwriting risk;

• market risk which cannot be eliminated through the de-risking of assets;

• certain credit risks (including reinsurance); and

• operational risk.

The Solvency II rules also set out a number of other key assumptions which insurers should make when calculating the risk margin:

• any reinsurance contracts held by the insurer transfer to the reference undertaking;

• the SCR is calculated using the same model as is currently used by the insurer: be that the standard formula or a partial or full internal model that has been approved by the regulator;

• the SCR makes no allowance for the loss-absorbing capacity of deferred tax;

• the reference undertaking adopts future management actions that are consistent with those of the insurer.

In addition to these explicitly stated assumptions, Huber and Kinrade (Reference Huber and Kinrade2017) point out that the risk margin methodology also relies on a number of implicit assumptions not set out in the rules. These include

• capital is raised in the form of equity;

• the investment return on the assets in which the capital is invested is not subject to tax; and

• the cost-of-capital rate is charged only on the SCR even though, in practice, most insurers aim to hold a multiple of the SCR.

3.1 Challenges for the Insurance Industry

The risk margin has faced much criticism, from the UK life insurance industry in particular. In the working party’s experience, the bulk of this criticism has not focused on the assumptions underpinning the risk margin model but has instead focused on the result that emerges. Specifically, the risk margin is said to:

• be too large;

• be too volatile;

• incentivise the transfer of risk to jurisdictions that are not subject to Solvency II;

• be too inflexible.

This section discusses each of these areas in turn.

3.1.2 Volatility of the risk margin

The PRA has estimated that a 100 basis points (bps) fall in the basic risk-free rate would result in the risk margin increasing by around 27% (Rule, Reference Rule2017). Illustrative modelling carried out by the working party, as described in section 5, shows a similar picture. Figure 1 illustrates how the size of a typical risk margin might have changed from the point at which Solvency II came into force, up to the end of 2018.

Figure 1. Variation of risk margin with risk-free rates.

Insurers understandably dislike sources of volatility on their balance sheets. Volatile balance sheets increase the likelihood that an insurer may be temporarily unable to meet its SCR, an event which can disrupt the insurer’s business plans by, for example, preventing it from paying dividends, forcing it to change its investment strategy and/or restricting its ability to write new business.

It is worth noting that the risk margin is typically significantly more volatile than the BEL for the same business. This can be seen by calculating the risk margin as a percentage of BEL, and then looking at how this metric has moved from 31 December 2015 to the end of 2018. This is illustrated in Figure 2.

Figure 2. Variation of risk margin as proportion of BEL with risk-free rates. Note: The RM/BEL ration for Dec 2015 is 7.7%. This is shown as 100% in the graph.

When faced with a volatile balance sheet item, the standard response is generally to look to hedge the volatility. The working party’s experience is that insurers have sometimes been reluctant to hedge the risk margin. Possible reasons for this include

• there is a reluctance to lock in to long-term interest rates at a time when rates are low compared to historical levels. This is particularly true for the risk margin, which does not represent a true liability in the sense that it does not represent future payments to policyholders;

• there is a cost associated with those hedging options which will simultaneously protect the insurer against future falls in interest rates and allow the insurer to benefit in a scenario where rates revert to historical averages; and

• the risk margin does not normally feature as part of the accounting balance sheet under current International Financial Reporting Standards (IFRS) and UK Generally Accepted Accounting Principles. It therefore seems that hedging the risk margin may increase the volatility of the accounting balance sheet.

In section A3 of Appendix A, we discuss some of the issues relating to hedging the risk margin.

3.2 Potential Issues with the Market Consistency Objective

Section 3.1 outlines some of the main concerns expressed by the industry in relation to the risk margin. This section and section 3.3 examine how the method and assumptions used to calculate the risk margin have given rise to these concerns.

Before considering the detail of the method and assumptions, it is worthwhile considering whether the risk margin’s primary purpose – to ensure market-consistent valuation of technical provisions – is appropriate.

The following considerations are particularly relevant:

• Is market-consistent valuation appropriate for life insurance liabilities? Arguably, there are aspects of the Solvency II regime which, paradoxically, are not market-consistent, despite its original intentions (although it is not yet known whether these aspects will be retained in the UK post-Brexit). Market-consistent valuation may also have other drawbacks, such as introducing additional volatility to the regulatory balance sheet. This can undermine insurers’ ability to invest through the cycle: the more the balance sheets deteriorate in times of stress, the greater the incentive to de-risk balance sheets in times of stress, which can lead to procyclical investment behaviour. Solvency II has recognised this and, in an effort to reduce excessive volatility, has introduced various concepts such as the MA and volatility adjustment (VA) which have moved the regime away from pure market consistency.

• A common variant of the arguments against market consistency is that, while base liabilities should be struck on a market-consistent basis, solvency capital should be struck using a run-off obligation-based approach, causing the risk margin effectively to disappear from the stressed balance sheet. The Solvency II regime aims for a situation where each insurer has at least a 99.5% probability of surviving the next year (i.e. having assets in excess of its liabilities in 1-year’s time) and then being able to fully de-risk its balance sheet. There is, however, a question about whether this two-stage aim is too complicated. In particular, if this aim is to be achieved, then insurers will need to model the cost of transferring risk during times of stress. Given that one cannot even readily observe the current cost of transfer, and given the contention around the debate about how the current cost of transfer should be modelled, modelling the cost of transfer under stress is ambitious to say the least.

• Why is mortality/longevity risk treated as non-hedgeable, whereas almost all market risks are treated as being hedgeable? Reinsurance markets are arguably deeper than a number of the markets from which market-consistent assumptions are derived, for example, long-term equity or property volatility. Should reinsurance pricing therefore be given greater weight within the calculation of technical provisions? If so, this can be incorporated in a number of different ways.

• In general, it is worth re-considering the set of risks within scope of the risk margin calculation.

3.3 Critique of Risk Margin Method and Assumptions

As well as considering whether a market-consistent valuation is appropriate for technical provisions, it is also worthwhile discussing whether the method and assumptions used to estimate this market-consistent value are appropriate.

3.4 Impact of the Risk Margin on the Pricing of Annuities

The availability and cost of annuities is very significant to the system of retirement provision in the UK, and indeed to the economy as a whole.

Individual annuities still have an important role to play in retirement provision, although there are now alternative means of using built-up funds. In particular, they provide insurance against living longer than expected.

The bulk purchase annuity market enables company pension funds to transfer current or future pensions to insurers in order to remove longevity risk or indeed close down. This can be beneficial both for reducing risk to the sponsoring employer and improving the security of benefits for the members.

Furthermore, the provision of individual and/or bulk annuities is seen as an area of opportunity for the UK life insurance industry, at a time when other products are subject to competition from outside the industry.

However, concern has been expressed that these benefits are jeopardised by the size of the risk margin, which represents economic capital, the cost of which must be allowed for in pricing.

This concern was a significant theme in submissions to the Treasury Committee inquiry (see section 3.5.2).

However, the PRA, in its response to the Treasury Committee (PRA, 2018, page 11) stated

This conclusion was based on Figure 3. The working party’s view is that it is clear that interest rates continue to be the dominant driver, but the limited data in the figure do not conclusively indicate that the relationship has not changed.

Figure 3. Relationship between annuity rates and risk-free market interest rates.

3.5 Solvency II Reviews and Industry Proposals

During 2017 and 2018, there were two main enquiries into the operation of Solvency II generally, and the risk margin in particular.

1. 1. EIOPA advice on specific items in the Solvency II Delegated Regulation

   The consultation, which included the risk margin, took place in 2017 and EIOPA’s final advice was published in February 2018 (EIOPA, 2018).

2. 2. In the UK specifically, the Treasury Committee inquiry into EU Insurance Regulation

This inquiry took place in the first quarter of 2017 and, following the General Election and the reconstitution of the Committee, the report was published in September 2017 (Treasury Committee, 2017b).

4.1 Introduction

The working party has carried out a survey of selected UK life insurers, with the aim of providing insight into how the UK life insurance industry currently approaches the valuation and management of the risk margin, the main issues faced and potential future design changes.

The survey took place in the third quarter of 2018. In the period since implementation of Solvency II, the methodology and approach used by firms to measure the risk margin have largely bedded down, while the management and optimisation of it continue to date.

However, there are areas relating to the measurement of risk margin and management that are still open to debate.

The working party hopes that the results of the survey are useful in summarising industry attitudes at this point in time and as a comparator in future years.

4.2 Survey Structure

The structure of the survey was designed to capture

• the key methodology points for determining the risk margin, rather than details of calculations;

• the main issues being faced in managing it, particularly the volatility of interest rates; and

• the main areas to re-think or modify post-Brexit.

The survey questions were therefore grouped in two parts; first on the current approaches and practices and the second on the design issues and potential changes. Appendix C details the questions we asked in the survey.

The survey was sent to the Chief Actuaries of most major life insurance groups in the UK, in particular those with material volumes of immediate annuity business. In addition, the survey was also sent to firms writing a mix of other business such as protection and unit-linked and with-profits savings business.

We attempted to keep the length of the survey reasonable and hope it was not too onerous to complete. We are grateful to those who took the time to contribute to the results.

4.3 Survey Results

The response rate is considered healthy for this type of survey. The responses have been from firms of varying sizes, which cover a wide variety of product offerings in the UK. In the interests of data protection and commercial confidentiality, individual responses have been treated in strictest confidence. For the purpose of this report, all results have been presented in an aggregate format or have been made anonymous.

The survey results on the profile of respondents and current practice are presented in this section, whereas the results on the current challenges and aspects of the risk margin where firms would like to see changes, particularly post-Brexit, are presented in section 6, where we discuss these issues and potential options.

When presenting our analysis, we have also included the context of our findings, by including a profile of respondents, where relevant.

4.4 Respondent Profile

4.4.1 Size of respondents

The survey received participation from a wide spectrum of respondents (14 responses in total) ranging from small to large businesses in terms of their in-force liabilities. We have categorised respondents by the size of their technical provisions at 31 December 2017 and the results are summarised in Figure 4.

Figure 4. Size of respondents.

We use the following definitions when referring to the technical provisions of the respondent:

• Small: less than £5bn;

• Medium: more than £5bn, but less than £10bn;

• Large: more than £10bn.

The technical provisions include unit reserves, and are before allowance for TMTP, which mitigates the impact of the risk margin at the date of Solvency II implementation.

4.4.2 Lines of business

We asked respondents about their material lines of business.

As shown in Figure 5, unit-linked and protection were the most common lines of business offered by the respondents. There was also a broad range of other lines of business.

Figure 5. Lines of in-force business.

Although there were a few monoline respondents, most offered two or more lines of business. About 45% of the respondents offered three or more lines of business.

Firms are however expected under Solvency II to manage the impact of risk margin for new business written under the new regime. We therefore expect firms open to new business to be more active in managing the risk margin.

Consequently, we also asked the respondents about their material lines of new business.

As shown in Figure 6, unit-linked and protection were the most common lines of new business. There was also a broad range of other lines of business.

Figure 6. Lines of new business.

4.4.3 Solvency model used by respondents

As shown in Figure 7, the standard formula was the most common model used by the respondents, although some respondents used internal models.

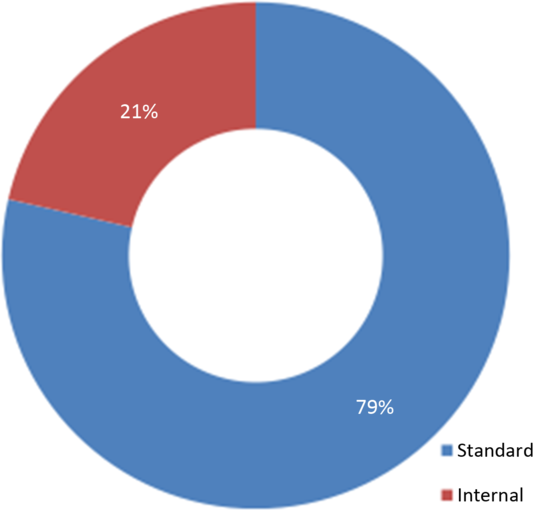
Figure 7. Solvency model.

The internal model respondents include those using partial internal models.

For these respondents, we also asked which risks were internally modelled. The main response is that the model is partial because some blocks of business are on the standard formula.

All respondents using internal models are large firms.

5.1 High-Level Summary of Model and Purpose

The working party has developed a simple model to carry out sensitivity analyses to show the volatility of the risk margin to key assumptions built in the current risk margin methodology and to assess the impact of alternatives to the current approach discussed later in the paper.

The model projects the results for a monoline annuity firm, writing immediate annuity business with no reinsurance. It projects benefit and expense cash flows for an assumed age/gender mix. Details of the model are set out in Appendix D.

The modelled SCR assumes no market and counterparty default risk (since no reinsurance is assumed) and allows for only longevity, expense and operational risks, in each case in accordance with the standard formula.

We are aware that the longevity stresses typically used by internal model firms for annuity business are somewhat larger than those required by the standard formula, because internal models stress both the mortality rate and the trend, whereas the standard formula stresses only the mortality rate. As an illustration of this, PwC (2018) states that the average increase in life expectancy at the age of 65 years resulting from the longevity stresses in internal models at 31 December 2017 is an increase of around 3 years, whereas the increase in our model is less than 2 years. Therefore, our model is likely to underestimate the amount of the risk margin for internal model firms.

The working party believes that our model is a reasonable means of illustrating simply the properties of the risk margin.

For the purpose of presentation in this paper, we have mainly used relative measures to show the impact on the risk margin, such as the percentage of BEL or SCR and/or the change in risk margin relative to December 2015.

The working party believes that the risk margin as a percentage of the modelled SCR is a useful metric, as most of the sensitivity testing and proposals considered in the paper are not likely to have any direct impact on market risk.

5.2 Base Model Results

The base position includes an allowance for MA of 100 bps in the calculation of BEL. Neither the RM SCR nor the discount factors used for the cost of capital allow for the MA. The allowance for MA relates only to the BEL used in the denominator in the ratios or in the calculation of the SCR. Unless stated otherwise, the results do not allow for transitional measures or reinsurance.

Table 1 presents the base modelled results at 31 December 2018 for key metrics. Appendix D also presents the sensitivity of base results to various changes in assumptions made.

Table 1. Base Results

5.3 Sensitivity to Interest Rates

The risk margin is particularly sensitive to changes in risk-free rates. To show this volatility, we have run the model with monthly EIOPA risk-free rates from December 2015 to December 2018 and have shown the results in section 3.1.2 for both movements in the risk margin as a percentage of the December 2015 risk margin and movement in the risk margin/BEL ratio as percentage of December 2015 risk margin/BEL ratio.

Figure 8 shows the movement in risk margin/BEL ratios for each year-end since the implementation of Solvency II, along with the sensitivity to a parallel shift of 50 bps down and up to the December 2018 curve. The Figure also shows the 12-year EIOPA spot rates, corresponding to the duration of modelled liabilities.

Figure 8. Variation of risk margin with and sensitivity to risk-free rates.

This volatility of the risk margin to interest rates represents one of the challenges in managing it in a rapidly changing interest rate environment. Interest rate changes have a dual impact on risk margin. With a reduction in the risk-free rate curve, firstly the BEL is re-valued upwards and so is the stressed BEL to calculate the individual risk capital, resulting in larger risk capital.

This effect is amplified for firms using MA or VA, as these measures are ignored for the purpose of the risk margin calculation. The BEL and stressed BEL used to calculate the risk capital for risk margin purposes cannot use the MA or VA, thereby increasing RM SCR in comparison with the SCR for Pillar 3 disclosures.

Secondly, the costs of capital are discounted at lower rates, resulting in a higher overall present value for the risk margin.

5.4 Assessment of Alternative Risk Margin Proposals

We have used the model to assess and present the financial impact of certain alternative options, and where relevant we have shown this impact relative to the base results. Furthermore, to assess whether the alternative proposals are helpful to mitigate the volatility issues highlighted in earlier sections, we have presented the results over time to show the volatility of the proposal in comparison to the base result.

The summary results are presented throughout section 6..

6.1 Cost-of-Capital Rate

The Level 2 Delegated Regulation, rather than the Level 1 Directive, specifies the 6% cost-of-capital rate used in the risk margin.

As stated in section 3.5.1, EIOPA (2018) focused in the review almost entirely on the cost-of-capital rate, and the conclusion was that if anything 6% was too low:

Not surprisingly, this conclusion received, and indeed continues to receive, significant pushback from the industry, who have consistently argued that 3−4% would be a more appropriate rate, as was typically used for embedded value calculations.

Table 2 sets out summaries of the key points made in support of a lower rate, EIOPA’s responses and the views of the working party relating to the responses from EIOPA.

Table 2. Proposals for a Lower Cost-of-Capital Rate

Offsetting these reductions is the view that the cost-of-capital rate could well increase following an extreme stress, that is, in the scenarios envisaged within the SCR calculation. Although not required by regulators to date, the SCR is meant to represent a stress to the full balance sheet, including the risk margin, and that firms should be required to reflect this. (Note that we are concerned here only with the cost-of-capital rate and not the cost of capital overall. It may well be that following a large stress, the non-hedgeable SCR itself reduces materially.)

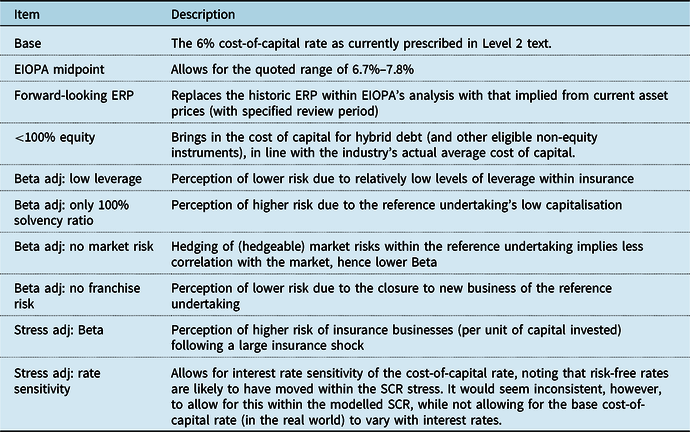
It is noted, however, that the 6% cost-of-capital rate will be reviewed as part of the 2020 EIOPA review. While we do not express a view here on the rate itself, we set out below the analysis steps that we believe should be allowed for. This is on the assumption that a cost-of-capital approach, calibrated independently of reinsurance pricing or market transaction data, is retained and is notwithstanding the various weaknesses of the cost-of-capital approach described elsewhere in this paper. Figure 9 is illustrative only and not to scale.

Figure 9. Illustration of steps that should be considered in defining the cost-of-capital rate.

Each step in Figure 9 is described in Table 3.

Table 3. Descriptions of Steps in Figure 9

The members of the working party do not have a consensus view on whether, having regard to the above factors, the current cost-of-capital rate of 6% is correctly calibrated.

A solution was also proposed and designed to reduce the sensitivity of the risk margin to interest rates, namely that the cost-of-capital rate (which it should be noted is already an excess cost over risk-free) should depend on the risk-free rate:

(2) $$Cost \hbox- of\hskip-1pt\hbox-capital = \left[ {x\% *risk \hbox-free\,rate} \right] + \left[ {y\%\ fixed\, addition} \right]$$

The Chief Risk Officers Forum (CRO Forum, Reference Forum2008, page 35) researched the cost-of-capital rate during the development of Solvency II. The Forum’s report includes

This suggests that x% might be 30−40%.

The field testing of ICS described in IAIS (2018), discussed in more in detail in section 7.7, is also considering an approach where the cost-of-capital rate = 3% + risk-free rate (i.e. x% = 100% in the formula above), albeit the working party is unaware of any theoretical justification for this particular choice.

EIOPA (2018, page 347) also argued that “empirical and academic evidence to support a theoretical link between the risk-free rate and the equity risk premium is mixed” and quoted the work of Damodaran (2016), amongst others, which suggests that although long-term historic data suggest a positive relationship between the equity risk premium and the risk-free rate, more recent experience, if anything suggests no relationship or even the opposite.

We note that while allowing the cost-of-capital rate to itself vary with interest rates would reduce the sensitivity of the risk margin to interest rates, it could add to practical hedging difficulties.

6.1.1 Working party survey on cost of capital

In our survey, we asked firms if they would support making the cost-of-capital rate dynamic that is linking cost of capital with the interest rate environment and/or firms’ actual cost of capital, and the reasons why.

As shown in Figure 10, a large majority supported making the cost-of-capital rate dynamic. The respondents who did not support it explained that it may not work for their block of business – potentially making managing the risk margin more difficult.

Figure 10. Support for dynamic cost-of-capital rate.

The main points gleaned from the respondents were

• Help with interest rate sensitivity – it may help with volatility or reduce the size of risk margin, possibly making volatility impacts less material.

• Theoretical arguments – intuitively, it seems reasonable for the cost-of-capital rate to have some dependency on the level of market interest rates, since investment decisions are made within the wider economic context including the level of risk-free rates.

• Ease of implementation – a change to the cost-of-capital rate would probably be the easiest to achieve with almost no to little change.

• Potential challenges – however, depending on the how cost of capital is linked to interest rates – it may be difficult to identify suitable parameterisation of the cost-of-capital rate to achieve the desired risk margin behaviour for different lines of business. If not considered carefully, we might end up in situation where risk margin behaviour could be unintuitive, for example, a rising risk margin as interest rates increase.

6.1.2 Model results on cost of capital

Figure 11 presents the model results for relative movement of RM/BEL as a percentage of the December 2015 ratios for a change in the cost-of-capital rate from the current 6% to being calculated as 35% of risk-free interest + 5.4%, where 5.4% is chosen so that the formula provides similar results to the base at December 2015.

Figure 11. Impact of changing the cost-of-capital rate to dynamic.

The results are shown relative to the base results.

The results show that relative to the base position, the change in cost-of-capital parameters will result in lower volatility in the risk margin. The proposal results in 50% lower volatility over this time period when measured by the standard deviation of RM/BEL.

In practical terms, this alternative will result in different cost-of-capital rates being applied to the amount of capital for every period. Note that the cost-of-capital rate (based on average duration for the modelled portfolio) for December 2018 is just under 6%. However, during times of high interest rates, this alternative could also result in materially higher risk margins.

6.2 Allowance for Longevity Reinsurance/management Actions

One key argument made against the risk margin for annuity business is that longevity is, unlike some other demographic risks, largely hedgeable.

The Comité Européen des Assurances (CEA) and the Groupe Consultatif Actuariel Européen (Groupe Consultatif) define a hedgeable risk under Solvency II as (see CEA and Groupe Consultatif, 2007, page 31):

Longevity risk arguably falls within this definition.

However, although the exclusion of hedgeable risks was a major criterion in the development of the Solvency II risk margin, the wording of the regulations took a different route. The concept of hedgeable risk is not mentioned in the Level 1 Directive or Level 2 Delegated Regulation at all. Rather, in the Level 2 Delegated Regulation, the SCR used in the risk margin projections is calculated under the assumptions that:

• market risk is minimised (by de-risking assets and, when required, by hedging), but

• the underwriting risk is the same as for the transferred business, allowing for reinsurance in-force.

Therefore, under the current Solvency II regulations, longevity risk cannot be excluded from the risk margin even if it is considered to be hedgeable.

When the ABI gave its initial evidence to the Treasury Committee, it revealed that the industry, in particular Legal & General, had made a detailed proposal to the PRA to allow for the ability to reinsure longevity in the risk margin within the constraints of the Level 1 and Level 2 text.

At the Treasury Committee’s request, the detailed proposal was published as part of the further written evidence (ABI, 2017).

The proposal was outlined to EIOPA in ABI (2018).

In summary, this involves an insurer approving a management action that provides that it would seek reinsurance to cover certain liabilities in specifically defined circumstances, namely when it de-risks its assets.

Owing to the provision in the Delegated Regulation that the reference undertaking is assumed to adopt management actions consistent with those of the insurer, those defined circumstances would be deemed to occur upon the transfer to the reference undertaking, and therefore, the reference undertaking would be treated for the purposes of the risk margin calculation as having put in place longevity reinsurance in line with the management action.

That management action would be reflected in determining the reference undertaking’s SCR for the purposes of calculating the risk margin.

In practice therefore, the longevity risk part of the risk margin would be replaced by the expected cost of reinsurance, allowing for a period to put the reinsurance in place.

This would have the benefit of producing a risk margin consistent with the market price for longevity risk, which would seem consistent with the intention of the risk margin.

As a consequence:

• the resulting risk margin would typically be lower than the present method, in current conditions; as indicated in section 3.1.3, we understand anecdotally that the market cost of reinsurance for a typical annuity allows for around 5% margin above the BEL, depending on the characteristics of the portfolio;

• the resulting risk margin would be significantly less sensitive to interest rates; and

• this would also avoid artificial incentives to transfer risk outside of the UK/EU.

The disadvantages of the approach, which we understand that the ABI acknowledged in its discussions with the PRA and intended to address as part of any implementation, include

• technical provisions would potentially be dependent on fluctuations in reinsurance market pricing. Insurers might argue for a through-the-cycle average given the long-term nature of the risk margin calculation but that would then add back incentives to transfer risk in times when market pricing was particularly competitive;

• the reinsurance market is arguably not sufficiently deep, liquid and transparent for market pricing to be readily and reliably observable, particularly for insurers who do not participate in the market (or who would not participate if this solution were permitted); and

• not all longevity risk is easily hedgeable (e.g. deferred annuities) which would raise the question of where the boundaries of this approach should be drawn.

The PRA (2017) initially rejected this proposal, arguing in its response to the Treasury Select Committee that:

• the management action proposed was hypothetical and would apply only in very specific circumstances;

• it would therefore reduce the risk margin without any corresponding reduction in actual risk;

• the future actions would be binding even if they might not be economically prudent;

• the use of such future hypothetical actions might be applied elsewhere to reduce Solvency II capital requirements.

As discussed in section 3.5.2, the PRA later became more sympathetic to this, or similar proposals, but put any implementation on hold pending clarity on the UK’s future relationship with the EU.

6.3 Inclusion of Matching Adjustment in Risk Margin

The Level 2 Delegated Regulation requires the future costs of capital to be calculated using the basic risk-free rate.

However, it is silent on the use of the MA or VA in the calculation of the RM SCR.

EIOPA (2015, page 3) addressed this issue in the Guidelines on the implementation of the long-term guarantee measures, where it states that

For UK annuity books, where the MA is typically used for the BEL, this means that in the risk margin the projected SCRs held for longevity risk are based on the increase in technical provisions calculated at the risk-free rate, rather than using the MA, and hence are significantly higher.

One seemingly easy change, which requires only changes to EIOPA’s guidelines, is that this restriction be relaxed and that insurers using the MA (or VA) can assume that the reference undertaking does so.

However, the history of the EIOPA consultation on the Guidance shows that this is actually not so straightforward. In order to apply the same level of MA as the insurer, the reference undertaking would need to hold to maturity a similar portfolio of credit assets.

This would logically require, as indeed was initially proposed by EIOPA in the first drafts of its Guidance, that the SCRs projected for the reference undertaking should include a SCR for the credit risk associated with the assets supporting the MA, which would materially offset any gain.

The industry also raised the issue of the discount rate used to value the projected costs of capital, but noted that this required a more fundamental change to Level 2. The proposal made by one commentator to set the discount rate to the cost-of-capital rate (see section 6.6) is also relevant here.

6.3.1 Working party survey on inclusion of long-term guarantee measures in risk margin calculation

We asked firms whether they would support use of VA and MA in calculation of RM SCR and why. We also asked whether they would support use of VA and MA in the risk-free rates to discount the future cost-of-capital amounts.

The survey results are presented in Figure 12, along with the main points put forward by the respondents.

Figure 12. Support for allowing for VA and MA in risk margin calculations.

VA in RM SCR

The main points in favour were that:

• VA may not have a material impact on the risk margin but possibly applies to a wider range of firms;

• there is some theoretical justification for this, since a third party taking on the liabilities would look to minimise its capital requirements and use the VA to do this in the same way that the current undertaking does; and

• it would also reduce calculation complexity by removing the need to calculate SCR with and without VA.

The main points against were that:

• it is not clear to respondents whether the proposal will really mitigate volatility in the risk margin; and

• applying VA to RM SCR, without including RM SCR for spread risk, seems contradictory. It is unclear how VA can be earned with a fully spread risk-free portfolio.

MA in RM SCR

Even respondents who supported the proposal highlighted that it may not be totally consistent with the non-hedgeable SCR approach that implies a reference portfolio based on de-risked assets. The main issues highlighted were

• consistency with the transfer scenario being envisaged in the rules, unless credit risk inherent in the MA was included in SCR run-off calculations or the MA allowed was reduced by removing credit risk;

• although the proposal may reduce the size of risk margin, it is not clear whether it will really mitigate its volatility;

• applying MA to RM SCR, without including in RM SCR for spread risk, seems contradictory. It is not clear how can the MA be earned with a fully spread risk-free portfolio.

VA/MA in risk margin discounting

Although about 50−60% of respondents supported these proposals, it is clear from comments that they were less convinced, with the main points being:

• while some assets may be available providing returns above swaps with minimal additional risk, it is possible that the reference undertaking’s assets backing liabilities might not support the use of a VA and in particular the MA; and

• inconsistency with the existing Level 2 Regulations

6.3.2 Modelling results on inclusion of long-term guarantee measures in risk margin

The working party’s model shows that including MA allowance in risk margin does not help materially with the volatility of the risk margin. In comparison with the base position, the proposal reduces the volatility by less than 10% (as measured by standard deviation of RM/BEL) from the implementation of Solvency II to the end of December 2018.

However, the proposal helps with the size of the risk margin. Figure 13 presents the model results for RM/BEL ratios where the risk capital for the risk margin (RM SCR) includes allowance for MA relative to the base results. The figure also shows the impact on the risk margin, if MA is allowed for in the discount rate as well as in RM SCR.

Figure 13. Impact of allowing for MA in the SCR and discount rate for risk margin calculations.

To show the results over time, we have assumed a constant MA throughout this period.

The results show that inclusion of the MA in calculating RM SCR reduces the size of the risk margin by 14%, whereas allowing for MA in the discount rate in addition results in an overall reduction of about 23%.

However, as noted above, there are theoretical challenges to allowing MA within the discount rate to calculate risk margin.

In accordance with our model, allowing for the credit risk associated with the assets held to maturity to back the SCR would result in an increase in RM/BEL% (with MA in discount rate) from 6.5% at December 2018 to 7.8%, thus removing most of the benefit of allowing for MA.

6.4 Diversification

Recital 18 of the Level 2 Delegated Regulation states that the risk margin “should take the diversification of the whole portfolio into account”.

However, the detailed rules require that this is not applied when an insurer writes life and non-life business, with the two blocks of business assumed to be taken over separately by two different reference undertakings, although we note that this is consistent with EU regulation not permitting new composite insurers.

Similarly, for a group, the risk margin is calculated at entity level, but for group purposes the insurer is not allowed to assume additional diversification benefits apply, but must simply add the risk margins from each group entity.

Both of these assumptions are inconsistent with the calculation of the SCR and appear artificial.

Indeed, the working party notes that for a properly market-consistent and objective calculation of the risk margin, the capital required for each risk and the diversification taken into account should be a market level of capital and diversification. This point was made by the IAIS (2006, page 10) in its review of the IASB’s accounting standards project and was quoted in CEIOPS’ (2007, page 31) Advice to the European Commission (see section 1.3 of Appendix B):

One could argue that one reason that the risk margin, as calculated under Solvency II, exceeds the market price of longevity risk transfer into the international markets is that the Solvency II calculation gives no credit for the diversification benefit relating to longevity enjoyed by international reinsurers who, for example, may have significant exposure to senior life mortality risk in the United States (see also section 3.1.3).

6.5 Non-Independence of Risks

One key point raised by the industry in its response to EIOPA is that the method of projecting the SCRs can lead to an overstatement of the risk to the reference undertaking. To illustrate this, the ABI (2018) submission to the EIOPA consultation includes an example of an annuity where the risk margin, calculated in accordance with the Solvency II standard formula, provides more capital over time than would be required to cover the risk of zero mortality.

Essentially, the risk margin as construed assumes that the reference undertaking is recapitalised at each period end and only holds capital against risk for the next year. The SCRs projected are unconditional on events in previous periods.

However, in practice, the risks in each period over a product lifetime are not independent. For instance, certain risks are non-repeatable: that cancer can only be cured once is a simple example. Therefore, if there were losses in one period, the SCRs in future periods might be expected to be lower. This is important, as the rationale for a 6% cost-of-capital rate in the risk margin calculation is that the capital injected each period is at risk – if losses in one period would mean lower SCRs in future periods, then either the cost of capital may be too high or the projected SCRs too high. To reflect this properly would require a full stochastic projection of the future evolution of the business.

The ABI proposes that an alternative approach, and one more consistent with how an actual reference undertaking would price a transaction, might be to allow for an “extreme but plausible lifetime shock”. The simple approximation proposed to deal with this is to allow in the projected SCRs for a time scaling factor, where a tapering parameter λ (0 < λ < 1) is applied to future projected SCRs:

(3) $$SCR'\left( t \right) = {\lambda ^t}*SCR\left( t \right)$$

where SCR(t) is the unconditional projected SCR at time t and SCR’(t) is used in the risk margin.

The detailed proposal is the subject of a separate ABI paper “Addressing the Size and Volatility of the Solvency II Risk Margin Using a Tapering Approach”, which is summarised in ABI (2018), page 47. The ABI did not make this paper available to the working party, so we are unable to comment in detail – in particular on how the choice of λ could be justified or even whether the method is theoretically sound.

The ABI submission to EIOPA included illustrations with λ set equal to 0.97, 0.92 and 0.84, with high-level explanations for each. However, it is our view that an assumed 16% or even 8% reduction in SCR in every future year would be difficult to justify on the grounds of non-independence of future risks and would clearly make a very large difference to the result.

The working party notes that the theoretical justification for this tapering approach rests on the argument that the cost-of-capital rate cannot be 6% for capital that cannot be lost.

In practice, the proposal is equivalent to increasing the discount rate used in the risk margin calculation to

(4) $$(1 + risk \hbox-free)/\lambda - 1$$

There are therefore some similarities with the proposals of Hans Waszink discussed in section 6.6.

We have estimated the impact of this approach by assuming λ = 97.5%, that is, in every future projection year, the SCR would reduce by 2.5%. See Figure 14.

Figure 14. Impact of allowing for non-independence of risks (λ = 97.5%).

It can be seen that it helps with the magnitude of the risk margin. Furthermore, this also marginally helps with volatility when compared to the base position.

6.6 Hans Waszink’s Proposal

An interesting contribution to the risk margin debate was made by Waszink (Reference Waszink2013) in a paper presented to the Actuarial Studies in Non-Life Insurance Colloquium. He argues that:

• the cost-of-capital method fails to satisfy a number of desirable properties, in particular that it has no upper bound related to the SCR (or to the maximum value of the liability); and

• the issues can be resolved if the cost-of-capital rate is also used as the discount rate in the risk margin calculation, which he reasoned was the theoretically correct approach.

Waszink also made similar arguments to the EIOPA consultations in 2009 and 2017, but it rejected his suggestions, opining that the risk margin could theoretically exceed the SCR.

The Waszink proposals and EIOPA response have also been discussed in the pages of The Actuary magazine, being promoted by Woods, B. (Reference Woods2016; Reference Woods2017).

The working party respectfully disagrees with the arguments made by Waszink and Woods, as outlined in our reply in The Actuary (Risk Margin Working Party, 2018). We note the reply from Woods, B. (Reference Woods2018), but would still argue as follows:

The risk margin is determined as the cost of capital required by the reference undertaking which receives the whole of the firm’s liabilities for run-off and is itself subject to Solvency II. The reference undertaking must itself hold a risk margin, because of the risk of losing its own capital. Waszink developed his approach on the basis that the risk margin should not exceed the SCR. We do not believe that this approach is theoretically justified, as it assumes that the risk capital cannot be lost again during run-off and ignores the requirement for the reference undertaking itself to hold a risk margin, as well as the requirement of the reference undertaking to finance its SCR on an ongoing basis even where it is never lost.

However, as indicated in section 3.3.3, the risk margin is based on the implicit assumption that the security of policy benefits is to full Solvency II standards during run-off. Although this assumption could be challenged, our views above are based on the belief that at present Solvency II clearly intends the assumption to hold.

6.7 Summary

Figure 15 summarises the modelled estimate of the risk margin as percentage of BEL as at 31 December 2018 for the various options discussed in this section.

Figure 15. Summary of impact of various options.

6.8 Amendments to Regulations

These different proposals require different levels of regulatory approval or amendments to the regulation. Our understanding is set out in Table 4.

Table 4. Amendments to Regulations

6.9 EIOPA 2020 Review

As no changes are made as a result of the 2018 review by EIOPA and the Commission (see section 3.5.1), the next opportunity for changes to the risk margin within Solvency II will be in the fundamental review of Solvency II that the Commission is required to undertake 5 years after implementation.

In February 2019, the European Commission (2019) sent a request to EIOPA in connection with this review, which requires EIOPA to respond with advice by 30 June 2020. The input sought is technical and does not necessarily prejudge the outcomes of the review nor prevent wider consultation, but nevertheless we feel it can be taken as a guide to the Commission’s thinking.

Rather disappointingly in the working party’s view, the scope specifically excludes any changes to the cost-of-capital method itself. Indeed, EIOPA has been instructed to “assess the appropriateness of the design of the risk margin, without challenging the approach based on the cost-of-capital” (ibid., page 4).

In our view, some of the issues highlighted in this paper and elsewhere, such as the high sensitivity to interest rates, may stem from the cost-of-capital method itself, as much as any particular calibration. Some of the modifications we have considered to the cost-of-capital method (such as a cost-of-capital rate that varies with interest rates) are more short-term fixes to produce a more market-consistent answer, rather than solving the fundamental issues with the risk margin as currently constructed.

Instead, EIOPA has been asked to revisit many of the issues highlighted but dismissed in the 2018 review, specifically:

• the design of the risk margin, in light of any information on actual transfer prices of liabilities, itself the subject of a separate Call for Information from the European Commission (2018);

• the assumption as to the asset-mix of the reference undertaking, and potential interactions between recognition of market risk and the use of the VA and MA in the risk margin;

• the assumptions supporting the cost-of-capital rate, in particular the absence of leverage and the derivation of the equity risk premium; and

• the use of a fixed cost-of-capital rate for all insurers and reinsurers.

It is not clear to us whether the scope of these questions, and in particular the final bullet point, might extend to considering a cost-of-capital rate that varies with interest rates or a technique similar to the ABI’s tapering suggestion.

Notwithstanding the above scope, in section 7, we consider potential alternatives to the cost-of-capital method.

7.1 Alternatives Considered in Solvency II – Run-off Percentile

As noted in Appendix B, when Solvency II was originally being calibrated, an alternative to the cost-of-capital method was considered, a percentile approach. Here, the risk margin was given by the difference between the 75th percentile of the underlying probability distribution (over a full run-off) and the BEL, subject to a minimum of half a standard deviation to allow for strongly skewed distributions.

The choice of 75th percentile was taken as an appropriate level of confidence for a firm to be able to meet its liabilities over a full run-off after an adverse event. However, CEIOPS (2007) noted that there was no evidence that this was consistent with the market price for transferring liabilities to a third party. Nevertheless, the results of the second quantitative impact study (QIS 2) suggested that, in aggregate, this method produced similar results to the cost-of-capital method for the EU insurers who participated in the study.

It was assumed – in contrast to the VaR methods discussed in section 7.3 and the Prudence-MOCE (P-MOCE) and Percentile-MOCE being considered in ICS (see section 7.7) – that this 75th percentile would be based on a full run-off distribution and would not necessarily be lower than the SCR, which is based on a 99.5th percentile but over only a 1-year time horizon.

CEIOPS noted that this provided protection in a run-off scenario (see section B1.3 of Appendix B), but ultimately rejected this approach on the basis that there was no evidence of a link to actual transfer prices in the market. Indeed, the theoretical rationale for this approach is more an “own fulfilment” value than an attempt to reproduce a market-consistent exit value.

However, as noted in section 8.1.2, the cost-of-capital method that was adopted instead may be more market-consistent in theory, but does not necessarily appear to be so in practice.

Note also that if this percentile were set at 50%, that is, approximately equal to BEL (technically a 50th percentile is only equal to BEL if the mean equals the median of the distribution), then there would be no risk margin (see section 7.6).

7.2 Provision for Adverse Deviation (PAD)

Traditionally, when actuaries were asked to allow for uncertainty in setting assumptions, the approach would have been to add a margin, or PAD, to the best estimate assumptions. Typically, a PAD would be set individually for each assumption, for example, mortality, expenses, etc. These PADs were often judgemental and not set with detailed regard to any probability distribution, nor was there any requirement to calculate the equivalent confidence level for a given set of PADs. Often, diversification benefits were also not taken into account between different risks, that is, to the extent that the PADs did represent a level of confidence, this was typically assessed for each risk in isolation, but then the stresses were applied simultaneously.

In modern actuarial terms, such an overall set of prudent assumptions could be considered as a single combined scenario of risk factor realisations, analogous to a scenario generated by an internal model, albeit fully specified rather than random. In theory, it would then be possible to determine the implied confidence level for a given set of PADs by referencing the internal model capital distribution. If a certain confidence level were to be targeted, the actuary could then adjust some or all of the individual PADs until the desired outcome was achieved. This process might seem a little onerous, however, and is open to the obvious challenge that if the capital distribution is already known, why not simply pick the desired point from that distribution without the need to derive a set of equivalent PADs. This leads us directly to the VaR approaches described in section 7.3, although these approaches do not in general rely upon the existence of an internal model or knowledge of the full capital distribution.

We note in Appendix E, section E3 that a PAD type approach is currently favoured by the regulator in China as part of its new regulatory regime, where a set of combined stresses is prescribed.

Where PADs are set simply for prudence, rather than via some theoretical justification such as to make liabilities market-consistent, then there is an argument that there is some double counting with capital requirements. See section 7.7.1 for further discussion on this topic.

7.3 Value-at-Risk

VaR is the approach required to determine the SCR under Solvency II. It is also known as the confidence level approach. In this approach, the insurer sets its reserves equal to the level at which it is X% confident of remaining solvent over an N-year time horizon. For example, for the Solvency II SCR, X has a value of 99.5 and N is equal to 1 year.

However, VaR approaches can also be used to set the risk margin held in technical provisions on top of BEL. Anecdotally, this is the approach being considered by a number of insurers for the risk adjustment under IFRS 17 (see section 8.1.7 for further discussion).

The Solvency II alternative approach discussed in section 7.1 involves setting X to 75% and N to full run-off of the business.

However, emerging international practice, including ICS, seems to look instead at 1-year time horizons.

We note in section 7.7 that the current field testing of ICS is considering a Percentile-MOCE as an alternative to a cost-of-capital method, based on a range of 1-year percentiles for insurance risks. The field test also suggests a practical way in which these percentiles could be derived without needing a full loss distribution, by simply assuming that overall capital requirement has a normal distribution. As the normal distribution is thinner-tailed than many other distributions commonly used in insurance, this implies a relatively high ratio of the 75th to 99.5th percentile. Of course, while not necessarily applicable to ICS, firms employing internal capital models to produce VaR estimates at a given percentile would not need to apply such simplifications.

The benefits of the VaR approach over the cost-of-capital approach include

• computational simplicity, indeed the starting point of the cost-of-capital calculation is the derivation of a VaR-based non-hedgeable SCR. Furthermore, it is possible to derive the risk margin, and hence technical provisions, independently of the SCR;

• lower interest rate sensitivity, when compared to a cost-of-capital approach in which the cost-of-capital rate is interest rate invariant.

As with the PAD, some argue that such risk margins involve double counting with the SCR. The working party disagrees, as outlined in section 7.7.1.

The confidence level for the risk margin VaR calculation would likely be specified by the regulator, whether that be EIOPA or the PRA post-Brexit. This differs from IFRS 17, where the calculation is principles-based and the confidence level is based on firm-specific risk appetite.

In section 8.1.9, we consider further the PRA objective of maintaining Solvency II Equivalence, and this would of course have implications for the calibration of any VaR confidence level. Such a confidence level would likely be well below the 99.5th percentile implicitly within the cost-of-capital calculation, as the latter reflects a cost of financing capital as opposed to capital per se; however, this does mean that the resulting risk margin is by definition less that the corresponding SCR for insurance risk.

Under the general VaR approach, there are two main potential calculation methods:

• correlation stress testing; and

• use of an internal model.

The former is akin to the standard formula calculation for the SCR, albeit with different parameters and in-scope risks. It aims to calculate the required percentile stress directly, through stressing individual risk factors and applying an output correlation matrix to these results. It implicitly assumes multivariate normality of the risk factors, as well as linearity of the aggregate loss function with respect to the risk factors.

The latter involves generation of a full capital distribution, from which the required percentile or confidence level can be read off, and may be preferable to firms who have invested heavily in internal model development for the SCR calculation.

7.4 Run-off Capitalisation

Some of the logical flaws in the risk margin highlighted in section 6 result from the way in which the SCR is projected over time, as a succession of 1-year costs of capital.

As stated in section 6.5, the ABI (2018) suggested that the projected SCRs should instead be based on an “extreme but plausible lifetime shock”.

A more appropriate method to derive the total capital requirement for an insurer might look at the lifetime capital required to run-off the book at a defined confidence level, for example, 90% or 95% over a lifetime.

This percentile could be calibrated to a similar level of confidence, for example based on credit rating default data, as the 1-year 99.5th percentile, and be dependent on the term of the run-off.

This could potentially replace both the SCR and the risk margin, thereby absorbing the risk margin into the capital requirements. Alternatively, a risk margin for non-hedgeable risk could still be required within the technical provisions, both in the base balance sheet and in the projected balance sheet simulations. In the latter case, a relatively simple formula for the risk margin would be required. Either way, a run-off approach to capital would represent a more fundamental overhaul of the Directive.

Some insurers used a run-off time horizon in their Pillar 2 Individual Capital Assessment (ICA) under Solvency I, but these suffered from a number of drawbacks or practical concerns at the time. Firstly, the Pillar 2 rules stipulated that firms using run-off models (with some derived run-off confidence level) had to demonstrate that the resulting capital was no weaker than a 1-year one-in-200-year assessment. Secondly, it became clear in the run-up to Solvency II that the new regime would require a 1-year one-in-200-year assessment. A further modelling challenge that existed at the time was around demonstrating intermediate solvency, that is, although the resulting capital may have been sufficient (in, say, 95% of scenarios) to ensure that the firm had positive assets at the end of the book’s lifetime, it was by no means clear for all “pass” scenarios that the firm remained continuously solvent throughout the projection period.

There are some similarities between this and the run-off percentile method (described in section 7.1), except that here the risk margin and SCR would be combined, and the calibration made to an overall failure probability, at a lower (i.e. more prudent) probability of failure.

One example of a proposed regime of annuity-like liabilities based on run-off capitalisation comes in the proposed regime for superfund consolidation vehicles, as per the Department of Work and Pensions (DWP) consultation (DWP, 2018). It essentially proposes regulating these vehicles based on a 99% probability of solvency over the full run-off, with no separate risk margin. The DWP (2018, paragraph 24) define superfunds as:

• a superfund is, or contains, an occupational pension scheme set up for the purposes of effecting consolidation of defined benefits pension schemes’ liabilities;

• a transferring scheme’s link to a ceding employer is severed on transfer to the superfund;

• the “covenant” is a capital buffer provided through external investment that sits within the superfund structure; and

• there is a mechanism to enable returns to be payable to persons other than members or service providers.

Developments in this framework could provide a useful pointer for insurance firms which write pension buyout business, which in many respects share similar characteristics, albeit under an insurance rather than occupational pension scheme regime.

7.5 Market Reinsurance Pricing

The risk margin is defined in Recital 55 of the Solvency II Directive as being a margin added to the BEL to ensure technical provisions “correspond to the amount an insurance or reinsurance undertaking would have to pay if it transferred its contractual rights and obligations immediately to another undertaking”.

Therefore, where there is clear evidence of a reliable market price, one could argue that this should be used in place of the prescribed calculation, in the same way that other aspects of the Solvency II assets and liabilities are consistent with observable market prices.

We would contend that this applies for large parts of the longevity risks retained by insurers, and indeed the ABI suggestion discussed in section 6.2 would have essentially replaced the risk margin with a (prudent) market cost via a technical construct in the rules.

We would note that, even if international reinsurers followed a method conceptually similar to the risk margin, they would be unlikely to get a similar answer as:

• the reinsurer’s own cost of capital may differ from the prescribed 6%;

• for non-EU insurers, capital requirements are unlikely to be the same as the Solvency II SCR; and

• reinsurers would typically benefit from greater diversification, for example, against mortality risk on existing books, whereas the risk margin assumes transfer to a new entity.

7.6 No Risk Margin/loading to SCR

Under the Solvency I Pillar 2 ICA, UK insurers were not required to hold a risk margin on top of BEL. Arguably, this could be justified by prudence in the Pillar 1 calculations, but this argument is weaker where the Pillar 2 requirements were the binding constraint.

Indeed, it is the presence of the risk margin in Solvency II technical provisions but not the Solvency I ICA that has given rise to a large TMTP for many UK annuity writers.

As noted in section 3.5.2, in its submission to the Treasury Committee, the IFoA questioned whether the risk margin under Solvency II provided excessive security to policyholders to the detriment to consumer choice and costs.

In particular the current risk margin, when combined with the SCR, means an insurer should be able to withstand a one-in-200-year event and then still have sufficient assets to transfer liabilities to another insurer who would be (re-)capitalised from the risk margin to be able to withstand further one-in-200-year shocks.

An alternative would be to argue that after this one-in-200-year event, policyholders should expect no more than that the firm has assets equal to the BEL, with no additional capital. There would then be a c.50/50 chance of having benefits provided in full from the resources of the firm, with any shortfall potentially borne by industry compensation schemes. It is the view of the working party that this alternative is inadequate.

If this level of security is indeed deemed inadequate, a further alternative would simply be to increase the level of the SCR requirement to a higher level of confidence. As noted previously, the risk margin represents a reduction in capital resources, rather than an increase in capital requirements; however, it has the same bottom line impact.

Another alternative to add more prudence would be to increase the chance of having benefits provided in full following the one-in-200-year SCR event to a desired confidence level above 50%, which essentially leads back to the run-off percentile methods discussed in section 7.1.

7.7 International Capital Standards – Margin over Current Estimate

The IAIS is developing a framework for ICS. This also includes a MOCE, similar in concept to the risk margin.

As documented in IAIS (2018; 2019), the 2018 and 2019 field tests of the ICS include three approaches to the MOCE.

1. 1. Cost-of-capital MOCE (C-MOCE)

   This method is essentially consistent with that used currently in Solvency II.

   However, two different parameters for the cost-of-capital rate (in excess of the risk-free rate) are being tested.

   The main approach is to use a cost-of-capital rate of 5%, similar to but lower than Solvency II rate of 6%.

   The alternative is to consider an adjusted cost-of-capital rate dependent on risk-free rates: specifically, a cost-of-capital rate (in excess of risk-free) of risk-free + 3%, with a floor of 3% and cap of 10%. See section 6.1 for a discussion of why the cost of capital might itself vary with risk-free rates. The working party’s view is that it is difficult to justify an excess cost-of-capital rate varying one-for-one with risk-free rates, and that a more reasonable approach may lie between the two parameterisations tested in the 2018 field test.

2. 2. Prudence-MOCE (P-MOCE)

   The P-MOCE, as an alternative to a cost-of-capital method, adds a margin to the best estimate for parameter uncertainty.

   An estimated standard deviation for insurance liabilities is computed using the relevant aggregated ICS risk charges as a proxy for the stressed current estimate and assuming a normal distribution for the current estimate. Specifically, the standard deviation is assumed to be the ICS risk charge for insurance risk charge/2.576, since under the standard normal distribution, the 99.5th percentile is 2.576.

   The P-MOCE is then taken as 66.7% of that standard deviation, which is taken as approximately equal to the 75th percentile. (The 75th percentile for the standard normal distribution is actually 0.6745).

   Hence, overall the P-MOCE is simply calculated by taking the SCR equivalent (ICS risk charge) for insurance risk and multiplying this by 0.259 (66.7%/2.576).

3. 3. Percentile-MOCE

   The Percentile-MOCE was introduced in the 2019 field tests. For life business, it is identical to the P-MOCE, except that it is not regarded as loss absorbing – see section 7.7.1. The field tests are for calibrations of 80th and 85th percentiles as well as 75th percentile.

The default methodology in the 2018 field tests was to use the C-MOCE at a 5% cost-of-capital rate. The 2019 field tests included only the Percentile-MOCE

8.1 Evaluation Criteria

The working party found it instructive to derive criteria for assessing the performance of the current Solvency II risk margin and for assessing the merits and weaknesses of alternative proposals.

In particular, in doing so, we have returned to the original Solvency II Directive and to the stated objectives of Solvency II in general and the risk margin in particular, including those originally proposed by the Groupe Consultatif (2006). We have also updated these in light of experience since (such as the global financial crisis, which highlighted the importance of avoiding procyclicality) and international developments.

We suggest the following are key criteria to be considered. Note that in some cases, these might conflict and we comment on cases where we believe the criteria might need to be relaxed in order to achieve the best overall aim.

8.2 Summary of Evaluation of Current Solvency II Methodology

We can summarise our evaluation of the current Solvency II methodology in Table 5, where we consider each of the 11 criteria detail in section 8.1.

Table 5. Evaluation of Current Methodology

✓denotes a strength of the method, and ✓✓ a particular strength;

x denotes a weakness and xx a particular weakness; and

? denotes where there is some ambiguity

✓x or similar can show where a method has both a strength and weakness in the same category (e.g. that the cost-of-capital method is theoretically market-consistent but seems inconsistent with the price at which risk transfer actually takes place in practice)

8.3 Evaluation of Alternatives

8.3.1 Re-calibration of cost-of-capital method

In this section, we appraise some of the key variations on the cost-of-capital method that we discussed in section 6.

In Table 6, we show our appraisal of these various alternatives on our 11 criteria from section 8.1.

Table 6. Evaluation of cost-of-capital alternatives

Note that all comparisons are relative to the existing Solvency II methodology rather than in absolute terms, so they should not be compared to Table 5, and that our main focus is on application to UK annuity business.

This analysis is of necessity simplistic and also caused some debate in the working party, so it should not be taken as a definitive view. Nor do we believe that any attempt should be made to derive an overall score for each approach. However, we believe it provides a useful visual summary.

The table should be read with the commentary below, highlighting key points for each variation.

8.3.1.1 Reducing the cost-of-capital rate from 6% to a fixed lower %

The biggest advantage of this method is its simplicity: it would typically be easy to parameterise in systems and should not create significant additional issues. Many of the issues associated with the current risk margin are reduced in impact, if not all solved.

The theoretical justification for reducing the cost-of-capital rate is debatable, with strong arguments on either side, as discussed in section 6.1. However, comparing current market pricing with the risk margin, the 6% parameter does appear to produce too high an answer, at least in current conditions.

Cost-of-capital rates of 5−6% are also commonly used internationally where the cost-of-capital method is applied, for example 6% in the SST and, under the main option for field testing, 5% in ICS. Hence, a material reduction of the cost-of-capital rate, in either the EU or unilaterally in the UK post-Brexit, could leave the resulting regime out of line.

This change does not reduce the relative interest rate volatility of the risk margin which, as noted in our modelling, appears too high in comparison with our understanding of actual market pricing. However, it does reduce the volatility in absolute terms, and so could lessen the volume of hedging required and hence the market impact.

8.3.1.2 Reducing the cost-of-capital rate from 6% to a level dependent on interest rates

There is some theoretical justification that the cost of capital, in excess of risk-free rates, may itself reduce as risk-free rates reduce, albeit this is not definitive.

The advantages of this method, compared to simply reducing the cost-of-capital rate to a fixed lower figure, are:

• one could defend a re-parameterisation by ensuring that the cost-of-capital rate was 6% when rates were at the levels when Solvency II was originally calibrated;

• the volatility of the risk margin in relation to interest rates would be reduced, which in turns aids counter-cyclicality and may produce a behaviour more in line with market prices; and

• a cost-of-capital rate, in excess of risk-free, of risk-free + 3% was field-tested in ICS, so if calibrated appropriately this could be more consistent internationally.

This approach also may be easier to implement than many other suggestions. However, it does introduce complexities of its own: notably, the resulting risk margin would have an unusual interest rate sensitivity, may have an artificial sensitivity to whichever rate is used to calibrate the cost of capital and may be very difficult to hedge precisely with standard instruments.

8.3.1.3 Allowing for MA or VA when projecting SCRs

At face value, it may seem reasonable to assume that the receiving undertaking uses the same long-term guarantees measures as the transferring insurer. Furthermore, to do so is consistent with the fact that the risk margin does not allow for any change to the BEL between the insurer and the transferee.

The Level 2 Delegated Regulation is silent on this point: current practice instead is dictated by EIOPA Guidelines, so this is a relatively easy change to implement within Solvency II. It might also be less controversial than some others in an Equivalence context post-Brexit. Furthermore, it appears potentially allowable under IFRS 17 and ICS.

Allowing for the VA and MA in projected SCRs would aid counter-cyclicality in that the risk margin would reduce under times of credit market stress, although it would not make a material difference to issues such as interest rate sensitivity.

However, the biggest challenge to this method is the theoretical justification for including the MA and VA, without also allowing for the ongoing credit risk associated with holding the assets that would support the discount rate. Projecting the cost of capital to support this risk is, according to our model, likely to offset much of the benefit from valuing the projected SCRs using the MA or VA.

There could be more theoretical justification if the liabilities, for SCR purposes, were discounted at an illiquidity premium that it is assumed could be earned at minimal risk, from high credit quality but illiquid assets.

8.3.1.4 Discounting future projected cost of capital at other than basic risk-free

This idea potentially encompasses a range from:

• allowing for the MA or VA in the discount rate, similarly to allowing for them in SCRs, on the basis that the risk margin can also be invested in illiquid assets; to

• allowing for much higher discount rate, for example, a discount rate equal to the cost-of-capital rate, as suggested by Waszink (Reference Waszink2013)

The comments for the former are similar to those for allowing for the MA and VA when projecting SCRs – in particular the calculations would need to allow for capital supporting the risk on the assets held to back the risk margin.

The working party is unconvinced of the justification for the latter approach, as discussed in section 6.6.

8.3.1.5 Other areas

We do not consider here the full list of variations, as some, such as allowing for diversification within groups, we see as addressing specific issues rather than changes to the overall design.

The management actions proposal in section 6.2 is really a potential path through the Solvency II rules to achieve a more fundamental change to the risk margin, that is, replacing it with the market price of risk transfer, which we discussed in section 7.5 and consider in section 8.3.2.3.

We are unable to comment in detail on the tapering proposal from the ABI (discussed in section 6.5) since we have not had sight of the justification for the approach.

8.3.2 Alternative methods

In Table 7, we consider the alternative methods in section 8 against our desirable criteria.

Table 7. Evaluation of alternative methods

8.3.2.1 Run-off percentile/Provisions for adverse deviation/Value-at-Risk

In many respects, these methods are all variations on a theme, in that they are attempting to replace the cost-of-capital method with a direct addition for risk to the assumptions in the technical provisions.

The key differences, as discussed in detail in section 8, are that:

• the run-off percentile method focuses on risks over the full term of the distribution, whereas the VaR approach looks at shorter-term risks, typically 1 year as in the SCR and so may be easier to calculate;

• the PADs are applied to individual assumptions, therefore do not reflect diversification, at least at a firm level, and can also be more arbitrary in their calibration. However, it would be possible to make the choice of PADs more scientific and to reflect at least an industry average level of risk correlation (which may, as noted in section 6.4, be in any case more appropriate for a proxy for a market price of risk).

The different variations should each be applicable, on a consistent basis, for a full range of insurance risks.

The exact parameterisation of the methods will dictate issues such as the impact on market consistency, creating the right incentives and policyholder protection.

The run-off percentile makes policyholder protection most explicit in that it calibrates to the desired level of confidence to meet liabilities over time after the SCR has been exhausted.

IFRS 17 is relatively agnostic, so could permit any of these variations, and they are arguably closer to the “fulfilment value” concept of IFRS 17, rather than the “exit value” concept that underpins Solvency II. The key under IFRS 17 is to reflect the compensation the entity itself would require for bearing the risk, rather than a market price.

However, IFRS 17 requires entities not using a confidence level technique (which would include those applying a cost-of-capital method) to provide disclosures as to an equivalent confidence level. The wording of the standard is ambiguous as to what this confidence level means: one interpretation is that this is closest to a “run-off percentile”, in our terminology. However, anecdotally, we believe some insurers are opting for what we could call a VaR approach for the risk adjustment and are interpreting this as equivalent to a confidence level.

ICS field tests also include alternatives to the cost-of-capital method, P-MOCE and Percentile-MOCE, which in our framework equate to the VaR approach. Our analysis of other markets suggests that this approach is gaining ground as the preferred one as Asian markets move to risk-based solvency methods, although the large Chinese market has adopted a PAD approach.

A method permitted in ICS could assist with post-Brexit Solvency II Equivalence, since the UK could argue that it is complying with international standards.

8.3.2.2 Run-off capitalisation

This is the most fundamental change to the existing Solvency II regime and hence the most complex to implement and indeed even design and calibrate – it could be considered more of a Solvency III regime.

In theory, a run-off capitalisation method could be very counter-cyclical as short-term market moves may not necessarily impact the perception of the ability of an insurer to meet liabilities as they fall due. However, the Board and indeed the regulator under such a regime would face a decision as to when they would intervene.

8.3.2.3 Market reinsurance pricing

The use of market reinsurance pricing has the significant advantage that it should be, by definition, market-consistent and is theoretically sound.

It should reduce any artificial regulatory incentives to transfer risks to another regime, although the working party would emphasise that there can be strong economic reasons for such risk transfer.

While not compatible with the letter of the Solvency II Directive, it is arguably compatible with the spirit. The UK could, for example, argue post-Brexit that it would simply be reverting to the original distinction between hedgeable and non-hedgeable risk, and designating (some) longevity risk as hedgeable.

Nevertheless, the proposal does come with significant disadvantages.

Firstly, it does not provide a solution for many other risks such as lapse risk, where complete market risk transfer pricing may not be available. Furthermore, for certain annuity risks, such as deferred annuities, the market is much less deep and pricing less transparent.

Even for annuities in payment, where there is an active market, the market would not satisfy the usual Solvency II deep, liquid and transparent criteria. Without actually completing a transaction, a price is only theoretical and to an extent subjective. This may also cause practical issues for insurers with less access to market pricing. The regulators could prescribe the method for determining the cost of reinsurance, but depending on how this was specified (e.g. as fixed margins on best estimate assumptions), this could make the method closer to the PAD or other approaches.

Depending on how the price is derived and recalibrated over time, procyclicality could be introduced if the risk margin itself became sensitive to supply and demand in the longevity risk transfer market, and, inter alia, to reinsurers’ own cost of capital.

While in theory providing policyholder protection, since the risk margin covers the cost of market risk transfer, reinsurance could become more expensive, or may not even be available, after an event that had caused a large loss of own funds for one or more insurers.

Given the above two points, there is therefore an argument that the market reinsurance pricing used should be a prudent through-the-cycle price, rather than the current price, albeit that then moves away from pure market consistency.

If this solution were adopted, insights into how international reinsurers price longevity risk transfer in practice would be helpful in terms of calibrating the risk margin, and this might represent a useful investigation for EIOPA or PRA.

The use of market reinsurance pricing also appears inconsistent with ICS as drafted, and also with IFRS 17, given the emphasis there on the entity’s own fulfilment cost.

8.3.2.4 No risk margin/loading to SCR

This method is clearly simple and solves the issues with the risk margin by simply eliminating it.

However, the working party would question whether this really provides adequate policyholder protection: policyholders are entitled to assume that after exhausting the SCR that the insurer still has sufficient assets to meet liabilities as they fall due. It also potentially creates an artificial incentive to retain longevity risk given that the market price is in excess of the best estimate.

Policyholder security could in theory be improved by instead increasing the SCR to the desired level, but here we think there is rightly a distinction in treatment between hedgeable risk, which can be eliminated and for which there is a clear market price, and non-hedgeable risk which does not share these characteristics.

The concept of a risk margin (or risk adjustment, or MOCE) is increasingly enshrined in international best practice, and IFRS 17 and ICS specifically. Eliminating the risk margin is also likely to be viewed adversely in any post-Brexit Equivalence discussions.

8.4 Conclusions

The working party’s analysis has not reached a definitive conclusion on recommending one method. Indeed, the different objectives are at times in conflict so there is no one solution that meets all of them. Furthermore, the context in which changes will be considered is highly dependent on unknown factors, such as the outcome of Brexit.

Nevertheless, having regard to our evaluations of the various alternatives, the working party sees merit in considering the following changes:

As indicated in section 8.1.1, the working party believes that a risk margin in some form is necessary. However, our view is that striking the balance between protecting policyholders and ensuring value for money is primarily a political choice, which affects the definition of both the risk margin and the SCR. This choice can be reflected in the calibration of any method that may be chosen.