No CrossRef data available.
Published online by Cambridge University Press: 25 November 2024
In this paper, we establish a new version of one-dimensional discrete improved Hardy’s inequality with shifts by introducing a shifting discrete Dirichlet’s Laplacian. We prove that the general discrete Hardy’s inequality as well as its variants in some special cases admit improvements. Further, it is proved that two-variable discrete  $p$-Hardy inequality can also be improved via improved discrete
$p$-Hardy inequality can also be improved via improved discrete 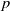 $p$-Hardy inequality in one dimension. The result is also extended to the multivariable cases.
$p$-Hardy inequality in one dimension. The result is also extended to the multivariable cases.