Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-05T13:38:57.376Z Has data issue: false hasContentIssue false
  • English
  • Français

On the interaction of Taylor length scale size droplets and isotropic turbulence

Published online by Cambridge University Press:  30 September 2016

Michael S. Dodd Open the ORCID record for Michael S. Dodd [Opens in a new window]  and
Antonino Ferrante
Michael S. Dodd
Affiliation:
William E. Boeing Department of Aeronautics and Astronautics, University of Washington, Seattle, WA 98195, USA
Antonino Ferrante*
Affiliation:
William E. Boeing Department of Aeronautics and Astronautics, University of Washington, Seattle, WA 98195, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Droplets in turbulent flows behave differently from solid particles, e.g. droplets deform, break up, coalesce and have internal fluid circulation. Our objective is to gain a fundamental understanding of the physical mechanisms of droplet–turbulence interaction. We performed direct numerical simulations (DNS) of 3130 finite-size, non-evaporating droplets of diameter approximately equal to the Taylor length scale and with 5 % droplet volume fraction in decaying isotropic turbulence at initial Taylor-scale Reynolds number $\mathit{Re}_{\unicode[STIX]{x1D706}}=83$. In the droplet-laden cases, we varied one of the following three parameters: the droplet Weber number based on the r.m.s. velocity of turbulence (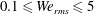$0.1\leqslant \mathit{We}_{rms}\leqslant 5$), the droplet- to carrier-fluid density ratio ($1\leqslant \unicode[STIX]{x1D70C}_{d}/\unicode[STIX]{x1D70C}_{c}\leqslant 100$) or the droplet- to carrier-fluid viscosity ratio (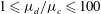$1\leqslant \unicode[STIX]{x1D707}_{d}/\unicode[STIX]{x1D707}_{c}\leqslant 100$). In this work, we derive the turbulence kinetic energy (TKE) equations for the two-fluid, carrier-fluid and droplet-fluid flow. These equations allow us to explain the pathways for TKE exchange between the carrier turbulent flow and the flow inside the droplet. We also explain the role of the interfacial surface energy in the two-fluid TKE equation through the power of the surface tension. Furthermore, we derive the relationship between the power of surface tension and the rate of change of total droplet surface area. This link allows us to explain how droplet deformation, breakup and coalescence play roles in the temporal evolution of TKE. Our DNS results show that increasing $\mathit{We}_{rms}$, 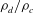$\unicode[STIX]{x1D70C}_{d}/\unicode[STIX]{x1D70C}_{c}$ and 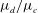$\unicode[STIX]{x1D707}_{d}/\unicode[STIX]{x1D707}_{c}$ increases the decay rate of the two-fluid TKE. The droplets enhance the dissipation rate of TKE by enhancing the local velocity gradients near the droplet interface. The power of the surface tension is a source or sink of the two-fluid TKE depending on the sign of the rate of change of the total droplet surface area. Thus, we show that, through the power of the surface tension, droplet coalescence is a source of TKE and breakup is a sink of TKE. For short times, the power of the surface tension is less than 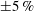$\pm 5\,\%$ of the dissipation rate. For later times, the power of the surface tension is always a source of TKE, and its magnitude can be up to 50 % of the dissipation rate.

JFM classification

Multiphase and Particle-laden Flows: Multiphase flow Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 806 , 10 November 2016 , pp. 356 - 412
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aris, R. 1989 Vectors, Tensors and the Basic Equations of Fluid Mechanics. Dover.Google Scholar
Aulisa, E., Manservisi, S., Scardovelli, R. & Zaleski, S. 2007 Interface reconstruction with least-squares fit and split advection in three-dimensional Cartesian geometry. J. Comput. Phys. 225 (2), 2301–2319.Google Scholar
Balachandar, S. & Eaton, J. K. 2010 Turbulent dispersed multiphase flow. Annu. Rev. Fluid Mech. 42, 111–133.Google Scholar
Baraldi, A., Dodd, M. S. & Ferrante, A. 2014 A mass-conserving volume-of-fluid method: volume tracking and droplet surface-tension in incompressible isotropic turbulence. Comput. Fluids 96, 322–337.Google Scholar
Berkman, P. D. & Calabrese, R. V. 1988 Dispersion of viscous liquids by turbulent flow in a static mixer. AIChE J. 34 (4), 602–609.CrossRefGoogle Scholar
Brackbill, J. U., Kothe, D. B. & Zemach, C. 1992 A continuum method for modeling surface tension. J. Comput. Phys. 100 (2), 335–354.CrossRefGoogle Scholar
Cummins, S. J., Francois, M. M. & Kothe, D. B. 2005 Estimating curvature from volume fractions. Comput. Struct. 83 (6–7), 425–434.Google Scholar
Dodd, M. S. & Ferrante, A. 2014 A fast pressure-correction method for incompressible two-fluid flows. J. Comput. Phys. 273, 416–434.Google Scholar
Dong, S. & Shen, J. 2012 A time-stepping scheme involving constant coefficient matrices for phase-field simulations of two-phase incompressible flows with large density ratios. J. Comput. Phys. 231 (17), 5788–5804.Google Scholar
Elghobashi, S. 1994 On predicting particle-laden turbulent flows. Appl. Sci. Res. 52, 309–329.Google Scholar
Faeth, G. M., Hsiang, L. P. & Wu, P. K. 1995 Structure and breakup properties of sprays. Intl J. Multiphase Flow 21, 99–127.Google Scholar
Ferrante, A. & Elghobashi, S. 2003 On the physical mechanism of two-way coupling in particle-laden isotropic turbulence. Phys. Fluids 15 (2), 315–329.Google Scholar
Frohn, A. & Roth, N. 2000 Dynamics of Droplets. Springer.Google Scholar
Hadamard, J. S. 1911 Mouvement permanent lent d’une sphère liquide et visqueuse dans un liquide visqueux. C. R. Acad. Sci. 152 (25), 1735–1738.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Joseph, D. D. 1976 Stability of Fluid Motions, vol. 2. Springer.Google Scholar
Joseph, D. D. & Renardy, Y. Y. 1993 Fundamentals of Two-Fluid Dynamics. Part I. Mathematical Theory and Applications. Springer.Google Scholar
Kwakkel, M., Breugem, W.-P. & Boersma, B. J. 2013 Extension of a CLSVOF method for droplet-laden flows with a coalescence/breakup model. J. Comput. Phys. 253, 166–188.Google Scholar
López, J., Zanzi, C., Gómez, P., Zamora, R., Faura, F. & Hernández, J. 2009 An improved height function technique for computing interface curvature from volume fractions. Comput. Meth. Appl. Mech. Engng 198 (33), 2555–2564.Google Scholar
Lucci, F., Ferrante, A. & Elghobashi, S. 2010 Modulation of isotropic turbulence by particles of Taylor length-scale size. J. Fluid Mech. 650, 1–51.Google Scholar
Lucci, F., Ferrante, A. & Elghobashi, S. 2011 Is Stokes number an appropriate indicator for turbulence modulation by particles of Taylor-length-scale size? Phys. Fluids 23, 1–7.CrossRefGoogle Scholar
Miller, G. H. & Colella, P. 2002 A conservative three-dimensional Eulerian method for coupled solid–fluid shock capturing. J. Comput. Phys. 183 (1), 26–82.Google Scholar
Perlekar, P., Biferale, L., Sbragaglia, M., Srivastava, S. & Toschi, F. 2012 Droplet size distribution in homogeneous isotropic turbulence. Phys. Fluids 24 (6), 065101.Google Scholar
Picano, F., Breugem, W.-P. & Brandt, L. 2015 Turbulent channel flow of dense suspensions of neutrally buoyant spheres. J. Fluid Mech. 764, 463–487.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Popinet, S. 2009 An accurate adaptive solver for surface-tension-driven interfacial flows. J. Comput. Phys. 228 (16), 5838–5866.CrossRefGoogle Scholar
Qin, C., Loth, E., Li, P., Simon, T. & Van de Ven, J. 2014 Spray-cooling concept for wind-based compressed air energy storage. J. Renew. Sustain. Energy 6 (4), 043125.CrossRefGoogle Scholar
Rybczyński, W. 1911 Über die fortschreitende Bewegung einer flüssigen Kugel in einen zähen Medium. Bull. Acad. Sci. Cracovie A, 40–46.Google Scholar
Scardovelli, R., Aulisa, E., Manservisi, S. & Marra, V. 2002 A marker-VOF algorithm for incompressible flows with interfaces. In Advances in Free Surface and Interface Fluid Dynamics, ASME Conference Proceedings, Joint U.S.-European Fluids Engineering Division Conference, vol. 1, pp. 905–910.Google Scholar
Schmidt, H., Schumann, U. & Volkert, H.1984 Three dimensional, direct and vectorized elliptic solvers for various boundary conditions. Rep. 84-15. DFVLR-Mitt.Google Scholar
Schumann, U. 1977 Realizability of Reynolds-stress turbulence models. Phys. Fluids 20 (5), 721–725.Google Scholar
Shaw, R. A. 2003 Particle–turbulence interactions in atmospheric clouds. Annu. Rev. Fluid Mech. 35 (1), 183–227.Google Scholar
Sirignano, W. A. 1983 Fuel droplet vaporization and spray combustion theory. Prog. Energy Combust. Sci. 9 (4), 291–322.CrossRefGoogle Scholar
Sirignano, W. A. 1999 Fluid Dynamics and Transport of Droplets and Sprays. Cambridge University Press.Google Scholar
Ten Cate, A., Derksen, J. J., Portela, L. M. & Van den Akker, H. E. A. 2004 Fully resolved simulations of colliding monodisperse spheres in forced isotropic turbulence. J. Fluid Mech. 519, 233–271.Google Scholar
Towns, J., Cockerill, T., Dahan, M., Foster, I., Gaither, K., Grimshaw, A., Hazlewood, V., Lathrop, S., Lifka, D., Peterson, G. D. et al. 2014 XSEDE: accelerating scientific discovery. Comput. Sci. Engng 16 (5), 62–74.CrossRefGoogle Scholar
Wilkins-Diehr, N., Sanielevici, S., Alameda, J., Cazes, J., Crosby, L., Pierce, M. & Roskies, R. 2016 An Overview of the XSEDE Extended Collaborative Support Program. pp. 3–13. Springer.Google Scholar
Yang, T. S. & Shy, S. S. 2005 Two-way interaction between solid particles and homogeneous air turbulence: particle settling rate and turbulence modification measurements. J. Fluid Mech. 526, 171–216.Google Scholar
Youngs, D. L. 1982 Time-dependent multi-material flow with large fluid distortion. Numer. Meth. Fluid Dyn. 1 (1), 41–51.Google Scholar
Zhang, Z. & Prosperetti, A. 2005 A second-order method for three-dimensional particle simulation. J. Comput. Phys. 210, 292–324.Google Scholar
Supplementary material: File

Download undefined(File)
File 4.4 MB

Dodd and Ferrante supplementary movie

Instantaneous contours of kl = 1/2 (ρujuj ) in the x–z plane (1 < t < 3.5) for single-phase flow (case A, left) and droplet-laden flow (case C, right).

Download Dodd and Ferrante supplementary movie(Video)
Video 3.6 MB

Dodd and Ferrante supplementary movie

Instantaneous contours of εl = Re−1(Tij Sij ) in the x–z plane (1 < t < 3.5) for single-phase flow (case A, left) and droplet-laden flow (case C, right).

Download Dodd and Ferrante supplementary movie(Video)
Video 3.7 MB

Dodd and Ferrante supplementary movie

Instantaneous contours of εl = Re−1(Tij Sij ) in the x–z plane (1 < t < 3.5) for density ratio of 1 (ϕ = 1, case E, left) and 100 (ϕ = 100, case F, right).

Download Dodd and Ferrante supplementary movie(Video)
Video 4.5 MB

Dodd and Ferrante supplementary movie

Instantaneous contours of εl = Re−1(Tij Sij ) in the x–z plane (1 < t < 3.5) for Weber number based on the r.m.s. velocity of turbulence of 0.1 (Werms = 0.1, case B, left) and 5 (Werms = 5, case D, right).

Download Dodd and Ferrante supplementary movie(Video)
Video 4.3 MB

Dodd and Ferrante supplementary movie

Instantaneous contours of T l = Re−1∂i(uj Tij ) in the x–z plane (1 < t < 3.5) for single-phase flow (case A, left) and droplet-laden flow (case C, right).

Download Dodd and Ferrante supplementary movie(Video)
Video 5.6 MB