No CrossRef data available.
Article contents
ON ARITHMETIC SUMS OF CONNECTED SETS IN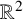 
$\mathbb {R}^2$
Published online by Cambridge University Press: 13 January 2023
Abstract
We prove that for two connected sets
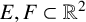$E,F\subset \mathbb {R}^2$
with cardinalities greater than
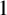$1$
, if one of E and F is compact and not a line segment, then the arithmetic sum
$E+F$
has nonempty interior. This improves a recent result of Banakh et al. [‘The continuity of additive and convex functions which are upper bounded on non-flat continua in
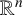$\mathbb {R}^n$
’, Topol. Methods Nonlinear Anal. 54(1)(2019), 247–256] in dimension two by relaxing their assumption that E and F are both compact.
Keywords
MSC classification
Primary:
54F15: Continua and generalizations
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
References
Ahlfors, L. V., Complex Analysis. An Introduction to the Theory of Analytic Functions of One Complex Variable, 3rd edn (McGraw-Hill, New York, 1978).Google Scholar
Astels, S., ‘Cantor sets and numbers with restricted partial quotients’, Trans. Amer. Math. Soc. 352(1) (2000), 133–170.CrossRefGoogle Scholar
Banakh, T., Jabłońska, E. and Jabłoński, W., ‘The continuity of additive and convex functions which are upper bounded on non-flat continua in
${\mathbb{R}}^n$
’, Topol. Methods Nonlinear Anal. 54(1) (2019), 247–256.Google Scholar
${\mathbb{R}}^n$
’, Topol. Methods Nonlinear Anal. 54(1) (2019), 247–256.Google ScholarBorwein, J. M. and Lewis, A. S., Convex Analysis and Nonlinear Optimization. Theory and Examples, 2nd edn (Springer, New York, 2006).Google Scholar
Chang, A., ‘On the Minkowski sum of two curves’, Real Anal. Exchange 43(1) (2018), 221–222.CrossRefGoogle Scholar
Feng, D.-J. and Wu, Y.-F., ‘On arithmetic sums of fractal sets in
${\mathbb{R}}^d$
’, J. Lond. Math. Soc. (2) 104(1) (2021), 35–65.CrossRefGoogle Scholar
${\mathbb{R}}^d$
’, J. Lond. Math. Soc. (2) 104(1) (2021), 35–65.CrossRefGoogle ScholarHall, M. J., ‘On the sum and product of continued fractions’, Ann. of Math. (2) 48(2) (1947), 966–993.CrossRefGoogle Scholar
Jones, F. B., ‘Connected and disconnected plane sets and the functional equation
$f\left(x+y\right)=f(x)+f(y)$
’, Bull. Amer. Math. Soc. (N.S.) 48(2) (1942), 115–120.CrossRefGoogle Scholar
$f\left(x+y\right)=f(x)+f(y)$
’, Bull. Amer. Math. Soc. (N.S.) 48(2) (1942), 115–120.CrossRefGoogle ScholarNewhouse, S. E., ‘The abundance of wild hyperbolic sets and nonsmooth stable sets for diffeomorphisms’, Publ. Math. Inst. Hautes Études Sci. 50 (1979), 101–151.CrossRefGoogle Scholar
Oxtoby, C. J., Measure and Category: A Survey of the Analogies between Topological and Measure Spaces, 2nd edn (Springer, New York–Berlin, 1980).CrossRefGoogle Scholar
Rockafellar, R. T., Convex Analysis, Princeton Mathematical Series, 28 (Princeton University Press, Princeton, NJ, 1970).CrossRefGoogle Scholar
Rosen, H., ‘An earlier fractal graph’, Real Anal. Exchange 43(2) (2018), 451–454.CrossRefGoogle Scholar
Simon, K. and Taylor, K., ‘Interior of sums of planar sets and curves’, Math. Proc. Cambridge Philos. Soc. 168(1) (2020), 119–148.CrossRefGoogle Scholar
Simon, K. and Taylor, K., ‘Dimension and measure of sums of planar sets and curves’, Mathematika 68 (2022), 1364–1392.CrossRefGoogle Scholar
Steinhaus, H., ‘A new property of G. Cantor’s set’, Wektor 6 (1917), 105–107 (in Polish).Google Scholar