Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-05T14:09:18.024Z Has data issue: false hasContentIssue false
  • English
  • Français

On the competition between the stress-induced formation of martensite and dislocation plasticity during crack propagation in pseudoelastic NiTi shape memory alloys

Published online by Cambridge University Press:  17 July 2017

Tamas Ungár ,
Jan Frenzel ,
Susanne Gollerthan ,
Gábor Ribárik ,
Levente Balogh  and
Gunther Eggeler
Tamas Ungár*
Affiliation:
Department of Materials Physics, Eötvös University Budapest, Budapest H-1518, Hungary; and School of Materials, The University of Manchester, Manchester M13 9PL, U.K.
Jan Frenzel
Affiliation:
Institut für Werkstoffe, Ruhr-Universität Bochum, 44801 Bochum, Germany
Susanne Gollerthan
Affiliation:
Institut für Werkstoffe, Ruhr-Universität Bochum, 44801 Bochum, Germany
Gábor Ribárik
Affiliation:
Department of Materials Physics, Eötvös University Budapest, Budapest H-1518, Hungary
Levente Balogh
Affiliation:
Department of Materials Physics, Eötvös University Budapest, Budapest H-1518, Hungary; and Department of Mechanical and Materials Engineering, Queen’s University, Kingston, Ontario K7L 3N6, Canada
Gunther Eggeler
Affiliation:
Institut für Werkstoffe, Ruhr-Universität Bochum, 44801 Bochum, Germany
*
a)Address all correspondence to this author. e-mail: [email protected]
Get access

Abstract

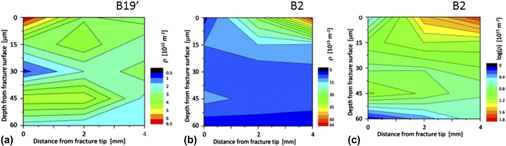
The present work addresses the competition between dislocation plasticity and stress-induced martensitic transformations in crack affected regions of a pseudoelastic NiTi miniature compact tension specimen. For this purpose X-ray line profile analysis was performed after fracture to identify dislocation densities and remnant martensite volume fractions in regions along the crack path. Special emphasis was placed on characterizing sub fracture surface zones to obtain depth profiles. The stress affected zone in front of the crack-tip is interpreted in terms of a true plastic zone associated with dislocation plasticity and a pseudoelastic zone where stress-induced martensite can form. On unloading, most of the stress-induced martensite transforms back to austenite but a fraction of it is stabilized by dislocations in both, the irreversible martensite and the surrounding austenite phase. The largest volume fraction of the irreversible or remnant martensite along with the highest density of dislocations in this phase was found close to the primary crack-tip. With increasing distance from the primary crack-tip both, the dislocation density and the volume fraction of irreversible martensite decrease to lower values.

Keywords

shape memory alloys (SMAs)fracture mechanicsX-ray line profile analysisdislocation plasticityremnant martensite
Type
Articles
Information
Journal of Materials Research , Volume 32 , Issue 23: Focus Issue: Mechanical Properties and Microstructure of Advanced Metallic Alloys—in Honor of Prof. Haël Mughrabi PART A , 14 December 2017 , pp. 4433 - 4442
Check for updates
Copyright
Copyright © Materials Research Society 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Contributing Editor: Mathias Göken

Dedicated to the 80th Birthday of Professor Haël Mughrabi.

b)

This author was an editor of this journal during the review and decision stage. For the JMR policy on review and publication of manuscripts authored by editors, please refer to http://www.mrs.org/editor-manuscripts/.

A previous error in this article has been corrected. For details, see 10.1557/jmr.2017.459

References

REFERENCES

Otsuka, K. and Waymann, C.M.: Shape Memory Materials (Cambridge University Press, Cambridge, 1998).Google Scholar
Funakubo, H.: Shape Memory Alloys (Gordon and Breach, New York, 1987).Google Scholar
Frenzel, J., George, E.P., Dlouhy, A., Somsen, C., Wagner, M.F.X., and Eggeler, G.: Influence of Ni on martensitic phase transformations in NiTi shape memory alloys. Acta Mater. 58, 3444 (2010).Google Scholar
Frenzel, J., Wieczorek, A., Opahle, I., Maaß, B., Drautz, R., and Eggeler, G.: On the effect of alloy composition on martensite start temperatures and latent heats in Ni–Ti-based shape memory alloys. Acta Mater. 90, 213 (2015).Google Scholar
Van Humbeeck, J.: Non-medical applications of shape memory alloys. Mater. Sci. Eng., A 273–275, 134 (1999).CrossRefGoogle Scholar
Duerig, T., Pelton, A., and Stöckel, D.: An overview of nitinol medical applications. Mater. Sci. Eng., A 273–275, 149 (1999).Google Scholar
Pushin, V.G., Stolyarov, V.V., Valiev, R.Z., Lowe, T.C., and Zhu, Y.T.: Nanostructured TiNi-based shape memory alloys processed by severe plastic deformation. Mater. Sci. Eng., A 410, 386 (2005).CrossRefGoogle Scholar
Valiev, R., Gunderov, D., Prokofiev, E., Pushin, V., and Zhu, Y.: Nanostructuring of TiNi alloy by SPD processing for advanced properties. Mater. Trans. 49, 97 (2008).Google Scholar
Grossmann, C., Frenzel, J., Sampath, V., Depka, T., Oppenkowski, A., Somsen, C., Neuking, K., Theisen, W., and Eggeler, G.: Processing and property assessment of NiTi and NiTiCu shape memory actuator springs. Materialwiss. Werkstofftech. 39, 499 (2008).Google Scholar
Robertson, S.W., Pelton, A.R., and Ritchie, R.O.: Mechanical fatigue and fracture of Nitinol. Int. Mater. Rev. 57, 1 (2012).Google Scholar
Rahim, M., Frenzel, J., Frotscher, M., Pfetzing-Micklich, J., Steegmüller, R., Wohlschlögel, M., Mughrabi, H., and Eggeler, G.: Impurity levels and fatigue lives of pseudoelastic NiTi shape memory alloys. Acta Mater. 61, 3667 (2013).Google Scholar
Launey, M., Robertson, S.W., Scott, W., Vien, L., Senthilnathan, K., Karthikeyan, Ch., Chintapalli, P., and Pelton, A.R.: Influence of microstructural purity on the bending fatigue behavior of VAR-melted superelastic Nitinol. J. Mech. Behav. Biomed. Mater. 34, 181 (2014).Google Scholar
Fähler, S., Rößler, U.K., Kastner, O., Eckert, J., Eggeler, G., Emmerich, H., Entel, P., Müller, S., Quandt, E., and Albe, K.: Caloric effects in ferroic materials: New concepts for cooling. Adv. Eng. Mater. 14, 10 (2012).Google Scholar
Baxevanis, T. and Lagoudas, D.C.: Fracture mechanics of shape memory alloys: Review and perspectives. Int. J. Fract. 191, 191 (2015).CrossRefGoogle Scholar
Gollerthan, S., Herberg, D., Baruj, A., and Eggeler, G.: Compact tension testing of martensitic/pseudoplastic NiTi shape memory alloys. Mater. Sci. Eng., A 481, 156 (2008).Google Scholar
Gollerthan, S., Young, M.L., Baruj, A., Frenzel, J., Schmahl, W.W., and Eggeler, G.: Fracture mechanics and microstructure in NiTi shape memory alloys. Acta Mater. 57, 2015 (2009).Google Scholar
Gollerthan, S., Young, M.L., Neuking, K., Ramamurty, U., and Eggeler, G.: Direct physical evidence for the back-transformation of stress-induced martensite in the vicinity of cracks in pseudoelastic NiTi shape memory alloys. Acta Mater. 57, 5892 (2009).Google Scholar
Maletta, C., Bruno, L., Corigliano, P., Crupi, V., and Guglielmino, E.: Crack-tip thermal and mechanical hysteresis in shape memory alloys under fatigue loading. Mater. Sci. Eng., A 616, 281 (2014).Google Scholar
Young, M.L., Gollerthan, S., Baruj, A., Frenzel, J., Schmahl, W.W., and Eggeler, G.: Strain mapping of crack extension in pseudoelastic NiTi shape memory alloys during static loading. Acta Mater. 61, 5800 (2013).Google Scholar
Sgambitterra, E., Maletta, C., and Furgiuele, F.: Investigation on crack tip transformation in NiTi alloys: Effect of the temperature. Shape Mem. Superelastic 1, 275 (2015).Google Scholar
Baxevanis, T., Chemisky, Y., and Lagoudas, D.C.: Finite element analysis of the plane strain crack-tip mechanical fields in pseudoelastic shape memory alloys. Smart Mater. Struct. 21, 094012 (2012).CrossRefGoogle Scholar
Baxevanis, T., Parrinello, A.F., and Lagoudas, D.C.: On the fracture toughness enhancement due to stress-induced phase transformation in shape memory alloys. Int. J. Plast. 50, 158 (2013).Google Scholar
Baxevanis, T., Landis, C.M., and Lagoudas, D.C.: On the fracture toughness of pseudoelastic shape memory alloys. J. Appl. Mech. 81, 041005 (2014).CrossRefGoogle Scholar
Baxevanis, T., Landis, C.M., and Lagoudas, D.C.: On the effect of latent heat on the fracture toughness of pseudoelastic shape memory alloys. J. Appl. Mech. 81, 101006 (2014).CrossRefGoogle Scholar
Stam, G. and Giessen, E.: Effect of reversible phase transformations on crack growth. Mech. Mater. 21, 51 (1995).Google Scholar
Freed, Y. and Banks-Sills, L.: Crack growth resistance of shape memory alloys by means of a cohesive zone model. J. Mech. Phys. Solids 55, 2157 (2007).CrossRefGoogle Scholar
Ungár, T., Ott, S., Sanders, P.G., Borbély, A., and Weertman, J.R.: Dislocations, grain size and planar faults in nanostructured copper determined by high resolution X-ray diffraction and a new procedure of peak profile analysis. Acta Mater. 46, 3693 (1998).Google Scholar
Ungár, T., Dragomir, I., Révész, Á., and Borbély, A.: The contrast factors of dislocations in cubic crystals: The dislocation model of strain anisotropy in practice. J. Appl. Crystallogr. 34, 298 (1999).Google Scholar
Ribárik, G. and Ungár, T.: Characterization of the microstructure in random and textured polycrystals and single crystals by diffraction line profile analysis. Mater. Sci. Eng., A 528, 112 (2010).CrossRefGoogle Scholar
Ungár, T., Balogh, L., and Ribárik, G.: Defect-related physical-profile-based X-ray and neutron line profile analysis. Metall. Mater. Trans. A 41, 1202 (2010).Google Scholar
Ribárik, G., Jóni, B., and Ungár, T.: Monte-Carlo and least-squares procedures combined for global minimum of the physical parameters in line profile analysis. In preparation.Google Scholar
Krivoglaz, M.A.: X-ray and Neutron Diffraction in Nonideal Crystals (Springer, Berlin, Heidelberg, 1996).CrossRefGoogle Scholar
Wilkens, M.: Theoretical aspects of kinematical X-ray diffraction profiles from crystals containing dislocation distributions. In Fundamental Aspects of Dislocation Theory, Vol. II, Simmons, J.A., de Wit, R., and Bullough, R., eds.; (Nat. Bur. Stand. (US), Spec. Publ. No. 317, Washington, DC, USA, 1970); p. 1195.Google Scholar
Groma, I., Ungár, T., and Wilkens, M.: Asymmetric X-ray line broadening of plastically deformed crystals. I. Theory. J. Appl. Crystallogr. 21, 47 (1988).CrossRefGoogle Scholar
Bailey, J.E. and Hirsch, P.B.: The dislocation distribution, flow stress, and stored energy in cold-worked polycrystalline silver. Philos. Mag. 5, 485 (1960).CrossRefGoogle Scholar
Ungár, T. and Tichy, G.: The effect of dislocation contrast on X-ray line profiles in untextured polycrystals. Phys. Status Solidi 147, 425 (1999).Google Scholar
Csiszár, G., Pantleon, K., Alimadadi, H., Ribárik, G., and Ungár, T.: Dislocation density and Burgers vector population in fiber-textured Ni thin films determined by high-resolution X-ray line profile analysis. J. Appl. Crystallogr. 45, 61 (2012).Google Scholar
Jóni, B., Al-Samman, T., Chowdhury, S.G., Csiszár, G., and Ungár, T.: Dislocation densities and prevailing slip-system types determined by X-ray line profile analysis in a textured AZ31 magnesium alloy deformed at different temperatures. J. Appl. Crystallogr. 46, 55 (2013).Google Scholar
Ungár, T., Tichy, G., Gubicza, J., and Hellmig, R.J.: Correlation between subgrains and coherently scattering domains. Powder Diffr. 20, 366 (2005).Google Scholar
Zilahi, Gy., Ungár, T., and Tichy, G.: A common theory of line broadening and rocking curves. J. Appl. Crystallogr. 48, 418 (2015).Google Scholar
Nemat-Nasser, S., Choi, J-Y., Guo, W-G., and Isaacs, J.B.: Very high strain-rate response of a NiTi shape-memory alloy. Mech. Mater. 37, 287 (2005).Google Scholar
Nemat-Nasser, S. and Guo, W-G.: Superelastic and cyclic response of NiTi SMA at various strain rates and temperatures. Mech. Mater. 38, 463 (2006).Google Scholar
Guo, W.G., Su, J., Su, Y., and Chu, S.Y.: On phase transition velocities of NiTi shape memory alloys. J. Alloys Compd. 501, 70 (2010).CrossRefGoogle Scholar
Freund, L.B. and Hutchinson, J.W.: High strain-rate crack growth in rate-dependent plastic solids. J. Mech. Phys. Solids 33, 169 (1985).Google Scholar
Freund, L.B., Hutchinson, J.W., and Lam, P.S.: Analysis of high-strain-rate elastic-plastic crack growth. Eng. Fract. Mech. 23, 119 (1986).Google Scholar
Grey, G.T. III: High-strain-rate deformation: Mechanical behavior and deformation substructures induced. Annu. Rev. Mater. Res. 42, 285 (2012).Google Scholar