No CrossRef data available.
Article contents
Order-automorphism groups of Archimedean ordered groups
Part of:
Structure and classification of infinite or finite groups
Abelian groups
Ordered structures
Special aspects of infinite or finite groups
Published online by Cambridge University Press: 23 December 2024
Abstract
The group of order-preserving automorphisms of a finitely generated Archimedean ordered group of rank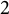 
$2$ is either infinite cyclic or trivial according as the ratio in 
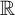$\mathbb {R}$ of the generators of the subgroup is or is not quadratic over 
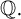$\mathbb {Q}.$ In the case of an Archimedean ordered group of rank 
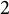$2$ that is not finitely generated, the group of order-preserving automorphisms is free abelian. Criteria determining the rank of this free abelian group are established.
- Type
- Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press on behalf of Canadian Mathematical Society
References
Baker, A., A concise introduction to the theory of numbers, Cambridge University Press, Cambridge, 1984.CrossRefGoogle Scholar
Botto Mura, R. and Rhemtulla, A., Orderable groups, Lecture Notes in Pure and Applied Mathematics, 27, Marcel Dekker, Inc., New York, 1977.Google Scholar
Bourbaki, N., Commutative algebra (English translation), Elements of Mathematics, Hermann, Paris; Addison-Wesley, Reading, MA, 1972.Google Scholar
Cartan, H.,
Un théorème sur les groupes ordonnés (French). Bull. Sci. Math. 63(1939), 201–205.Google Scholar
Čarin, V. S.,
On groups of automorphisms of nilpotent groups (Russian). Ukrain. Mat. Ž. 6(1954), 295–304.Google Scholar
Conrad, P.,
Extensions of ordered groups
. Proc. Amer. Math. Soc. 6(1955), no. 4, 516–528.CrossRefGoogle Scholar
Conrad, P.,
The group of order preserving automorphisms of an ordered abelian group
. Proc. Amer. Math. Soc. 9(1958), no. 3, 382–389.CrossRefGoogle Scholar
Conrad, P.,
A correction and improvement of a theorem on ordered groups
. Proc. Amer. Math. Soc. 10(1959), no. 2, 182–184.CrossRefGoogle Scholar
Fuchs, L., Infinite Abelian groups, vol. 1, Pure and Applied Mathematics, 36, Academic Press, New York, 1970.Google Scholar
Glass, A. M. W. and Ribenboim, P.,
Automorphisms of the ordered multiplicative group of positive rational numbers
. Proc. Amer. Math. Soc. 122(1994), no. 1, 15–18.CrossRefGoogle Scholar
Hion, Y. V.,
Archimedean ordered rings (Russian). Uspehi Mat. Nauk (N.S.) 9(1954), no. 4(62), 237–242.Google Scholar
Hölder, O.,
Die Axiome der Quantität und die Lehre vom Mass
. Ber. Verk. Sächs. Wiss. Leipzig, Math. Phys. Cl. 53(1901), 1–64.Google Scholar
Lafuente-Rodríguez, R. H.,
o-Groups of finite rank and divisibility in their groups of o-automorphisms
. Algebra Universalis 79(2018), no. 4, 81, 16 pp.CrossRefGoogle Scholar
Williams, K. S. and Buck, N., Comparison of the lengths of the continued fractions of
$\sqrt{D}$
and
$\frac{1}{2}\left(1+\sqrt{D}\;\right)$
. Proc. Amer. Math. Soc. 120(1994), no. 4, 995–1002.Google Scholar
$\sqrt{D}$
and
$\frac{1}{2}\left(1+\sqrt{D}\;\right)$
. Proc. Amer. Math. Soc. 120(1994), no. 4, 995–1002.Google ScholarZariski, O. and Samuel, P., Commutative algebra, vol. 2, Graduate Texts in Mathematics, 29, Springer-Verlag, New York, 1960.CrossRefGoogle Scholar