1. Introduction
The interior of the oceans may be considered as a vast field of internal gravity waves. Continuous stratification, gravitational forcing due to the lunar orbit (Rattray Reference Rattray1960) and suitable bathymetry conspire to produce a complex interior system of mechanical wave transmission. Amplitudes of these waves may be hundreds of metres (Susanto, Mitnik & Zheng Reference Susanto, Mitnik and Zheng2005), but they are known to propagate at shallow angles and in beam-like geometric patterns. In general, waveforms are modified by boundary topography (van Haren, Maas & van Aken Reference van Haren, Maas and van Aken2002), and in particular, their spectral form is crucial to predicting their interaction. There are several well known features of internal wave mechanics that arise due to nonlinearity in the underlying physics, and primarily these arise from the quadratic structure of the advection operator. Viewed in spectral space, the advection operator may be cast as a geometric relationship between wavevectors and frequencies known as triadic interaction (Phillips Reference Phillips1960; Thorpe Reference Thorpe1966). Special cases include the interaction of two crossing wave beams (McComas & Bretherton Reference McComas and Bretherton1977; Sun & Kunze Reference Sun and Kunze1999a,Reference Sun and Kunzeb; Javam, Imberger & Armfield Reference Javam, Imberger and Armfield2000; Tabaei, Akylas & Lamb Reference Tabaei, Akylas and Lamb2005; Smith & Crockett Reference Smith and Crockett2014), triadic resonant instability (Davis & Acrivos Reference Davis and Acrivos1967; Martin, Simmons & Wunsch Reference Martin, Simmons and Wunsch1969; McEwan Reference McEwan1971; Bourget et al. Reference Bourget, Dauxois, Joubaud and Odier2013) and a limiting case known as parametric subharmonic instability (McEwan & Robinson Reference McEwan and Robinson1975; Benielli & Sommeria Reference Benielli and Sommeria1998; Koudella & Staquet Reference Koudella and Staquet2006; Karimi & Akylas Reference Karimi and Akylas2014). We will discuss in depth interactions of crossing wave beams as part of this paper, but we refer the reader to Dauxois et al. (Reference Dauxois, Joubaud, Odier and Venaille2018) for a review of instabilities and Müller et al. (Reference Müller, Holloway, Henyey and Pomphrey1986) for a broader overview.
Experiments have played an important role in refining our understanding of internal wave systems ranging from early studies of oscillating cylinders (Görtler Reference Görtler1943; Mowbray & Rarity Reference Mowbray and Rarity1967) to complex mechanical devices for generating quasi-planar waves (McEwan Reference McEwan1971; Gostiaux et al. Reference Gostiaux, Didelle, Mercier and Dauxois2007). There are broadly three approaches to analysing wave systems: characteristics, Green's functions and Fourier methods. The oscillating cylinder is the natural analogue of characteristic (Hurley Reference Hurley1972) and Green's function approaches (Hurley Reference Hurley1969; Voisin Reference Voisin1991), because spatially localised beams emerge in a St. Andrew's cross pattern and these are aligned with the characteristics. On the other hand, Fourier methods more naturally correspond to quasi-planar systems (Mercier et al. Reference Mercier, Martinand, Mathur, Gostiaux, Peacock and Dauxois2010), where there is implicit spatial periodicity as well as temporal periodicity.
In this paper, we shall build a more general framework based on Green's functions and seek to validate using laboratory experiments, firstly on a polychromatic aperiodic example case of lee waves, and then develop to a case where steady, periodic wave beams show significant nonlinear interaction. The experiments utilise the unique capabilities of the ‘magic carpet’ (Dobra, Lawrie & Dalziel Reference Dobra, Lawrie and Dalziel2019) to generate a full spectrum of wave beams, synthetic Schlieren (Dalziel, Hughes & Sutherland Reference Dalziel, Hughes and Sutherland1998; Sutherland et al. Reference Sutherland, Dalziel, Hughes and Linden1999; Dalziel, Hughes & Sutherland Reference Dalziel, Hughes and Sutherland2000; Dalziel et al. Reference Dalziel, Carr, Sveen and Davies2007) to diagnose the resulting wave field from density gradients and dynamic mode decomposition (Schmid Reference Schmid2010) to dissect the modal structure. Using these tools, figure 1 illustrates a typical wave–wave interaction with two incident beams in figures 1(a) and 1(b) with direction of propagation shown by the arrows. Figure 1(c) shows a snapshot of the experimentally observed field, and figure 1(d–f) shows ‘daughter’ modes that are observed to emerge nonlinearly from the interaction and have directions of propagation as shown.

Figure 1. Schematic showing a decomposition of a wave–wave interaction between two incident internal wave beams (a,b). Panel (c) shows the full experimentally observed wave field. Panels (d–f) show nonlinearly generated ‘daughter’ modes that are identifiable from the experiment. The arrows indicate the direction of propagation of each wave beam.
To address the question of nonlinear wave–wave interactions, our new framework will allow for weakly nonlinear interactions between a hierarchy of Green's functions. We utilise Green's functions to represent the driving waves and derive the weakly nonlinear transfer terms that pass energy into other frequency and wavenumber components, these also being represented in terms of Green's functions. Our framework is sufficiently broad to deal not only with interactions of the form shown in figure 1 that lead to resonance (disturbances that satisfy both the geometric conditions on wavenumber and frequency and also satisfy the relevant dispersion relation) but also those where the linear dispersion relation is not satisfied.
The structure of this article is as follows. We present the background material to the governing equations in § 2 and discuss the tractability of other analytical options. Focussing on the monochromatic Green's function solution to the linear equation in § 3, we prepare the building blocks of a hierarchical numerical approach. In § 4, we demonstrate application of this approach to inviscid, aperiodic systems, and carefully validate against experiments using our ‘magic carpet’ (Dobra et al. Reference Dobra, Lawrie and Dalziel2019). We then generalise in § 5 our numerical Green's function approach so that we may capture the physics of nonlinearly interacting internal waves. We employ the perturbation expansion technique of Tabaei et al. (Reference Tabaei, Akylas and Lamb2005) and developed further in Dobra, Lawrie & Dalziel (Reference Dobra, Lawrie and Dalziel2021) to account for successive layers of wave–wave interactions and demonstrate that the resultant field compares well with experimental observations. Finally, in § 6, we draw our conclusions.
2. Internal wave equation
We begin by considering two-dimensional, inviscid, linear internal waves in a quiescent, Boussinesq density stratification, ![]() $\rho _0 \left ( z \right )$. These restrictions closely approximate the conditions in our laboratory experiments, where it is particularly advantageous to consider flows with limited variation in the third dimension for ease of diagnosis. We define
$\rho _0 \left ( z \right )$. These restrictions closely approximate the conditions in our laboratory experiments, where it is particularly advantageous to consider flows with limited variation in the third dimension for ease of diagnosis. We define ![]() $\boldsymbol {x} = \left ( x , z \right )$ as the horizontal and vertical coordinates with corresponding unit basis vectors
$\boldsymbol {x} = \left ( x , z \right )$ as the horizontal and vertical coordinates with corresponding unit basis vectors ![]() $\left \{ \boldsymbol {e}_x , \boldsymbol {e}_z \right \}$, and we assume there is no diffusion of mass or heat. Let
$\left \{ \boldsymbol {e}_x , \boldsymbol {e}_z \right \}$, and we assume there is no diffusion of mass or heat. Let ![]() $t$ be time,
$t$ be time, ![]() $\boldsymbol {u} = \left ( u , w \right )$ the velocity field,
$\boldsymbol {u} = \left ( u , w \right )$ the velocity field, ![]() $p'$ the perturbation from hydrostatic pressure,
$p'$ the perturbation from hydrostatic pressure, ![]() $\rho _{00}$ be the Boussinesq reference density,
$\rho _{00}$ be the Boussinesq reference density, ![]() $\rho '$ (with
$\rho '$ (with ![]() $\left | \rho ' \right | \ll \rho _{00}$) the perturbation from
$\left | \rho ' \right | \ll \rho _{00}$) the perturbation from ![]() $\rho _0 \left ( z \right )$ and
$\rho _0 \left ( z \right )$ and ![]() $g$ gravitational acceleration. Then, the three nonlinear governing equations are the conservation of momentum (Euler equation),
$g$ gravitational acceleration. Then, the three nonlinear governing equations are the conservation of momentum (Euler equation),
the conservation of volume (equivalent to incompressibility in the case of a homogeneous fluid),
and consequently the conservation of mass may be written as
In the linear wave approximation, the two nonlinear terms arising from the advection operator ![]() $\boldsymbol {u} \boldsymbol {\cdot } \boldsymbol {\nabla }$ are considered to be negligible. The remaining derivative operators can be isolated into a complex matrix
$\boldsymbol {u} \boldsymbol {\cdot } \boldsymbol {\nabla }$ are considered to be negligible. The remaining derivative operators can be isolated into a complex matrix ![]() ${\boldsymbol{\mathsf{P}}}$ that acts on a state vector
${\boldsymbol{\mathsf{P}}}$ that acts on a state vector ![]() $\boldsymbol {\phi }$, say, and the system arranged into homogeneous form. Taking a single Fourier mode of
$\boldsymbol {\phi }$, say, and the system arranged into homogeneous form. Taking a single Fourier mode of ![]() $\boldsymbol {\phi }$ with wavevector
$\boldsymbol {\phi }$ with wavevector ![]() $\boldsymbol {k} = \left ( k , m \right )$ and frequency
$\boldsymbol {k} = \left ( k , m \right )$ and frequency ![]() $\omega$, we can write
$\omega$, we can write
The derivative operator, ![]() ${\boldsymbol{\mathsf{P}}}$, then takes the complex algebraic form
${\boldsymbol{\mathsf{P}}}$, then takes the complex algebraic form ![]() $\hat {{\boldsymbol{\mathsf{P}}}}$. For a homogeneous system, non-trivial symmetries are found when the determinant
$\hat {{\boldsymbol{\mathsf{P}}}}$. For a homogeneous system, non-trivial symmetries are found when the determinant ![]() $| \hat {{\boldsymbol{\mathsf{P}}}} | = 0$, and these correspond to resonant wave behaviours. From
$| \hat {{\boldsymbol{\mathsf{P}}}} | = 0$, and these correspond to resonant wave behaviours. From
arises a natural frequency, the buoyancy (Brunt–Väisälä) frequency,
 \begin{equation} N = \sqrt{- \frac{g}{\rho_{00}} \frac{\mathrm{d} \rho_0}{\mathrm{d} z}}, \end{equation}
\begin{equation} N = \sqrt{- \frac{g}{\rho_{00}} \frac{\mathrm{d} \rho_0}{\mathrm{d} z}}, \end{equation}
and by examining the geometry of ![]() $k / \left | \boldsymbol {k} \right |$, the dispersion relation,
$k / \left | \boldsymbol {k} \right |$, the dispersion relation,
is obtained, where ![]() $\varTheta$ is the angle between wavevector
$\varTheta$ is the angle between wavevector ![]() $\boldsymbol {k}$ and the horizontal. Since this system is linear, any perturbation quantity
$\boldsymbol {k}$ and the horizontal. Since this system is linear, any perturbation quantity ![]() $\chi$ satisfies the dispersion relation provided that
$\chi$ satisfies the dispersion relation provided that
Taking the inverse Fourier transform yields the linear internal wave equation,
where we define ![]() $\mathcal {L}$ to be the corresponding operator. From any choice of
$\mathcal {L}$ to be the corresponding operator. From any choice of ![]() $\chi$, the polarisation of any other quantity can be derived by appropriate substitution into the linearised equations. In particular, any such quantity will also satisfy the linear internal wave equation.
$\chi$, the polarisation of any other quantity can be derived by appropriate substitution into the linearised equations. In particular, any such quantity will also satisfy the linear internal wave equation.
Source terms may be configured to be equivalent to the action of boundaries, and we will see in § 5 that they can also inductively account for discrepancies between a linear wave approximation and the corresponding nonlinear field. Thus, we consider solution approaches to the inhomogeneous internal wave equation, ![]() $\mathcal {L} \chi = f$, with source distribution
$\mathcal {L} \chi = f$, with source distribution ![]() $f \left ( \boldsymbol {x}, t \right )$.
$f \left ( \boldsymbol {x}, t \right )$.
While we could choose to work with any variable ![]() $\chi$, it is important to select a representation of the system that has a clear physical interpretation. In view of this, two interesting choices of
$\chi$, it is important to select a representation of the system that has a clear physical interpretation. In view of this, two interesting choices of ![]() $\chi$ are an internal potential,
$\chi$ are an internal potential, ![]() $\xi$, as used by Voisin (Reference Voisin1994) and Scase & Dalziel (Reference Scase and Dalziel2004), and the streamfunction,
$\xi$, as used by Voisin (Reference Voisin1994) and Scase & Dalziel (Reference Scase and Dalziel2004), and the streamfunction, ![]() $\psi$. We now consider the physical interpretation of point source terms for each of these potentials in turn.
$\psi$. We now consider the physical interpretation of point source terms for each of these potentials in turn.
The internal potential is defined by
and is chosen such that ![]() $\boldsymbol {\nabla } \boldsymbol {\cdot } \boldsymbol {u} = \mathcal {L} \xi$. We consider an instantaneous point source of unit strength at
$\boldsymbol {\nabla } \boldsymbol {\cdot } \boldsymbol {u} = \mathcal {L} \xi$. We consider an instantaneous point source of unit strength at ![]() $\boldsymbol {x}_0$ that is active at time
$\boldsymbol {x}_0$ that is active at time ![]() $t_0$, expressed in terms of Dirac-
$t_0$, expressed in terms of Dirac-![]() $\delta$ functions as
$\delta$ functions as ![]() $f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \delta \left ( t - t_0 \right )$. Integrating along a short time interval including
$f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \delta \left ( t - t_0 \right )$. Integrating along a short time interval including ![]() $t_0$ over some fixed volume
$t_0$ over some fixed volume ![]() $V$ around
$V$ around ![]() $\boldsymbol {x}_0$ with boundary
$\boldsymbol {x}_0$ with boundary ![]() $\partial V$ and using
$\partial V$ and using ![]() $\mathcal {L} \xi = \boldsymbol {\nabla }\boldsymbol {\cdot } \boldsymbol {u}$ in conjunction with the divergence theorem gives
$\mathcal {L} \xi = \boldsymbol {\nabla }\boldsymbol {\cdot } \boldsymbol {u}$ in conjunction with the divergence theorem gives
which is the total volume of fluid ejected through enclosing surface, ![]() $S$. Therefore, the point source of unit strength injects one unit of fluid volume.
$S$. Therefore, the point source of unit strength injects one unit of fluid volume.
The other interesting choice, the streamfunction, ![]() $\psi$, is an integral of the velocity field according to
$\psi$, is an integral of the velocity field according to
It follows immediately that the vorticity ![]() $\boldsymbol {\nabla } \times \boldsymbol {u} = - \nabla ^{2} \psi$, and it appears in the first term of the internal wave equation (2.9) if we set
$\boldsymbol {\nabla } \times \boldsymbol {u} = - \nabla ^{2} \psi$, and it appears in the first term of the internal wave equation (2.9) if we set ![]() $\chi = \psi$. Expressing the linear terms of (2.3) in terms of
$\chi = \psi$. Expressing the linear terms of (2.3) in terms of ![]() $\psi$, multiplying by
$\psi$, multiplying by ![]() $g / \rho _{00}$ and differentiating with respect to
$g / \rho _{00}$ and differentiating with respect to ![]() $x$, we obtain
$x$, we obtain
The left-hand side appears in (2.9) and so we may integrate with respect to ![]() $t$ to obtain the vorticity equation,
$t$ to obtain the vorticity equation,
which can also be derived directly from the linearised momentum equation. Vorticity in a fixed control volume changes only due to baroclinic generation or by the introduction of sources applied on the right-hand side of (2.14). For a source ![]() $f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \delta \left ( t - t_0 \right )$ in the internal wave (2.9), the corresponding vorticity source in (2.14) may be expressed in terms of the Heaviside step function,
$f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \delta \left ( t - t_0 \right )$ in the internal wave (2.9), the corresponding vorticity source in (2.14) may be expressed in terms of the Heaviside step function, ![]() ${{\rm H}}$, as
${{\rm H}}$, as ![]() $\int f \, \mathrm {d} t = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) {{\rm H}} \left ( t - t_0 \right )$, which we may interpret as a supply of vorticity at unit rate after
$\int f \, \mathrm {d} t = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) {{\rm H}} \left ( t - t_0 \right )$, which we may interpret as a supply of vorticity at unit rate after ![]() $t_0$.
$t_0$.
While steady-state waves in any system violate causality, they provide a good approximation to their long term behaviour, so in practice, we use monochromatic sources of the form ![]() $f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \exp {\left ( - \mathrm {i} \omega t \right )}$ for any choice of
$f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \exp {\left ( - \mathrm {i} \omega t \right )}$ for any choice of ![]() $\chi$. For the internal potential,
$\chi$. For the internal potential, ![]() $\xi$, the volume source is of unit amplitude and is in phase with
$\xi$, the volume source is of unit amplitude and is in phase with ![]() $f$, and for the streamfunction,
$f$, and for the streamfunction, ![]() $\psi$, we account for the phase difference of a vorticity source by introducing a factor of
$\psi$, we account for the phase difference of a vorticity source by introducing a factor of ![]() $- \mathrm {i} / \omega$.
$- \mathrm {i} / \omega$.
With any choice of ![]() $\chi$, one candidate approach uses Fourier transforms in both time and space (denoted by a circumflex) to yield the algebraic equation,
$\chi$, one candidate approach uses Fourier transforms in both time and space (denoted by a circumflex) to yield the algebraic equation,
 \begin{equation} \hat{\psi} = \frac{\hat{f}}{\omega^{2} \left| \boldsymbol{k} \right|^{2} - N^{2} k^{2}}. \end{equation}
\begin{equation} \hat{\psi} = \frac{\hat{f}}{\omega^{2} \left| \boldsymbol{k} \right|^{2} - N^{2} k^{2}}. \end{equation}We note, however, that the denominator is zero for any Fourier modes that satisfy the dispersion relation, and these correspond to resonant modes. In common with a simple harmonic oscillator, the amplitudes of resonant modes grow linearly. This growth may occur in time, however, over a broad class of wave equations that exist in multiple dimensions, growth may equally occur along spatial directions, and this remains the case for any linear combination of space–time directions (Dobra Reference Dobra2018). Although in the internal wave system each mode is a plane wave of infinite extent, a broadband linear superposition of such modes may be configured to produce an internal wave beam in space with finite width. Counterintuitively, there exists the limiting case of steady-state resonance, where all of the energy is transported away from the source and amplitude growth is found in purely spatial directions.
Dobra (Reference Dobra2018) combined these resonant waves with non-resonant forced oscillations to obtain an integral solution in terms of inverse Fourier transforms. However, exact solutions only apply to periodic domains, yet the experimental configurations we consider in §§ 4 and 5 are best approximated by a combination of reflecting and non-reflecting boundary conditions, which Fourier methods do not in general support. Given that an intermediate aim in § 3 is to establish a numerical method with broad enough generality to handle aperiodicity in both space and time, we must explore alternative techniques for a computationally efficient implementation.
One such approach uses a suitably chosen Green's function, encoding the system response to a point source. A distribution of point sources in space and time may be configured to represent an arbitrary excitation of the system, and in this work we consider distributions that produce interference patterns representing both boundary displacements and mode–mode interactions. For the simplest point source, ![]() $f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \delta \left ( t - t_0 \right )$, Sekerzh-Zen'kovich (Reference Sekerzh-Zen'kovich1981) derived the instantaneous Green's function by Fourier transforming in space only, solving the resulting ordinary differential equation in time and taking the inverse transform. Once again, however, we have non-vanishing solutions at the boundary, and in any finite domain (such as one requires to compute an approximate numerical solution), the Green's function obtained using Fourier techniques encodes the response to a periodic array of isolated point sources. By instead using a sustained monochromatic source,
$f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \delta \left ( t - t_0 \right )$, Sekerzh-Zen'kovich (Reference Sekerzh-Zen'kovich1981) derived the instantaneous Green's function by Fourier transforming in space only, solving the resulting ordinary differential equation in time and taking the inverse transform. Once again, however, we have non-vanishing solutions at the boundary, and in any finite domain (such as one requires to compute an approximate numerical solution), the Green's function obtained using Fourier techniques encodes the response to a periodic array of isolated point sources. By instead using a sustained monochromatic source, ![]() $f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \exp {\left ( - \mathrm {i} \omega t \right )}$, we will obtain a solution in terms of elementary functions (see § 3), so we will avoid difficulties with non-vanishing solutions at the boundary.
$f = \delta \left ( \boldsymbol {x} - \boldsymbol {x}_0 \right ) \exp {\left ( - \mathrm {i} \omega t \right )}$, we will obtain a solution in terms of elementary functions (see § 3), so we will avoid difficulties with non-vanishing solutions at the boundary.
3. Monochromatic Green's function
3.1. Analysis
The monochromatic Green's function, ![]() $G_{\omega } \left ( \boldsymbol {x} ; \boldsymbol {x}_0 \right )$, is the solution to the internal wave equation with point forcing as given by
$G_{\omega } \left ( \boldsymbol {x} ; \boldsymbol {x}_0 \right )$, is the solution to the internal wave equation with point forcing as given by
Provided we have a solution for ![]() $G_{\omega }$, the solution to the internal wave equation with source distribution of the form
$G_{\omega }$, the solution to the internal wave equation with source distribution of the form ![]() $f = f_{\omega } \left ( x, z \right ) \exp {\left ( - \mathrm {i} \omega t \right )}$ is
$f = f_{\omega } \left ( x, z \right ) \exp {\left ( - \mathrm {i} \omega t \right )}$ is
where ![]() $\mathbb {R}^{2}$ spans the two-dimensional physical space.
$\mathbb {R}^{2}$ spans the two-dimensional physical space.
The precise form of the Green's function, ![]() $G_{\omega }$, depends on the configuration of the domain and boundary conditions. In the well studied case of internal tides (e.g. Robinson Reference Robinson1969; Pétrélis, Smith & Young Reference Pétrélis, Smith and Young2006; Balmforth & Peacock Reference Balmforth and Peacock2009), the appropriate Green's function takes the form of a sum of normal modes. However, this is less general than the spatially unbounded case considered by Voisin (Reference Voisin1991), who presented a comprehensive derivation of the three-dimensional Green's functions. His work considered both instantaneous and monochromatic sources and considers in some depth the implications for causality of using Green's functions for internal waves. Motivated by physical arguments, earlier work by Hurley (Reference Hurley1969) quoted the two-dimensional streamfunction due to a monochromatic point vorticity source, which we identify as
$G_{\omega }$, depends on the configuration of the domain and boundary conditions. In the well studied case of internal tides (e.g. Robinson Reference Robinson1969; Pétrélis, Smith & Young Reference Pétrélis, Smith and Young2006; Balmforth & Peacock Reference Balmforth and Peacock2009), the appropriate Green's function takes the form of a sum of normal modes. However, this is less general than the spatially unbounded case considered by Voisin (Reference Voisin1991), who presented a comprehensive derivation of the three-dimensional Green's functions. His work considered both instantaneous and monochromatic sources and considers in some depth the implications for causality of using Green's functions for internal waves. Motivated by physical arguments, earlier work by Hurley (Reference Hurley1969) quoted the two-dimensional streamfunction due to a monochromatic point vorticity source, which we identify as ![]() $-\mathrm {i} \omega G_{\omega }$ in our own work, but this does not include the instantaneous source solution we discussed at the end of § 2. This is important because instantaneous sources are potentially an attractive foundation for a semi-analytical model with sufficient generality to study both wave and non-wave perturbations to a density field. Unfortunately, there is no numerical method for an unbounded Fourier transform, and there are concerns over causality in the spatially periodic domain that we would require for a corresponding numerical method. The simplest causal foundation is the monochromatic source. We note in addition that both Reference HurleyHurley and Reference VoisinVoisin use exponential, rather than linear, density stratifications. The exponential form leads to a distinct interpretation of the buoyancy frequency,
$-\mathrm {i} \omega G_{\omega }$ in our own work, but this does not include the instantaneous source solution we discussed at the end of § 2. This is important because instantaneous sources are potentially an attractive foundation for a semi-analytical model with sufficient generality to study both wave and non-wave perturbations to a density field. Unfortunately, there is no numerical method for an unbounded Fourier transform, and there are concerns over causality in the spatially periodic domain that we would require for a corresponding numerical method. The simplest causal foundation is the monochromatic source. We note in addition that both Reference HurleyHurley and Reference VoisinVoisin use exponential, rather than linear, density stratifications. The exponential form leads to a distinct interpretation of the buoyancy frequency, ![]() $N$, and the linear wave equation includes an additional term arising from the curvature,
$N$, and the linear wave equation includes an additional term arising from the curvature, ![]() $\partial ^{2} \rho _0 / \partial z^{2}$, of the stratification. The solutions in linear and exponential stratifications are related by a conformal map. Given these points and further technical intricacies that are specific to the two-dimensional case and influenced our choice of integration scheme, there is need for presenting our own solution in preparation for a flexible, general numerical implementation.
$\partial ^{2} \rho _0 / \partial z^{2}$, of the stratification. The solutions in linear and exponential stratifications are related by a conformal map. Given these points and further technical intricacies that are specific to the two-dimensional case and influenced our choice of integration scheme, there is need for presenting our own solution in preparation for a flexible, general numerical implementation.
Our solution approach is summarised as follows, with full details in Appendix A. Evaluating the time derivatives in (3.1), defining the constant ![]() $\varGamma = ( 1 - \left ( N / \omega \right )^{2} )^{1/2}$ and cancelling the temporal exponential terms yields
$\varGamma = ( 1 - \left ( N / \omega \right )^{2} )^{1/2}$ and cancelling the temporal exponential terms yields
We note that ![]() $\varGamma$ is real for evanescent internal waves,
$\varGamma$ is real for evanescent internal waves, ![]() $\left | \omega \right | > N$, but is imaginary for
$\left | \omega \right | > N$, but is imaginary for ![]() $\left | \omega \right | < N$. For
$\left | \omega \right | < N$. For ![]() $\varGamma \in \mathbb {R}$, this elliptic equation is a skewed Poisson's equation, and a dilatation allows us to use the free-space Green's function for the unskewed Poisson's equation. Specifically, if
$\varGamma \in \mathbb {R}$, this elliptic equation is a skewed Poisson's equation, and a dilatation allows us to use the free-space Green's function for the unskewed Poisson's equation. Specifically, if ![]() $r$ is the distance from the source in the transformed space so that
$r$ is the distance from the source in the transformed space so that
then the standard Green's function for a source that will generate an evanescent wave is
Analytic continuation from ![]() $\left | \omega \right | > N$ to all
$\left | \omega \right | > N$ to all ![]() $\omega \in \mathbb {R}$ enables a solution to the corresponding hyperbolic equation, and for
$\omega \in \mathbb {R}$ enables a solution to the corresponding hyperbolic equation, and for ![]() $\left | \omega \right | < N$ wavepackets propagate along the real-valued characteristics, as discussed in Dobra et al. (Reference Dobra, Lawrie and Dalziel2021). There are branch points where the argument of a logarithm or a number raised to a fractional power is zero or infinity, so the branch points are at
$\left | \omega \right | < N$ wavepackets propagate along the real-valued characteristics, as discussed in Dobra et al. (Reference Dobra, Lawrie and Dalziel2021). There are branch points where the argument of a logarithm or a number raised to a fractional power is zero or infinity, so the branch points are at ![]() $r^{2} = \left \{ 0 , \infty \right \}$ and
$r^{2} = \left \{ 0 , \infty \right \}$ and ![]() $1 / \varGamma = \left \{ 0 , \infty \right \}$. The
$1 / \varGamma = \left \{ 0 , \infty \right \}$. The ![]() $r^{2} = 0$ branch points,
$r^{2} = 0$ branch points,
 \begin{equation} \omega={\pm} \frac{N}{\sqrt{1 + \left( \dfrac{x - x_0}{z - z_0} \right)^{2}}}, \end{equation}
\begin{equation} \omega={\pm} \frac{N}{\sqrt{1 + \left( \dfrac{x - x_0}{z - z_0} \right)^{2}}}, \end{equation}
only occur where ![]() $\left | \omega \right | \leq N$ and are on the characteristics passing through
$\left | \omega \right | \leq N$ and are on the characteristics passing through ![]() $\boldsymbol {x}_0$. The
$\boldsymbol {x}_0$. The ![]() $1 / \varGamma =\left \{0,\infty \right \}$ branch points correspond to
$1 / \varGamma =\left \{0,\infty \right \}$ branch points correspond to ![]() $\omega =0$ and
$\omega =0$ and ![]() $\omega =\pm N$, respectively, the latter coinciding with
$\omega =\pm N$, respectively, the latter coinciding with ![]() $r^{2}\to \infty$. We tabulate the Green's function for each solution region in table 3 in Appendix A, where we classify by the complex argument of
$r^{2}\to \infty$. We tabulate the Green's function for each solution region in table 3 in Appendix A, where we classify by the complex argument of ![]() $r^{2}$ and
$r^{2}$ and ![]() $1 / \varGamma$. By defining
$1 / \varGamma$. By defining ![]() $\gamma = ( ( N / \omega )^{2} - 1 )^{1/2} = \tan {\varTheta }$, as may be inferred from the dispersion relation (2.7), we condense all the propagating cases to
$\gamma = ( ( N / \omega )^{2} - 1 )^{1/2} = \tan {\varTheta }$, as may be inferred from the dispersion relation (2.7), we condense all the propagating cases to
 \begin{align} G_{\omega} = \mathrm{i} {{\rm sgn}}{\left( \omega \right)} \frac{\log{\left| \left( \dfrac{x - x_0}{\gamma} \right)^{2} - \left( z - z_0 \right)^{2} \right|}}{4 {\rm \pi}\omega^{2} \gamma} + \frac{1}{4 \omega^{2} \gamma} {{\rm H}}{\left( \left( \frac{x - x_0}{\gamma} \right)^{2} - \left( z - z_0 \right)^{2} \right)} . \end{align}
\begin{align} G_{\omega} = \mathrm{i} {{\rm sgn}}{\left( \omega \right)} \frac{\log{\left| \left( \dfrac{x - x_0}{\gamma} \right)^{2} - \left( z - z_0 \right)^{2} \right|}}{4 {\rm \pi}\omega^{2} \gamma} + \frac{1}{4 \omega^{2} \gamma} {{\rm H}}{\left( \left( \frac{x - x_0}{\gamma} \right)^{2} - \left( z - z_0 \right)^{2} \right)} . \end{align} For sources that generate evanescent waves, ![]() $\gamma \in \mathbb {I}$, the Green's function is real, so the response is in phase with the forcing. A contour plot of the Green's function is shown in figure 2. As
$\gamma \in \mathbb {I}$, the Green's function is real, so the response is in phase with the forcing. A contour plot of the Green's function is shown in figure 2. As ![]() $\omega \to \infty$, or equivalently as
$\omega \to \infty$, or equivalently as ![]() $N \to 0$, the elliptical contours broaden to become circular. In the limiting case, this is the unstratified potential flow response corresponding to our choice of
$N \to 0$, the elliptical contours broaden to become circular. In the limiting case, this is the unstratified potential flow response corresponding to our choice of ![]() $\chi$. The contours of the streamfunction,
$\chi$. The contours of the streamfunction, ![]() $\psi$, always represent streamlines in the flow, whereas only in the case when the internal potential,
$\psi$, always represent streamlines in the flow, whereas only in the case when the internal potential, ![]() $\xi$, is monochromatic and
$\xi$, is monochromatic and ![]() $N = 0$ do its contours coincide with those of the classical velocity potential,
$N = 0$ do its contours coincide with those of the classical velocity potential, ![]() $\phi$, as defined by
$\phi$, as defined by ![]() $\boldsymbol {u} = \boldsymbol {\nabla } \phi$. The fundamental streamfunction flow is a monochromatic point vortex, whereas for the internal potential, it is a monochromatic volume source.
$\boldsymbol {u} = \boldsymbol {\nabla } \phi$. The fundamental streamfunction flow is a monochromatic point vortex, whereas for the internal potential, it is a monochromatic volume source.

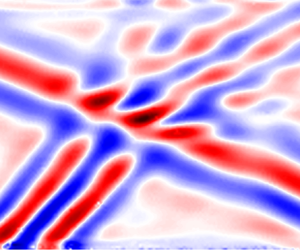
Figure 2. Real component of the evanescent Green's function for ![]() $\omega = 1.1N$, which shows (a) the streamlines and (b) contours of the internal potential, and the derived velocity fields at
$\omega = 1.1N$, which shows (a) the streamlines and (b) contours of the internal potential, and the derived velocity fields at ![]() $t = 0$. The velocity indicators have been scaled for plotting. The potentials and their corresponding fluid speeds grow unboundedly at the origin. The imaginary part is identically zero.
$t = 0$. The velocity indicators have been scaled for plotting. The potentials and their corresponding fluid speeds grow unboundedly at the origin. The imaginary part is identically zero.
For ![]() $\left | \omega \right | < N$
$\left | \omega \right | < N$ ![]() $\left ( \varGamma \in \mathbb {I}, \gamma \in \mathbb {R} \right )$, we obtain propagating solutions with characteristics of gradient
$\left ( \varGamma \in \mathbb {I}, \gamma \in \mathbb {R} \right )$, we obtain propagating solutions with characteristics of gradient ![]() $\pm 1 / \gamma$. The imaginary part of the Green's function for
$\pm 1 / \gamma$. The imaginary part of the Green's function for ![]() $\omega = 0.5N$ is plotted in figure 3. The real part is piecewise constant with discontinuities across the characteristics. When
$\omega = 0.5N$ is plotted in figure 3. The real part is piecewise constant with discontinuities across the characteristics. When ![]() $\left | x - x_0 \right | > \gamma \left | z - z_0 \right |$, the real part equals
$\left | x - x_0 \right | > \gamma \left | z - z_0 \right |$, the real part equals ![]() $1 / ( 4 \omega ^{2} \gamma )$ and equals zero elsewhere. We see a St. Andrew's cross pattern analogous to that produced by a small cylinder undergoing vertical oscillations (Görtler Reference Görtler1943; Mowbray & Rarity Reference Mowbray and Rarity1967). The potential and derived velocities grow unboundedly on approaching the characteristics, which is a consequence of the idealisations (e.g. neglecting viscosity) embedded in this model. Nonetheless, when integrated over point sources of zero area, a finite contribution is obtained in the same way that an integration over
$1 / ( 4 \omega ^{2} \gamma )$ and equals zero elsewhere. We see a St. Andrew's cross pattern analogous to that produced by a small cylinder undergoing vertical oscillations (Görtler Reference Görtler1943; Mowbray & Rarity Reference Mowbray and Rarity1967). The potential and derived velocities grow unboundedly on approaching the characteristics, which is a consequence of the idealisations (e.g. neglecting viscosity) embedded in this model. Nonetheless, when integrated over point sources of zero area, a finite contribution is obtained in the same way that an integration over ![]() $\delta$-functions produces a finite integral, a property we will exploit in § 3.2.
$\delta$-functions produces a finite integral, a property we will exploit in § 3.2.

Figure 3. Imaginary component of the propagating Green's function for ![]() $\omega = 0.5N$, which shows (a) the streamlines and (b) contours of the internal potential, and the derived velocity fields at time
$\omega = 0.5N$, which shows (a) the streamlines and (b) contours of the internal potential, and the derived velocity fields at time ![]() $t = {\rm \pi}/ \left ( 2 \omega \right )$. The velocity indicators have the same scale as those in the evanescent case (figure 2). The potentials and corresponding fluid speeds grow unboundedly at the characteristics, with the largest ones, which would only be visible near the origin, omitted for clarity. The real part is zero in the regions above both characteristics and below both characteristics, and is
$t = {\rm \pi}/ \left ( 2 \omega \right )$. The velocity indicators have the same scale as those in the evanescent case (figure 2). The potentials and corresponding fluid speeds grow unboundedly at the characteristics, with the largest ones, which would only be visible near the origin, omitted for clarity. The real part is zero in the regions above both characteristics and below both characteristics, and is ![]() $1 / ( 4 \omega ^{2} \gamma )$ in the remaining regions to the left of both characteristics and to the right of both characteristics.
$1 / ( 4 \omega ^{2} \gamma )$ in the remaining regions to the left of both characteristics and to the right of both characteristics.
For ![]() $\left | \omega \right | < N$
$\left | \omega \right | < N$ ![]() $\left ( \gamma \in \mathbb {R} \right )$, the separable form of
$\left ( \gamma \in \mathbb {R} \right )$, the separable form of ![]() $r^{2}$ allows us to decompose the argument of the logarithm into two characteristic coordinates,
$r^{2}$ allows us to decompose the argument of the logarithm into two characteristic coordinates,
such that the ![]() $\eta _+$ characteristics have positive slope and
$\eta _+$ characteristics have positive slope and ![]() $\eta _-$ negative. The argument of the logarithm,
$\eta _-$ negative. The argument of the logarithm, ![]() $\left | r^{2} \right |$, becomes a difference of squares because
$\left | r^{2} \right |$, becomes a difference of squares because ![]() $\varGamma ^{2} < 0$, so decomposes into the characteristic coordinates,
$\varGamma ^{2} < 0$, so decomposes into the characteristic coordinates,
Therefore, the logarithm splits into two linearly superposed components with no cross-term,
The solution to a cylinder undergoing small vertical oscillations shares this decoupling into ![]() $\eta _{\pm }$ components (Hurley Reference Hurley1997). In the critical limit
$\eta _{\pm }$ components (Hurley Reference Hurley1997). In the critical limit ![]() $\omega \to N$ from below, the characteristics are vertical, which smoothly transition to the ellipses of contours with infinite aspect ratio in the limit
$\omega \to N$ from below, the characteristics are vertical, which smoothly transition to the ellipses of contours with infinite aspect ratio in the limit ![]() $\omega \to N$ from above.
$\omega \to N$ from above.
3.2. Numerical implementation
In general, we are unable to analytically integrate the Green's function, ![]() $G_{\omega }$, over the distribution of point sources to determine
$G_{\omega }$, over the distribution of point sources to determine ![]() $\chi _{\omega }$ from (3.2). Consequently, we now seek to use our Green's function solution as the basis for a semi-analytical method to evaluate the potential,
$\chi _{\omega }$ from (3.2). Consequently, we now seek to use our Green's function solution as the basis for a semi-analytical method to evaluate the potential, ![]() $\chi _{\omega }$, at arbitrary locations in space. We anticipate distributed sources, so the potential strength at any evaluation point in space will be composed of a linear superposition of solutions from all sources. Unfortunately, our solution has logarithmic singularities along the characteristics, and so any numerical method based on pointwise evaluation will suffer from unresolvable infinities. However, with careful treatment we may regularise these over finite integration elements, and thus we discretise the domain into elements,
$\chi _{\omega }$, at arbitrary locations in space. We anticipate distributed sources, so the potential strength at any evaluation point in space will be composed of a linear superposition of solutions from all sources. Unfortunately, our solution has logarithmic singularities along the characteristics, and so any numerical method based on pointwise evaluation will suffer from unresolvable infinities. However, with careful treatment we may regularise these over finite integration elements, and thus we discretise the domain into elements, ![]() $\mathcal {E}\left (\boldsymbol {x}_D \right )$, of size
$\mathcal {E}\left (\boldsymbol {x}_D \right )$, of size ![]() ${\rm \Delta} x \Delta z$. We account for the effect of integrating over an element by introducing a corresponding modified discrete Green's function,
${\rm \Delta} x \Delta z$. We account for the effect of integrating over an element by introducing a corresponding modified discrete Green's function, ![]() $G_D \left ( \boldsymbol {x} ; \boldsymbol {x}_D \right )$, and source distribution,
$G_D \left ( \boldsymbol {x} ; \boldsymbol {x}_D \right )$, and source distribution, ![]() $f_D \left ( \boldsymbol {x}_D \right )$, where the centres of such elements are at
$f_D \left ( \boldsymbol {x}_D \right )$, where the centres of such elements are at ![]() $\boldsymbol {x}_D$, so that
$\boldsymbol {x}_D$, so that
While much of what follows is required to determine ![]() $G_D$, we may simply take
$G_D$, we may simply take ![]() $f_D \left ( \boldsymbol {x}_D \right ) = \left ( 1 / \left ( \Delta x \Delta z \right ) \right ) \iint _{ {}{\mathcal {E}\left (\boldsymbol {x}_D \right )}} f_{\omega } \left ( \boldsymbol {x}_0 \right ) \, \mathrm {d}^{2} \boldsymbol {x}_0$, integrated over the element. For smooth source distributions, we make the approximation
$f_D \left ( \boldsymbol {x}_D \right ) = \left ( 1 / \left ( \Delta x \Delta z \right ) \right ) \iint _{ {}{\mathcal {E}\left (\boldsymbol {x}_D \right )}} f_{\omega } \left ( \boldsymbol {x}_0 \right ) \, \mathrm {d}^{2} \boldsymbol {x}_0$, integrated over the element. For smooth source distributions, we make the approximation ![]() $f_D \left ( \boldsymbol {x}_D \right ) \approx f_{\omega } \left ( \boldsymbol {x}_D \right )$. If instead there is an isolated
$f_D \left ( \boldsymbol {x}_D \right ) \approx f_{\omega } \left ( \boldsymbol {x}_D \right )$. If instead there is an isolated ![]() $\delta$-function source of strength
$\delta$-function source of strength ![]() $q$ that lies somewhere within the element, the mean source density is
$q$ that lies somewhere within the element, the mean source density is ![]() $f_D = q / ( \Delta x \Delta z )$. Correspondingly, a smooth line source distribution of the form
$f_D = q / ( \Delta x \Delta z )$. Correspondingly, a smooth line source distribution of the form ![]() $f_{\omega } = q \left ( x \right ) \delta \left ( z - z_0 \right )$ has mean density
$f_{\omega } = q \left ( x \right ) \delta \left ( z - z_0 \right )$ has mean density ![]() $f_D \approx q \left ( x_D \right ) / \Delta z$.
$f_D \approx q \left ( x_D \right ) / \Delta z$.
We note in passing that while the transformed coordinate system ![]() $\left ( \eta _+ , \eta _- \right )$ aligns with the characteristic directions of propagating waves for some
$\left ( \eta _+ , \eta _- \right )$ aligns with the characteristic directions of propagating waves for some ![]() $\left | \omega \right | < N$, no single coordinate system would be optimal for a polychromatic wave field (as will be highlighted in § 4). Thus, we opt to discretise a regular Cartesian grid in
$\left | \omega \right | < N$, no single coordinate system would be optimal for a polychromatic wave field (as will be highlighted in § 4). Thus, we opt to discretise a regular Cartesian grid in ![]() $\left ( x , z \right )$.
$\left ( x , z \right )$.
The Green's function only depends on the displacement from the source to the evaluation point, so by moving the reference frame to the centre of the finite element enclosing the source, ![]() $\boldsymbol {x}_D$, we may define a continuous variable
$\boldsymbol {x}_D$, we may define a continuous variable ![]() $\boldsymbol {x}' = \boldsymbol {x} - \boldsymbol {x}_D$ over which we may integrate to determine
$\boldsymbol {x}' = \boldsymbol {x} - \boldsymbol {x}_D$ over which we may integrate to determine ![]() $G_D$ for all elements. Since the grid is regular, then by accepting a small discretisation error no larger than
$G_D$ for all elements. Since the grid is regular, then by accepting a small discretisation error no larger than ![]() $\Delta x / 2$, between the location of the source and the centre of the element we only need to calculate the Green's function once for each relative displacement at any given frequency. Then, we translate the resulting array of values according to
$\Delta x / 2$, between the location of the source and the centre of the element we only need to calculate the Green's function once for each relative displacement at any given frequency. Then, we translate the resulting array of values according to ![]() $\boldsymbol {x}_D$ when evaluating the summation for
$\boldsymbol {x}_D$ when evaluating the summation for ![]() $\chi _{\omega }$ (3.11), truncating any values that fall outside the numerical domain.
$\chi _{\omega }$ (3.11), truncating any values that fall outside the numerical domain.
We choose approximate formulae for each evaluation of the Green's function, ![]() $G_D$, according to the classification in figure 4. The figure only shows elements in the first quadrant, with the other quadrants deduced by symmetry. In the remainder of this section, we explain the decision points and formulae referenced in the figure.
$G_D$, according to the classification in figure 4. The figure only shows elements in the first quadrant, with the other quadrants deduced by symmetry. In the remainder of this section, we explain the decision points and formulae referenced in the figure.

Figure 4. Classification of finite element types in the first quadrant showing the breakdown according to whether ![]() $G_{\omega }$ remains finite within the element, whether propagating or evanescent and by the geometry of the intersections between characteristics and the element boundary. The thumbnail images show
$G_{\omega }$ remains finite within the element, whether propagating or evanescent and by the geometry of the intersections between characteristics and the element boundary. The thumbnail images show ![]() $\mbox {Re}{\left \{ G_{\omega } \right \} }$, which equals
$\mbox {Re}{\left \{ G_{\omega } \right \} }$, which equals ![]() $1 / ( 4 \omega ^{2} \gamma )$ in the shaded regions and zero elsewhere. Formulae for evaluating
$1 / ( 4 \omega ^{2} \gamma )$ in the shaded regions and zero elsewhere. Formulae for evaluating ![]() $G_D$ are given for each case, and the areas for calculating
$G_D$ are given for each case, and the areas for calculating ![]() $\mbox {Re}{\left \{ G_D \right \} }$ in the propagating case are referenced by their case numbers in table 1.
$\mbox {Re}{\left \{ G_D \right \} }$ in the propagating case are referenced by their case numbers in table 1.
Except at the source and elsewhere near its characteristics, the continuous Green's function is regular and may be approximated by a Riemann sum of the form
For ![]() $\left | \omega \right | > N$, the only singular element is that which encloses
$\left | \omega \right | > N$, the only singular element is that which encloses ![]() $\boldsymbol {x} = \boldsymbol {x}_0$, and in this case the integral is given by
$\boldsymbol {x} = \boldsymbol {x}_0$, and in this case the integral is given by
 \begin{equation} G_D \left( \boldsymbol{x}_D ; \boldsymbol{x}_D \right) = \int_{- \Delta x / 2}^{\Delta x / 2} {\int_{- \Delta z / 2}^{\Delta z / 2} {-\frac{\log{\left( \left( \dfrac{x'}{\varGamma} \right)^{2} + z'^{2} \right)}}{4 {\rm \pi}\omega^{2} \varGamma} \, \mathrm{d} z'} \, \mathrm{d}\kern0.7pt x'} . \end{equation}
\begin{equation} G_D \left( \boldsymbol{x}_D ; \boldsymbol{x}_D \right) = \int_{- \Delta x / 2}^{\Delta x / 2} {\int_{- \Delta z / 2}^{\Delta z / 2} {-\frac{\log{\left( \left( \dfrac{x'}{\varGamma} \right)^{2} + z'^{2} \right)}}{4 {\rm \pi}\omega^{2} \varGamma} \, \mathrm{d} z'} \, \mathrm{d}\kern0.7pt x'} . \end{equation}
The dominant contribution to the integral comes from the logarithm close to the singularity, so we approximate the integral on the rectangular element by an ellipse of equivalent area. After dilatation, the radius, ![]() $R$, of the resulting circle is given by
$R$, of the resulting circle is given by ![]() ${\rm \pi} R^{2} = \Delta x \Delta z / \varGamma$. We re-express the Green's function in polar coordinates,
${\rm \pi} R^{2} = \Delta x \Delta z / \varGamma$. We re-express the Green's function in polar coordinates,
Integration by parts gives
For the case where internal waves may be generated, ![]() $\left | \omega \right | < N$, the imaginary part of the Green's function decomposes into the sum of two linearly independent components (3.10), one for each characteristic direction. Using symmetry, singular elements along the
$\left | \omega \right | < N$, the imaginary part of the Green's function decomposes into the sum of two linearly independent components (3.10), one for each characteristic direction. Using symmetry, singular elements along the ![]() $x'$ or
$x'$ or ![]() $z'$ axes intersect both characteristics (the case of two characteristics in figure 4). Conversely, singular elements away from the axes may only intersect one characteristic. We calculate each
$z'$ axes intersect both characteristics (the case of two characteristics in figure 4). Conversely, singular elements away from the axes may only intersect one characteristic. We calculate each ![]() $\eta _{\pm }$ component of
$\eta _{\pm }$ component of ![]() $G_D$ separately and then add them together. For elements significantly away from the corresponding characteristic,
$G_D$ separately and then add them together. For elements significantly away from the corresponding characteristic, ![]() $\eta _{\pm } = 0$, the component of the Green's function varies approximately linearly across the element and we invoke the centre-value approximation for a regular point (3.12). Otherwise, when the characteristic passes through an element or close to one of its corners, we approximate this component of
$\eta _{\pm } = 0$, the component of the Green's function varies approximately linearly across the element and we invoke the centre-value approximation for a regular point (3.12). Otherwise, when the characteristic passes through an element or close to one of its corners, we approximate this component of ![]() $G_D$ using integrals as follows.
$G_D$ using integrals as follows.
Let us consider the ![]() $\eta _+$ component for a singular element, and define
$\eta _+$ component for a singular element, and define ![]() $\eta _R$ and
$\eta _R$ and ![]() $\eta _L$ to be the maximum and minimum values respectively of
$\eta _L$ to be the maximum and minimum values respectively of ![]() $\eta _+ = x' / \gamma - z'$ in this element. Because the level sets of
$\eta _+ = x' / \gamma - z'$ in this element. Because the level sets of ![]() $\eta _+$ are lines of positive gradient and
$\eta _+$ are lines of positive gradient and ![]() $\eta _+$ is increasing in
$\eta _+$ is increasing in ![]() $x'$, the maximum value of
$x'$, the maximum value of ![]() $\eta _+$ occurs in the bottom-right corner of the element and the minimum in the top-left corner, so
$\eta _+$ occurs in the bottom-right corner of the element and the minimum in the top-left corner, so
 \begin{gather} \eta_R = \eta_+ \left( x' + \frac{\Delta x}{2} , z' - \frac{\Delta z}{2} \right) = \frac{x' + \dfrac{\Delta x}{2}}{\gamma} - \left( z' - \frac{\Delta z}{2} \right) , \end{gather}
\begin{gather} \eta_R = \eta_+ \left( x' + \frac{\Delta x}{2} , z' - \frac{\Delta z}{2} \right) = \frac{x' + \dfrac{\Delta x}{2}}{\gamma} - \left( z' - \frac{\Delta z}{2} \right) , \end{gather} \begin{gather}\eta_L = \eta_+ \left( x' - \frac{\Delta x}{2} , z' + \frac{\Delta z}{2} \right) = \frac{x' - \dfrac{\Delta x}{2}}{\gamma} - \left( z' + \frac{\Delta z}{2} \right) . \end{gather}
\begin{gather}\eta_L = \eta_+ \left( x' - \frac{\Delta x}{2} , z' + \frac{\Delta z}{2} \right) = \frac{x' - \dfrac{\Delta x}{2}}{\gamma} - \left( z' + \frac{\Delta z}{2} \right) . \end{gather}
We approximate the contribution across the element by integrating over a rectangle aligned with the characteristic that intersects the element corners where ![]() $\eta _+ = \eta _R$ and
$\eta _+ = \eta _R$ and ![]() $\eta _+ = \eta _L$, and then scale the value by the ratio of areas. The contribution to
$\eta _+ = \eta _L$, and then scale the value by the ratio of areas. The contribution to ![]() $G_D$ is approximately
$G_D$ is approximately
 \begin{align} & \left( \frac{\Delta x \Delta z}{\left| \eta_R - \eta_L \right|} \right) \left( \frac{\mathrm{i}}{4 {\rm \pi}\omega^{2} \gamma} \int_{\eta_L}^{\eta_R} {\log{ \left| \eta_+ \right|} \, \mathrm{d} \eta_+} \right) \nonumber\\ &\quad = \left( \frac{\Delta x \Delta z}{\left| \eta_R - \eta_L \right|} \right) \left( \frac{\mathrm{i}}{4 {\rm \pi}\omega^{2} \gamma} \right) \left( \eta_R \left( \log{\left| \eta_R \right|} - 1 \right) - \eta_L \left( \log{\left| \eta_L \right|} - 1 \right) \right), \end{align}
\begin{align} & \left( \frac{\Delta x \Delta z}{\left| \eta_R - \eta_L \right|} \right) \left( \frac{\mathrm{i}}{4 {\rm \pi}\omega^{2} \gamma} \int_{\eta_L}^{\eta_R} {\log{ \left| \eta_+ \right|} \, \mathrm{d} \eta_+} \right) \nonumber\\ &\quad = \left( \frac{\Delta x \Delta z}{\left| \eta_R - \eta_L \right|} \right) \left( \frac{\mathrm{i}}{4 {\rm \pi}\omega^{2} \gamma} \right) \left( \eta_R \left( \log{\left| \eta_R \right|} - 1 \right) - \eta_L \left( \log{\left| \eta_L \right|} - 1 \right) \right), \end{align}
after integration by parts, where we clarify that ![]() $\eta \log {\eta } = 0$ when
$\eta \log {\eta } = 0$ when ![]() $\eta = 0$. By symmetry, the same expression holds for the singular contribution due to
$\eta = 0$. By symmetry, the same expression holds for the singular contribution due to ![]() $\eta _-$ terms.
$\eta _-$ terms.
This leaves the real part of ![]() $G_{\omega }$ to consider. It is only non-zero in the regions to the left and to the right of the source bounded by the characteristics, which are shown for the first quadrant as shaded regions in figure 4. The real part of the integral over the element is given by
$G_{\omega }$ to consider. It is only non-zero in the regions to the left and to the right of the source bounded by the characteristics, which are shown for the first quadrant as shaded regions in figure 4. The real part of the integral over the element is given by ![]() $1 / ( 4 \omega ^{2} \gamma )$ multiplied by the shaded area. We present in table 1 the formulae for all permutations of shaded area expressed in the
$1 / ( 4 \omega ^{2} \gamma )$ multiplied by the shaded area. We present in table 1 the formulae for all permutations of shaded area expressed in the ![]() $\boldsymbol {x}'$ coordinate system centred on an element.
$\boldsymbol {x}'$ coordinate system centred on an element.
Table 1. Shaded area of each type of singular element centred on ![]() $\boldsymbol {x}'$. These thumbnails are shown for quadrant
$\boldsymbol {x}'$. These thumbnails are shown for quadrant ![]() $1$; other quadrants are deduced by symmetry. It is helpful to observe that
$1$; other quadrants are deduced by symmetry. It is helpful to observe that ![]() $\left | x' \right | = \gamma \left | z' \right |$ on the characteristics. In cases 7–10, in addition to the given criteria, we explicitly require that a characteristic passes through the element: in the first and third quadrants, only the
$\left | x' \right | = \gamma \left | z' \right |$ on the characteristics. In cases 7–10, in addition to the given criteria, we explicitly require that a characteristic passes through the element: in the first and third quadrants, only the ![]() $\eta _+$ characteristic may intersect the element, but in the second and fourth quadrants, only the
$\eta _+$ characteristic may intersect the element, but in the second and fourth quadrants, only the ![]() $\eta _-$ characteristic may intersect it. These areas are multiplied by
$\eta _-$ characteristic may intersect it. These areas are multiplied by ![]() $1 / ( 4 \omega ^{2} \gamma )$ to give
$1 / ( 4 \omega ^{2} \gamma )$ to give ![]() $\mbox {Re}{\left \{ G_D \right \} }$.
$\mbox {Re}{\left \{ G_D \right \} }$.

4. Application to aperiodic configurations
4.1. Introduction
Internal waves are frequently generated by moving boundaries. For example, in the laboratory, McEwan (Reference McEwan1971, Reference McEwan1973) used articulated paddles and Gostiaux et al. (Reference Gostiaux, Didelle, Mercier and Dauxois2007) used rotating cams, but these are best suited to monochromatic excitations. We installed a ‘magic carpet’ (Dobra et al. Reference Dobra, Lawrie and Dalziel2019) in the base of our tank, which has more general possibilities for excitation. Likewise, we generalise our numerical method for a single frequency, ![]() $\chi _{\omega } \exp {\left ( - \mathrm {i} \omega t \right )}$, described in § 3 to those that have a continuous spectrum of frequencies.
$\chi _{\omega } \exp {\left ( - \mathrm {i} \omega t \right )}$, described in § 3 to those that have a continuous spectrum of frequencies.
For a distribution of sources ![]() $f \left ( x , z , t \right ) = \int f_{\omega } \left ( x, z \right ) \exp {\left ( - \mathrm {i} \omega t \right )} \, \mathrm {d} \omega$, we may write
$f \left ( x , z , t \right ) = \int f_{\omega } \left ( x, z \right ) \exp {\left ( - \mathrm {i} \omega t \right )} \, \mathrm {d} \omega$, we may write
Our numerical method allows replacement of these integrals with the discrete Fourier transform, and thus we may approximate general wave fields. We summarise our procedure in algorithm 1. In the special case of a discrete set of input frequencies, we no longer need to resolve the Fourier transform and all the frequencies can be represented exactly.
Algorithm 1 Calculation of potential χ for an arbitrary source distribution f(x, t). It is calculated mode by mode using the discrete monochromatic Green's function, G D. At each frequency, we first evaluate f D and G D, then finally we accumulate contributions to the potential field.

In our model, we consider flexible boundaries as sources of either volume or vorticity. As we saw in § 2, source terms in the internal wave equation for internal potential and streamfunction represent volume and vorticity sources respectively. We now derive formulae for the source terms of both potentials, ![]() $\xi$ and
$\xi$ and ![]() $\psi$, to describe each temporal mode of an arbitrary vertical displacement of a horizontal boundary.
$\psi$, to describe each temporal mode of an arbitrary vertical displacement of a horizontal boundary.
4.2. Representing active boundaries with finite element sources
In both cases, we can readily derive volume fluxes for a monochromatic source of unit strength by integrating the Green's function, so we rescale these fluxes to match a discrete physical representation of a short distance along the boundary. The rescaling factors are collectively the required distribution of sources along the entire length of the boundary. Here, we outline the method and summarise key results; see §§ 4.2.1 and 4.2.2 for full derivations. Throughout this section, all sources are at the zero-height of the magic carpet, so without loss of generality we take ![]() $z_0 = 0$.
$z_0 = 0$.
We seek to determine the volume flux, ![]() $Q \left ( t \right ) = Q_{\omega } \exp {\left ( - \mathrm {i} \omega t \right )}$, induced by a monochromatic source of unit strength across a transect of the domain. For the internal potential, the transect is a horizontal line at
$Q \left ( t \right ) = Q_{\omega } \exp {\left ( - \mathrm {i} \omega t \right )}$, induced by a monochromatic source of unit strength across a transect of the domain. For the internal potential, the transect is a horizontal line at ![]() $z > 0$ ranging from
$z > 0$ ranging from ![]() $x = -\infty$ to
$x = -\infty$ to ![]() $+ \infty$, across which the flux amplitude
$+ \infty$, across which the flux amplitude ![]() $Q_{\omega } = \frac {1}{2}$. Whereas, the corresponding transect for the streamfunction is a vertical line segment to the right of the wave maker (assuming the case of rightward propagating waves,
$Q_{\omega } = \frac {1}{2}$. Whereas, the corresponding transect for the streamfunction is a vertical line segment to the right of the wave maker (assuming the case of rightward propagating waves, ![]() $\omega k >0$) ranging from
$\omega k >0$) ranging from ![]() $z = 0$ to
$z = 0$ to ![]() $+ \infty$, across which
$+ \infty$, across which ![]() $Q_{\omega } = 1 / ( 4 \omega ^{2} \gamma )$. In both cases, we find that the component of the Green's function flow satisfying the conditions imposed by the physical model of the magic carpet is in phase with the forcing,
$Q_{\omega } = 1 / ( 4 \omega ^{2} \gamma )$. In both cases, we find that the component of the Green's function flow satisfying the conditions imposed by the physical model of the magic carpet is in phase with the forcing, ![]() $\mbox {Re}{\left \{ Q_{\omega } \right \} }$, and the implied flow has the form of linear jets along the characteristics, which can be represented by
$\mbox {Re}{\left \{ Q_{\omega } \right \} }$, and the implied flow has the form of linear jets along the characteristics, which can be represented by ![]() $\delta$-functions.
$\delta$-functions.
The total volume flux from one finite grid element of width ![]() $\Delta x$ and height
$\Delta x$ and height ![]() $\Delta z$ that is centred on
$\Delta z$ that is centred on ![]() $\left ( x_D , 0 \right )$ and contains the distribution of monochromatic point sources
$\left ( x_D , 0 \right )$ and contains the distribution of monochromatic point sources ![]() $f = f_{\omega } \left ( x , z \right ) \exp {\left ( - \mathrm {i} \omega t \right )}$ is
$f = f_{\omega } \left ( x , z \right ) \exp {\left ( - \mathrm {i} \omega t \right )}$ is
 \begin{align} \int_{x_D - \Delta x / 2}^{x_D + \Delta x / 2}{\int_{- \Delta z / 2}^{\Delta z / 2}{Q_{\omega} f_{\omega} \left( x', z' \right) \exp{\left( - \mathrm{i} \omega t \right)} \, \mathrm{d} z'} \, \mathrm{d}\kern0.7pt x'} \approx \Delta x \Delta z Q_{\omega} f_{\omega} \left( x_D , 0 \right) \exp{\left( - \mathrm{i} \omega t \right)} . \end{align}
\begin{align} \int_{x_D - \Delta x / 2}^{x_D + \Delta x / 2}{\int_{- \Delta z / 2}^{\Delta z / 2}{Q_{\omega} f_{\omega} \left( x', z' \right) \exp{\left( - \mathrm{i} \omega t \right)} \, \mathrm{d} z'} \, \mathrm{d}\kern0.7pt x'} \approx \Delta x \Delta z Q_{\omega} f_{\omega} \left( x_D , 0 \right) \exp{\left( - \mathrm{i} \omega t \right)} . \end{align}
Then, we equate this expression with the corresponding volume flux, ![]() $R \left ( t \right ) = R_{\omega } \exp {\left ( - \mathrm {i} \omega t \right )}$, predicted by a physical model of volume displacement by the wave maker surface to obtain the distribution of sources,
$R \left ( t \right ) = R_{\omega } \exp {\left ( - \mathrm {i} \omega t \right )}$, predicted by a physical model of volume displacement by the wave maker surface to obtain the distribution of sources,
For the internal potential, ![]() $R_{\omega } = - \mathrm {i} \omega \Delta x h_{\omega } \left ( x_D \right )$, so
$R_{\omega } = - \mathrm {i} \omega \Delta x h_{\omega } \left ( x_D \right )$, so
Whereas, for the streamfunction, ![]() $R_{\omega } = - ( \mathrm {i} \omega \Delta x / 2 ) h_{\omega } \left ( x_D \right )$, so
$R_{\omega } = - ( \mathrm {i} \omega \Delta x / 2 ) h_{\omega } \left ( x_D \right )$, so
4.2.1. Internal potential
For the internal potential, we determine the total vertical volume flux through a line of constant ![]() $z \neq 0$,
$z \neq 0$,
for the Green's function when ![]() $0 < \omega < N$. The vertical velocity field,
$0 < \omega < N$. The vertical velocity field, ![]() $w$, is given by
$w$, is given by ![]() $\partial ^{3} \left ( G_{\omega } \exp {\left ( - \mathrm {i} \omega t \right )} \right ) / \partial t^{2} \partial z$ and thus
$\partial ^{3} \left ( G_{\omega } \exp {\left ( - \mathrm {i} \omega t \right )} \right ) / \partial t^{2} \partial z$ and thus ![]() $w = - \omega ^{2} ( \partial G_{\omega } / \partial z ) \exp {\left ( - \mathrm {i} \omega t \right )}$. Since the following is equally applicable to continuous and discretised sources, we adopt
$w = - \omega ^{2} ( \partial G_{\omega } / \partial z ) \exp {\left ( - \mathrm {i} \omega t \right )}$. Since the following is equally applicable to continuous and discretised sources, we adopt ![]() $\left (x_0,z_0\right )$ notation to represent a source. Applying the chain rule to
$\left (x_0,z_0\right )$ notation to represent a source. Applying the chain rule to ![]() $G_{\omega }$ (3.7) when
$G_{\omega }$ (3.7) when ![]() $z_0 = 0$, we obtain
$z_0 = 0$, we obtain
 \begin{equation} \frac{\partial G_{\omega}}{\partial z} ={-} \mathrm{i} \frac{z}{2 {\rm \pi}\omega^{2} \gamma \left[ \left( \dfrac{x - x_0}{\gamma} \right)^{2} - z^{2} \right]} - \frac{z}{2 \omega^{2} \gamma} \delta{\left( \left( \frac{x - x_0}{\gamma} \right)^{2} - z^{2} \right)}. \end{equation}
\begin{equation} \frac{\partial G_{\omega}}{\partial z} ={-} \mathrm{i} \frac{z}{2 {\rm \pi}\omega^{2} \gamma \left[ \left( \dfrac{x - x_0}{\gamma} \right)^{2} - z^{2} \right]} - \frac{z}{2 \omega^{2} \gamma} \delta{\left( \left( \frac{x - x_0}{\gamma} \right)^{2} - z^{2} \right)}. \end{equation} Along a path of constant ![]() $z$ (where
$z$ (where ![]() $z \neq 0$) as shown in figure 5, the imaginary part has two simple poles,
$z \neq 0$) as shown in figure 5, the imaginary part has two simple poles, ![]() $x = x_0 \pm \gamma z$, which are where the path crosses the characteristics of
$x = x_0 \pm \gamma z$, which are where the path crosses the characteristics of ![]() $G_{\omega }$, and
$G_{\omega }$, and ![]() $\partial G_{\omega } / \partial z$ asymptotes inverse–linearly towards them. Between the poles (in the line segment containing
$\partial G_{\omega } / \partial z$ asymptotes inverse–linearly towards them. Between the poles (in the line segment containing ![]() $x = x_0$),
$x = x_0$), ![]() ${{\rm sgn}}{\left ( \mbox {Im}{\left \{ \partial G_{\omega } / \partial z \right \}} \right )} = + {{\rm sgn}}{\left ( z \right )}$, and outside the poles (where
${{\rm sgn}}{\left ( \mbox {Im}{\left \{ \partial G_{\omega } / \partial z \right \}} \right )} = + {{\rm sgn}}{\left ( z \right )}$, and outside the poles (where ![]() $x \to \pm \infty$),
$x \to \pm \infty$), ![]() ${{\rm sgn}}{\left ( \mbox {Im}{\left \{ \partial G_{\omega } / \partial z \right \} } \right )} = - {{\rm sgn}}{\left ( z \right )}$. Thus, we may use the Cauchy principle value to regularise
${{\rm sgn}}{\left ( \mbox {Im}{\left \{ \partial G_{\omega } / \partial z \right \} } \right )} = - {{\rm sgn}}{\left ( z \right )}$. Thus, we may use the Cauchy principle value to regularise ![]() $Q_{\omega }$ at each pole. The imaginary part exhibits even symmetry about
$Q_{\omega }$ at each pole. The imaginary part exhibits even symmetry about ![]() $x = x_0$, so it suffices to consider only half of the domain and double the result,
$x = x_0$, so it suffices to consider only half of the domain and double the result,
 \begin{align} \mbox{Im}{\left\{ Q_{\omega} \left( z \right) \right\}} = \lim_{\epsilon \to 0} \left\{ \frac{z}{{\rm \pi} \gamma} \left( \int_{x_0}^{x_0 + \gamma z - \epsilon} \frac{\mathrm{d}\kern0.7pt x}{\left( \dfrac{x - x_0}{\gamma} \right)^{2} - z^{2}} - \int_{x_0 + \gamma z + \epsilon}^{\infty} \frac{\mathrm{d}\kern0.7pt x}{\left( \dfrac{x - x_0}{\gamma} \right)^{2} - z^{2}} \right) \right\} . \end{align}
\begin{align} \mbox{Im}{\left\{ Q_{\omega} \left( z \right) \right\}} = \lim_{\epsilon \to 0} \left\{ \frac{z}{{\rm \pi} \gamma} \left( \int_{x_0}^{x_0 + \gamma z - \epsilon} \frac{\mathrm{d}\kern0.7pt x}{\left( \dfrac{x - x_0}{\gamma} \right)^{2} - z^{2}} - \int_{x_0 + \gamma z + \epsilon}^{\infty} \frac{\mathrm{d}\kern0.7pt x}{\left( \dfrac{x - x_0}{\gamma} \right)^{2} - z^{2}} \right) \right\} . \end{align}
Factoring out ![]() $z^{2}$ and using the substitution
$z^{2}$ and using the substitution ![]() $p = \left ( x - x_0 \right ) / \left ( \gamma z \right )$ leaves
$p = \left ( x - x_0 \right ) / \left ( \gamma z \right )$ leaves
 \begin{equation} \mbox{Im}{\left\{ Q_{\omega} \left( z \right) \right\}} = \lim_{\epsilon \to 0} \left\{ \frac{1}{\rm \pi} \left( \int_{0}^{1 - \epsilon / ( \gamma z )}{\frac{\mathrm{d} p}{p^{2} - 1}} - \int_{1 + \epsilon / ( \gamma z )}^{\infty}{\frac{\mathrm{d} p}{p^{2} - 1}} \right) \right\} . \end{equation}
\begin{equation} \mbox{Im}{\left\{ Q_{\omega} \left( z \right) \right\}} = \lim_{\epsilon \to 0} \left\{ \frac{1}{\rm \pi} \left( \int_{0}^{1 - \epsilon / ( \gamma z )}{\frac{\mathrm{d} p}{p^{2} - 1}} - \int_{1 + \epsilon / ( \gamma z )}^{\infty}{\frac{\mathrm{d} p}{p^{2} - 1}} \right) \right\} . \end{equation}
The scaling on the limit variable, ![]() $\epsilon$, is the same for both integrals, so we may replace the corresponding limits on the integrals by
$\epsilon$, is the same for both integrals, so we may replace the corresponding limits on the integrals by ![]() $1 \mp \epsilon$. Then, evaluating the definite integrals yields
$1 \mp \epsilon$. Then, evaluating the definite integrals yields
 \begin{equation} \mbox{Im}{\left\{ Q_{\omega} \left( z \right) \right\}} = \lim_{\epsilon \to 0} {\left\{ \frac{1}{2 {\rm \pi}} \left( \left[ \log{\frac{1 - p}{1 + p}} \right]_0^{1-\epsilon} - \left[ \log{\frac{p - 1}{p + 1}} \right]_{1+\epsilon}^{\infty} \right) \right\}} = 0 . \end{equation}
\begin{equation} \mbox{Im}{\left\{ Q_{\omega} \left( z \right) \right\}} = \lim_{\epsilon \to 0} {\left\{ \frac{1}{2 {\rm \pi}} \left( \left[ \log{\frac{1 - p}{1 + p}} \right]_0^{1-\epsilon} - \left[ \log{\frac{p - 1}{p + 1}} \right]_{1+\epsilon}^{\infty} \right) \right\}} = 0 . \end{equation}
Figure 5. Integration path for calculating the volume flux, ![]() $Q$, for the internal potential, showing the locations of the poles in the imaginary part of
$Q$, for the internal potential, showing the locations of the poles in the imaginary part of ![]() $\partial G_{\omega } / \partial z$, which are also the locations of the singularities in the real part.
$\partial G_{\omega } / \partial z$, which are also the locations of the singularities in the real part.
Next, we consider the integral over the ![]() $\delta$-function in the real part. Along a path of constant
$\delta$-function in the real part. Along a path of constant ![]() $z$, the argument of the
$z$, the argument of the ![]() $\delta$-function has two simple zeros,
$\delta$-function has two simple zeros, ![]() $y_{1,2} = x_0 \pm \gamma z$, for which we use the standard formula,
$y_{1,2} = x_0 \pm \gamma z$, for which we use the standard formula,
 \begin{equation} \delta \left( f \left( x \right) \right) = \sum_{k = 1}^{2} \frac{\delta \left( x - y_k \right)}{\left| \left. \dfrac{\mathrm{d} f}{\mathrm{d}\kern0.7pt x} \right|_{y_k} \right|}. \end{equation}
\begin{equation} \delta \left( f \left( x \right) \right) = \sum_{k = 1}^{2} \frac{\delta \left( x - y_k \right)}{\left| \left. \dfrac{\mathrm{d} f}{\mathrm{d}\kern0.7pt x} \right|_{y_k} \right|}. \end{equation}
Here, ![]() $\mathrm {d} f / \mathrm {d} x = 2 ( x - x_0 ) / \gamma ^{2}$, so we have
$\mathrm {d} f / \mathrm {d} x = 2 ( x - x_0 ) / \gamma ^{2}$, so we have
 \begin{equation} \mbox{Re}{\left\{ Q_{\omega} \left( z \right) \right\}} = \int_{- \infty}^{\infty}{\frac{z}{2 \gamma} \left( \frac{\delta \left( x - \left[ x_0 + \gamma z \right] \right)}{\left| \dfrac{2}{\gamma^{2}} \gamma z \right|} + \frac{\delta \left( x - \left[ x_0 - \gamma z \right] \right)}{\left| - \dfrac{2}{\gamma^{2}} \gamma z \right|} \right) \, \mathrm{d}\kern0.7pt x}. \end{equation}
\begin{equation} \mbox{Re}{\left\{ Q_{\omega} \left( z \right) \right\}} = \int_{- \infty}^{\infty}{\frac{z}{2 \gamma} \left( \frac{\delta \left( x - \left[ x_0 + \gamma z \right] \right)}{\left| \dfrac{2}{\gamma^{2}} \gamma z \right|} + \frac{\delta \left( x - \left[ x_0 - \gamma z \right] \right)}{\left| - \dfrac{2}{\gamma^{2}} \gamma z \right|} \right) \, \mathrm{d}\kern0.7pt x}. \end{equation}
Each ![]() $\delta$-function contributes a value of one to the integral and
$\delta$-function contributes a value of one to the integral and ![]() $z / | z | = {{\rm sgn}}{\left ( z \right )}$, so
$z / | z | = {{\rm sgn}}{\left ( z \right )}$, so ![]() $\mbox {Re}{\{ Q_{\omega } \left ( z \right ) \}} = \frac {1}{2} {{\rm sgn}}{\left ( z \right )}$. Therefore,
$\mbox {Re}{\{ Q_{\omega } \left ( z \right ) \}} = \frac {1}{2} {{\rm sgn}}{\left ( z \right )}$. Therefore, ![]() $Q \left ( z \right ) = \frac {1}{2} {{\rm sgn}}{\left ( z \right )} \exp {(-\mathrm {i} \omega t)}$.
$Q \left ( z \right ) = \frac {1}{2} {{\rm sgn}}{\left ( z \right )} \exp {(-\mathrm {i} \omega t)}$.
The total vertical volume flux through a horizontal transect is half the strength of the internal potential point source and is in phase with the source. The flux has a vertical component everywhere except ![]() $z = 0$ and points away from the source when the source is positive. Closing a rectangular contour along
$z = 0$ and points away from the source when the source is positive. Closing a rectangular contour along ![]() $z = \pm z_0$ and
$z = \pm z_0$ and ![]() $x = \pm \infty$, symmetry arguments determine that the vertical integrals at
$x = \pm \infty$, symmetry arguments determine that the vertical integrals at ![]() $x = \pm \infty$ are both zero and integration along the horizontal edges doubles due to the direction in which they are taken. Thus, a monochromatic point source of internal potential of unit strength forces the internal wave equation such that the total volume flux is monochromatic and of unit strength.
$x = \pm \infty$ are both zero and integration along the horizontal edges doubles due to the direction in which they are taken. Thus, a monochromatic point source of internal potential of unit strength forces the internal wave equation such that the total volume flux is monochromatic and of unit strength.
We remark that this result also applies to a corresponding integral when the Green's function is for the streamfunction,
Using the same rectangular contour, we obtain the circulation around the point source to be ![]() $\frac {1}{2} \exp {(-\mathrm {i} \omega t)}$. Letting
$\frac {1}{2} \exp {(-\mathrm {i} \omega t)}$. Letting ![]() $z \to 0$ so that the area enclosed in the contour tends to zero and invoking Stokes’ theorem shows that the source is a point vortex of strength
$z \to 0$ so that the area enclosed in the contour tends to zero and invoking Stokes’ theorem shows that the source is a point vortex of strength ![]() $\frac {1}{2} \exp {(-\mathrm {i} \omega t)}$.
$\frac {1}{2} \exp {(-\mathrm {i} \omega t)}$.
Similar to a resonant simple harmonic oscillator, there are components of the internal potential field both in phase to the forcing and with a phase lag of a quarter oscillation behind the source. Here, the in-phase response ensures the conservation of volume by generating line jets only and exactly along the characteristics, while the phase-lagged response has zero net volume flux despite inducing a flow over the whole domain.
Physically modelling the wave maker, the upwards volume flux generated by an element with vertical displacement ![]() $h$, as shown in figure 6, is
$h$, as shown in figure 6, is
 \begin{equation} R \left( t \right) = \int_{x_D - \Delta x / 2}^{x_D + \Delta x / 2}{\frac{\partial h}{\partial t} \, \mathrm{d}\kern0.7pt x'}, \end{equation}
\begin{equation} R \left( t \right) = \int_{x_D - \Delta x / 2}^{x_D + \Delta x / 2}{\frac{\partial h}{\partial t} \, \mathrm{d}\kern0.7pt x'}, \end{equation}
which is approximately equal to ![]() $- \mathrm {i} \omega \, \Delta x \, h_{\omega } \exp {(-\mathrm {i} \omega t)}$ for small elements. Substituting this into the formula for the required element source strength (4.3) yields the required source strengths for use in the discrete Green's function,
$- \mathrm {i} \omega \, \Delta x \, h_{\omega } \exp {(-\mathrm {i} \omega t)}$ for small elements. Substituting this into the formula for the required element source strength (4.3) yields the required source strengths for use in the discrete Green's function, ![]() $f_{\omega } \left ( x_D , 0 \right ) = - ( 2 \mathrm {i} \omega / \Delta z ) h_{\omega } \left ( x_D \right )$.
$f_{\omega } \left ( x_D , 0 \right ) = - ( 2 \mathrm {i} \omega / \Delta z ) h_{\omega } \left ( x_D \right )$.

Figure 6. Finite element of width ![]() $\Delta x$ representing wave maker displacement at a single location. We use this model to calculate the induced vertical volume flux required for sources to the internal potential, which is
$\Delta x$ representing wave maker displacement at a single location. We use this model to calculate the induced vertical volume flux required for sources to the internal potential, which is ![]() $\Delta x \left. \partial h / \partial t \right |_{\left ( x_D , t \right )}$. The vertical dashed lines indicate the element centrelines.
$\Delta x \left. \partial h / \partial t \right |_{\left ( x_D , t \right )}$. The vertical dashed lines indicate the element centrelines.
4.2.2. Streamfunction
When the Green's function represents the streamfunction, the volume flux across any horizontal or vertical transect is zero, because sources in the streamfunction internal wave equation are vortices. Instead, since the volume flux across a path is equal to the difference between the values of the streamfunction at each end, we note that the real part of the volume flux induced by ![]() $G_{\omega }$ (3.7) across any semi-infinite vertical line from
$G_{\omega }$ (3.7) across any semi-infinite vertical line from ![]() $z = 0$ to
$z = 0$ to ![]() $z = \infty$ for constant
$z = \infty$ for constant ![]() $x$ is
$x$ is ![]() $Q_{\omega } = 1 / ( 4 \omega ^{2} \gamma )$. The point vortex is in phase with the source, so it is not necessary to consider the imaginary part.
$Q_{\omega } = 1 / ( 4 \omega ^{2} \gamma )$. The point vortex is in phase with the source, so it is not necessary to consider the imaginary part.
For the physical model, we consider a wave maker profile that is spatially sampled every ![]() $\delta x$ and is zero everywhere except at one sample point,
$\delta x$ and is zero everywhere except at one sample point, ![]() $x = x_p$, as shown in figure 7. We assume that the wave maker is piecewise linear between the sample points. The rightwards volume flux generated to the right of the displaced infinitesimal element is
$x = x_p$, as shown in figure 7. We assume that the wave maker is piecewise linear between the sample points. The rightwards volume flux generated to the right of the displaced infinitesimal element is ![]() $R \left ( x_p + \delta x , t \right ) = ( \Delta x / 2 ) \partial h / \partial t |_{\left ( x_p , t \right )}$. Conversely, the rightwards volume flux to the left of the element is
$R \left ( x_p + \delta x , t \right ) = ( \Delta x / 2 ) \partial h / \partial t |_{\left ( x_p , t \right )}$. Conversely, the rightwards volume flux to the left of the element is ![]() $R \left ( x_p - \delta x , t \right ) = - ( \Delta x / 2 ) \partial h / \partial t |_{\left ( x_p , t \right )}$. In the continuum limit, we have
$R \left ( x_p - \delta x , t \right ) = - ( \Delta x / 2 ) \partial h / \partial t |_{\left ( x_p , t \right )}$. In the continuum limit, we have
 \begin{equation} \left. \frac{\partial R}{\partial x} \right|_{\left( x_p , t \right)} = \lim_{\delta x \to 0}{\frac{R \left( x_p + \delta x , t \right) - R \left( x_p - \delta x , t \right)}{2 \delta x}} = \frac{1}{2} \left. \frac{\partial h}{\partial t} \right|_{\left( x_p , t \right)}. \end{equation}
\begin{equation} \left. \frac{\partial R}{\partial x} \right|_{\left( x_p , t \right)} = \lim_{\delta x \to 0}{\frac{R \left( x_p + \delta x , t \right) - R \left( x_p - \delta x , t \right)}{2 \delta x}} = \frac{1}{2} \left. \frac{\partial h}{\partial t} \right|_{\left( x_p , t \right)}. \end{equation}
Integrating such point contributions over a finite element of width ![]() $\Delta x$ centred on
$\Delta x$ centred on ![]() $\left ( x_D , 0 \right )$ gives the total horizontal volume flux across one source element,
$\left ( x_D , 0 \right )$ gives the total horizontal volume flux across one source element,
 \begin{equation} R \left( x_D , t \right) = \int_{x_D - \Delta x / 2}^{x_D + \Delta x / 2}{\frac{1}{2} \frac{\partial h}{\partial t} \, \mathrm{d}\kern0.7pt x} \approx \frac{\Delta x}{2} \left. \frac{\partial h}{\partial t} \right|_{\left( x_D , t \right)} , \end{equation}
\begin{equation} R \left( x_D , t \right) = \int_{x_D - \Delta x / 2}^{x_D + \Delta x / 2}{\frac{1}{2} \frac{\partial h}{\partial t} \, \mathrm{d}\kern0.7pt x} \approx \frac{\Delta x}{2} \left. \frac{\partial h}{\partial t} \right|_{\left( x_D , t \right)} , \end{equation}
to leading order in ![]() $\Delta x$. Thus,
$\Delta x$. Thus, ![]() $R_{\omega } = - ( \mathrm {i} \omega \Delta x / 2 ) h_{\omega } \left ( x_D \right )$ and
$R_{\omega } = - ( \mathrm {i} \omega \Delta x / 2 ) h_{\omega } \left ( x_D \right )$ and

Figure 7. Infinitesimal element representing wave maker displacement at a single location, assuming the profile to be linear between sample points ![]() $\delta x$ apart and the domain to have a rigid lid. We use this model to calculate along-wave maker gradients of induced horizontal volume flux, whose integrals are required for finite sources of width
$\delta x$ apart and the domain to have a rigid lid. We use this model to calculate along-wave maker gradients of induced horizontal volume flux, whose integrals are required for finite sources of width ![]() $\Delta x$ to the streamfunction.
$\Delta x$ to the streamfunction.
4.3. Boundary considerations
Non-zero-frequency internal waves in a finite domain will inevitably reflect off the top and bottom. In both cases, the fluid cannot flow across the boundary, so we take ![]() $w = 0$ as the boundary condition and exploit the characteristic structure of internal waves to enforce it. The required potential,
$w = 0$ as the boundary condition and exploit the characteristic structure of internal waves to enforce it. The required potential, ![]() $\chi$, for the reflected wave is calculated along the boundary and then projected along its characteristics using an approach introduced in Dobra et al. (Reference Dobra, Lawrie and Dalziel2021). The characteristics of the reflected wave are oriented in the opposite vertical, but same horizontal, direction as the incident wave.
$\chi$, for the reflected wave is calculated along the boundary and then projected along its characteristics using an approach introduced in Dobra et al. (Reference Dobra, Lawrie and Dalziel2021). The characteristics of the reflected wave are oriented in the opposite vertical, but same horizontal, direction as the incident wave.
For the internal potential in a monochromatic flow, ![]() $w = - \omega ^{2}\partial \xi / \partial z$ and on the boundary, the reflected wave may take the same value of the internal potential as the incident wave. By contrast, the vertical velocity is obtained from the streamfunction as
$w = - \omega ^{2}\partial \xi / \partial z$ and on the boundary, the reflected wave may take the same value of the internal potential as the incident wave. By contrast, the vertical velocity is obtained from the streamfunction as ![]() $w = \partial \psi / \partial x$, giving
$w = \partial \psi / \partial x$, giving ![]() $\psi = \textrm {const.}$ on the boundary, so we require that the reflected streamfunction is the negative of the incident. In both cases, we set the gauge constant to zero for convenience.
$\psi = \textrm {const.}$ on the boundary, so we require that the reflected streamfunction is the negative of the incident. In both cases, we set the gauge constant to zero for convenience.
Evaluating ![]() $\chi$ only on the boundary is insufficient to deduce the horizontal direction of incident characteristics. A wave field of a particular frequency may contain waves in all directions, so we use a principal axes transformation to decompose the incident wave field into left- and right-travelling waves according to the direction of the gradient vector (Dobra Reference Dobra2018, pp. 37–39) and reflect each component in turn.
$\chi$ only on the boundary is insufficient to deduce the horizontal direction of incident characteristics. A wave field of a particular frequency may contain waves in all directions, so we use a principal axes transformation to decompose the incident wave field into left- and right-travelling waves according to the direction of the gradient vector (Dobra Reference Dobra2018, pp. 37–39) and reflect each component in turn.
The interference pattern arising from the distribution of sources generates the desired wave field, but where the source array is abruptly truncated, powerful harmonics are emitted and they may contaminate the solution within the domain. To reduce the severity of such truncation, we smoothly reduce the source strength to zero at the lateral extremities of the calculation domain according to a ![]() $C^{3}$-continuous ramp that extends well beyond the field of view. Similarly, we use a significantly extended temporal domain for the Fourier transform to avoid periodic reflection in time activity that is aperiodic and short in duration.
$C^{3}$-continuous ramp that extends well beyond the field of view. Similarly, we use a significantly extended temporal domain for the Fourier transform to avoid periodic reflection in time activity that is aperiodic and short in duration.
4.4. Experimental method
The ‘magic carpet’ or Arbitrary Spectrum Wave Maker (Dobra et al. Reference Dobra, Lawrie and Dalziel2019) is a flexible 1 m long and flush with the base of a tank that is 11 m long, 0.255 m wide and 0.48 m deep. The magic carpet's shape is controlled by an array of ![]() $100$ linear stepper motors positioned at a pitch of 10 mm, each with a vertical resolution of 0.0127 mm and a stroke of 48 mm.
$100$ linear stepper motors positioned at a pitch of 10 mm, each with a vertical resolution of 0.0127 mm and a stroke of 48 mm.
The surface of the wave maker is a nylon-faced neoprene foam sheet of thickness 3 mm. The material has some resistance to bending, but the attachment mechanism is designed to minimise the tensile stress in the sheet and the bending moments on the actuating rods. We model the surface deformations at each instant, ![]() $h \left ( x , t \right )$, as satisfying
$h \left ( x , t \right )$, as satisfying
where ![]() $E$ is Young's modulus,
$E$ is Young's modulus, ![]() $s$ is the sheet thickness,
$s$ is the sheet thickness, ![]() $T$ is the longitudinal tension in the sheet and
$T$ is the longitudinal tension in the sheet and ![]() $p_*$ is the pressure difference across the sheet, normally taken as zero. Defining
$p_*$ is the pressure difference across the sheet, normally taken as zero. Defining ![]() $\lambda = ( 12 T / ( E s^{3} ) )^{1/2}$, this equation has eigensolutions
$\lambda = ( 12 T / ( E s^{3} ) )^{1/2}$, this equation has eigensolutions ![]() $\boldsymbol {f} \left ( x \right ) = \left [\begin {smallmatrix} 1 & x & \cosh {\lambda x} & \sinh {\lambda x}\end {smallmatrix}\right ]^{\operatorname {T}}$. For our magic carpet, we find that
$\boldsymbol {f} \left ( x \right ) = \left [\begin {smallmatrix} 1 & x & \cosh {\lambda x} & \sinh {\lambda x}\end {smallmatrix}\right ]^{\operatorname {T}}$. For our magic carpet, we find that ![]() $\lambda \approx 400$. These solutions differ from the typical Euler–Bernoulli linear beam by the presence of hyperbolic functions instead of cubic polynomials, and these differences arise from longitudinal tension. Defining a vector of constants
$\lambda \approx 400$. These solutions differ from the typical Euler–Bernoulli linear beam by the presence of hyperbolic functions instead of cubic polynomials, and these differences arise from longitudinal tension. Defining a vector of constants ![]() $\boldsymbol {b}$ to be determined by the rod heights and enforcing
$\boldsymbol {b}$ to be determined by the rod heights and enforcing ![]() $C^{2}$-continuity, the general solution between each rod is
$C^{2}$-continuity, the general solution between each rod is ![]() $h \left ( x \right ) = \boldsymbol {b} \boldsymbol {\cdot } \boldsymbol {f} \left ( x \right )$. Combining the boundary conditions for all sections of the wave maker gives a linear system of equations with constant coefficients, which can be easily inverted numerically.
$h \left ( x \right ) = \boldsymbol {b} \boldsymbol {\cdot } \boldsymbol {f} \left ( x \right )$. Combining the boundary conditions for all sections of the wave maker gives a linear system of equations with constant coefficients, which can be easily inverted numerically.
We fill the tank using the double bucket method (Fortuin Reference Fortuin1960; Oster Reference Oster1965) with a linear density stratification in brine producing a constant buoyancy frequency ![]() $N = 1.45\,{\rm rad}\,{\rm s}^{-1}$. We observe density perturbations using Synthetic Schlieren, an optical technique (Dalziel et al. Reference Dalziel, Hughes and Sutherland1998; Sutherland et al. Reference Sutherland, Dalziel, Hughes and Linden1999; Dalziel et al. Reference Dalziel, Hughes and Sutherland2000). A static, random pattern of black and white dots is displayed on a 4k (UHD) television screen measuring 1.4 m
$N = 1.45\,{\rm rad}\,{\rm s}^{-1}$. We observe density perturbations using Synthetic Schlieren, an optical technique (Dalziel et al. Reference Dalziel, Hughes and Sutherland1998; Sutherland et al. Reference Sutherland, Dalziel, Hughes and Linden1999; Dalziel et al. Reference Dalziel, Hughes and Sutherland2000). A static, random pattern of black and white dots is displayed on a 4k (UHD) television screen measuring 1.4 m ![]() $\left ( 55'' \right )$ on the diagonal that is 0.2 m behind the tank, following Sveen & Dalziel (Reference Sveen and Dalziel2005). The light rays emitting from the screen bend as they pass through the varying refractive indices in the tank, and the distorted images are recorded at
$\left ( 55'' \right )$ on the diagonal that is 0.2 m behind the tank, following Sveen & Dalziel (Reference Sveen and Dalziel2005). The light rays emitting from the screen bend as they pass through the varying refractive indices in the tank, and the distorted images are recorded at ![]() $4\,\textrm {f.p.s.}$ on a 12-megapixel ISVI IC-X12CXP video camera located 3.8 m in front of the tank. A pattern-matching algorithm in the software package DigiFlow (Dalziel Research Partners 2018) is used to reconstruct the gradient of the density perturbation from the recorded images, and we plot its horizontal component, which is related to the internal potential according to
$4\,\textrm {f.p.s.}$ on a 12-megapixel ISVI IC-X12CXP video camera located 3.8 m in front of the tank. A pattern-matching algorithm in the software package DigiFlow (Dalziel Research Partners 2018) is used to reconstruct the gradient of the density perturbation from the recorded images, and we plot its horizontal component, which is related to the internal potential according to ![]() $( 1 / \rho _{00} ) \partial \rho ' / \partial x = ( N^{2} / g ) \partial ^{3} \xi / \partial x \partial z \partial t$ for linear waves.
$( 1 / \rho _{00} ) \partial \rho ' / \partial x = ( N^{2} / g ) \partial ^{3} \xi / \partial x \partial z \partial t$ for linear waves.
4.5. An example: atmospheric lee waves
A travelling solitary hump is perhaps the simplest aperiodic waveform, directly analogous to flow over an isolated mountain ridge (Dalziel et al. Reference Dalziel, Patterson, Caulfield and Le Brun2011). In our experiments, the fluid is stationary in the tank, so boundary layers do not form upstream and we obtain cleaner waveforms.
We seek to validate our numerical model in this configuration, and we choose to calculate the wave field using the internal potential, although we could equally obtain the same wave field using the streamfunction. Our hump consists of a complete wavelength of a sinusoid ranging from trough to trough, where the troughs are flush with the zero height of the magic carpet, with wavelength 0.081 m and peak-to-trough amplitude 0.028 m propagating to the right at ![]() $U = 0.0357\,{\rm m}\,{\rm s}^{-1}$. We use
$U = 0.0357\,{\rm m}\,{\rm s}^{-1}$. We use ![]() $1024$ points spanning 50 s for the discrete Fourier transform, giving a frequency resolution of 0.13 rad s
$1024$ points spanning 50 s for the discrete Fourier transform, giving a frequency resolution of 0.13 rad s![]() $^{-1}$. The hump takes 2.3 s to pass any fixed location, which corresponds to a frequency of 2.8 rad s
$^{-1}$. The hump takes 2.3 s to pass any fixed location, which corresponds to a frequency of 2.8 rad s![]() $^{-1}$. With this adequate temporal resolution, we thus avoid spurious reflections in the time domain.
$^{-1}$. With this adequate temporal resolution, we thus avoid spurious reflections in the time domain.
The passing time of the hump corresponds to a frequency ratio of ![]() $\omega / N = 1.88$, which is evanescent. Thus, it is clear that in this case the propagating modes will arise only from peripheral harmonics in the spectrum, an observation to which we will return in § 5. Figure 8 compares our experiments with the wave train predicted by our model.
$\omega / N = 1.88$, which is evanescent. Thus, it is clear that in this case the propagating modes will arise only from peripheral harmonics in the spectrum, an observation to which we will return in § 5. Figure 8 compares our experiments with the wave train predicted by our model.

Figure 8. Prediction and experiment comparing ![]() $( 1 / \rho _{00} ) \partial \rho ' / \partial x$ for a solitary sinusoidal hump of height 0.028 m and width 0.081 m moving at
$( 1 / \rho _{00} ) \partial \rho ' / \partial x$ for a solitary sinusoidal hump of height 0.028 m and width 0.081 m moving at ![]() $U = 0.0357\,{\rm m}\,{\rm s}^{-1}$. Each image is separated by 5 s, and
$U = 0.0357\,{\rm m}\,{\rm s}^{-1}$. Each image is separated by 5 s, and ![]() $N = 1.45\,{\rm rad}\,{\rm s}^{-1}$. The majority of the wave energy exists in waves phase-locked with the hump, and these waves are restricted to a semi-circular envelope, indicated by the black arc. Wave energy to the right of the arc is carried by non-phase-locked waves, but whose spectrum results in a quasi-steady pattern of waves moving with the hump. There are also evanescent modes forming an interference pattern near the hump, but due to discretisation of the temporal spectrum in our prediction, some leakage of energy occurs along the wave maker surface but the response remains localised.
$N = 1.45\,{\rm rad}\,{\rm s}^{-1}$. The majority of the wave energy exists in waves phase-locked with the hump, and these waves are restricted to a semi-circular envelope, indicated by the black arc. Wave energy to the right of the arc is carried by non-phase-locked waves, but whose spectrum results in a quasi-steady pattern of waves moving with the hump. There are also evanescent modes forming an interference pattern near the hump, but due to discretisation of the temporal spectrum in our prediction, some leakage of energy occurs along the wave maker surface but the response remains localised.
Firstly, from selective withdrawal of modes in our numerical prediction, we deduce that there are significant evanescent modes local to the hump, whose interference pattern is required to capture the structure of the wave train observed in the experiment.
Secondly, we see disturbances spread across the domain, both in front and behind the hump. The waves ahead of the hump appear to be quasi-stationary and persist in the observed timeframe between six and eleven passing periods of the hump after its release. We conclude that these are not simply startup transients, and so we use geometric reasoning to understand the distribution of wave energy in the system.
One common approach is to use the principle of stationary phase (e.g. Lighthill Reference Lighthill1978) to restrict our analysis to elements of the wave field that move in phase with the hump. The solitary hump may be characterised as a broadband spectrum of modes all travelling with a common horizontal phase velocity, ![]() $\omega / k = U$. It follows that for a given range of wavenumbers,
$\omega / k = U$. It follows that for a given range of wavenumbers, ![]() $k$, there must directly correspond a range of frequencies,
$k$, there must directly correspond a range of frequencies, ![]() $\omega$. Thus, any internal wave propagation generated by the hump has no preferential direction but must share the same horizontal component of phase velocity. The dispersion relation (2.7) constrains the magnitudes of all such wavevectors to the circle
$\omega$. Thus, any internal wave propagation generated by the hump has no preferential direction but must share the same horizontal component of phase velocity. The dispersion relation (2.7) constrains the magnitudes of all such wavevectors to the circle ![]() $\left | \boldsymbol {k} \right | = N / U$. Consequently, for positive frequencies and upwards propagation, only fourth-quadrant wavevectors remain, and their corresponding group velocities point into the first quadrant. These modes comprise the majority of the observed signal, and their superposition results in curved phase lines. Furthermore, by following rays traced parallel to each mode's respective group velocity, we may determine a propagation envelope for this class of quasi-steady wave. This envelope forms a semi-circle joining the hump's current and initial release locations (Dalziel et al. Reference Dalziel, Patterson, Caulfield and Le Brun2011), as shown by black arcs in figure 8. These advancing semi-circles grow in radius until the envelope asymptotically forms a vertical front.
$\left | \boldsymbol {k} \right | = N / U$. Consequently, for positive frequencies and upwards propagation, only fourth-quadrant wavevectors remain, and their corresponding group velocities point into the first quadrant. These modes comprise the majority of the observed signal, and their superposition results in curved phase lines. Furthermore, by following rays traced parallel to each mode's respective group velocity, we may determine a propagation envelope for this class of quasi-steady wave. This envelope forms a semi-circle joining the hump's current and initial release locations (Dalziel et al. Reference Dalziel, Patterson, Caulfield and Le Brun2011), as shown by black arcs in figure 8. These advancing semi-circles grow in radius until the envelope asymptotically forms a vertical front.
Clearly, both the experiment and the prediction contain waves propagating ahead of this envelope, so, as previously noted by Voisin (Reference Voisin1994), the principle of stationary phase is insufficient to account for the whole wave field. Given that there is signal high above the wave maker and ahead of the hump, we deduce that these waves must have significant vertical component to their group velocity and therefore have non-zero frequency. Moreover, for internal waves the horizontal component of the group velocity is bounded above by the horizontal component of the phase velocity, and any observable wave ahead of the hump must have group velocity with horizontal component greater than ![]() $U$, so the same must also be true for its phase velocity. Although counterintuitive, it is possible for a composition of modes from a spectrum of phase velocities to form a quasi-steady wave field that translates with a single apparent phase velocity. Akin to the decomposition of a standing wave into opposing travelling waves, a carefully chosen difference of frequencies is sufficient to create the required behaviour, although many combinations of wavevector and frequency would also produce an equivalent result. We conjecture that just such a superposition of modes is responsible for propagation ahead of the envelope shown in figure 8.
$U$, so the same must also be true for its phase velocity. Although counterintuitive, it is possible for a composition of modes from a spectrum of phase velocities to form a quasi-steady wave field that translates with a single apparent phase velocity. Akin to the decomposition of a standing wave into opposing travelling waves, a carefully chosen difference of frequencies is sufficient to create the required behaviour, although many combinations of wavevector and frequency would also produce an equivalent result. We conjecture that just such a superposition of modes is responsible for propagation ahead of the envelope shown in figure 8.
We note that our approach requires the Fourier transform in time of the entire timeline. Since the source strengths are zero at all times except when the hump is passing, the ![]() $\omega$-spectrum is broad. However, a discrete Fourier transform introduces discretisation error, which when inverted produces sources at unwanted times. We see their effect as forced oscillations along the magic carpet, which have insignificant effect on the rest of the wave field.
$\omega$-spectrum is broad. However, a discrete Fourier transform introduces discretisation error, which when inverted produces sources at unwanted times. We see their effect as forced oscillations along the magic carpet, which have insignificant effect on the rest of the wave field.
Our wave propagation model does not directly account for energy leaving the modelled system during a reflection, yet is present in the experiments. We employed a line-search optimisation to determine suitable calibration parameters and accordingly multiply the amplitude of the reflections at the free top surface by ![]() $0.6$ while maintaining pure reflections at the solid bottom boundary. Figure 9 demonstrates the necessity of accounting for this energy loss.
$0.6$ while maintaining pure reflections at the solid bottom boundary. Figure 9 demonstrates the necessity of accounting for this energy loss.

Figure 9. Predictions assuming pure reflections (a) and with calibrated attenuation at the free surface with coefficient ![]() $0.6$ (b) compared with experiment (c). These correspond to figure 8 at 25 s and show
$0.6$ (b) compared with experiment (c). These correspond to figure 8 at 25 s and show ![]() $( 1 / \rho _{00} ) \partial \rho ' / \partial x$. Without attenuation, the predicted amplitude of the wave field behind the hump is larger than observed. The reflection coefficient accounts for energy dissipated at the free surface through mechanisms not directly modelled.
$( 1 / \rho _{00} ) \partial \rho ' / \partial x$. Without attenuation, the predicted amplitude of the wave field behind the hump is larger than observed. The reflection coefficient accounts for energy dissipated at the free surface through mechanisms not directly modelled.
5. Interactions of finite-width internal wave beams
5.1. Introduction
The literature on internal wave laboratory experiments can be divided into two broad lines of enquiry: work following from Görtler (Reference Görtler1943) and Mowbray & Rarity (Reference Mowbray and Rarity1967) on waves generated by a small oscillating body, and work on quasi-monochromatic line sources following McEwan (Reference McEwan1971, Reference McEwan1973). The capability of our magic carpet allows us to span the range between these limiting cases, and although previous work using it (Dobra et al. Reference Dobra, Lawrie and Dalziel2021) validated new theoretical predictions in the line-source limit, we seek here to demonstrate the generality of these findings by applying them to an intermediate regime. We examine the interactions of finite-width wave beams a few wavelengths across, since recent explorations of such configurations (Smith & Crockett Reference Smith and Crockett2014) have uncovered a rich dynamical structure. We have unparalleled access to observe and analyse such wave fields processed first with synthetic Schlieren and then with Dynamic Mode Decomposition (DMD, Schmid Reference Schmid2010). For the cases we consider here, DMD is an ideal tool because the frequency discretisation is responsive to the input, so it takes many fewer samples to accurately recover the dominant frequencies compared with Fourier methods, which project onto basis functions at a fixed discretisation. Furthermore, DMD enables us to distinguish between steady-state behaviours and transient modes. Our experiments have been carefully configured so that steady-state behaviours dominate, and we do not observe the common unsteady phenomenon of triadic resonant instability.
5.2. A series expansion for triadic interactions
Building on the recent developments of Dobra et al. (Reference Dobra, Lawrie and Dalziel2021), here we introduce a fusion of our perturbation expansion framework and the method of solution by Green's function, enabling the construction of general wave fields from the interference patterns produced by a distribution of sources. In Dobra et al., the perturbation expansion at each order yields the internal wave equation in terms of the streamfunction with sources that are Jacobian determinants. Under particular symmetries, we found that these sources cancel, preventing a broad class of wave–wave interactions from occurring. Here, we instead consider configurations where these sources play a significant role in the structure of the wave field, and employing the Green's function with the streamfunction potential, it integrates naturally. We now outline a generalisation of our perturbation framework for these arbitrary wave fields.
We reformulate the conservation of momentum (2.1) and mass (2.3) in terms of streamfunction, ![]() $\psi$, and buoyancy,
$\psi$, and buoyancy, ![]() $b = - g \rho ' / \rho _{00}$,
$b = - g \rho ' / \rho _{00}$,
 \begin{gather} \frac{\partial}{\partial t} \nabla^{2} \psi + \left| \frac{\partial \left( \psi , \nabla^{2} \psi \right)}{\partial \left( x, z \right)} \right| = \frac{\partial b}{\partial x}, \end{gather}
\begin{gather} \frac{\partial}{\partial t} \nabla^{2} \psi + \left| \frac{\partial \left( \psi , \nabla^{2} \psi \right)}{\partial \left( x, z \right)} \right| = \frac{\partial b}{\partial x}, \end{gather}
where the Jacobian determinant of two scalars, ![]() $\alpha$ and
$\alpha$ and ![]() $\beta$, is given by
$\beta$, is given by
Eliminating the linear ![]() $b$ terms leaves the nonlinear internal wave equation,
$b$ terms leaves the nonlinear internal wave equation,
 \begin{equation} \frac{\partial^{2}}{\partial t^{2}} \nabla^{2} \psi + N^{2} \frac{\partial^{2} \psi}{\partial x^{2}} = \frac{\partial}{\partial t} \left| \frac{\partial \left( \nabla^{2} \psi , \psi \right)}{\partial \left( x, z \right)} \right| + \frac{\partial}{\partial x} \left| \frac{\partial \left( b , \psi \right)}{\partial \left( x, z \right)} \right| . \end{equation}
\begin{equation} \frac{\partial^{2}}{\partial t^{2}} \nabla^{2} \psi + N^{2} \frac{\partial^{2} \psi}{\partial x^{2}} = \frac{\partial}{\partial t} \left| \frac{\partial \left( \nabla^{2} \psi , \psi \right)}{\partial \left( x, z \right)} \right| + \frac{\partial}{\partial x} \left| \frac{\partial \left( b , \psi \right)}{\partial \left( x, z \right)} \right| . \end{equation}Nonlinearity associated with triadic interactions is captured by source terms of the form of Jacobian determinants, and here we consider their behaviour in the case
 \begin{equation} \psi = \sum_{j = 1}^{3}{\left\{ A_j \exp{\left( \mathrm{i} \left[ \boldsymbol{k}_j \boldsymbol{\cdot} \boldsymbol{x} - \omega_j t \right] \right)} + A_j^{*} \exp{\left( - \mathrm{i} \left[ \boldsymbol{k}_j \boldsymbol{\cdot} \boldsymbol{x} - \omega_j t \right] \right) } \right\}} , \end{equation}
\begin{equation} \psi = \sum_{j = 1}^{3}{\left\{ A_j \exp{\left( \mathrm{i} \left[ \boldsymbol{k}_j \boldsymbol{\cdot} \boldsymbol{x} - \omega_j t \right] \right)} + A_j^{*} \exp{\left( - \mathrm{i} \left[ \boldsymbol{k}_j \boldsymbol{\cdot} \boldsymbol{x} - \omega_j t \right] \right) } \right\}} , \end{equation}
where we require complex conjugate ![]() $\left ( {}^{*} \right )$ pairs to represent real wave fields. The source terms multiply pairs of waves, so we must consider each possible pairing in turn. Self-interactions equate to zero (McEwan Reference McEwan1973; Tabaei & Akylas Reference Tabaei and Akylas2003; Dobra et al. Reference Dobra, Lawrie and Dalziel2021), but the interaction of beam
$\left ( {}^{*} \right )$ pairs to represent real wave fields. The source terms multiply pairs of waves, so we must consider each possible pairing in turn. Self-interactions equate to zero (McEwan Reference McEwan1973; Tabaei & Akylas Reference Tabaei and Akylas2003; Dobra et al. Reference Dobra, Lawrie and Dalziel2021), but the interaction of beam ![]() $j = 1$ with beam
$j = 1$ with beam ![]() $j = 2$ produces terms proportional to
$j = 2$ produces terms proportional to ![]() $\exp {\left ( \mathrm {i} \left [ \left ( \boldsymbol {k}_2 + \boldsymbol {k}_1 \right ) \boldsymbol {\cdot } \boldsymbol {x} - \left ( \omega _2 + \omega _1 \right ) t \right ] \right ) }$,
$\exp {\left ( \mathrm {i} \left [ \left ( \boldsymbol {k}_2 + \boldsymbol {k}_1 \right ) \boldsymbol {\cdot } \boldsymbol {x} - \left ( \omega _2 + \omega _1 \right ) t \right ] \right ) }$, ![]() $\exp {\left ( \mathrm {i} \left [ \left ( \boldsymbol {k}_2 - \boldsymbol {k}_1 \right ) \boldsymbol {\cdot } \boldsymbol {x} - \left ( \omega _2 - \omega _1 \right ) t \right ] \right ) }$ and their complex conjugates. Thus, by index manipulation we may define
$\exp {\left ( \mathrm {i} \left [ \left ( \boldsymbol {k}_2 - \boldsymbol {k}_1 \right ) \boldsymbol {\cdot } \boldsymbol {x} - \left ( \omega _2 - \omega _1 \right ) t \right ] \right ) }$ and their complex conjugates. Thus, by index manipulation we may define
Should this disturbance characterised by ![]() $\boldsymbol {k}_3$ and
$\boldsymbol {k}_3$ and ![]() $\omega _3$ satisfy the dispersion relation (2.7),
$\omega _3$ satisfy the dispersion relation (2.7), ![]() $\omega _3 = N k_3 / \left | \boldsymbol {k}_3 \right |$, the disturbance is also a wave and the source terms are an eigensolution of the internal wave equation (2.9). Such combinations are commonly described as resonant triads.
$\omega _3 = N k_3 / \left | \boldsymbol {k}_3 \right |$, the disturbance is also a wave and the source terms are an eigensolution of the internal wave equation (2.9). Such combinations are commonly described as resonant triads.
We examine in figure 10(a) the geometric permutations of wave triad that may be constructed for a given ![]() $\boldsymbol {k}_1$,
$\boldsymbol {k}_1$, ![]() $\omega _1$ and fixed frequency
$\omega _1$ and fixed frequency ![]() $\omega _2$ but where wavevector
$\omega _2$ but where wavevector ![]() $\boldsymbol {k}_2$ is unconstrained. These triangles are compatible with the selection rules derived by Tabaei et al. (Reference Tabaei, Akylas and Lamb2005) and Jiang & Marcus (Reference Jiang and Marcus2009) that determine into which quadrants, if any, a new wave beam may be emitted. These configurations are typical of wave beams a few wavelengths across for which the wavenumber spectra are broad. In these cases, the spectrum of the source terms is significant across a patch of wavevector space, as shown in figure 10(b). Wavevectors that lie on the dashed locus of dispersion-relation-satisfying
$\boldsymbol {k}_2$ is unconstrained. These triangles are compatible with the selection rules derived by Tabaei et al. (Reference Tabaei, Akylas and Lamb2005) and Jiang & Marcus (Reference Jiang and Marcus2009) that determine into which quadrants, if any, a new wave beam may be emitted. These configurations are typical of wave beams a few wavelengths across for which the wavenumber spectra are broad. In these cases, the spectrum of the source terms is significant across a patch of wavevector space, as shown in figure 10(b). Wavevectors that lie on the dashed locus of dispersion-relation-satisfying ![]() $\boldsymbol {k}_3$ will resonate, and new waves will emerge by mode selection; these correspond to cases where the triangle of wavevectors can be closed.
$\boldsymbol {k}_3$ will resonate, and new waves will emerge by mode selection; these correspond to cases where the triangle of wavevectors can be closed.

Figure 10. Wavevector triangles for the sum of frequencies ![]() $\omega _1 + \omega _2$ in the case
$\omega _1 + \omega _2$ in the case ![]() $\omega _1 = 0.55\,{\rm rad}\,{\rm s}^{-1} = 0.37 N$ and
$\omega _1 = 0.55\,{\rm rad}\,{\rm s}^{-1} = 0.37 N$ and ![]() $\omega _2 = 1.5 \omega _1$. In (a), all permutations of wavevector triangles are presented for the case where
$\omega _2 = 1.5 \omega _1$. In (a), all permutations of wavevector triangles are presented for the case where ![]() $\boldsymbol {k}_1$ points into the first quadrant. The triangles for all other quadrants are obtained by reflective symmetry. From the dispersion relation (2.7), each frequency has four possible directions for its wavevector, one in each quadrant. Given
$\boldsymbol {k}_1$ points into the first quadrant. The triangles for all other quadrants are obtained by reflective symmetry. From the dispersion relation (2.7), each frequency has four possible directions for its wavevector, one in each quadrant. Given ![]() $\boldsymbol {k}_1$, the loci of
$\boldsymbol {k}_1$, the loci of ![]() $\boldsymbol {k}_2$ and
$\boldsymbol {k}_2$ and ![]() $\boldsymbol {k}_3$ will in general close to form a triangle in one of four different ways. A closed triangle is a resonant triad. In (b), we plot in greyscale the distribution of source term amplitudes in Fourier space for incident wavevector distributions
$\boldsymbol {k}_3$ will in general close to form a triangle in one of four different ways. A closed triangle is a resonant triad. In (b), we plot in greyscale the distribution of source term amplitudes in Fourier space for incident wavevector distributions ![]() $\boldsymbol {k}_1$ (blue) and
$\boldsymbol {k}_1$ (blue) and ![]() $\boldsymbol {k}_2$ (red). The resonant, propagating
$\boldsymbol {k}_2$ (red). The resonant, propagating ![]() $\boldsymbol {k}_3$ lie at the intersections of each of the dashed lines with regions of significant source amplitude. The remainder produce a local interference pattern of forced oscillations. In this configuration, two waves at
$\boldsymbol {k}_3$ lie at the intersections of each of the dashed lines with regions of significant source amplitude. The remainder produce a local interference pattern of forced oscillations. In this configuration, two waves at ![]() $\omega _3$ are emitted: a weaker one with
$\omega _3$ are emitted: a weaker one with ![]() $\boldsymbol {k}_3$ pointing into the first quadrant (wave propagating down and to the right, triangle marked in orange in (a)), and a stronger one with
$\boldsymbol {k}_3$ pointing into the first quadrant (wave propagating down and to the right, triangle marked in orange in (a)), and a stronger one with ![]() $\boldsymbol {k}_3$ pointing into the fourth quadrant (up and to the right, green triangle in (a)).
$\boldsymbol {k}_3$ pointing into the fourth quadrant (up and to the right, green triangle in (a)).
No polychromatic solutions containing multiple horizontal phase velocities are known for the fully nonlinear equation (5.3), so in Dobra et al. (Reference Dobra, Lawrie and Dalziel2021), we performed a perturbation expansion to give a recursive algorithm that we can truncate at finite order to calculate an approximate solution. In this earlier work, we expanded ![]() $\psi$ in powers of a small parameter,
$\psi$ in powers of a small parameter, ![]() $a$, which we took to be the wave steepness. Instead, here we modify the expansion to be
$a$, which we took to be the wave steepness. Instead, here we modify the expansion to be ![]() $\psi = \sum _{n = 1}^{\infty } \psi _n$, where each subsequent term drops an order of magnitude, and the expansion for
$\psi = \sum _{n = 1}^{\infty } \psi _n$, where each subsequent term drops an order of magnitude, and the expansion for ![]() $b$ behaves correspondingly. Then, as our earlier work showed, each order satisfies
$b$ behaves correspondingly. Then, as our earlier work showed, each order satisfies
 \begin{equation} \frac{\partial^{2}}{\partial t^{2}} \nabla^{2} \psi_n + N^{2} \frac{\partial^{2} \psi_n}{\partial x^{2}} = \sum_{p=1}^{n-1}{\left\{ \frac{\partial}{\partial t} \left| \frac{\partial \left( \nabla^{2} \psi_{p} , \psi_{n-p} \right)}{\partial \left( x, z \right)} \right| + \frac{\partial}{\partial x} \left| \frac{\partial \left( b_{p} , \psi_{n-p} \right)}{\partial \left( x, z \right)} \right| \right\} } . \end{equation}
\begin{equation} \frac{\partial^{2}}{\partial t^{2}} \nabla^{2} \psi_n + N^{2} \frac{\partial^{2} \psi_n}{\partial x^{2}} = \sum_{p=1}^{n-1}{\left\{ \frac{\partial}{\partial t} \left| \frac{\partial \left( \nabla^{2} \psi_{p} , \psi_{n-p} \right)}{\partial \left( x, z \right)} \right| + \frac{\partial}{\partial x} \left| \frac{\partial \left( b_{p} , \psi_{n-p} \right)}{\partial \left( x, z \right)} \right| \right\} } . \end{equation}
There is a cascade of information from lower order to higher, but not in reverse, thus the expansion is purely inductive. However, at all orders greater than three, there are contributions to frequencies that already exist at lower orders. There is an infinite series of such contributions to the wave field, some of which manifest as corrections to existing wave beams (as we will see later in figure 14) but may also generate waves propagating in new directions. We use the polarisation relation of linear internal waves to calculate ![]() $b = N^{2} \int \partial \psi / \partial x \, \mathrm {d} t$.
$b = N^{2} \int \partial \psi / \partial x \, \mathrm {d} t$.
5.3. Computational method
We use a method based on integration across finite elements to predict the steady-state wave field due to two crossing internal wave beams, exploiting the symmetry of the complex conjugate to avoid unnecessary execution. In the general case, we use a calculation domain of ![]() $346 \times 57$ elements with aspect ratio one, and incident waves are produced using an array of sources, following the method in § 4. For these configurations, we plot
$346 \times 57$ elements with aspect ratio one, and incident waves are produced using an array of sources, following the method in § 4. For these configurations, we plot ![]() $(1 / \rho _{00} ) \partial \rho ' / \partial z = \left ( N^{2} / g \right ) \int ( \partial ^{2} \psi / \partial x \partial z ) \mathrm {d} t$.
$(1 / \rho _{00} ) \partial \rho ' / \partial z = \left ( N^{2} / g \right ) \int ( \partial ^{2} \psi / \partial x \partial z ) \mathrm {d} t$.
The source terms to the internal wave equation involve third-order derivatives, and any errors may propagate across the domain in spurious wave beams. These derivatives are recursively applied each time we increase the order of the perturbation expansion. In our calculations, we evaluate the expansion to third order and thus the original field is differentiated six times. To control numerical noise, we use ghost cells to employ eighth-order centred finite differences, and we perform one sweep of elliptic smoothing to eliminate mesh-scale truncation error in these derivatives. We take care to ensure that there is a separation of length scale between those of the input and those associated with the mesh, thus the smoothing has negligible effect on derivatives that contribute to the physics of the system.
Where we look at the detailed physics of wave–wave interactions, we initialise the streamfunction with idealised waveforms corresponding to magic carpet displacement profiles,
The amplitude of the wave, ![]() $A$, and the width of the envelope,
$A$, and the width of the envelope, ![]() $L$, are configured to match the experiments, which themselves are configured to approximate the asymptotic limit of a wave beam propagating in a viscous fluid (Hurley & Keady Reference Hurley and Keady1997; Sutherland et al. Reference Sutherland, Dalziel, Hughes and Linden1999). However, such waveforms have a broad spectrum including both left- and right-travelling waves (see Dobra et al. Reference Dobra, Lawrie and Dalziel2019). To produce a unidirectional wave, we nullify Fourier modes according to their sign and transform back into physical space, a procedure known as the Hilbert transform (Mercier, Garnier & Dauxois Reference Mercier, Garnier and Dauxois2008). Then, we project this profile along the characteristics, using cubic spline interpolation to obtain element-centred values. For these calculations, we use a grid of
$L$, are configured to match the experiments, which themselves are configured to approximate the asymptotic limit of a wave beam propagating in a viscous fluid (Hurley & Keady Reference Hurley and Keady1997; Sutherland et al. Reference Sutherland, Dalziel, Hughes and Linden1999). However, such waveforms have a broad spectrum including both left- and right-travelling waves (see Dobra et al. Reference Dobra, Lawrie and Dalziel2019). To produce a unidirectional wave, we nullify Fourier modes according to their sign and transform back into physical space, a procedure known as the Hilbert transform (Mercier, Garnier & Dauxois Reference Mercier, Garnier and Dauxois2008). Then, we project this profile along the characteristics, using cubic spline interpolation to obtain element-centred values. For these calculations, we use a grid of ![]() $128 \times 128$ elements with aspect ratio that are non-unity.
$128 \times 128$ elements with aspect ratio that are non-unity.
5.4. Experimental method
We use the same experimental apparatus and diagnostics as § 4. To maximise the amplitudes of the wave beams without inducing locally separated flow near the magic carpet, the amplitudes are increased linearly from rest before reaching a steady state. Data acquisition is performed over two minutes in this steady state. To build on the work of Tabaei et al. (Reference Tabaei, Akylas and Lamb2005), Jiang & Marcus (Reference Jiang and Marcus2009) and Smith & Crockett (Reference Smith and Crockett2014), we seek to examine multiple orientations of incident wave beams and achieve these by exploiting reflections off the free surface, as shown in figure 11(a).

Figure 11. Geometry of wave beams in tank for intersecting two internal waves with the same horizontal direction and opposite vertical direction of the group velocity. Panel (a) shows our experiment, (b) shows our corresponding prediction and (c) shows a schematic of all visible wave beams with blue, red, green and orange corresponding to first-, second-, third- and fourth-order waves, respectively. Beam ![]() $1$, of frequency
$1$, of frequency ![]() $\omega _1 = 0.55\,{\rm rad}\,{\rm s}^{-1} \approx 0.37 N$, is generated at the left end of the wave maker, then reflects off the free surface to intersect beam
$\omega _1 = 0.55\,{\rm rad}\,{\rm s}^{-1} \approx 0.37 N$, is generated at the left end of the wave maker, then reflects off the free surface to intersect beam ![]() $2$, of frequency
$2$, of frequency ![]() $\omega _2 = 2.2 \omega _1$. Among others, a triadic interaction generates a third wave beam at frequency
$\omega _2 = 2.2 \omega _1$. Among others, a triadic interaction generates a third wave beam at frequency ![]() $\omega _2 - \omega _1$ in the grey rectangle, which is the region of interest in subsequent figures. The diagnostic shown is the vertical gradient of the normalised density perturbation,
$\omega _2 - \omega _1$ in the grey rectangle, which is the region of interest in subsequent figures. The diagnostic shown is the vertical gradient of the normalised density perturbation, ![]() $( 1 / \rho _{00} ) \partial \rho ' / \partial z$.
$( 1 / \rho _{00} ) \partial \rho ' / \partial z$.
Here, we use the technique of DMD (Schmid Reference Schmid2010) to identify the temporal modes of a video sequence. Closely related to proper orthogonal decomposition, the method takes an observable representation of the system's state, ![]() $\boldsymbol {y}$, and finds the best-fit system evolution operator,
$\boldsymbol {y}$, and finds the best-fit system evolution operator, ![]() ${\boldsymbol{\mathsf{A}}}$, such that
${\boldsymbol{\mathsf{A}}}$, such that ![]() $\mathrm {d} \boldsymbol {y} / \mathrm {d} t = {\boldsymbol{\mathsf{A}}} \boldsymbol {y}$ when averaged over some period. If
$\mathrm {d} \boldsymbol {y} / \mathrm {d} t = {\boldsymbol{\mathsf{A}}} \boldsymbol {y}$ when averaged over some period. If ![]() ${\boldsymbol{\mathsf{Y}}}$ is composed of a temporal sequence of column vectors of states
${\boldsymbol{\mathsf{Y}}}$ is composed of a temporal sequence of column vectors of states ![]() $\boldsymbol {y}$ and we let
$\boldsymbol {y}$ and we let ![]() ${\boldsymbol{\mathsf{Y}}} = {\boldsymbol{\mathsf{U}}} \boldsymbol {\varSigma } {\boldsymbol{\mathsf{V}}}^{\operatorname {T}}$ be its singular value decomposition, then
${\boldsymbol{\mathsf{Y}}} = {\boldsymbol{\mathsf{U}}} \boldsymbol {\varSigma } {\boldsymbol{\mathsf{V}}}^{\operatorname {T}}$ be its singular value decomposition, then ![]() $\hat {{\boldsymbol{\mathsf{U}}}}$ may denote a truncation of
$\hat {{\boldsymbol{\mathsf{U}}}}$ may denote a truncation of ![]() ${\boldsymbol{\mathsf{U}}}$ that only includes modes with important singular values. Performing an approximate principal axis transformation of
${\boldsymbol{\mathsf{U}}}$ that only includes modes with important singular values. Performing an approximate principal axis transformation of ![]() ${\boldsymbol{\mathsf{A}}}$ to the truncated basis
${\boldsymbol{\mathsf{A}}}$ to the truncated basis ![]() $\hat {{\boldsymbol{\mathsf{U}}}}$ and then an eigendecomposition of
$\hat {{\boldsymbol{\mathsf{U}}}}$ and then an eigendecomposition of ![]() ${\boldsymbol{\mathsf{A}}}$ in the new basis, we have
${\boldsymbol{\mathsf{A}}}$ in the new basis, we have
The dynamic modes are the pairing ![]() $\hat {{\boldsymbol{\mathsf{U}}}} \hat {{\boldsymbol{\mathsf{W}}}}$, and generally they each have distinct complex conjugate pairs of eigenvalues, whose phases determine their frequencies. They may be independently evolved in time, but here we plot these modes evaluated at a common time origin.
$\hat {{\boldsymbol{\mathsf{U}}}} \hat {{\boldsymbol{\mathsf{W}}}}$, and generally they each have distinct complex conjugate pairs of eigenvalues, whose phases determine their frequencies. They may be independently evolved in time, but here we plot these modes evaluated at a common time origin.
5.5. Results and discussion
We begin by comparing our numerical prediction with the output of the synthetic Schlieren in figure 11 using parameters as given in table 2. We highlight each visible wave beam schematically in figure 11(c) using the colours blue, red, green and orange to indicate successive orders of the perturbation expansion in § 5.2. Specifically, components at first order comprise the two primary input beams at frequencies ![]() $\omega _1$ and
$\omega _1$ and ![]() $\omega _2$, while at second order we have triadically generated beams at frequencies
$\omega _2$, while at second order we have triadically generated beams at frequencies ![]() $2\omega _1$ and
$2\omega _1$ and ![]() $\omega _2-\omega _1$. Here, we note that
$\omega _2-\omega _1$. Here, we note that ![]() $2\omega _2$ and
$2\omega _2$ and ![]() $\omega _2+\omega _1$ are evanescent disturbances that contribute to the second-order field, but do not manifest as wave beams.
$\omega _2+\omega _1$ are evanescent disturbances that contribute to the second-order field, but do not manifest as wave beams.
Table 2. Parameters for each configuration considered, as defined by (5.7).

Our priority is to examine wave–wave interactions, and while there are many in this figure, the principal interaction zone is outlined by the grey box. Since this will be our region of focus for subsequent results, we take care to optimise the wave field geometry for diagnostic quality in this region. For our prediction to match well, we account for experimental artefacts such as weak viscous spreading of wave beams and some unavoidable curvature in the stratification near the top and bottom boundaries, so we make small perturbations to waves generated on the synthetic wave maker to ensure that waves incident to the boxed region have beam widths and amplitudes that match the experiment. Given comprehensive frequency-decomposed post-processing of experiments, we are able to perform a thorough calibration of the transmission efficiency from order to order. We find by line-search optimisation that it takes a globally constant value of ![]() $\sim \frac {1}{2}$ across all interactions and all experiments. It remains an open question why the perturbation expansion requires such order-to-order calibration, but by matching our hierarchical decomposition with suitably post-processed experiments, we have identified a discrepancy that could not have been anticipated in advance.
$\sim \frac {1}{2}$ across all interactions and all experiments. It remains an open question why the perturbation expansion requires such order-to-order calibration, but by matching our hierarchical decomposition with suitably post-processed experiments, we have identified a discrepancy that could not have been anticipated in advance.
In the primary interaction zone, two significant new waves are emitted up and to the right: one at second order (shown in red in figure 11c) of frequency ![]() $\omega _2 - \omega _1$ due to the interaction of beam
$\omega _2 - \omega _1$ due to the interaction of beam ![]() $2$ with beam
$2$ with beam ![]() $1$, and the other at third order (shown in green) of frequency
$1$, and the other at third order (shown in green) of frequency ![]() $\omega _2 - 2 \omega _1$ due to the interaction of the first additional wave with beam
$\omega _2 - 2 \omega _1$ due to the interaction of the first additional wave with beam ![]() $2$. Where the reflections of both beams
$2$. Where the reflections of both beams ![]() $1$ and
$1$ and ![]() $2$ intersect, we note an interference pattern leads to a distortion of the phase lines in the bottom-right corner of the grey box.
$2$ intersect, we note an interference pattern leads to a distortion of the phase lines in the bottom-right corner of the grey box.
The left-hand end of the magic carpet, just outside our diagnostic field of view in the experiment (and replicated in the numerical prediction), also emits a second harmonic for beam ![]() $1$, which reflects off the free surface before interacting with its fundamental beam. From this interaction, an additional wave of frequency
$1$, which reflects off the free surface before interacting with its fundamental beam. From this interaction, an additional wave of frequency ![]() $\omega _1$ (shown in green in figure 11c) is emitted though here its direction is down and to the right. This beam reflects off the bottom boundary and also happens to intersect the primary interaction zone. Since the second harmonic is present only at second order (Dobra et al. Reference Dobra, Lawrie and Dalziel2021), this additional
$\omega _1$ (shown in green in figure 11c) is emitted though here its direction is down and to the right. This beam reflects off the bottom boundary and also happens to intersect the primary interaction zone. Since the second harmonic is present only at second order (Dobra et al. Reference Dobra, Lawrie and Dalziel2021), this additional ![]() $\omega _1$ beam is third-order, so for a prediction truncated at third order, we do not include any of its interactions with other wave beams. Also visible in the experiment is a fourth-order zero-frequency wave (shown in orange) arising from the interaction of the first- and third-order waves of frequency
$\omega _1$ beam is third-order, so for a prediction truncated at third order, we do not include any of its interactions with other wave beams. Also visible in the experiment is a fourth-order zero-frequency wave (shown in orange) arising from the interaction of the first- and third-order waves of frequency ![]() $\omega _1$. While strictly zero-frequency modes have no phase propagation, they closely resemble gravity currents generated by transient irreversible displacement of mass. Indeed, these also form near the bottom boundary, and we attribute this small aberration to boundary-layer mixing.
$\omega _1$. While strictly zero-frequency modes have no phase propagation, they closely resemble gravity currents generated by transient irreversible displacement of mass. Indeed, these also form near the bottom boundary, and we attribute this small aberration to boundary-layer mixing.
On its third reflection, the second harmonic of ![]() $\omega _1$ intersects its fundamental once more, this time just after its own reflection off the free surface. We calibrate the strength of reflections in our predictions to account for surface wave transmission away from reflection sites, and we find an absorption coefficient of 30 %. Furthermore, evaporative cooling acts to smooth the top interface, which in turn creates complex reflection geometries; we account for these by applying a phase shift and a higher absorption coefficient of 55 % to beam
$\omega _1$ intersects its fundamental once more, this time just after its own reflection off the free surface. We calibrate the strength of reflections in our predictions to account for surface wave transmission away from reflection sites, and we find an absorption coefficient of 30 %. Furthermore, evaporative cooling acts to smooth the top interface, which in turn creates complex reflection geometries; we account for these by applying a phase shift and a higher absorption coefficient of 55 % to beam ![]() $1$ only.
$1$ only.
In figure 12 for a similar configuration, we expand out all the wave contributions at each order and frequency, and compare with the DMD of the experiment. We restrict the viewing window to the grey box in figure 11. The first row contains the superposition of all the wave beams at each order of truncation. At first order, there are no interactions, so we have only the linear superposition of incident waves. At second order, we obtain by triadic interactions a new pair of frequencies, ![]() $\omega _2 - \omega _1$ and
$\omega _2 - \omega _1$ and ![]() $\omega _2 + \omega _1$.
$\omega _2 + \omega _1$.

Figure 12. Hierarchical decomposition of the internal wave field where ![]() $\omega _1 \approx 0.37N$ and
$\omega _1 \approx 0.37N$ and ![]() $\omega _2 = 2.2 \omega _1$. We plot the real part of evolutionary modes of the diagnostic,
$\omega _2 = 2.2 \omega _1$. We plot the real part of evolutionary modes of the diagnostic, ![]() $( 1 / \rho _{00} ) \partial \rho ' / \partial z$, and for reference mark the parallelogram where the incident waves cross with dotted lines. At first order in the expansion, we only obtain the incident waves. At second order, we obtain
$( 1 / \rho _{00} ) \partial \rho ' / \partial z$, and for reference mark the parallelogram where the incident waves cross with dotted lines. At first order in the expansion, we only obtain the incident waves. At second order, we obtain ![]() $\omega _2 - \omega _1$ and
$\omega _2 - \omega _1$ and ![]() $\omega _2 + \omega _1$. At third order, we not only obtain four new frequencies, but we obtain new contributions to
$\omega _2 + \omega _1$. At third order, we not only obtain four new frequencies, but we obtain new contributions to ![]() $\omega _1$ and
$\omega _1$ and ![]() $\omega _2$ that in this configuration are small in amplitude but broaden the wave beams. There are no further contributions to
$\omega _2$ that in this configuration are small in amplitude but broaden the wave beams. There are no further contributions to ![]() $\omega _2 - \omega _1$ and
$\omega _2 - \omega _1$ and ![]() $\omega _2 + \omega _1$ until fourth order. The final column compares with DMD, and the dashed grey boxes indicate where experimental noise obscured the frequency from detection. We do not constrain the DMD to deliver prescribed frequencies; the best-fit modes are always returned.
$\omega _2 + \omega _1$ until fourth order. The final column compares with DMD, and the dashed grey boxes indicate where experimental noise obscured the frequency from detection. We do not constrain the DMD to deliver prescribed frequencies; the best-fit modes are always returned.
Eight triads are possible at third order, formed from each combination of a first-order and a second-order wave, and in each combination both difference and sum of frequencies may emerge. Four of these triads produce new frequencies, meanwhile there is a pair of triads from which will emerge new contributions to ![]() $\omega _1$ and a corresponding pair for
$\omega _1$ and a corresponding pair for ![]() $\omega _2$. The triads for
$\omega _2$. The triads for ![]() $\omega _1$ are
$\omega _1$ are ![]() $- \left ( \omega _2 - \omega _1 \right ) + \omega _2$ and
$- \left ( \omega _2 - \omega _1 \right ) + \omega _2$ and ![]() $\left ( \omega _2 + \omega _1 \right ) - \omega _2$, and for
$\left ( \omega _2 + \omega _1 \right ) - \omega _2$, and for ![]() $\omega _2$, they are
$\omega _2$, they are ![]() $\left ( \omega _2 - \omega _1 \right ) + \omega _1$ and
$\left ( \omega _2 - \omega _1 \right ) + \omega _1$ and ![]() $\left ( \omega _2 + \omega _1 \right ) - \omega _1$. For the configuration in this figure, these contributions are present but very weak and must not be confused with the third-order
$\left ( \omega _2 + \omega _1 \right ) - \omega _1$. For the configuration in this figure, these contributions are present but very weak and must not be confused with the third-order ![]() $\omega _1$ wave in the bottom-left that, similarly to figure 11, arises from the interaction of
$\omega _1$ wave in the bottom-left that, similarly to figure 11, arises from the interaction of ![]() $2 \omega _1$ and
$2 \omega _1$ and ![]() $\omega _1$ well to the left of the viewing window; we verified the wave direction in the experiment using the Hilbert transform. We also note that at third order, there are neither contributions to
$\omega _1$ well to the left of the viewing window; we verified the wave direction in the experiment using the Hilbert transform. We also note that at third order, there are neither contributions to ![]() $\omega _2 - \omega _1$ nor
$\omega _2 - \omega _1$ nor ![]() $\omega _2 + \omega _1$; such additional contributions only appear from fourth order onwards.
$\omega _2 + \omega _1$; such additional contributions only appear from fourth order onwards.
Of the new frequencies generated at third order, only ![]() $\omega _2 - 2 \omega _1$ has appreciable amplitude. This propagating wave bends on the boundary of the interaction zone, because dominant wavevectors in the source terms do not satisfy the dispersion relation so the associated modes are confined as forced oscillations, meanwhile the slightly weaker resonant modes are preferentially selected and propagate away from the interaction zone. We also note other artefacts visible in both the experiment and the prediction at this frequency. The other three new frequencies are evanescent and are too weak to have significant singular values when computing the DMD, so we represent these missing modes by boxes with dashed borders.
$\omega _2 - 2 \omega _1$ has appreciable amplitude. This propagating wave bends on the boundary of the interaction zone, because dominant wavevectors in the source terms do not satisfy the dispersion relation so the associated modes are confined as forced oscillations, meanwhile the slightly weaker resonant modes are preferentially selected and propagate away from the interaction zone. We also note other artefacts visible in both the experiment and the prediction at this frequency. The other three new frequencies are evanescent and are too weak to have significant singular values when computing the DMD, so we represent these missing modes by boxes with dashed borders.
As noted by Bourget et al. (Reference Bourget, Scolan, Dauxois, Le Bars, Odier and Joubaud2014), the amplitudes of new propagating waves, such as ![]() $\omega _2 - \omega _1$, grow linearly across the interaction zone where the source terms are large. Outside this zone, propagating over an area with insignificant sources, they have approximately constant amplitude. Conversely, forced oscillations have amplitudes that are proportional to the local source terms. In this example,
$\omega _2 - \omega _1$, grow linearly across the interaction zone where the source terms are large. Outside this zone, propagating over an area with insignificant sources, they have approximately constant amplitude. Conversely, forced oscillations have amplitudes that are proportional to the local source terms. In this example, ![]() $\omega _2 + \omega _1 > N$ and produces weak evanescent modes that decay exponentially with distance from the interaction zone, so we amplify its images by a factor of ten. We see a second generation zone of this mode where the reflection of
$\omega _2 + \omega _1 > N$ and produces weak evanescent modes that decay exponentially with distance from the interaction zone, so we amplify its images by a factor of ten. We see a second generation zone of this mode where the reflection of ![]() $\omega _2$ intersects
$\omega _2$ intersects ![]() $\omega _1$ again in the bottom-right of the domain.
$\omega _1$ again in the bottom-right of the domain.
Figure 13 is similar to the previous two cases, but configured such that ![]() $\omega _2 + \omega _1 < N$. This mode is emitted to the right both upwards and downwards, but the upwards-propagating mode is stronger and noticeably reflects several times within our field of view, thus the configuration is dense with opportunities for third-order interactions in the right half of the image. One such interaction is the broad addition to
$\omega _2 + \omega _1 < N$. This mode is emitted to the right both upwards and downwards, but the upwards-propagating mode is stronger and noticeably reflects several times within our field of view, thus the configuration is dense with opportunities for third-order interactions in the right half of the image. One such interaction is the broad addition to ![]() $\omega _2$ in the bottom-right corner, and there is a corresponding beam at
$\omega _2$ in the bottom-right corner, and there is a corresponding beam at ![]() $\omega _1$ in the top-right corner.
$\omega _1$ in the top-right corner.

Figure 13. Hierarchical decomposition of the internal wave field where ![]() $\omega _1 \approx 0.37N$ and
$\omega _1 \approx 0.37N$ and ![]() $\omega _2 = 1.5 \omega _1$. We plot the real part of evolutionary modes of the diagnostic,
$\omega _2 = 1.5 \omega _1$. We plot the real part of evolutionary modes of the diagnostic, ![]() $( 1 / \rho _{00} ) \partial \rho ' / \partial z$, and for reference mark the parallelogram where the incident waves cross with dotted lines. Here,
$( 1 / \rho _{00} ) \partial \rho ' / \partial z$, and for reference mark the parallelogram where the incident waves cross with dotted lines. Here, ![]() $\omega _2 + \omega _1 < N$, so new waves can be emitted. This corresponds to the geometry presented in figure 10, and we see that these waves are emitted in two directions. In this case, several frequencies are duplicated by contributions from multiple sources; in particular,
$\omega _2 + \omega _1 < N$, so new waves can be emitted. This corresponds to the geometry presented in figure 10, and we see that these waves are emitted in two directions. In this case, several frequencies are duplicated by contributions from multiple sources; in particular, ![]() $\left | \omega _2 - 2 \omega _1 \right | = \left | \omega _2 - \omega _1 \right |$. Other duplicates arise from the second harmonic of beam
$\left | \omega _2 - 2 \omega _1 \right | = \left | \omega _2 - \omega _1 \right |$. Other duplicates arise from the second harmonic of beam ![]() $1$, which first appears at second order and just misses the main interaction zone. The final column compares with DMD, and the dashed grey boxes indicate where experimental noise obscured the frequency from detection.
$1$, which first appears at second order and just misses the main interaction zone. The final column compares with DMD, and the dashed grey boxes indicate where experimental noise obscured the frequency from detection.
Due to our choice here of ![]() $\omega _2 = 1.5 \omega _1$, some frequencies are duplicated by multiple modes. In particular,
$\omega _2 = 1.5 \omega _1$, some frequencies are duplicated by multiple modes. In particular, ![]() $\left | \omega _2 - 2 \omega _1 \right | = \omega _2 - \omega _1$, but geometrically the wavevectors cannot organise to form a contribution from
$\left | \omega _2 - 2 \omega _1 \right | = \omega _2 - \omega _1$, but geometrically the wavevectors cannot organise to form a contribution from ![]() $\omega _2 - 2 \omega _1$, consistent with the selection rules of Tabaei et al. (Reference Tabaei, Akylas and Lamb2005) and Jiang & Marcus (Reference Jiang and Marcus2009). In addition, a reflection of the second harmonic of beam
$\omega _2 - 2 \omega _1$, consistent with the selection rules of Tabaei et al. (Reference Tabaei, Akylas and Lamb2005) and Jiang & Marcus (Reference Jiang and Marcus2009). In addition, a reflection of the second harmonic of beam ![]() $1$ passes close to the interaction zone, and its interaction with beam
$1$ passes close to the interaction zone, and its interaction with beam ![]() $2$ near the left vertex of the main interaction zone also produces two waves at
$2$ near the left vertex of the main interaction zone also produces two waves at ![]() $\omega _2 - \omega _1$, which propagate in each of the downward directions. Although the dominant components of
$\omega _2 - \omega _1$, which propagate in each of the downward directions. Although the dominant components of ![]() $2 \omega _1$ and
$2 \omega _1$ and ![]() $\omega _1$ have a common horizontal phase velocity and thus have a symmetry that prevents them from interacting (Dobra et al. Reference Dobra, Lawrie and Dalziel2021), each wave beam is monochromatic in frequency but has a broad wavenumber spectrum, so provided we satisfy the geometric selection rules, a full spectrum of resonant modes will still be generated. Moreover,
$\omega _1$ have a common horizontal phase velocity and thus have a symmetry that prevents them from interacting (Dobra et al. Reference Dobra, Lawrie and Dalziel2021), each wave beam is monochromatic in frequency but has a broad wavenumber spectrum, so provided we satisfy the geometric selection rules, a full spectrum of resonant modes will still be generated. Moreover, ![]() $2 \omega _1$ has third-order interactions with
$2 \omega _1$ has third-order interactions with ![]() $\omega _1$ and
$\omega _1$ and ![]() $\omega _2$, but the only appreciable contribution is
$\omega _2$, but the only appreciable contribution is ![]() $2 \omega _1 + \omega _1 = 3 \omega _1$. One component of this signal is a weak evanescent second harmonic of beam
$2 \omega _1 + \omega _1 = 3 \omega _1$. One component of this signal is a weak evanescent second harmonic of beam ![]() $2$, visible in the bottom-left corner, and appears here because
$2$, visible in the bottom-left corner, and appears here because ![]() $2 \omega _2 = 3 \omega _1$ by construction. However, the dominant signal in the DMD mode at
$2 \omega _2 = 3 \omega _1$ by construction. However, the dominant signal in the DMD mode at ![]() $3 \omega _1$ is a forced oscillation associated with beam
$3 \omega _1$ is a forced oscillation associated with beam ![]() $2$, and we do not consider this mechanism in our model, so a direct comparison cannot easily be drawn.
$2$, and we do not consider this mechanism in our model, so a direct comparison cannot easily be drawn.
In numerous places, our experiment demonstrates the presence of yet higher-order interactions. Firstly, in the bottom-right corner of the ![]() $\omega _2 - \omega _1$ panel, there is a broadening of this wave beam that appears analogous to the previously noted third-order contributions to
$\omega _2 - \omega _1$ panel, there is a broadening of this wave beam that appears analogous to the previously noted third-order contributions to ![]() $\omega _1$ and
$\omega _1$ and ![]() $\omega _2$. This contribution may be generated by a fourth-order interaction between
$\omega _2$. This contribution may be generated by a fourth-order interaction between ![]() $\omega _1$ and the
$\omega _1$ and the ![]() $\omega _2 - \omega _1$ component that is itself generated by the second harmonic,
$\omega _2 - \omega _1$ component that is itself generated by the second harmonic, ![]() $2 \omega _1$, and its fundamental,
$2 \omega _1$, and its fundamental, ![]() $\omega _1$. Secondly, the DMD at
$\omega _1$. Secondly, the DMD at ![]() $2 \omega _2 - \omega _1$ exhibits waves originating in the main interaction zone. We do not predict them at third order, but do expect to find them at higher orders. Although our prediction of their amplitudes is poor, we do capture elements of their structure. We also note that in the top-right corner, we have successfully predicted the third-order interaction
$2 \omega _2 - \omega _1$ exhibits waves originating in the main interaction zone. We do not predict them at third order, but do expect to find them at higher orders. Although our prediction of their amplitudes is poor, we do capture elements of their structure. We also note that in the top-right corner, we have successfully predicted the third-order interaction ![]() $\left ( \omega _2 - \omega _1 \right ) + \omega _2$.
$\left ( \omega _2 - \omega _1 \right ) + \omega _2$.
In the following figures, we select some interesting alternative geometries. Figure 14 considers the interaction of left- and right-running waves, and figure 15 considers incident waves from the same quadrant that interact obliquely.

Figure 14. Decomposition of the internal wave field where ![]() $\omega _1 \approx 0.28N$ and
$\omega _1 \approx 0.28N$ and ![]() $\omega _2 = 3 \omega _1$. We plot the real part of evolutionary modes of the diagnostic,
$\omega _2 = 3 \omega _1$. We plot the real part of evolutionary modes of the diagnostic, ![]() $( 1 / \rho _{00} ) \partial \rho ' / \partial z$, and for reference mark the parallelogram where the incident waves cross with dotted lines. We notice in particular distortion of phase lines of
$( 1 / \rho _{00} ) \partial \rho ' / \partial z$, and for reference mark the parallelogram where the incident waves cross with dotted lines. We notice in particular distortion of phase lines of ![]() $\omega _1$ due to third-order contributions. For completeness, we include the second harmonic of
$\omega _1$ due to third-order contributions. For completeness, we include the second harmonic of ![]() $\omega _1$, since this has the same frequency as
$\omega _1$, since this has the same frequency as ![]() $\omega _2 - \omega _1$.
$\omega _2 - \omega _1$.

Figure 15. Decomposition of the internal wave field where ![]() $\omega _1 \approx 0.54N$ and
$\omega _1 \approx 0.54N$ and ![]() $\omega _2 = 1.5 \omega _1$. We plot the real part of evolutionary modes of the diagnostic,
$\omega _2 = 1.5 \omega _1$. We plot the real part of evolutionary modes of the diagnostic, ![]() $( 1 / \rho _{00} ) \partial \rho ' / \partial z$, and for reference mark the parallelogram where the incident waves cross with dotted lines. We observe that beams
$( 1 / \rho _{00} ) \partial \rho ' / \partial z$, and for reference mark the parallelogram where the incident waves cross with dotted lines. We observe that beams ![]() $1$ and
$1$ and ![]() $2$ exhibit broadening at third order.
$2$ exhibit broadening at third order.
With waves in opposite horizontal directions, we have the opportunity to maximise the interaction strength by choice of frequencies. The source terms arise from the ![]() $\boldsymbol {u} \boldsymbol {\cdot } \boldsymbol {\nabla }$ advection operators in the governing equations (2.1) and (2.3). The velocity,
$\boldsymbol {u} \boldsymbol {\cdot } \boldsymbol {\nabla }$ advection operators in the governing equations (2.1) and (2.3). The velocity, ![]() $\boldsymbol {u}$, points along the wave beam, meanwhile all gradients are perpendicular to the beam. In the case where the two beams are orthogonal,
$\boldsymbol {u}$, points along the wave beam, meanwhile all gradients are perpendicular to the beam. In the case where the two beams are orthogonal, ![]() $\boldsymbol {u}$ of one beam is aligned with the gradient vector of the other, and thus the source terms will be maximal. In figure 14, we demonstrate a near-orthogonal configuration with the additional property that the dominant
$\boldsymbol {u}$ of one beam is aligned with the gradient vector of the other, and thus the source terms will be maximal. In figure 14, we demonstrate a near-orthogonal configuration with the additional property that the dominant ![]() $\boldsymbol {k}_2 - \boldsymbol {k}_1$ is near-resonant.
$\boldsymbol {k}_2 - \boldsymbol {k}_1$ is near-resonant.
Due to these strong interactions at second order, we have a clear view of the third-order contributions to ![]() $\omega _1$. These broaden the beam significantly, introduce a distortion of the phase and slightly increase the amplitude. In addition, the second harmonic of beam
$\omega _1$. These broaden the beam significantly, introduce a distortion of the phase and slightly increase the amplitude. In addition, the second harmonic of beam ![]() $1$ has frequency
$1$ has frequency ![]() $\omega _2 - \omega _1$ and appears in the top-left corner, which interacts with its fundamental beam to produce third-order forced oscillations at
$\omega _2 - \omega _1$ and appears in the top-left corner, which interacts with its fundamental beam to produce third-order forced oscillations at ![]() $\omega _1$ whose wavevectors do not align with those of beam
$\omega _1$ whose wavevectors do not align with those of beam ![]() $1$. Of the remaining contributions to
$1$. Of the remaining contributions to ![]() $\omega _1$, we distinguish between the following permutations: the standard pairings of
$\omega _1$, we distinguish between the following permutations: the standard pairings of ![]() $- \left ( \omega _2 - \omega _1 \right ) + \omega _2$ and
$- \left ( \omega _2 - \omega _1 \right ) + \omega _2$ and ![]() $\left ( \omega _2 + \omega _1 \right ) - \omega _2$, and an additional possible interaction,
$\left ( \omega _2 + \omega _1 \right ) - \omega _2$, and an additional possible interaction, ![]() $\left ( \omega _2 - \omega _1 \right ) - \omega _1$, whose frequency coincides with
$\left ( \omega _2 - \omega _1 \right ) - \omega _1$, whose frequency coincides with ![]() $\omega _1$ in this configuration. It turns out that the additional interaction produces a wave that propagates down and to the left, whereas the standard pairings produce waves that propagate in the same direction as beam
$\omega _1$ in this configuration. It turns out that the additional interaction produces a wave that propagates down and to the left, whereas the standard pairings produce waves that propagate in the same direction as beam ![]() $1$ and are responsible for broadening the beam. Given this clarity, we revisit the
$1$ and are responsible for broadening the beam. Given this clarity, we revisit the ![]() $\omega _1$ and
$\omega _1$ and ![]() $\omega _2$ panels in figures 12 and 13, and we note that the DMD shows clear distortion of
$\omega _2$ panels in figures 12 and 13, and we note that the DMD shows clear distortion of ![]() $\omega _2$. Although we underpredict the additional
$\omega _2$. Although we underpredict the additional ![]() $\omega _2$ contribution, further numerical investigations have confirmed that this third-order contribution is primarily responsible for the observed distortion of phase. Other less significant factors are due to slight curvature of the stratification, which causes waves to refract.
$\omega _2$ contribution, further numerical investigations have confirmed that this third-order contribution is primarily responsible for the observed distortion of phase. Other less significant factors are due to slight curvature of the stratification, which causes waves to refract.
Returning to figure 14, at third order in ![]() $\omega _2$, a weak wave is emitted down and to the left, which we have determined from source terms must arise from the interaction
$\omega _2$, a weak wave is emitted down and to the left, which we have determined from source terms must arise from the interaction ![]() $\left ( \omega _2 - \omega _1 \right ) + \omega _1$. Furthermore, the
$\left ( \omega _2 - \omega _1 \right ) + \omega _1$. Furthermore, the ![]() $\omega _2 - \omega _1$ beam is broader than the main interaction zone in a manner analogous to the broadening of
$\omega _2 - \omega _1$ beam is broader than the main interaction zone in a manner analogous to the broadening of ![]() $\omega _1$, and we attribute this to higher-order contributions.
$\omega _1$, and we attribute this to higher-order contributions.
It is of interest that the wavevector of the signal in the top-right of the ![]() $\omega _2 - \omega _1$ experimental image is not aligned with the direction given by the dispersion relation, so we conclude that these are forced oscillations. Since no other wave beams intersect beam
$\omega _2 - \omega _1$ experimental image is not aligned with the direction given by the dispersion relation, so we conclude that these are forced oscillations. Since no other wave beams intersect beam ![]() $1$ in this region, we deduce that these forced oscillations must be driven by the interaction of beam
$1$ in this region, we deduce that these forced oscillations must be driven by the interaction of beam ![]() $1$ with itself, but a single inviscid wave cannot self-interact because its gradients are strictly normal to its velocities. If a process, such as viscous spreading of the wave beam, were to cause the direction of some wavevectors to vary, triadic interactions would then be possible. We consider the sum of two modulated modes. Should the variations in direction be small, the wavevectors of the sources must point approximately in the direction of
$1$ with itself, but a single inviscid wave cannot self-interact because its gradients are strictly normal to its velocities. If a process, such as viscous spreading of the wave beam, were to cause the direction of some wavevectors to vary, triadic interactions would then be possible. We consider the sum of two modulated modes. Should the variations in direction be small, the wavevectors of the sources must point approximately in the direction of ![]() $2 \boldsymbol {k}_1$, and thus these wavevectors would be narrowly distributed about the resonant locus at the fundamental frequency,
$2 \boldsymbol {k}_1$, and thus these wavevectors would be narrowly distributed about the resonant locus at the fundamental frequency, ![]() $\omega _1$, represented by a straight line through the origin. These wavevectors would not intersect the resonant locus of the second harmonic, which is also a straight line through the origin but has steeper gradient, and thus no propagating waves would be emitted at
$\omega _1$, represented by a straight line through the origin. These wavevectors would not intersect the resonant locus of the second harmonic, which is also a straight line through the origin but has steeper gradient, and thus no propagating waves would be emitted at ![]() $2 \omega _1$. We hypothesise that such viscous mechanisms are responsible for these features, and in general, these are likely to be strongest close to the magic carpet. Indeed, in the
$2 \omega _1$. We hypothesise that such viscous mechanisms are responsible for these features, and in general, these are likely to be strongest close to the magic carpet. Indeed, in the ![]() $3 \omega _1$ DMD mode of figure 13, we notice the same feature and attribute viscous action to its appearance.
$3 \omega _1$ DMD mode of figure 13, we notice the same feature and attribute viscous action to its appearance.
Figure 15 shows an oblique interaction where the ![]() $\omega _2 - \omega _1$ beam is emitted back into the same quadrant from which the incident waves originate. Once again, the interactions are strong, and we successfully capture third-order beam-broadening contributions to both
$\omega _2 - \omega _1$ beam is emitted back into the same quadrant from which the incident waves originate. Once again, the interactions are strong, and we successfully capture third-order beam-broadening contributions to both ![]() $\omega _1$ and
$\omega _1$ and ![]() $\omega _2$. Furthermore, we find shifts in phase to the left of the main interaction zone at both
$\omega _2$. Furthermore, we find shifts in phase to the left of the main interaction zone at both ![]() $\omega _2$ and
$\omega _2$ and ![]() $\omega _2 - \omega _1$, and a propagating beam down and to the right at
$\omega _2 - \omega _1$, and a propagating beam down and to the right at ![]() $\omega _2 - \omega _1$. This frequency includes both second- and third-order effects because again
$\omega _2 - \omega _1$. This frequency includes both second- and third-order effects because again ![]() $\omega _2 = 1.5 \omega _1$. In the top-left of the DMD mode at this frequency, there is another weak wave that we attribute to a higher-order interaction. Finally, we remark that in this experiment, it turned out that there was a smooth, weak variation in buoyancy frequency from top to bottom.
$\omega _2 = 1.5 \omega _1$. In the top-left of the DMD mode at this frequency, there is another weak wave that we attribute to a higher-order interaction. Finally, we remark that in this experiment, it turned out that there was a smooth, weak variation in buoyancy frequency from top to bottom.
6. Conclusions
We have developed a robust hierarchically organised prediction tool for arbitrarily complex two-dimensional internal wave systems and contend that this is a necessary and sufficient model for determining the structure of wave–wave interactions near the inviscid limit. In this work, we introduce for the first time the fusion of a weakly nonlinear perturbation expansion with a semi-analytical implementation of the monochromatic free-space Green's function for the governing equation. Our method has indeed been shown to accurately recover the structure of wave–wave interactions, showing a remarkable level of agreement between our experiments and our method. Having carefully validated our approach using frequency-decomposed post-processing, we have now been able to identify wave–wave interactions up to third order by direct comparison and infer the origins of other features observed in experiments that must arise from higher orders or from secondary effects. This unparalleled access to individual components and isolation of interaction behaviour provides clarity to the mechanisms in the system, and we have attempted to explain with reference to the weakly nonlinear perturbation expansion previously unnoticed physical features, such as forced oscillations that share a frequency with other waves but do not satisfy the dispersion relation for a wave to form. Furthermore, we have strong evidence of a previously undiscovered open question regarding the order-to-order transmission efficiency of wave–wave interactions.
As necessitated by our choice of experimental validation, we have already generalised our approach to aperiodic configurations and arbitrary time dependence. With careful consideration of causality, we have also provided our calculations for a range of boundary conditions for two field potentials so that our free-space source implementation is suitable for bounded flows and, in particular, for our case that includes a flexible boundary. We have configured our implementation to be minimally elaborate while remaining causal.
We remark that there is no particular restriction to systems of internal waves. Our hierarchical decomposition is equally valid for any system for which a Green's function may be obtained. These include gravito-inertial systems, Rossby waves and some aspects of nonlinear acoustics. Further generalisations we envisage could include solving the linear inverse problem to determine suitable source strengths equivalent to a boundary displacement computed from data observed at a distance. Our experimental and numerical study has already led us to new insights on specific systems, and we anticipate the approach will be adopted for a much broader range of problems in the imminent future.
Declaration of interests
The authors report no conflict of interest.
Appendix A. Derivation of monochromatic Green's function
Repurposing the method of Hurley (Reference Hurley1972, Reference Hurley1997), we first calculate the Green's function for evanescent oscillations at ![]() $\omega > N$, then analytically continue it to other values of
$\omega > N$, then analytically continue it to other values of ![]() $\omega$. Defining the transformed coordinates to perform a dilatation,
$\omega$. Defining the transformed coordinates to perform a dilatation,
 \begin{equation} \begin{bmatrix} x \\ z \end{bmatrix} \mapsto \begin{bmatrix} x_{\alpha} \\ z_{\alpha} \end{bmatrix} = \begin{bmatrix} \dfrac{x}{\varGamma} \\ z \end{bmatrix} , \end{equation}
\begin{equation} \begin{bmatrix} x \\ z \end{bmatrix} \mapsto \begin{bmatrix} x_{\alpha} \\ z_{\alpha} \end{bmatrix} = \begin{bmatrix} \dfrac{x}{\varGamma} \\ z \end{bmatrix} , \end{equation}
the point-forced internal wave equation (3.3) becomes Poisson's equation in the new coordinate system for ![]() $\omega > N$,
$\omega > N$,
 \begin{equation} \frac{\partial^{2} G_{\omega}}{\partial x_{\alpha}^{2}} + \frac{\partial^{2} G_{\omega}}{\partial z_{\alpha}^{2}} ={-} \frac{\delta \left( x_{\alpha} - \dfrac{x_0}{\varGamma} \right) \delta \left( z_{\alpha} - z_0 \right)}{\varGamma \omega^{2}} . \end{equation}
\begin{equation} \frac{\partial^{2} G_{\omega}}{\partial x_{\alpha}^{2}} + \frac{\partial^{2} G_{\omega}}{\partial z_{\alpha}^{2}} ={-} \frac{\delta \left( x_{\alpha} - \dfrac{x_0}{\varGamma} \right) \delta \left( z_{\alpha} - z_0 \right)}{\varGamma \omega^{2}} . \end{equation}
Thus, ![]() $G_{\omega }$ is proportional to the corresponding free-space Green's function,
$G_{\omega }$ is proportional to the corresponding free-space Green's function,
where ![]() $r^{2} = \left ( x - x_0 \right )^{2} / ( 1 - \left ( N / \omega \right )^{2} ) + \left ( z - z_0 \right )^{2}$ and
$r^{2} = \left ( x - x_0 \right )^{2} / ( 1 - \left ( N / \omega \right )^{2} ) + \left ( z - z_0 \right )^{2}$ and ![]() $C$ is the integration constant, which is a gauge freedom that we take to be zero.
$C$ is the integration constant, which is a gauge freedom that we take to be zero.
We now extend ![]() $G_{\omega }$ to be valid at all frequencies using analytic continuation in complex
$G_{\omega }$ to be valid at all frequencies using analytic continuation in complex ![]() $\omega$ space. The logarithm has branch points at
$\omega$ space. The logarithm has branch points at ![]() $r^{2} = \left \{ 0, \infty \right \}$, which rearranges to
$r^{2} = \left \{ 0, \infty \right \}$, which rearranges to
 \begin{equation} 1 - \left( \frac{N}{\omega} \right)^{2} = \left\{ - \left( \frac{x - x_0}{z - z_0} \right)^{2} , 0 \right\} , \end{equation}
\begin{equation} 1 - \left( \frac{N}{\omega} \right)^{2} = \left\{ - \left( \frac{x - x_0}{z - z_0} \right)^{2} , 0 \right\} , \end{equation}and thus the logarithm has four branch points,
 \begin{equation} \omega = \left\{{\pm} \frac{N}{\sqrt{1 + \left( \dfrac{x - x_0}{z - z_0} \right)^{2}}} , \pm N \right\} . \end{equation}
\begin{equation} \omega = \left\{{\pm} \frac{N}{\sqrt{1 + \left( \dfrac{x - x_0}{z - z_0} \right)^{2}}} , \pm N \right\} . \end{equation}
In addition, ![]() $1 / \varGamma$ has three branch points,
$1 / \varGamma$ has three branch points, ![]() $\omega = \left \{ 0, \pm N \right \}$, so, in total, there are five distinct branch points, as marked in figure 16. Those at
$\omega = \left \{ 0, \pm N \right \}$, so, in total, there are five distinct branch points, as marked in figure 16. Those at ![]() $\omega = \pm N$ correspond to the regime change from evanescent to propagating internal waves. The branch points all need joining with branch cuts, which we chose carefully to provide physically realisable conditions. Since any steady-state internal wave must have grown from a stationary ambient at some time in the past, we assume that the wave is in fact growing exponentially slowly in time and so
$\omega = \pm N$ correspond to the regime change from evanescent to propagating internal waves. The branch points all need joining with branch cuts, which we chose carefully to provide physically realisable conditions. Since any steady-state internal wave must have grown from a stationary ambient at some time in the past, we assume that the wave is in fact growing exponentially slowly in time and so ![]() $\mbox {Im}{ \left \{ \omega \right \} } > 0$ (Lighthill Reference Lighthill1960). Thus, we deform all the branch cuts to below the real line; these are shown by the wiggly lines in the figure.
$\mbox {Im}{ \left \{ \omega \right \} } > 0$ (Lighthill Reference Lighthill1960). Thus, we deform all the branch cuts to below the real line; these are shown by the wiggly lines in the figure.

Figure 16. Branch points, shown with crosses, and branch cuts, shown with wiggly lines, for analytic continuation in ![]() $\omega$. The five branch points are all on the real axis and the branch cut must be in the lower half-plane to satisfy causality.
$\omega$. The five branch points are all on the real axis and the branch cut must be in the lower half-plane to satisfy causality.
To perform the analytic continuation, we consider the complex arguments of the square root in ![]() $\varGamma$ and of the logarithm. For complex
$\varGamma$ and of the logarithm. For complex ![]() $\omega = \omega _r + \mathrm {i} \epsilon$ with real part
$\omega = \omega _r + \mathrm {i} \epsilon$ with real part ![]() $\omega _r$ and a small imaginary part
$\omega _r$ and a small imaginary part ![]() $\epsilon$, we make the expansion
$\epsilon$, we make the expansion
 \begin{equation} \frac{1}{\varGamma^{2}} = \frac{1}{1 - \left( \dfrac{N}{\omega_r + \mathrm{i} \epsilon} \right)^{2}} = \frac{\omega_r^{2} \left( \omega_r^{2} - N^{2} \right) + \left( 2 \omega_r^{2} + N^{2} \right) \epsilon^{2} + \epsilon^{4} - 2 \mathrm{i} N^{2} \omega_r \epsilon}{\left( \omega_r^{2} - N^{2} - \epsilon^{2} \right)^{2} + 4 \omega_r^{2} \epsilon^{2}} . \end{equation}
\begin{equation} \frac{1}{\varGamma^{2}} = \frac{1}{1 - \left( \dfrac{N}{\omega_r + \mathrm{i} \epsilon} \right)^{2}} = \frac{\omega_r^{2} \left( \omega_r^{2} - N^{2} \right) + \left( 2 \omega_r^{2} + N^{2} \right) \epsilon^{2} + \epsilon^{4} - 2 \mathrm{i} N^{2} \omega_r \epsilon}{\left( \omega_r^{2} - N^{2} - \epsilon^{2} \right)^{2} + 4 \omega_r^{2} \epsilon^{2}} . \end{equation}
The denominator is always positive and ![]() $\epsilon \geq 0$, so
$\epsilon \geq 0$, so ![]() ${{\rm sgn}}{( \mbox {Im}{\{ 1 / \varGamma ^{2} \}} )} = - {{\rm sgn}}{\left ( \mbox {Re}{\left \{ \omega \right \}} \right )}$.
${{\rm sgn}}{( \mbox {Im}{\{ 1 / \varGamma ^{2} \}} )} = - {{\rm sgn}}{\left ( \mbox {Re}{\left \{ \omega \right \}} \right )}$.
When ![]() $\omega > N$ and is real, the complex argument,
$\omega > N$ and is real, the complex argument, ![]() $\arg {( r^{2} )} = 0$. On proceeding around the first branch point at
$\arg {( r^{2} )} = 0$. On proceeding around the first branch point at ![]() $\omega = N$, where
$\omega = N$, where ![]() $r^{2}$ becomes infinite,
$r^{2}$ becomes infinite, ![]() $\mbox {Im}{\{ 1 / \varGamma ^{2} \}} < 0$, so
$\mbox {Im}{\{ 1 / \varGamma ^{2} \}} < 0$, so ![]() $\mbox {Im}{\{ r^{2} \}} < 0$. Thus,
$\mbox {Im}{\{ r^{2} \}} < 0$. Thus, ![]() $\arg {( r^{2} )}$ decreases to become
$\arg {( r^{2} )}$ decreases to become ![]() $-{\rm \pi}$ for
$-{\rm \pi}$ for ![]() $N ( 1 + ( \left ( x - x_0 \right ) / \left ( z - z_0 \right ) )^{2} )^{-1/2} < \omega < N$; in other words,
$N ( 1 + ( \left ( x - x_0 \right ) / \left ( z - z_0 \right ) )^{2} )^{-1/2} < \omega < N$; in other words, ![]() $r^{2}$ increases from
$r^{2}$ increases from ![]() $-\infty$ to zero between these branch points. As
$-\infty$ to zero between these branch points. As ![]() $\omega$ decreases further, the term
$\omega$ decreases further, the term ![]() $\left ( x - x_0 \right )^{2} / ( 1 - \left ( N / \omega \right )^{2} )$ becomes less significant, such that
$\left ( x - x_0 \right )^{2} / ( 1 - \left ( N / \omega \right )^{2} )$ becomes less significant, such that ![]() $r^{2}$ becomes positive real again after the next branch point,
$r^{2}$ becomes positive real again after the next branch point, ![]() $N ( 1 + ( \left ( x - x_0 \right ) / \left ( z - z_0 \right ) )^{2} )^{-1/2}$, with its argument yet to be determined. Since
$N ( 1 + ( \left ( x - x_0 \right ) / \left ( z - z_0 \right ) )^{2} )^{-1/2}$, with its argument yet to be determined. Since ![]() $\mbox {Re}{\left \{ \omega \right \}} > 0$, we have
$\mbox {Re}{\left \{ \omega \right \}} > 0$, we have ![]() $\mbox {Im}{\{ 1 / \varGamma ^{2} \} } < 0$ and hence
$\mbox {Im}{\{ 1 / \varGamma ^{2} \} } < 0$ and hence ![]() $\mbox {Im}{\{ r^{2} \}} < 0$, so
$\mbox {Im}{\{ r^{2} \}} < 0$, so ![]() $\arg {( r^{2} )}$ increases around the branch point to become zero for
$\arg {( r^{2} )}$ increases around the branch point to become zero for ![]() $-N ( 1 + ( ( x - x_0 ) / ( z - z_0 ) )^{2} )^{-1/2} < \omega < N ( 1 + ( ( x - x_0 ) / ( z - z_0 ) )^{2} )^{-1/2}$. The frequency,
$-N ( 1 + ( ( x - x_0 ) / ( z - z_0 ) )^{2} )^{-1/2} < \omega < N ( 1 + ( ( x - x_0 ) / ( z - z_0 ) )^{2} )^{-1/2}$. The frequency, ![]() $\omega$, is now negative for the remaining two branch points of the logarithm, so the analytic continuation is in the upper half-
$\omega$, is now negative for the remaining two branch points of the logarithm, so the analytic continuation is in the upper half-![]() $\omega$ plane. Therefore,
$\omega$ plane. Therefore, ![]() $\arg {( r^{2} )} = + {\rm \pi}$ for
$\arg {( r^{2} )} = + {\rm \pi}$ for ![]() $-N < \omega < -N ( 1 + ( ( x - x_0 ) / ( z - z_0 ) )^{2} )^{-1/2}$ and zero for
$-N < \omega < -N ( 1 + ( ( x - x_0 ) / ( z - z_0 ) )^{2} )^{-1/2}$ and zero for ![]() $\omega < -N$. Thus,
$\omega < -N$. Thus, ![]() $\mbox {Re}{\{ r^{2} \}}$ exhibits even symmetry about
$\mbox {Re}{\{ r^{2} \}}$ exhibits even symmetry about ![]() $\omega = 0$ but
$\omega = 0$ but ![]() $\arg {( r^{2} )}$ has odd symmetry. The value of the logarithm can now be determined using the standard formula,
$\arg {( r^{2} )}$ has odd symmetry. The value of the logarithm can now be determined using the standard formula,
Next, we consider the three branch points of ![]() $1 / \varGamma$. For
$1 / \varGamma$. For ![]() $\omega > N$, its complex argument is zero. Proceeding round the first branch point, at
$\omega > N$, its complex argument is zero. Proceeding round the first branch point, at ![]() $\omega = N$,
$\omega = N$, ![]() $\arg {( 1 / \varGamma ^{2} )}$ decreases to
$\arg {( 1 / \varGamma ^{2} )}$ decreases to ![]() $-{\rm \pi}$, so
$-{\rm \pi}$, so ![]() $\arg {( 1 / \varGamma )} = - {\rm \pi}/ 2$ and
$\arg {( 1 / \varGamma )} = - {\rm \pi}/ 2$ and ![]() $1 / \varGamma = - \mathrm {i} ( \left ( N / \omega \right )^{2} - 1 )^{-1/2}$ for
$1 / \varGamma = - \mathrm {i} ( \left ( N / \omega \right )^{2} - 1 )^{-1/2}$ for ![]() $0 < \omega < N$. The second branch point is at
$0 < \omega < N$. The second branch point is at ![]() $\omega = 0$. When
$\omega = 0$. When ![]() $\epsilon > 0$,
$\epsilon > 0$, ![]() $\mbox {Im}{\{ 1 / \varGamma ^{2} \}} = 0$ only when
$\mbox {Im}{\{ 1 / \varGamma ^{2} \}} = 0$ only when ![]() $\mbox {Re}{\left \{ \omega \right \}} = 0$. At this point,
$\mbox {Re}{\left \{ \omega \right \}} = 0$. At this point, ![]() $\mbox {Re}{\{ 1 / \varGamma ^{2} \}} = \epsilon ^{2} / ( N^{2} + \epsilon ^{2} ) > 0$, despite being negative when
$\mbox {Re}{\{ 1 / \varGamma ^{2} \}} = \epsilon ^{2} / ( N^{2} + \epsilon ^{2} ) > 0$, despite being negative when ![]() $\omega$ is significantly away from zero. Thus, the analytic continuation path in complex
$\omega$ is significantly away from zero. Thus, the analytic continuation path in complex ![]() $1 / \varGamma ^{2}$ space goes anticlockwise around the branch point, as shown in figure 17. So,
$1 / \varGamma ^{2}$ space goes anticlockwise around the branch point, as shown in figure 17. So, ![]() $\arg {( 1 / \varGamma ^{2} )} = + {\rm \pi}$ for
$\arg {( 1 / \varGamma ^{2} )} = + {\rm \pi}$ for ![]() $-N < \omega < 0$, thus
$-N < \omega < 0$, thus ![]() $1 / \varGamma$ changes sign at
$1 / \varGamma$ changes sign at ![]() $\omega = 0$. At the final branch point,
$\omega = 0$. At the final branch point, ![]() $\omega = -N$,
$\omega = -N$, ![]() $\mbox {Im}{\{ 1 / \varGamma ^{2} \}} > 0$, so its argument decreases from
$\mbox {Im}{\{ 1 / \varGamma ^{2} \}} > 0$, so its argument decreases from ![]() $+ {\rm \pi}$ to zero.
$+ {\rm \pi}$ to zero.

Figure 17. Analytic continuation path of ![]() $1 / \varGamma ^{2}$ for decreasing
$1 / \varGamma ^{2}$ for decreasing ![]() $\omega$ around
$\omega$ around ![]() $0$. In order to satisfy causality,
$0$. In order to satisfy causality, ![]() $\mbox {Im}{\left \{ \omega \right \}} \geq 0$, so the path shown is obtained by deforming
$\mbox {Im}{\left \{ \omega \right \}} \geq 0$, so the path shown is obtained by deforming ![]() $\omega$ into the upper half-plane. The quantity
$\omega$ into the upper half-plane. The quantity ![]() $1 / \varGamma ^{2}$ is real and negative around
$1 / \varGamma ^{2}$ is real and negative around ![]() $\omega = 0$, but the argument of
$\omega = 0$, but the argument of ![]() $1 / \varGamma$ changes from
$1 / \varGamma$ changes from ![]() $-{\rm \pi}$ to
$-{\rm \pi}$ to ![]() $+{\rm \pi}$.
$+{\rm \pi}$.
The assembled Green's function for each case is listed in table 3.
Table 3. Monochromatic Green's function, including results of analytic continuation, for all cases of ![]() $\omega$. An integration constant can be added onto the Green's function, but this does not affect derived quantities such as the velocity, so without loss of generality we take it to be zero.
$\omega$. An integration constant can be added onto the Green's function, but this does not affect derived quantities such as the velocity, so without loss of generality we take it to be zero.