Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-25T16:33:31.306Z Has data issue: false hasContentIssue false
  • English
  • Français

Estimation of local error by a neural model in an inverse scattering problem

Published online by Cambridge University Press:  21 June 2005

S. Robert ,
A. Mure-Rauvaud ,
S. Thiria  and
F. Badran
S. Robert*
Affiliation:
Laboratoire Dispositifs et Instrumentation pour l'Opto-électronique et Micro-ondes, EA 3526, 23 rue du Docteur Paul Michelon, 42023 Saint-Etienne Cedex 2, France
A. Mure-Rauvaud
Affiliation:
Laboratoire Traitement du Signal et Instrumentation, UMR CNRS 5516, 10 rue Barrouin, 42000 Saint-Etienne, France
S. Thiria
Affiliation:
Laboratoire d'Océanographie Dynamique et de Climatologie, UMR CNRS 7617, 4 place Jussieu, 75005 Paris, France
F. Badran
Affiliation:
Laboratoire d'Océanographie Dynamique et de Climatologie, UMR CNRS 7617, 4 place Jussieu, 75005 Paris, France Centre de Recherche en Informatique du CNAN, 292 rue Saint Martin, 75141 Paris Cedex 03, France
*
[email protected]
Get access

Abstract

Characterization of optical gratings by resolution of inverse scattering problem has become a widely used tool. Indeed, it is known as a non-destructive, rapid and non-invasive method in opposition with microscopic characterizations. Use of a neural model is generally implemented and has shown better results by comparison with other regression methods. The neural network learns the relationship between the optical signature and the corresponding profile shape. The performance of such a non-linear regression method is usually estimated by the root mean square error calculated on a data set not involved in the training process. However, this error estimation is not very significant and tends to flatten the error in the different areas of variable space. We introduce, in this paper, the calculation of local error for each geometrical parameter representing the profile shape. For this purpose a second neural network is implemented to learn the variance of results obtained by the first one. A comparison with the root mean square error confirms a gain of local precision. Finally, the method is applied in the optical characterization of a semi-conductor grating with a 1 $\mu $ 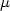m period.

Keywords

Type
Research Article
Information
The European Physical Journal - Applied Physics , Volume 31 , Issue 1 , July 2005 , pp. 71 - 76
Copyright
© EDP Sciences, 2005

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Roger, A., Maystre, D., J. Opt. Soc. Am. 70, 1483 (1980) CrossRef
Naqvi, S.S.H., Krukar, R.H., McNeil, J.R., Franke, J.E., Niemczyk, T.M., Haaland, D.M., Gottscho, R.A., Kornblit, A., J. Opt. Soc. Am. A 11, 2485 (1994) CrossRef
Drege, E.M., Reed, J.A., Byrne, D.M., Opt. Eng. 41, 225 (2002) CrossRef
Holden, J.M., Gubiotti, T. , McGaham, W.A. , Dusa, M., Kiers, T., in Metrology, Inspection, and Process Control for Microlithography XVI, edited by D.J. Herr, Proc. SPIE 4689, 1110 (2002) CrossRef
Hazart, J., Grand, G., Thony, P., Herisson, D., Garcia, S., Lartigue, O., in Process and Materials Characterization and Diagnostics in IC Manufacturing, edited by K.W. Tobin Jr., I. Emami, Proc. SPIE 5041, 9 (2003) CrossRef
Bischoff, J. , Truckenbrodt, H., Bauer, J., in Micro-optical Technologies for Measurement, Sensors and Microsystems II and Optical Fiber Sensor Technologies and Applications, edited by O.M. Parriaux, B. Culshaw, M. Breidne, E.B. Kley, Proc. SPIE 3099, 212 (1997) CrossRef
Kallioniemi, I., Saarinem, S., Oja, E., Appl. Opt. 37, 5830 (1998) CrossRef
Kallioniemi, I. , Saarinem, S., Oja, E., Appl. Opt. 38, 5920 (1999) CrossRef
Robert, S., Mure Ravaud, A., Lacour, D., J. Opt. Soc. Am. A 19, 24 (2002) CrossRef
Bischoff, J., Bauer, J.J., Haak, U., Hutschenreuther, L., Truckenbrodt, H., in Conference on metrology and Process control for microlithography XII, edited by B. Singh, Proc. SPIE 3332, 526 (1998) CrossRef
Robert, S., Mure-Ravaud, A., in Optical Fabrication, Testing, and Metrology, edited by R. Geyl, D. Rimmer, L. Wang, Proc. SPIE 5252, 156 (2003) CrossRef
Robert, S., Mure Ravaud, A., Reynaud, S., Fourment, S., Carcenac, F., Arguuel, P., J. Opt. Soc. Am. A 19, 2394 (2002) CrossRef
Li, L., Haggans, C.W., J. Opt. Soc. Am. A 10, 1184 (1993) CrossRef
Li, L., J. Opt. Soc. Am. A 10, 2581 (1993) CrossRef
C.M. Bishop, Neural Networks for Pattern recognition (Clarendon Press, Oxford, 1995)
Hornik, K., Stinchcombe, M., White, H., Auer, P., Neural Comput. 6, 1262 (1994) CrossRef
Barron, A., IEEE T. Inform. Theory 39, 930 (1993) CrossRef
Levenberg, K., Q. Appl. Math. 2, 164 (1994) CrossRef
Marquardt, D., J. Appl. Math. 11, 431 (1963)
G. Dreyfus, J.M. Martinez, M. Samuelides, M.B. Gordon, F. Bardan, S. Thiria, L. Herault, Réseaux de neurones : Méthodologie et applications (Editions Eyrolles, Paris, 2002)
D. Nix, A. Weigend, in Advanced in neural information processing systems, edited by G. Tesauro et al. (MIT Press, Cambridge, 1995), p. 489