Published online by Cambridge University Press: 09 March 2009
A manipulator regressor is an n x l matrix function in the dynamic expression τ = Y 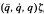 r or
r or 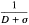 τ = W
τ = W r, which linearizes the robotic dynamics with respect to a properly defined inertia parameter vector ζr є R1. Many modern adaptive controllers require on-line computation of a regressor to estimate the unknown inertia parameters and ensure robustness of the closed-loop system.
r, which linearizes the robotic dynamics with respect to a properly defined inertia parameter vector ζr є R1. Many modern adaptive controllers require on-line computation of a regressor to estimate the unknown inertia parameters and ensure robustness of the closed-loop system.
While the computation of Y 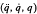 is studied by Atkeson, An and Hollerbach1 and Khosla and Kanade,2 the computation of W
is studied by Atkeson, An and Hollerbach1 and Khosla and Kanade,2 the computation of W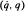 for a general n–link robot has not been reported in the literature. This paper presents an algorithm to compute W
for a general n–link robot has not been reported in the literature. This paper presents an algorithm to compute W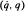 for a general n–link robotic manipulator. The variables used to construct the regressor matrix are directly available from the outward iteration of a Newton-Euler algorithm; some additional arithmetic operations and first-order, low-pass filtering are needed. The identification of unknown inertia parameters is also discussed.
for a general n–link robotic manipulator. The variables used to construct the regressor matrix are directly available from the outward iteration of a Newton-Euler algorithm; some additional arithmetic operations and first-order, low-pass filtering are needed. The identification of unknown inertia parameters is also discussed.