Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2025-01-03T13:45:19.030Z Has data issue: false hasContentIssue false
  • English
  • Français

MODAL LOGICS OF METRIC SPACES

Published online by Cambridge University Press:  18 December 2014

GURAM BEZHANISHVILI ,
DAVID GABELAIA  and
JOEL LUCERO-BRYAN
GURAM BEZHANISHVILI*
Affiliation:
Department of Mathematical Sciences, New Mexico State University
DAVID GABELAIA*
Affiliation:
A. Razmadze Mathematical Institute, Tbilisi State University
JOEL LUCERO-BRYAN*
Affiliation:
Department of Applied Mathematics and Sciences, Khalifa University
*
*DEPARTMENT OF MATHEMATICAL SCIENCES, NEW MEXICO STATE UNIVERSITY, LAS CRUCES NM 88003, USA E-mail:[email protected]
†A. RAZMADZE MATHEMATICAL INSTITUTE, TBILISI STATE UNIVERSITY, 6 TAMARASHVILI STR., TBILISI 0177, GEORGIA E-mail:[email protected]
‡DEPARTMENT OF APPLIED MATHEMATICS AND SCIENCES, KHALIFA UNIVERSITY, ABU DHABI, UAE E-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

It is a classic result (McKinsey & Tarski, 1944; Rasiowa & Sikorski, 1963) that if we interpret modal diamond as topological closure, then the modal logic of any dense-in-itself metric space is the well-known modal system S4. In this paper, as a natural follow-up, we study the modal logic of an arbitrary metric space. Our main result establishes that modal logics arising from metric spaces form the following chain which is order-isomorphic (with respect to the ⊃ relation) to the ordinal ω + 3:

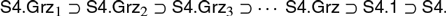$S4.Gr{z_1} \supset S4.Gr{z_2} \supset S4.Gr{z_3} \supset \cdots \,S4.Grz \supset S4.1 \supset S4.$

It follows that the modal logic of an arbitrary metric space is finitely axiomatizable, has the finite model property, and hence is decidable.

Type
Research Article
Information
The Review of Symbolic Logic , Volume 8 , Issue 1 , March 2015 , pp. 178 - 191
Copyright
Copyright © Association for Symbolic Logic 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Bezhanishvili, G., & Harding, J. (2012). Modal logics of Stone spaces. Order, 29, 271–292.CrossRefGoogle Scholar
Blackburn, P., de Rijke, M., & Venema, Y. (2001). Modal Logic. Cambridge: Cambridge University Press, UK.Google Scholar
Chagrov, A., & Zakharyaschev, M. (1997). Modal Logic. Oxford: Oxford University Press, UK.Google Scholar
Engelking, R. (1989). General Topology (second edition). Berlin: Heldermann Verlag.Google Scholar
Esakia, L. (1981). Diagonal constructions, Löb’s formula and Cantor’s scattered spaces. Studies in Logic and Semantics. Tbilisi: Metsniereba, pp. 128–143. (In Russian).Google Scholar
McKinsey, J. C. C., & Tarski, A. (1944). The algebra of topology. Annals of Mathematics, 45, 141–191.CrossRefGoogle Scholar
Rasiowa, H., & Sikorski, R. (1963). The Mathematics of Metamathematics, Monografie Matematyczne, Tom 41. Warsaw: Państwowe Wydawnictwo Naukowe.Google Scholar
Stone, M. H. (1936). The theory of representations for Boolean algebras. Transactions of the American Mathematical Society, 40, 37–111.Google Scholar
Telgársky, R. (1968). Total paracompactness and paracompact dispersed spaces. Bulletin de l’Académie Polonaise des Sciences. Série des Sciences Mathématiques, Astronomiques et Physiques, 16, 567–572.Google Scholar
van Benthem, J., Bezhanishvili, G., & Gehrke, M. (2003). Euclidean hierarchy in modal logic. Studia Logica, 75, 327–344.Google Scholar