Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hromkovič, Juraj
2001.
Algorithmics for Hard Problems.
p.
213.
Engebretsen, Lars
and
Karpinski, Marek
2001.
Automata, Languages and Programming.
Vol. 2076,
Issue. ,
p.
201.
Demange, Marc
and
Paschos, Vangelis
2002.
Autour de nouvelles notions pour l'analyse des algorithmes d'approximation : de la structure de NPO à la structure des instances.
RAIRO - Operations Research,
Vol. 36,
Issue. 4,
p.
311.
Böckenhauer, Hans-Joachim
Bongartz, Dirk
Hromkovič, Juraj
Klasing, Ralf
Proietti, Guido
Seibert, Sebastian
and
Unger, Walter
2002.
FST TCS 2002: Foundations of Software Technology and Theoretical Computer Science.
Vol. 2556,
Issue. ,
p.
59.
Böckenhauer, Hans-Joachim
Bongartz, Dirk
Hromkovič, Juraj
Klasing, Ralf
Proietti, Guido
Seibert, Sebastian
and
Unger, Walter
2003.
Algorithms and Complexity.
Vol. 2653,
Issue. ,
p.
189.
Hromkovič, Juraj
2004.
Exploring New Frontiers of Theoretical Informatics.
Vol. 155,
Issue. ,
p.
3.
Böckenhauer, Hans-Joachim
Bongartz, Dirk
Hromkovič, Juraj
Klasing, Ralf
Proietti, Guido
Seibert, Sebastian
and
Unger, Walter
2004.
On the hardness of constructing minimal 2-connected spanning subgraphs in complete graphs with sharpened triangle inequality.
Theoretical Computer Science,
Vol. 326,
Issue. 1-3,
p.
137.
Böckenhauer, Hans-Joachim
Forlizzi, Luca
Hromkovič, Juraj
Kneis, Joachim
Kupke, Joachim
Proietti, Guido
and
Widmayer, Peter
2006.
Fourth IFIP International Conference on Theoretical Computer Science- TCS 2006.
Vol. 209,
Issue. ,
p.
251.
Engebretsen, Lars
and
Karpinski, Marek
2006.
TSP with bounded metrics.
Journal of Computer and System Sciences,
Vol. 72,
Issue. 4,
p.
509.
Böckenhauer, Hans-Joachim
Hromkovič, Juraj
Kneis, Joachim
and
Kupke, Joachim
2006.
Algorithm Theory – SWAT 2006.
Vol. 4059,
Issue. ,
p.
184.
Böckenhauer, Hans-Joachim
Bongartz, Dirk
Hromkovič, Juraj
Klasing, Ralf
Proietti, Guido
Seibert, Sebastian
and
Unger, Walter
2008.
On k-connectivity problems with sharpened triangle inequality.
Journal of Discrete Algorithms,
Vol. 6,
Issue. 4,
p.
605.
Böckenhauer, Hans-Joachim
Hromkovič, Juraj
Mömke, Tobias
and
Widmayer, Peter
2008.
SOFSEM 2008: Theory and Practice of Computer Science.
Vol. 4910,
Issue. ,
p.
50.
Hromkovič, Juraj
2010.
Computer Science – Theory and Applications.
Vol. 6072,
Issue. ,
p.
181.
Karpinski, Marek
Lampis, Michael
and
Schmied, Richard
2013.
Algorithms and Computation.
Vol. 8283,
Issue. ,
p.
568.
Lampis, Michael
2014.
Guest column.
ACM SIGACT News,
Vol. 45,
Issue. 1,
p.
48.
Chen, Li-Hsuan
Hsieh, Sun-Yuan
Hung, Ling-Ju
and
Klasing, Ralf
2017.
Computing and Combinatorics.
Vol. 10392,
Issue. ,
p.
112.
Chen, Li-Hsuan
Hsieh, Sun-Yuan
Hung, Ling-Ju
Klasing, Ralf
Lee, Chia-Wei
and
Wu, Bang Ye
2017.
Algorithms and Complexity.
Vol. 10236,
Issue. ,
p.
152.
Klasing, Ralf
and
Mömke, Tobias
2018.
Adventures Between Lower Bounds and Higher Altitudes.
Vol. 11011,
Issue. ,
p.
393.
Chen, Li-Hsuan
Cheng, Dun-Wei
Hsieh, Sun-Yuan
Hung, Ling-Ju
Klasing, Ralf
Lee, Chia-Wei
and
Wu, Bang Ye
2018.
Approximability and inapproximability of the star p-hub center problem with parameterized triangle inequality.
Journal of Computer and System Sciences,
Vol. 92,
Issue. ,
p.
92.
Chen, Li-Hsuan
Hsieh, Sun-Yuan
Hung, Ling-Ju
and
Klasing, Ralf
2018.
Combinatorial Algorithms.
Vol. 10979,
Issue. ,
p.
115.
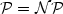 ). First of all, we present an improved lower bound for the Traveling Salesman Problemwith Triangle Inequality, Delta-TSP for short. Moreover our technique, an extension of the method ofEngebretsen [11], also applies to the case of relaxed and sharpened triangle inequality,respectively, denoted $\Delta_\beta$
). First of all, we present an improved lower bound for the Traveling Salesman Problemwith Triangle Inequality, Delta-TSP for short. Moreover our technique, an extension of the method ofEngebretsen [11], also applies to the case of relaxed and sharpened triangle inequality,respectively, denoted $\Delta_\beta$ 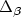 -TSP for an appropriate β. In case of theDelta-TSP, we obtain a lowerbound of $\frac{3813}{3812}-\varepsilon$
-TSP for an appropriate β. In case of theDelta-TSP, we obtain a lowerbound of $\frac{3813}{3812}-\varepsilon$  on the polynomial-time approximability (for any small $\varepsilon> 0$
on the polynomial-time approximability (for any small $\varepsilon> 0$ 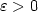 ), compared to the previous bound of $\frac{5381}{5380}-\varepsilon$
), compared to the previous bound of $\frac{5381}{5380}-\varepsilon$ 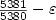 in [11]. Incase of the $\Delta_\beta$
in [11]. Incase of the $\Delta_\beta$ 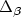 -TSP, for the relaxed case ( $\beta> 1$
-TSP, for the relaxed case ( $\beta> 1$ 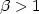 ) we present a lower bound of $\frac{3803+10\beta}{3804+8\beta}-\varepsilon$
) we present a lower bound of $\frac{3803+10\beta}{3804+8\beta}-\varepsilon$  , and for the sharpened triangle inequality( $\frac{1}{2}< \beta< 1$
, and for the sharpened triangle inequality( $\frac{1}{2}< \beta< 1$ 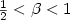 ), the lower bound is $\frac{7611+10\beta^2+5\beta}{7612+8\beta^2+4\beta}-\varepsilon$
), the lower bound is $\frac{7611+10\beta^2+5\beta}{7612+8\beta^2+4\beta}-\varepsilon$ 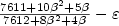 . The latter result is of interestespecially since it shows that the TSP is $\mathcal{APX}$
. The latter result is of interestespecially since it shows that the TSP is $\mathcal{APX}$  -hard even if one comes arbitrarily close to the trivialcase that all edges have the same cost.
-hard even if one comes arbitrarily close to the trivialcase that all edges have the same cost.