(1) The essence of Cayley's mode* of arriving at the formation of an orthogonal substitution lies, as is known, in the observation that if two sets of variables

x, y, z, w, and ξ, η, ζ, ω

be taken linear functions of a third set

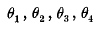
in such a way that the determinant of the coefficients is in the one case

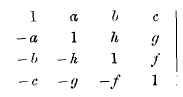
and in the other the conjugate of this, then the first and second set of variables are orthogonally related.