No CrossRef data available.
Published online by Cambridge University Press: 15 September 2014
1. Cayley commences his third paper on Skew Determinants (May, 1854) by recalling his development of them in terms of Pfaffians, and then goes on to say:—
“J'ai trouve recemment une formule analogue pour le developpement d'un déterminant gauche borde, tel que
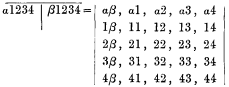
Cette formule est:

and he explains that the expressions 12, 1234, etc., are Pfaffians, whose law of formation is—
12 = 12,
1234 = 12·34 + 13·42 + 14·23,
123456 = 12·34·56 + 13·45·62 + 14·56·23 + 15·62·34 + 16·23·45 + 12·35·64 + 13·46·25 +14·52·36 +15·63·42 + 16·24·53 + 12·36·45 + 13·42·56 + 14·53·62 + 15·64·23 + 16·25·34.
No proof is given, and the law of formation of the development itself is not explained.
page 342 note * Cayley, A., “Recherches ulterieures snr les determinants gauches,” Crelle's Journ., 1. pp. 299–213Google Scholar; or Collected Math. Papers, ii. pp. 202–215Google Scholar.
page 343 note * Cayley, A., “Théorème sur les déterminants gauches,” Crelle's Journ., lv. pp. 277, 278Google Scholar; or Collected Math. Papers, iv. pp. 72, 73Google Scholar.
page 344 note * Quart. Journ. of Math., xviii. pp. 46–49Google Scholar.
page 354 note * Cunningham, Allan, “An Investigation of the Number of Constituents, Elements, and Minors of a Determinant,” Journ. of Science, iv. (1874), pp. 212–228Google Scholar.
page 355 note * Cayley, A., “Sur les déterminants gauches,” Crelle's Journ., xxxviii. pp. 93–96Google Scholar; or Collected Math. Papers, i. pp. 410–413Google Scholar.
page 356 note * Cf. Cunningham's paper, above referred to, p. 225.
page 356 note † Cayley, A., “Théorème sur les déterminants gauches,” Crelle's Journ., lv. pp. 277, 278Google Scholar; or Collected Math. Papers, iv. pp. 72, 73Google Scholar.
page 357 note * “On Bipartite Functions,” Trans. R.S.E., xxxii. pp. 461–481Google Scholar.