Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-24T11:57:20.975Z Has data issue: false hasContentIssue false
  • English
  • Français

IX.—Generalised Derivatives and Integrals*

Published online by Cambridge University Press:  15 September 2014

W. L. Ferrar
Get access

Extract

The various definitions which have been adopted by one or more writers for Dnf(x), where n is real but not an integer, fall roughly into three classes:—

(1) Liouville —a method which assumes a convergent expansion

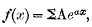
and defines Dnf(x) as ΣAαneax.

(2) Riemann, Grünwald, Laurent and others—methods which, however they begin, ultimately come to the “integral definition,”

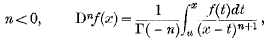
the lower limit of integration being arbitrary:

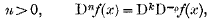
where k is a positive integer, 0<ρ<1, and n=k – ρ.

(3) Pincherle5—a method which, seeking an operator with certain properties, gives an infinite series as a definition of Dnf(x)

Type
Proceedings
Information
Proceedings of the Royal Society of Edinburgh , Volume 48 , 1929 , pp. 92 - 105
Copyright
Copyright © Royal Society of Edinburgh 1929

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

List of writings to which reference has been made

1Liouville, , Journ. de l'École Polytechnique, 13 (cahier 21) (1832), 1–186. There are also later writings in the same journal.Google Scholar
2Riemann, , Gesammelte Werke (1876), xix, pp. 331–344.Google Scholar
3Grünwald, , Zeitschrift für Math, und Physik (Schömilch), 12 (1867), 441–480. This is rather cumbersome, but is one of the comparatively few rigorous discussions that appear in the early literature of definition (2).Google Scholar
4Laurent, , Traité d'Analyse, vol. iii (1888), 487–495. He also gives a formula for generalised differences as an integral.Google Scholar
5Pincherle, , Memorie della R. Acad. di Bologna (Sci.) (5), 9 (1902), 745.Google Scholar
6Hadamard, , Journ. de Math. (4), 8 (1892), 154 [pt. iii of his thesis]. or Dienes, Borel Tract: “Leçons sur les Singularités des Fonctions Analytiques” (1913), pp. 7–9 in particular.Google Scholar
7Davis, , Amer. Journ. of Maths., 46 (1924), 95–109; 49 (1927), 123–142.CrossRefGoogle Scholar
8Andersen, , Studier over Cesàro's Summabilitetsmetode (Copenhagen, 1921) thesis. More conveniently, the reference is for differences only, Proc. Lond. Math. Soc. (2), 27 (1927), 44.Google Scholar
9Kelland, , Trans. Roy. Soc. Edin., 14 (1840), 567, 604 (two papers).CrossRefGoogle Scholar
10Bourlet, , Annales de l'École Normale (3), 14 (1897), 154.Google Scholar
11Cayley, , Collected Works, xi, 235, 6; or Math. Annalen, 16 (1880), 81, 2.Google Scholar
12Hardy, , Mess, of Maths., 47 (1918), 145–150.Google Scholar
Hardy, and Littlewood, , Math. Zeitschrift, 27 (1928), 565–606.CrossRefGoogle Scholar
The following articles in the Ency. Math. Wissenschaften contain numerous other references to the subject: Vol. ii, i, 2, p. 770 ; vol. ii, i, l, pp. 116–119.Google Scholar