Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-13T00:53:41.285Z Has data issue: false hasContentIssue false
  • English
  • Français

III.—The Application of Operators to the Solution of the Algebraic Equation

Published online by Cambridge University Press:  15 September 2014

James Littlejohn
Get access

Extract

The solution of the common quadratic

which is usually written

is, when expanded,

This expansion will be found to be the work of the operator 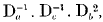each term being derived from its predecessor by this operator's agency, so that the whole solution may be written

Type
Proceedings
Information
Proceedings of the Royal Society of Edinburgh , Volume 37 , 1918 , pp. 18 - 49
Copyright
Copyright © Royal Society of Edinburgh 1918

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

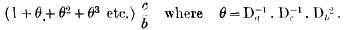
page 34 note * Dropping the constant ⅓, of course.

page 36 note * But see later.

page 38 note * We take here such a combination of signs as excludes negative operands. We are considering merely arithmetical ratios, and for an imaginary root its modulus may be substituted.

page 43 note * See note at end.