No CrossRef data available.
Published online by Cambridge University Press: 15 September 2014
Hamilton has shown that if
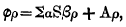
where α and β are given vectors, and A a given scalar, we have
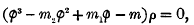
where m, m1, m2, are scalars depending only on φ.
When the function φ is its own conjugate, i.e., when

ρ and σ being any vectors whatever, the vectors for which
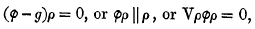
form in general a real and definite rectangular system. This, of course, may in particular cases degrade into one definite vector, and any pair of others perpendicular to it; and cases may occur in which the equation is satisfied for every vector.