Hostname: page-component-7b9c58cd5d-dkgms Total loading time: 0 Render date: 2025-03-16T13:00:45.383Z Has data issue: false hasContentIssue false
  • English
  • Français

A weighted Trudinger–Moser inequalities and applications to some weighted $(N,q)-$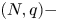Laplacian equation in $\mathbb {R}^N$ 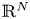with new exponential growth conditions

Part of: Linear function spaces and their duals Partial differential equations Representations of solutions Inequalities Elliptic equations and systems

Published online by Cambridge University Press:  07 September 2023

Sami Aouaoui
Sami Aouaoui*
Affiliation:
University of Kairouan, High Institute of Applied Mathematics and Informatics of Kairouan, Avenue Assad Iben Fourat, 3100 Kairouan, Tunisia ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we prove some weighted sharp inequalities of Trudinger–Moser type. The weights considered here have a logarithmic growth. These inequalities are completely new and are established in some new Sobolev spaces where the norm is a mixture of the norm of the gradient in two different Lebesgue spaces. This fact allowed us to prove a very interesting result of sharpness for the case of doubly exponential growth at infinity. Some improvements of these inequalities for the weakly convergent sequences are also proved using a version of the Concentration-Compactness principle of P.L. Lions. Taking profit of these inequalities, we treat in the last part of this work some elliptic quasilinear equation involving the weighted $(N,q)-$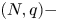Laplacian operator where $1 < q < N$ 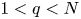and a nonlinearities enjoying a new type of exponential growth condition at infinity.

Keywords

Weighted Trudinger–Moser inequalityweighted Sobolev spaceslogarithmic weightsdoubly exponential growthradial functionsconcentration-compactnessweighted (N,q)–Laplacianelliptic equation

MSC classification

Primary: 26D15: Inequalities for sums, series and integrals
Secondary: 35A15: Variational methods 35A23: Inequalities involving derivatives and differential and integral operators, inequalities for integrals 35B38: Critical points 35D30: Weak solutions 35J20: Variational methods for second-order elliptic equations 35J62: Quasilinear elliptic equations 46E35: Sobolev spaces and other spaces of “smooth” functions, embedding theorems, trace theorems
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , First View , pp. 1 - 52
Check for updates
Copyright
Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abreu, A. and Fernandez, L. G. Jr. On a weighted Trudinger–Moser inequality in $\mathbb {R}^N$. J. Differ. Equ. 269 (2020), 3089–3118.CrossRefGoogle Scholar
Albuquerque, F. S. B., Alves, C. O. and Medeiros, E. S.. Nonlinear Schrödinger equation with unbounded or decaying radial potentials involving exponential critical growth in $\mathbb{R}2$. J. Math. Anal. Appl. 409 (2014), 1021–1031.CrossRefGoogle Scholar
Albuquerque, F. S. B.. Sharp constant and extremal function for weighted Trudinger–Moser type inequalities in $\mathbb {R}^2$. J. Math. Anal. Appl. 421 (2015), 963–970.CrossRefGoogle Scholar
Aouaoui, S.. A new Trudinger–Moser type inequality and an application to some elliptic equation with doubly exponential nonlinearity in the whole space $\mathbb {R}^2$. Arch. Math. 114 (2020), 199–214.CrossRefGoogle Scholar
Aouaoui, S. and Albuquerque, F. S. B.. A weighted Trudinger–Moser type inequality and its applications to quasilinear elliptic problems with critical growth in the whole Euclidean space. Topol. Methods Nonlinear Anal. 54 (2019), 109–130.Google Scholar
Aouaoui, S. and Jlel, R.. A new singular Trudinger–Moser type inequality with logarithmic weights and applications. Adv. Nonlinear Stud. 20 (2020), 113–139.CrossRefGoogle Scholar
Aouaoui, S. and Jlel, R.. On some elliptic equation in the whole Euclidean space $\mathbb {R}^2$ with nonlinearities having new exponential growth condition. Commun. Pure Appl. Anal. 19 (2020), 4771–4796.CrossRefGoogle Scholar
Aouaoui, S. and Jlel, R.. New weighted sharp Trudinger–Moser inequalities defined on the whole Euclidean space $\mathbb {R}^N$ and applications. Calc. Var. Partial Differ. Equ. 60 (2021), 50.CrossRefGoogle Scholar
Aouaoui, S. and Jlel, R.. Correction to: ‘New weighted sharp Trudinger–Moser inequalities defined on the whole Euclidean space $\mathbb {R}^N$ and applications’. Calc. Var. Partial Differ. Equ. 62 (2023), 154.CrossRefGoogle Scholar
Aouaoui, S. and Jlel, R.. Singular weighted sharp Trudinger–Moser inequalities defined on and applications to elliptic nonlinear equations. Discrete Contin. Dyn. Syst. 42 (2022), 781–813.CrossRefGoogle Scholar
Aouaoui, S. and Jlel, R.. Corrigendum and addendum to: ‘Singular weighted sharp Trudinger–Moser inequalities defined on $\mathbb {R}^N$ and applications to elliptic nonlinear equations’. DCDS 43 (2023), 3170–3173.CrossRefGoogle Scholar
Brezis, H.. Functional analysis, Sobolev spaces and Partial differential equations (New York, USA: Springer, 2011).CrossRefGoogle Scholar
Calanchi, M.. Some weighted inequalities of Trudinger–Moser Type. In (eds) Analysis and Topology in Nonlinear Differential Equations, Progress in Nonlinear Differential Equations and Applications, Vol. 85, pp. 163–174 (Springer, Birkhauser, 2014), Cham. https://doi.org/10.1007/978-3-319-04214-5_9CrossRefGoogle Scholar
Calanchi, M., Massa, E. and Ruf, B.. Weighted Trudinger–Moser inequalities and associated Liouville type equations. Proc. Am. Math. Soc. 146 (2018), 5243–5256.CrossRefGoogle Scholar
Calanchi, M. and Ruf, B.. On Trudinger–Moser type inequalities with logarithmic weights. J. Differ. Equ. 258 (2015), 1967–1989.CrossRefGoogle Scholar
Calanchi, M. and Ruf, B.. Trudinger–Moser type inequalities with logarithmic weights in dimension $N$. Nonlinear Anal. 121 (2015), 403–411.CrossRefGoogle Scholar
Calanchi, M., Ruf, B. and Sani, F.. Elliptic equations in dimension 2 with double exponential nonlinearities. Nonlinear Differ. Equ. Appl. 24 (2017), 29.CrossRefGoogle Scholar
Cavalheiro, A. C.. Weighted Sobolev spaces and degenerate elliptic equations. Bol. Soc. Paran. Mat. 26 (2008), 117–132.Google Scholar
Carvalho, J. L., Figueiredo, G. M., Furtado, M. F. and Medeiros, E.. On a zero-mass $(N,q)-$Laplacian equation in $\mathbb {R}^N$ with exponential critical growth. Nonlinear Anal. 213 (2021), 112488.CrossRefGoogle Scholar
Chen, S., Fiscella, A., Pucci, P. and Tang, X.. Coupled elliptic systems in $\mathbb {R}^N$ with $(p,N)$ Laplacian and critical exponential nonlinearities. Nonlinear Anal. 201 (2020), 112066.CrossRefGoogle Scholar
de Oliveira, J. F. and do Ò, J. M.. Trudinger–Moser type inequalities for weighted spaces involving fractional dimensions. Proc. Am. Math. Soc. 142 (2014), 2813–2828.CrossRefGoogle Scholar
do Ò, J. M. and de Souza, M.. On a class of singular Trudinger–Moser inequalities. Math. Nachr. 284 (2011), 1754–1776.CrossRefGoogle Scholar
do Ó, J. M., Medeiros, E. and Severo, U. B.. On a quasilinear nonhomogeneous elliptic equation with critical growth in $\mathbb {R}^n$. J. Differ. Equ. 246 (2009), 1363–1386.CrossRefGoogle Scholar
Figueiredo, G. M. and Nunes, F. B. M.. Existence of positive solutions for a class of quasilinear elliptic problems with exponential growth via the Nehari manifold method. Rev. Mat. Complut. 32 (2019), 1–18.CrossRefGoogle Scholar
Fiscella, A. and Pucci, P.. $(P,N)$ equations with critical exponential nonlinearities in $\mathbb {R}^N$. J. Math. Anal. Appl. 501 (2021), 123379.CrossRefGoogle Scholar
Furtado, M. F., Medeiros, E. S. and Severo, U. B.. A Trudinger–Moser inequality in a weighted Sobolev space and applications. Math. Nach. 287 (2014), 1255–1273.CrossRefGoogle Scholar
Haroske, D. D.. Sobolev spaces with Muckenhoupt weights, singularities and inequalities. Georgian Math. J. 15 (2008), 263–280.CrossRefGoogle Scholar
Kilpeläinen, T.. Weighted Sobolev spaces and capacity. Ann. Acad. Sci. Fenn. Math. 19 (1994), 95–113.Google Scholar
Lam, N.. Sharp Trudinger–Moser inequalities with monomial weights. Nonlinear Differ. Equ. Appl. 24 (2017), 29.CrossRefGoogle Scholar
Lions, P. L.. The concentration-compactness principle in the calculus of variations. The limit case. I. Rev. Mat. Iberoam. 1 (1985), 145–201.CrossRefGoogle Scholar
Moser, J.. A sharp form of an inequality by N. Trudinger. Indiana Univ. Math. J. 20 (1971), 1077–1092.CrossRefGoogle Scholar
Nakai, E., Tomita, N. and Yabuta, K.. Density of the set of all infinitely differentiable functions with compact support in weighted Sobolev spaces. Sc. Math. Jpn. 10 (2004), 39–45.Google Scholar
Nguyen, V. H. and Takahashi, F.. On a weighted Trudinger–Moser type inequality on the whole space and related maximizing problem. Differ. Integr. Equ. 31 (2018), 785–806.Google Scholar
Nguyen, V. H.. Remarks on the Moser–Trudinger type inequality with logarithmic weights in dimension N. Proc. Am. Math. Soc. 147 (2018), 5183–5193.CrossRefGoogle Scholar
Roy, P.. Extremal function for Moser–Trudinger type inequality with logarithmic weight. Nonlinear Anal. 135 (2016), 194–204.CrossRefGoogle Scholar
Roy, P.. On attainability of Moser Trudinger inequality with logarithmic weights in higher dimensions. Discrete Contin. Dyn. Syst. 39 (2019), 5207–5222.CrossRefGoogle Scholar
Su, J., Wang, Z. Q. and Willem, M.. Weighted Sobolev embedding with unbounded and decaying radial potentials. J. Differ. Equ. 238 (2007), 201–219.CrossRefGoogle Scholar
Trudinger, N. S.. On embedding into Orlicz spaces and some applications. J. Math. Mech. 17 (1967), 473–484.Google Scholar
Yang, Y. and Perera, K.. $(N,q)$-Laplacian problems with critical Trudinger–Moser nonlinearities. Bull. London Math. Soc. 48 (2016), 260–270.CrossRefGoogle Scholar
Zhang, C.. Concentration-compactness principle for Trudinger–Moser inequalities with logarithmic weights and their applications. Nonlinear Anal. 197 (2020), 111845.CrossRefGoogle Scholar