Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-28T02:47:02.671Z Has data issue: false hasContentIssue false
  • English
  • Français

A weighted norm inequality for the Hankel transformation

Published online by Cambridge University Press:  14 November 2011

P. Heywood  and
P. G. Rooney
P. Heywood
Affiliation:
Department of Mathematics, University of Edinburgh, Mayfield Road, Edinburgh EH9 3JZ
P. G. Rooney
Affiliation:
Department of Mathematics, University of Toronto, Toronto, Ontario M5S 1A1, Canada
Get access Rights & Permissions [Opens in a new window]

Extract

We give conditions on pairs of non-negative weight functions U and V which are sufficient that for 1<p≤<∞

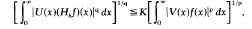
where Hλ is the Hankel transformation.

The technique of proof is a variant of Muckenhoupt's recent proof for the boundedness of the Fourier transformation between weighted Lp spaces, and we can also use this variant to prove a somewhat different boundedness theorem for the Fourier transformation.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 99 , Issue 1-2 , 1984 , pp. 45 - 50
Copyright
Copyright © Royal Society of Edinburgh 1984

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Aguilera, N. E. and Harboure, E. O.. On the search of weighted norm inequalities for the Fourier transform (to appear).Google Scholar
2Flett, T. M.. On a theorem of Pitt. J. London Math. Soc. 7 (1973), 376–384.Google Scholar
3Hardy, G. H., Littlewood, J. E. and Polya, G.. Inequalities (Cambridge University Press, 1934).Google Scholar
4Heinig, H. P.. Weighted norm inequalities for classes of operators (preprint).Google Scholar
5Jurkat, W. B. and Sampson, G.. On rearrangement and weight inequalities for the Fourier transform (preprint).Google Scholar
6Knopf, P. and Rudnick, K.. Weighted norm inequalities for the Fourier transform (preprint).Google Scholar
7Muckenhoupt, B.. Weighted norm inequalities for the Fourier transform. Trans. Amer. Math. Soc. 276 (1983), 729–742.Google Scholar
8Muckenhoupt, B.. A note on two weight function conditions for a Fourier transform norm inequality. Proc. Amer. Math. Soc. 88 (1983), 97–100.CrossRefGoogle Scholar
9Rooney, P. G.. A technique for studying the boundedness and extendability of certain types of operators. Canad. J. Math. 25 (1973), 1090–1102.CrossRefGoogle Scholar
10Sagher, Y.. Real interpolation with weights. Indiana Univ. Math. J. 30 (1981), 113–121.Google Scholar