No CrossRef data available.
Published online by Cambridge University Press: 18 September 2024
In loving memory of my beloved miniature dachshund Maddie (16 March 2002 – 16 March 2020). We consider nonlocal differential equations with convolution coefficients of the form\[{-}M\Big(\big(a*(g\circ |u|)\big)(1)\Big)u''(t)=\lambda f\big(t,u(t)\big),\quad t\in(0,1), \]in the case in which $g$
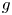 can satisfy very generalized growth conditions; in addition, $M$
can satisfy very generalized growth conditions; in addition, $M$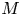 is allowed to be both sign-changing and vanishing. Existence of at least one positive solution to this equation equipped with boundary data is considered. We demonstrate that the nonlocal coefficient $M$
is allowed to be both sign-changing and vanishing. Existence of at least one positive solution to this equation equipped with boundary data is considered. We demonstrate that the nonlocal coefficient $M$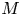 allows the forcing term $f$
allows the forcing term $f$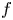 to be free of almost all assumptions other than continuity.
to be free of almost all assumptions other than continuity.