1. Introduction
Linear elasticity is a well-known and powerful mathematical approximation of the nonlinear theory of elasticity, with extensive application to the structural analysis and the numerical treatment of elastic bodies. In engineering textbooks its derivation is classical and is based on a formal linearization of finite elasticity about a reference configuration. A rigorous mathematical derivation via $\Gamma$![]() -convergence was developed only rather recently in the pioneering work [Reference Dal Maso, Negri and Percivale8], where a Dirichlet boundary value problem was considered. A similar approach was then applied to different frameworks in elasticity, such as rubber-like materials [Reference Agostiniani, Dal Maso and DeSimone3], multiwell models [Reference Alicandro, Dal Maso, Lazzaroni and Palombaro1, Reference Agostiniani, Blass and Koumatos2, Reference Schmidt32], elasticity with residual stress [Reference Paroni and Tomassetti27, Reference Paroni and Tomassetti28] and incompressible materials [Reference Mainini and Percivale21]. Beyond elasticity we also mention the papers [Reference Friedrich9, Reference Friedrich10, Reference Negri and Toader25, Reference Negri and Zanini26] for models in fracture mechanics, [Reference Friedrich and Kružik12] for viscoelasticity, [Reference Mielke and Stefanelli23] for plasticity and the recent contribution [Reference Friedrich, Kreutz and Zemas11] for materials with stress-driven rearrangement instabilities.
-convergence was developed only rather recently in the pioneering work [Reference Dal Maso, Negri and Percivale8], where a Dirichlet boundary value problem was considered. A similar approach was then applied to different frameworks in elasticity, such as rubber-like materials [Reference Agostiniani, Dal Maso and DeSimone3], multiwell models [Reference Alicandro, Dal Maso, Lazzaroni and Palombaro1, Reference Agostiniani, Blass and Koumatos2, Reference Schmidt32], elasticity with residual stress [Reference Paroni and Tomassetti27, Reference Paroni and Tomassetti28] and incompressible materials [Reference Mainini and Percivale21]. Beyond elasticity we also mention the papers [Reference Friedrich9, Reference Friedrich10, Reference Negri and Toader25, Reference Negri and Zanini26] for models in fracture mechanics, [Reference Friedrich and Kružik12] for viscoelasticity, [Reference Mielke and Stefanelli23] for plasticity and the recent contribution [Reference Friedrich, Kreutz and Zemas11] for materials with stress-driven rearrangement instabilities.
Linearization of pure traction problems has been recently studied in [Reference Jesenko and Schmidt14, Reference Maddalena, Percivale and Tomarelli16, Reference Maddalena, Percivale and Tomarelli17, Reference Mainini and Percivale19], again in the context of elasticity. In this setting a full $\Gamma$![]() -convergence result has been obtained in [Reference Maor and Mora22] and later extended to incompressible materials in [Reference Mainini and Percivale20]. As observed in [Reference Maor and Mora22], in the Dirichlet case the boundary conditions prescribe the rigid motion to linearize about, whereas in the purely Neumann case the linearization process occurs around suitable rotations that are preferred by the applied forces.
-convergence result has been obtained in [Reference Maor and Mora22] and later extended to incompressible materials in [Reference Mainini and Percivale20]. As observed in [Reference Maor and Mora22], in the Dirichlet case the boundary conditions prescribe the rigid motion to linearize about, whereas in the purely Neumann case the linearization process occurs around suitable rotations that are preferred by the applied forces.
In all this literature the main focus is on understanding the behaviour of the bulk elastic energy and the applied forces are usually assumed to be dead loads, namely their density in the reference configuration is independent of the actual deformation. This assumption is mathematically convenient, since the work done by the loadings turns out to be a continuous perturbation of the elastic energy, so that $\Gamma$![]() -convergence of the total energy immediately follows from $\Gamma$
-convergence of the total energy immediately follows from $\Gamma$![]() -convergence of the elastic energy. However, restricting the analysis to dead loads is physically unsatisfactory, since the only realistic examples of dead loads are the gravitational body force and the zero surface load (see, e.g. [Reference Podio-Guidugli29, Reference Podio-Guidugli and Vergara Caffarelli30] and [Reference Ciarlet4, § 2.7]).
-convergence of the elastic energy. However, restricting the analysis to dead loads is physically unsatisfactory, since the only realistic examples of dead loads are the gravitational body force and the zero surface load (see, e.g. [Reference Podio-Guidugli29, Reference Podio-Guidugli and Vergara Caffarelli30] and [Reference Ciarlet4, § 2.7]).
In this paper we begin a study of the derivation of linear elasticity in the presence of live loads. More precisely, we consider a pure traction problem for a hyperelastic body $\Omega \subset \mathbb {R}^n$![]() subject to a (small) pressure load on its boundary. In this setting the total energy of a deformation $y\colon \Omega \rightarrow \mathbb {R}^n$
subject to a (small) pressure load on its boundary. In this setting the total energy of a deformation $y\colon \Omega \rightarrow \mathbb {R}^n$![]() is given by
is given by
where the elastic energy density $W\colon \Omega \times \mathbb {R}^{n\times n}\rightarrow [0,\,+\infty ]$![]() satisfies the usual assumptions of nonlinear elasticity (see (W1)–(W5)) and $\varepsilon \pi$
satisfies the usual assumptions of nonlinear elasticity (see (W1)–(W5)) and $\varepsilon \pi$![]() is the intensity of the applied pressure load, with $\varepsilon >0$
is the intensity of the applied pressure load, with $\varepsilon >0$![]() a small parameter and $\pi \colon \mathbb {R}^n\rightarrow \mathbb {R}$
a small parameter and $\pi \colon \mathbb {R}^n\rightarrow \mathbb {R}$![]() a given function. For simplicity in this Introduction we assume $\pi$
a given function. For simplicity in this Introduction we assume $\pi$![]() to be continuous. As shown in [Reference Podio-Guidugli and Vergara Caffarelli30, proposition 5.1] (see also [Reference Kružik and Roubíček15, proposition 1.2.8]), the second term in the energy ${\mathcal {T}}_\varepsilon$
to be continuous. As shown in [Reference Podio-Guidugli and Vergara Caffarelli30, proposition 5.1] (see also [Reference Kružik and Roubíček15, proposition 1.2.8]), the second term in the energy ${\mathcal {T}}_\varepsilon$![]() is the potential of the pressure load
is the potential of the pressure load
acting on the whole boundary of $\Omega$![]() , where ${\rm cof}\,F$
, where ${\rm cof}\,F$![]() denotes the cofactor of the matrix $F$
denotes the cofactor of the matrix $F$![]() and $n_{\partial \Omega }$
and $n_{\partial \Omega }$![]() is the outward unit normal to $\partial \Omega$
is the outward unit normal to $\partial \Omega$![]() . In the deformed configuration $y(\Omega )$
. In the deformed configuration $y(\Omega )$![]() the pressure load (1.1) corresponds to the surface force
the pressure load (1.1) corresponds to the surface force
Since $W(x,\,\cdot )$![]() is frame-indifferent and minimized at the identity, it is immediate to see that for $\varepsilon =0$
is frame-indifferent and minimized at the identity, it is immediate to see that for $\varepsilon =0$![]() the minimizers of $\mathcal {T}_\varepsilon$
the minimizers of $\mathcal {T}_\varepsilon$![]() are all the rigid motions of $\Omega$
are all the rigid motions of $\Omega$![]() . When $\varepsilon$
. When $\varepsilon$![]() is small, it is thus natural to expect minimizers to be close to rigid motions and their asymptotic behaviour to be described by a linearization of the energy. In pure traction problems, as mentioned before, the applied forces select the class of rigid motions around which the linearization takes place (see [Reference Maor and Mora22]). Indeed, if $y_\varepsilon$
is small, it is thus natural to expect minimizers to be close to rigid motions and their asymptotic behaviour to be described by a linearization of the energy. In pure traction problems, as mentioned before, the applied forces select the class of rigid motions around which the linearization takes place (see [Reference Maor and Mora22]). Indeed, if $y_\varepsilon$![]() is a minimizer of $\mathcal {T}_\varepsilon$
is a minimizer of $\mathcal {T}_\varepsilon$![]() , then we have
, then we have
If we assume $y_\varepsilon$![]() to be of the form $y_\varepsilon (x)=R_0(x+\varepsilon u_0(x))$
to be of the form $y_\varepsilon (x)=R_0(x+\varepsilon u_0(x))$![]() with $R_0\in SO(n)$
with $R_0\in SO(n)$![]() , then by a formal expansion we obtain
, then by a formal expansion we obtain
for every rotation $R\in SO(n)$![]() . Here $Q(x,\,\cdot )$
. Here $Q(x,\,\cdot )$![]() is the quadratic form given by the Hessian of $W(x,\,\cdot )$
is the quadratic form given by the Hessian of $W(x,\,\cdot )$![]() computed at the identity and $e(u_0)$
computed at the identity and $e(u_0)$![]() is the symmetric gradient of $u_0$
is the symmetric gradient of $u_0$![]() . Dividing by $\varepsilon$
. Dividing by $\varepsilon$![]() and letting $\varepsilon$
and letting $\varepsilon$![]() tend to zero, we deduce that $R_0$
tend to zero, we deduce that $R_0$![]() is a so-called optimal rotation, that is, $R_0$
is a so-called optimal rotation, that is, $R_0$![]() belongs to the set
belongs to the set
Assume now for simplicity that the identity matrix belongs to $\mathcal {R}$![]() (one can always reduce to this case, up to rotating the whole system). The previous argument suggests that in order to identify the limiting behaviour of minimizers one needs to renormalize the energy as follows:
(one can always reduce to this case, up to rotating the whole system). The previous argument suggests that in order to identify the limiting behaviour of minimizers one needs to renormalize the energy as follows:
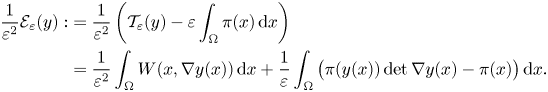
Under suitable assumptions for $\pi$![]() and a weak $p$
and a weak $p$![]() -coercivity condition on $W$
-coercivity condition on $W$![]() with $1< p\leq 2$
with $1< p\leq 2$![]() (see (W5)), we compute the $\Gamma$
(see (W5)), we compute the $\Gamma$![]() -limit of the rescaled energies $(1/{\varepsilon ^2})\mathcal {E}_\varepsilon$
-limit of the rescaled energies $(1/{\varepsilon ^2})\mathcal {E}_\varepsilon$![]() and we establish a compactness result for deformations with equibounded energies. Here deformations are assumed to have zero average on $\Omega$
and we establish a compactness result for deformations with equibounded energies. Here deformations are assumed to have zero average on $\Omega$![]() , as it is common in Neumann boundary value problems. More precisely, we prove the following results:
, as it is common in Neumann boundary value problems. More precisely, we prove the following results:
Compactness: If $\mathcal {E}_\varepsilon (y_\varepsilon )\leq C\varepsilon ^2$![]() , then there exist rotations $R_\varepsilon \in SO(n)$
, then there exist rotations $R_\varepsilon \in SO(n)$![]() and displacements $u_\varepsilon \in W^{1,p}(\Omega ;\mathbb {R}^n)$
and displacements $u_\varepsilon \in W^{1,p}(\Omega ;\mathbb {R}^n)$![]() such that
such that
and, up to subsequences, there holds
• $u_\varepsilon \rightharpoonup u_0$
 weakly in $W^{1,p}(\Omega ;\mathbb {R}^n)$
weakly in $W^{1,p}(\Omega ;\mathbb {R}^n)$ with $u_0\in H^{1}(\Omega ;\mathbb {R}^n)$
with $u_0\in H^{1}(\Omega ;\mathbb {R}^n)$ ,
,• $R_\varepsilon \rightarrow R_0$
 with $R_0\in \mathcal {R}$
with $R_0\in \mathcal {R}$ .
.
$\Gamma$![]() -convergence: Under the above notion of convergence $y_\varepsilon \rightarrow (u_0,\,R_0)$
-convergence: Under the above notion of convergence $y_\varepsilon \rightarrow (u_0,\,R_0)$![]() , the rescaled energies $({1}/{\varepsilon ^2})\mathcal {E}_\varepsilon$
, the rescaled energies $({1}/{\varepsilon ^2})\mathcal {E}_\varepsilon$![]() $\Gamma$
$\Gamma$![]() -converge to
-converge to
We also deduce strong convergence of (almost) minimizers: if $(y_\varepsilon )$![]() is a sequence of (almost) minimizers, then we have in addition that $u_\varepsilon \rightarrow u_0$
is a sequence of (almost) minimizers, then we have in addition that $u_\varepsilon \rightarrow u_0$![]() strongly in $W^{1,p}(\Omega ;\mathbb {R}^n)$
strongly in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() and the pair $(u_0,\,R_0)$
and the pair $(u_0,\,R_0)$![]() is a minimizer of $\mathcal {E}_0$
is a minimizer of $\mathcal {E}_0$![]() .
.
We now comment on the expression of the limiting energy $\mathcal {E}_0$![]() , which features two terms: the usual linear elastic energy and a potential term accounting for the surface load $-\pi (R_0x)n_{\partial \Omega }(x)$
, which features two terms: the usual linear elastic energy and a potential term accounting for the surface load $-\pi (R_0x)n_{\partial \Omega }(x)$![]() on $\partial \Omega$
on $\partial \Omega$![]() . The emergence of this boundary term can be explained by the following heuristic considerations: on the one hand, a formal linearization of (1.1) leads to a pressure load of the above form; on the other hand, if $y$
. The emergence of this boundary term can be explained by the following heuristic considerations: on the one hand, a formal linearization of (1.1) leads to a pressure load of the above form; on the other hand, if $y$![]() is smooth enough, the force term in (1.2) can be written as
is smooth enough, the force term in (1.2) can be written as
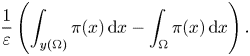
Hence, taking into account (1.3), computing the limit of the above expression on sequences $(y_\varepsilon )$![]() with equibounded energies corresponds to a sort of shape derivative of the functional $\Omega \mapsto \int _{\Omega }\pi (x)\,\mathrm {d} x$
with equibounded energies corresponds to a sort of shape derivative of the functional $\Omega \mapsto \int _{\Omega }\pi (x)\,\mathrm {d} x$![]() (see, e.g. [Reference Maggi18, proposition 17.8]). However, we stress that in the present setting deformations $y_\varepsilon$
(see, e.g. [Reference Maggi18, proposition 17.8]). However, we stress that in the present setting deformations $y_\varepsilon$![]() are only of Sobolev regularity and are close to rigid motions only in the sense of $W^{1,p}(\Omega ;\mathbb {R}^n)$
are only of Sobolev regularity and are close to rigid motions only in the sense of $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() , therefore the usual arguments in the context of shape derivatives do not apply.
, therefore the usual arguments in the context of shape derivatives do not apply.
From a mathematical viewpoint the main difference with respect to previous contributions dealing with dead loads, is that the force term in (1.2) is not a continuous perturbation of the elastic energy. Indeed, our assumptions on $W$![]() imply that deformations are at most strongly convergent in $W^{1,p}(\Omega ;\mathbb {R}^n)$
imply that deformations are at most strongly convergent in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() with $1< p\leq 2$
with $1< p\leq 2$![]() and this is not enough to guarantee convergence of the determinants. Moreover, the crucial step in the proof of compactness is to show that deformations satisfying the bound $\mathcal {E}_\varepsilon (y_\varepsilon )\leq C\varepsilon ^2$
and this is not enough to guarantee convergence of the determinants. Moreover, the crucial step in the proof of compactness is to show that deformations satisfying the bound $\mathcal {E}_\varepsilon (y_\varepsilon )\leq C\varepsilon ^2$![]() have an elastic energy of order $\varepsilon ^2$
have an elastic energy of order $\varepsilon ^2$![]() . Once this is established, one can apply the rigidity estimate by Friesecke et al. [Reference Friesecke, James and Müller13] and deduce (1.3), together with a uniform bound for $(u_\varepsilon )$
. Once this is established, one can apply the rigidity estimate by Friesecke et al. [Reference Friesecke, James and Müller13] and deduce (1.3), together with a uniform bound for $(u_\varepsilon )$![]() in $W^{1,p}(\Omega ;\mathbb {R}^n)$
in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() . In the case of dead loads deducing the $\varepsilon ^2$
. In the case of dead loads deducing the $\varepsilon ^2$![]() -bound on the elastic energy is straightforward, since the force term is linear with respect to the deformation. In our setting, instead, this is one of the main difficulties. We show that the problem can be solved under two different sets of conditions:
-bound on the elastic energy is straightforward, since the force term is linear with respect to the deformation. In our setting, instead, this is one of the main difficulties. We show that the problem can be solved under two different sets of conditions:
• $\pi$
 Lipschitz continuous in a suitable neighbourhood of $\Omega$
Lipschitz continuous in a suitable neighbourhood of $\Omega$ and nonnegative;
and nonnegative;• $\pi$
 Lipschitz continuous in a suitable neighbourhood of $\Omega$
Lipschitz continuous in a suitable neighbourhood of $\Omega$ , with a growth condition on its negative part (see ($\pi$
, with a growth condition on its negative part (see ($\pi$ 3)) and an additional coercivity property for $W(\cdot,\, F)$
3)) and an additional coercivity property for $W(\cdot,\, F)$ in terms of $\det F$
in terms of $\det F$ (see (W6)).
(see (W6)).
We note that this additional coercivity condition on $W$![]() is satisfied by a large class of elastic materials (see, e.g. [Reference Agostiniani, Dal Maso and DeSimone3, remark 2.8]).
is satisfied by a large class of elastic materials (see, e.g. [Reference Agostiniani, Dal Maso and DeSimone3, remark 2.8]).
We also observe that both the nonlinear energy (1.2) and the $\Gamma$![]() -limit $\mathcal {E}_0$
-limit $\mathcal {E}_0$![]() are well defined if $\pi$
are well defined if $\pi$![]() is merely a continuous function. However, because of the low regularity of deformations, in our proofs we need $\pi$
is merely a continuous function. However, because of the low regularity of deformations, in our proofs we need $\pi$![]() to be Lipschitz continuous, at least in a suitable neighbourhood of $\Omega$
to be Lipschitz continuous, at least in a suitable neighbourhood of $\Omega$![]() . How to extend our analysis to less regular pressure loads is an interesting question that we plan to consider in a future work.
. How to extend our analysis to less regular pressure loads is an interesting question that we plan to consider in a future work.
In the case of dead loads the set $\mathcal {R}$![]() of optimal rotations is a submanifold of $SO(n)$
of optimal rotations is a submanifold of $SO(n)$![]() and, as a consequence, one can prove that the distance of the approximating rotations $R_\varepsilon$
and, as a consequence, one can prove that the distance of the approximating rotations $R_\varepsilon$![]() in (1.3) from $\mathcal {R}$
in (1.3) from $\mathcal {R}$![]() is at most of order $\sqrt \varepsilon$
is at most of order $\sqrt \varepsilon$![]() , see [Reference Maor and Mora22]. In the last part of the paper we show that neither of these properties is true, in general, in the present setting.
, see [Reference Maor and Mora22]. In the last part of the paper we show that neither of these properties is true, in general, in the present setting.
Finally, we mention that the displacement-traction problem, where a Dirichlet condition is prescribed on a part $\partial _D\Omega$![]() of $\partial \Omega$
of $\partial \Omega$![]() and the remaining part of the boundary is subject to a pressure load, can be treated combining the techniques of this paper with the results in [Reference Agostiniani, Dal Maso and DeSimone3]. More precisely, assuming deformations $y$
and the remaining part of the boundary is subject to a pressure load, can be treated combining the techniques of this paper with the results in [Reference Agostiniani, Dal Maso and DeSimone3]. More precisely, assuming deformations $y$![]() to satisfy a boundary condition of the form $y(x)=x+\varepsilon w(x)$
to satisfy a boundary condition of the form $y(x)=x+\varepsilon w(x)$![]() for $x\in \partial _D\Omega$
for $x\in \partial _D\Omega$![]() , the compactness and $\Gamma$
, the compactness and $\Gamma$![]() -convergence results still hold with $R_0= I$
-convergence results still hold with $R_0= I$![]() and the boundary condition $u_0=w$
and the boundary condition $u_0=w$![]() on $\partial _D\Omega$
on $\partial _D\Omega$![]() . In particular, one can show that the approximating rotations $R_\varepsilon$
. In particular, one can show that the approximating rotations $R_\varepsilon$![]() in (1.3) are $\varepsilon$
in (1.3) are $\varepsilon$![]() -close to the identity, see [Reference Agostiniani, Dal Maso and DeSimone3, lemma 3.3]. Therefore, there is no need to introduce the set of optimal rotations and the analysis turns out to be simpler than the one considered here.
-close to the identity, see [Reference Agostiniani, Dal Maso and DeSimone3, lemma 3.3]. Therefore, there is no need to introduce the set of optimal rotations and the analysis turns out to be simpler than the one considered here.
Plan of the paper: In § 2 we set the problem and we state the main assumptions. In § 3 we discuss the case of a nonnegative pressure intensity $\pi$![]() . We then extend our analysis to pressures with arbitrary sign in § 4. Finally, in § 5 we compute a refined $\Gamma$
. We then extend our analysis to pressures with arbitrary sign in § 4. Finally, in § 5 we compute a refined $\Gamma$![]() -limit, which takes into account how much deformations differ from being optimal rotations, and we make a comparison with the results proved in [Reference Maor and Mora22] in the case of dead loads.
-limit, which takes into account how much deformations differ from being optimal rotations, and we make a comparison with the results proved in [Reference Maor and Mora22] in the case of dead loads.
2. Setting of the problem
2.1 Notation and preliminaries
Throughout the paper, the symbols $C$![]() or $c$
or $c$![]() will be used to denote some positive constants not depending on $\varepsilon$
will be used to denote some positive constants not depending on $\varepsilon$![]() , whose value may change from line to line.
, whose value may change from line to line.
Given two (extended) real numbers $a$![]() and $b$
and $b$![]() the notation $a\vee b$
the notation $a\vee b$![]() (respectively, $a\wedge b$
(respectively, $a\wedge b$![]() ) stands for the maximum (respectively, the minimum) between the two numbers. Given a scalar function $f$
) stands for the maximum (respectively, the minimum) between the two numbers. Given a scalar function $f$![]() , we denote its positive and negative part by $f^+$
, we denote its positive and negative part by $f^+$![]() and $f^-$
and $f^-$![]() , respectively, so that $f=f^+-f^-$
, respectively, so that $f=f^+-f^-$![]() . By $B_r\subset \mathbb {R}^n$
. By $B_r\subset \mathbb {R}^n$![]() we mean the open ball with radius $r>0$
we mean the open ball with radius $r>0$![]() centred at the origin.
centred at the origin.
Let $\Omega$![]() be an open set in $\mathbb {R}^n$
be an open set in $\mathbb {R}^n$![]() . For $p\in [1,\,\infty ]$
. For $p\in [1,\,\infty ]$![]() the norms in $L^p(\Omega )$
the norms in $L^p(\Omega )$![]() and $L^p(\Omega ;\mathbb {R}^n)$
and $L^p(\Omega ;\mathbb {R}^n)$![]() will be simply denoted by $\|\cdot \|_p$
will be simply denoted by $\|\cdot \|_p$![]() . The conjugate exponent of $p\in [1,\,\infty ]$
. The conjugate exponent of $p\in [1,\,\infty ]$![]() will be denoted by $p'$
will be denoted by $p'$![]() . The notation $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
. The notation $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() stands for the space of Sobolev functions $y\in {W}^{1,p}(\Omega ;\mathbb {R}^n)$
stands for the space of Sobolev functions $y\in {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() with zero average; if $p=2$
with zero average; if $p=2$![]() , we shall write $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
, we shall write $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)$![]() instead of $\mathring {W}^{1,2}(\Omega ;\mathbb {R}^n)$
instead of $\mathring {W}^{1,2}(\Omega ;\mathbb {R}^n)$![]() .
.
We denote by $\mathbb {R}^{n\times n}$![]() , $\mathbb {R}^{n\times n}_{\rm sym}$
, $\mathbb {R}^{n\times n}_{\rm sym}$![]() and $\mathbb {R}^{n\times n}_{\rm skew}$
and $\mathbb {R}^{n\times n}_{\rm skew}$![]() the set of $(n\times n)$
the set of $(n\times n)$![]() -matrices and the subsets of symmetric and skew-symmetric matrices, respectively. The set of rotations is denoted by $SO(n)$
-matrices and the subsets of symmetric and skew-symmetric matrices, respectively. The set of rotations is denoted by $SO(n)$![]() , namely
, namely
Finally, we recall that for every $F\in \mathbb {R}^{n\times n}$![]() and $\varepsilon >0$
and $\varepsilon >0$![]() there holds
there holds

where $\iota _k(F)$![]() is a homogeneous polynomial of degree $k$
is a homogeneous polynomial of degree $k$![]() in the entries of $F$
in the entries of $F$![]() . In particular, there exists a constant $C>0$
. In particular, there exists a constant $C>0$![]() , depending only on $n$
, depending only on $n$![]() , such that
, such that
For $k=1$![]() and $k=n$
and $k=n$![]() we have that $\iota _1(F)=\operatorname {tr} F$
we have that $\iota _1(F)=\operatorname {tr} F$![]() and $\iota _n(F)=\det F$
and $\iota _n(F)=\det F$![]() .
.
For the definition and the properties of $\Gamma$![]() -convergence we refer to the monograph [Reference Dal Maso7].
-convergence we refer to the monograph [Reference Dal Maso7].
2.2 The main assumptions
Let $\Omega \subset \mathbb {R}^n$![]() , with $n\ge 2$
, with $n\ge 2$![]() , be a bounded domain with Lipschitz boundary representing the reference configuration of a hyperelastic body. Up to a translation of the axes, we can assume without loss of generality that the origin is the barycentre of $\Omega$
, be a bounded domain with Lipschitz boundary representing the reference configuration of a hyperelastic body. Up to a translation of the axes, we can assume without loss of generality that the origin is the barycentre of $\Omega$![]() , i.e.
, i.e.
For future use we also introduce the set
which is an open annulus (if $0\not \in \Omega$![]() ; an open ball if $0\in \Omega$
; an open ball if $0\in \Omega$![]() ) centred at $0$
) centred at $0$![]() and containing $\Omega$
and containing $\Omega$![]() .
.
The stored energy density of the body is assumed to be a Carathéodory function $W\colon \Omega \times \mathbb {R}^{n\times n}\rightarrow [0,\,+\infty ]$![]() satisfying the following conditions for almost every $x\in \Omega$
satisfying the following conditions for almost every $x\in \Omega$![]() :
:
(W1) $W(x,\,F)=+\infty$
 if $\det F\leq 0$
if $\det F\leq 0$ (orientation preserving condition);
(orientation preserving condition);(W2) $W(x,\,RF)=W(x,\,F)$
 for every $F\in \mathbb {R}^{n\times n}$
for every $F\in \mathbb {R}^{n\times n}$ and $R\in SO(n)$
and $R\in SO(n)$ (frame indifference);
(frame indifference);(W3) $W(x,\,I)=0$
 (the reference configuration is stress-free);
(the reference configuration is stress-free);(W4) $W(x,\,\cdot )$
 is of class $C^2$
is of class $C^2$ in a neighbourhood of $SO(n)$
in a neighbourhood of $SO(n)$ , independent of $x$
, independent of $x$ , where the second derivatives of $W$
, where the second derivatives of $W$ are bounded, uniformly with respect to $x\in \Omega$
are bounded, uniformly with respect to $x\in \Omega$ ;
;(W5) $W(x,\,F)\ge c_1 g_p(\operatorname {dist}(F;SO(n)))$
 for every $F\in \mathbb {R}^{n\times n}$
for every $F\in \mathbb {R}^{n\times n}$ and for some $p\in (1,\,2]$
and for some $p\in (1,\,2]$ , where $g_p$
, where $g_p$ is defined as
(2.4)\begin{equation} g_p(t):=\begin{cases} \displaystyle\frac{t^2}{2} & \text{ if }t\in [0,1], \\ \displaystyle\frac{t^p}{p}+\frac 12-\frac 1p & \text{ if }t>1, \end{cases} \end{equation}and $c_1>0$
is defined as
(2.4)\begin{equation} g_p(t):=\begin{cases} \displaystyle\frac{t^2}{2} & \text{ if }t\in [0,1], \\ \displaystyle\frac{t^p}{p}+\frac 12-\frac 1p & \text{ if }t>1, \end{cases} \end{equation}and $c_1>0$
 is a constant independent of $x$
is a constant independent of $x$ (coercivity).
(coercivity).
Assumptions (W1)–(W3) are natural conditions in elasticity theory (see, e.g. [Reference Ciarlet4, Reference Kružik and Roubíček15]), assumption (W4) is the minimal regularity hypothesis needed to perform the linearization, while condition (W5) is satisfied by a large class of compressible rubber-like materials (see, e.g. [Reference Agostiniani, Blass and Koumatos2, Reference Agostiniani, Dal Maso and DeSimone3, Reference Mainini and Percivale19–Reference Mainini and Percivale21]).
We note that
Moreover, condition (W5) implies the following bound:

for a suitable constant $c>0$![]() , independent of $x$
, independent of $x$![]() . Indeed, by (W5) the bound (2.6) is satisfied for $F$
. Indeed, by (W5) the bound (2.6) is satisfied for $F$![]() outside a neighbourhood of $SO(n)$
outside a neighbourhood of $SO(n)$![]() . If instead $\operatorname {dist}(F;SO(n))$
. If instead $\operatorname {dist}(F;SO(n))$![]() is small enough, then one has
is small enough, then one has
which implies (2.6) by using again (W5).
We assume the body to be subjected to a pressure load, whose (unscaled) intensity is a Borel measurable function $\pi \colon \mathbb {R}^n\rightarrow \mathbb {R}$![]() such that
such that
(π1) $\pi$
 is Lipschitz continuous in an open set containing $\overline {\mathcal {O}}$
is Lipschitz continuous in an open set containing $\overline {\mathcal {O}}$ .
.
Since we do not prescribe any Dirichlet boundary condition, the linearization process will naturally select, as in [Reference Maor and Mora22] in the case of dead loads, a particular set of rotations that are ‘preferred’ by the force. This set is called the set of optimal rotations and in our framework it is defined as
Since the map $R\mapsto \int _\Omega \pi (Rx)\,\mathrm {d} x$![]() is continuous and $SO(n)$
is continuous and $SO(n)$![]() is compact, the set of optimal rotations is not empty and is a compact subset of $SO(n)$
is compact, the set of optimal rotations is not empty and is a compact subset of $SO(n)$![]() . For simplicity we assume that
. For simplicity we assume that
where $I$![]() is the identity matrix. Indeed, if this is not the case, we can always replace $\pi$
is the identity matrix. Indeed, if this is not the case, we can always replace $\pi$![]() by $\pi (R_0\cdot )$
by $\pi (R_0\cdot )$![]() and deformations $y$
and deformations $y$![]() by $R_0^Ty$
by $R_0^Ty$![]() , where $R_0$
, where $R_0$![]() is a given optimal rotation.
is a given optimal rotation.
Let $R_0\in \mathcal {R}$![]() . By computing the first variation of the functional in (2.7) along the curve $t\mapsto R_0{\rm e}^{tA}$
. By computing the first variation of the functional in (2.7) along the curve $t\mapsto R_0{\rm e}^{tA}$![]() with $A\in \mathbb {R}^{n\times n}_{\rm skew}$
with $A\in \mathbb {R}^{n\times n}_{\rm skew}$![]() , we deduce that any optimal rotation $R_0$
, we deduce that any optimal rotation $R_0$![]() satisfies the following Euler–Lagrange equation:
satisfies the following Euler–Lagrange equation:
Applying the divergence theorem, condition (2.9) can be rewritten as
where $n_{\partial \Omega }$![]() is the outward unit normal to $\partial \Omega$
is the outward unit normal to $\partial \Omega$![]() .
.
3. Nonnegative pressure loads
We start our analysis by considering a pressure load with nonnegative intensity, that is,
(π2) $\pi (y)\ge 0$
 for every $y\in \mathbb {R}^n$
for every $y\in \mathbb {R}^n$ .
.
This includes, for instance, the relevant case of hydrostatic pressure $\pi (y)=g\rho y_3^-$![]() , where $g$
, where $g$![]() is the gravitational constant, $\rho$
is the gravitational constant, $\rho$![]() is the constant density of the fluid and $y_3^-$
is the constant density of the fluid and $y_3^-$![]() denotes the negative part of the third component of $y$
denotes the negative part of the third component of $y$![]() .
.
For every $\varepsilon \in (0,\,1)$![]() we consider the energy $\mathcal {E}_\varepsilon \colon \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)\rightarrow (-\infty,\,+\infty ]$
we consider the energy $\mathcal {E}_\varepsilon \colon \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)\rightarrow (-\infty,\,+\infty ]$![]() defined as
defined as

where the set of admissible deformations is
In other words, admissible deformations are orientation preserving and, as it is common in Neumann boundary value problems, have zero average on $\Omega$![]() .
.
By (2.3) any rigid motion of the form
belongs to $Y^p$![]() . Moreover, under the assumption ($\pi$
. Moreover, under the assumption ($\pi$![]() 2), the energy is well defined since the two integrands $W(\cdot,\, \nabla y)$
2), the energy is well defined since the two integrands $W(\cdot,\, \nabla y)$![]() and $\pi (y)\det \nabla y$
and $\pi (y)\det \nabla y$![]() are nonnegative for $y\in Y^p$
are nonnegative for $y\in Y^p$![]() .
.
Remark 3.1 Here we do not assume deformations to be injective in any sense. However, one can easily include the requirement that admissible deformations are a.e. injective (see, e.g. [Reference Ciarlet4]), without affecting the results of the paper, see also remark 3.13.
The key ingredient in the proof of compactness is the following variant of the celebrated rigidity estimate by Friesecke et al. [Reference Friesecke, James and Müller13], whose proof can be found, e.g. in [Reference Agostiniani, Dal Maso and DeSimone3, lemma 3.1]. Similar variants of the rigidity estimates with mixed growth condition have been proved in [Reference Conti and Dolzmann5, Reference Müller and Palombaro24, Reference Scardia and Zeppieri31].
Theorem 3.2 There exists a positive constant $C=C(\Omega,\,p)>0$![]() with the following property: for every $y\in W^{1,p}(\Omega ;\mathbb {R}^n)$
with the following property: for every $y\in W^{1,p}(\Omega ;\mathbb {R}^n)$![]() there exists a constant rotation $R\in SO(n)$
there exists a constant rotation $R\in SO(n)$![]() such that
such that
The following generalized rigidity estimate will be used in theorem 3.14 to infer strong convergence of almost minimizers. For a proof we refer to [Reference Conti, Dolzmann and Müller6, theorem 1.1].
Theorem 3.3 Let $1< p_1< p_2<\infty$![]() . Then there exists a positive constant $C=C(\Omega,\,p_1,\,p_2)>0$
. Then there exists a positive constant $C=C(\Omega,\,p_1,\,p_2)>0$![]() with the following property: for every $y\in W^{1,1}(\Omega ;\mathbb {R}^n)$
with the following property: for every $y\in W^{1,1}(\Omega ;\mathbb {R}^n)$![]() with
with
there exist a constant rotation $\widetilde {R}\in SO(n)$![]() and two functions $g_i\in L^{p_i}(\Omega )$
and two functions $g_i\in L^{p_i}(\Omega )$![]() such that
such that
Our arguments will strongly rely on the Lipschitz continuity of the pressure function $\pi$![]() . This, however, holds only in a suitable set $\Omega '$
. This, however, holds only in a suitable set $\Omega '$![]() containing $\Omega$
containing $\Omega$![]() (see ($\pi$
(see ($\pi$![]() 1)). Since deformations $y\in Y^p$
1)). Since deformations $y\in Y^p$![]() may be a priori valued outside $\Omega '$
may be a priori valued outside $\Omega '$![]() , it is convenient to introduce an auxiliary Lipschitz continuous function that coincides with $\pi$
, it is convenient to introduce an auxiliary Lipschitz continuous function that coincides with $\pi$![]() in $\Omega '$
in $\Omega '$![]() and is bounded on the whole of $\mathbb {R}^n$
and is bounded on the whole of $\mathbb {R}^n$![]() . This is the content of the following lemma, which is clearly not necessary if $\pi$
. This is the content of the following lemma, which is clearly not necessary if $\pi$![]() itself is Lipschitz continuous and bounded.
itself is Lipschitz continuous and bounded.
Lemma 3.4 Assume ( $\pi$![]() 1) and ( $\pi$
1) and ( $\pi$![]() 2). Then there exists a Lipschitz continuous function $\hat{\pi} \colon \mathbb {R}^n\rightarrow [0,\,+\infty )$
2). Then there exists a Lipschitz continuous function $\hat{\pi} \colon \mathbb {R}^n\rightarrow [0,\,+\infty )$![]() , with compact support, such that $\hat{\pi}$
, with compact support, such that $\hat{\pi}$![]() coincides with $\pi$
coincides with $\pi$![]() in an open neighbourhood of $\overline {\mathcal {O}}$
in an open neighbourhood of $\overline {\mathcal {O}}$![]() and $\hat{\pi} (y)\le \pi (y)$
and $\hat{\pi} (y)\le \pi (y)$![]() for all $y\in \mathbb {R}^n$
for all $y\in \mathbb {R}^n$![]() . In particular, $\hat {\pi }$
. In particular, $\hat {\pi }$![]() is bounded.
is bounded.
Remark 3.5 Note that the set of optimal rotations (2.7) stays the same if $\pi$![]() is replaced by $\hat{\pi}$
is replaced by $\hat{\pi}$![]() , since $\pi$
, since $\pi$![]() and $\hat{\pi}$
and $\hat{\pi}$![]() coincide in a neighbourhood of $\overline {\mathcal {O}}$
coincide in a neighbourhood of $\overline {\mathcal {O}}$![]() .
.
Proof of lemma 3.4. Assume $0\not \in \Omega$![]() , so that $\mathcal {O}$
, so that $\mathcal {O}$![]() is an open annulus centred at $0$
is an open annulus centred at $0$![]() (the case where $0\in \Omega$
(the case where $0\in \Omega$![]() can be treated similarly). Let $0< r_1< r_2$
can be treated similarly). Let $0< r_1< r_2$![]() and $0<\delta < r_1$
and $0<\delta < r_1$![]() be such that $\overline {\mathcal {O}}\subset B_{r_2}\setminus \overline {B_{r_1}}$
be such that $\overline {\mathcal {O}}\subset B_{r_2}\setminus \overline {B_{r_1}}$![]() and $\pi$
and $\pi$![]() is Lipschitz continuous in $\overline {B_{r_2+\delta }}\setminus B_{r_1-\delta }$
is Lipschitz continuous in $\overline {B_{r_2+\delta }}\setminus B_{r_1-\delta }$![]() with Lipschitz constant $L$
with Lipschitz constant $L$![]() . Let $M\geq 0$
. Let $M\geq 0$![]() be the maximum of $\pi$
be the maximum of $\pi$![]() on $\partial B_{r_1}\cup \partial B_{r_2}$
on $\partial B_{r_1}\cup \partial B_{r_2}$![]() . We first define $\widetilde {\pi }: \overline {B_{r_2+\delta }}\setminus B_{r_1-\delta }\rightarrow \mathbb {R}$
. We first define $\widetilde {\pi }: \overline {B_{r_2+\delta }}\setminus B_{r_1-\delta }\rightarrow \mathbb {R}$![]() as
as

where $K:=L\vee {M}/{\delta }$![]() . It is easy to see that $\widetilde {\pi }$
. It is easy to see that $\widetilde {\pi }$![]() is Lipschitz continuous in its domain; moreover, by construction it coincides with $\pi$
is Lipschitz continuous in its domain; moreover, by construction it coincides with $\pi$![]() in an open neighbourhood of $\overline {\mathcal {O}}$
in an open neighbourhood of $\overline {\mathcal {O}}$![]() . We now show that $\widetilde {\pi }\le \pi$
. We now show that $\widetilde {\pi }\le \pi$![]() on $\overline {B_{r_2+\delta }}\setminus B_{r_1-\delta }$
on $\overline {B_{r_2+\delta }}\setminus B_{r_1-\delta }$![]() . Indeed, if $r_1-\delta \leq |y|< r_1$
. Indeed, if $r_1-\delta \leq |y|< r_1$![]() , by the Lipschitz continuity of $\pi$
, by the Lipschitz continuity of $\pi$![]() we have
we have
and similarly if $r_2\leq |y|\leq r_2+\delta$![]() . Finally, we note that, if $y\in \partial B_{r_1-\delta }\cup \partial B_{r_2+\delta }$
. Finally, we note that, if $y\in \partial B_{r_1-\delta }\cup \partial B_{r_2+\delta }$![]() , then
, then
We now conclude by considering $\hat{\pi} (y):=\widetilde {\pi }(y)\vee 0$![]() for $y\in \overline {B_{r_2+\delta }}\setminus B_{r_1-\delta }$
for $y\in \overline {B_{r_2+\delta }}\setminus B_{r_1-\delta }$![]() , $\hat{\pi} (y):=0$
, $\hat{\pi} (y):=0$![]() otherwise in $\mathbb {R}^n$
otherwise in $\mathbb {R}^n$![]() .
.
We are now in a position to state and prove some estimates which will be crucial to infer compactness of deformations with equibounded (rescaled) energy.
Lemma 3.6 Assume (W1)–(W5), ( $\pi$![]() 1), and ( $\pi$
1), and ( $\pi$![]() 2). If $\mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$
2). If $\mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$![]() for every $\varepsilon \in (0,\,1)$
for every $\varepsilon \in (0,\,1)$![]() , then there holds
, then there holds
Furthermore, there exist constant rotations $R_\varepsilon \in SO(n)$![]() such that the rescaled displacements $u_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
such that the rescaled displacements $u_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() defined by
defined by
(see (3.2) for the definition of $y_{R_\varepsilon }$![]() ) satisfy
) satisfy
and are uniformly bounded in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() .
.
If, moreover, $(R'_\varepsilon )\subset SO(n)$![]() is another sequence for which the rescaled displacements, defined as in (3.4) , satisfy (3.5) , then
is another sequence for which the rescaled displacements, defined as in (3.4) , satisfy (3.5) , then
for every $\varepsilon \in (0,\,1)$![]() .
.
Proof. Let $\widehat {\mathcal {E}}_\varepsilon$![]() be the auxiliary energy defined as in (3.1) with $\pi$
be the auxiliary energy defined as in (3.1) with $\pi$![]() replaced by the function $\hat{\pi}$
replaced by the function $\hat{\pi}$![]() given by lemma 3.4. Since $\pi \ge \hat{\pi}$
given by lemma 3.4. Since $\pi \ge \hat{\pi}$![]() everywhere and $\pi \equiv \hat{\pi}$
everywhere and $\pi \equiv \hat{\pi}$![]() on $\Omega$
on $\Omega$![]() , we have that
, we have that
For the sake of brevity we introduce the notation
Since $\widehat {\mathcal {E}}_\varepsilon (y_\varepsilon )\le \mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$![]() , we have that $y_\varepsilon$
, we have that $y_\varepsilon$![]() belongs to $Y^p$
belongs to $Y^p$![]() , hence in particular $\det \nabla y_\varepsilon >0$
, hence in particular $\det \nabla y_\varepsilon >0$![]() a.e. in $\Omega$
a.e. in $\Omega$![]() . By (W5) and theorem 3.2 we infer the existence of $R_\varepsilon \in SO(n)$
. By (W5) and theorem 3.2 we infer the existence of $R_\varepsilon \in SO(n)$![]() such that
such that
By (2.8) we deduce that

Since $\hat{\pi} \geq 0$![]() by construction, the integrand in the last integral above is nonpositive on $\Omega _\varepsilon ^+$
by construction, the integrand in the last integral above is nonpositive on $\Omega _\varepsilon ^+$![]() . Thus, using the fact that $\hat{\pi}$
. Thus, using the fact that $\hat{\pi}$![]() is Lipschitz continuous and bounded, and applying Hölder's inequality we deduce that
is Lipschitz continuous and bounded, and applying Hölder's inequality we deduce that
By (2.6) this implies
which, in turn, by Young's inequality yields
By combining (3.10) and (3.11) we deduce
and, as a consequence of (3.4), (3.9) and (3.12), we obtain
Using the definition (2.4) of $g_p$![]() this implies that
this implies that
By Hölder's inequality we obtain
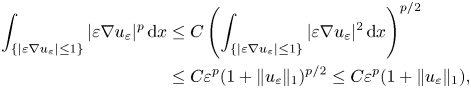
where the last inequality follows from the fact that $t^{{p}/{2}}\le 1+t$![]() for $t\ge 0$
for $t\ge 0$![]() .
.
Again from (2.4) and recalling that $p\le 2$![]() we also have that
we also have that
By (3.15), (3.16) and the continuous embedding of $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() into $L^1(\Omega ;\mathbb {R}^n)$
into $L^1(\Omega ;\mathbb {R}^n)$![]() we deduce that
we deduce that
Since $u_\varepsilon$![]() has zero average, Poincaré–Wirtinger inequality finally yields
has zero average, Poincaré–Wirtinger inequality finally yields
which implies $\|u_\varepsilon \|_{W^{1,p}}\le C$![]() . This inequality, combined with (3.11) and (3.13), provides (3.3) and (3.5).
. This inequality, combined with (3.11) and (3.13), provides (3.3) and (3.5).
Finally, if $(R_\varepsilon ')$![]() is a sequence of rotations whose corresponding rescaled displacements satisfy (3.5), then
is a sequence of rotations whose corresponding rescaled displacements satisfy (3.5), then
This concludes the proof.
As an immediate corollary we obtain that the infimum of the energy $\mathcal {E}_\varepsilon$![]() is of order $\varepsilon ^2$
is of order $\varepsilon ^2$![]() .
.
Corollary 3.7 Assume (W1)–(W5), ($\pi$![]() 1) and ($\pi$
1) and ($\pi$![]() 2). Then
2). Then
Proof. Let $(y_\varepsilon )$![]() be a minimizing sequence satisfying
be a minimizing sequence satisfying
Using the fact that $W$![]() is nonnegative and arguing as in the proof of lemma 3.6, we deduce that
is nonnegative and arguing as in the proof of lemma 3.6, we deduce that

where the last inequality follows from lemma 3.6. This proves the first inequality in (3.17).
The other inequality in (3.17) follows trivially by the fact that the energy $\mathcal {E}_\varepsilon$![]() is zero on the identity map.
is zero on the identity map.
We now have all the main ingredients to prove compactness of deformations with equibounded rescaled energies.
Proposition 3.8 (Compactness)
Assume (W1)–(W5), ($\pi$![]() 1) and ( $\pi$
1) and ( $\pi$![]() 2). If $\mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$
2). If $\mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$![]() for every $\varepsilon \in (0,\,1),$
for every $\varepsilon \in (0,\,1),$![]() then for any $R_\varepsilon,$
then for any $R_\varepsilon,$![]() $u_\varepsilon$
$u_\varepsilon$![]() given by lemma 3.6 we have that, up to subsequences,
given by lemma 3.6 we have that, up to subsequences,
• $u_\varepsilon \rightharpoonup u_0$
 weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$ with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n),$
with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n),$
• $R_\varepsilon \rightarrow R_0$
 with $R_0\in \mathcal {R},$
with $R_0\in \mathcal {R},$
as $\varepsilon \rightarrow 0$![]() . Moreover, $R_0$
. Moreover, $R_0$![]() is independent of the choice of $R_\varepsilon$
is independent of the choice of $R_\varepsilon$![]() and $u_0$
and $u_0$![]() is independent up to infinitesimal rigid motions of the form $Ax,$
is independent up to infinitesimal rigid motions of the form $Ax,$![]() with $A\in \mathbb {R}^{n\times n}_{\rm skew}$
with $A\in \mathbb {R}^{n\times n}_{\rm skew}$![]() .
.
Proof. By lemma 3.6 the sequence $(u_\varepsilon )$![]() is uniformly bounded in $W^{1,p}(\Omega ;\mathbb {R}^n)$
is uniformly bounded in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() . Hence, up to subsequences, $u_\varepsilon \rightharpoonup u_0$
. Hence, up to subsequences, $u_\varepsilon \rightharpoonup u_0$![]() weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() . We now show that $u_0$
. We now show that $u_0$![]() belongs to $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
belongs to $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)$![]() . We first introduce the set
. We first introduce the set
and we observe that by Tchebichev inequality
We claim that
(i) $\chi _{G_\varepsilon }\nabla u_\varepsilon$
 is bounded in $L^2(\Omega ;\mathbb {R}^{n\times n})$
is bounded in $L^2(\Omega ;\mathbb {R}^{n\times n})$ ;
;(ii) $\nabla u_0\in L^2(\Omega ;\mathbb {R}^{n\times n})$
 and, up to subsequences, $\chi _{G_\varepsilon }\nabla u_\varepsilon \rightharpoonup \nabla u_0$
and, up to subsequences, $\chi _{G_\varepsilon }\nabla u_\varepsilon \rightharpoonup \nabla u_0$ weakly in $L^2(\Omega ;\mathbb {R}^{n\times n})$
weakly in $L^2(\Omega ;\mathbb {R}^{n\times n})$ .
.
Assertion (i) easily follows from (3.5) arguing as in (3.14) and using that $G_\varepsilon \subset \{|\varepsilon \nabla u_\varepsilon |\le 1\}$![]() . To prove (ii) we first note that (i) ensures that $\chi _{G_\varepsilon }\nabla u_\varepsilon \rightharpoonup v$
. To prove (ii) we first note that (i) ensures that $\chi _{G_\varepsilon }\nabla u_\varepsilon \rightharpoonup v$![]() weakly in $L^2(\Omega ;\mathbb {R}^{n\times n})$
weakly in $L^2(\Omega ;\mathbb {R}^{n\times n})$![]() , up to subsequences, for some $v\in L^2(\Omega ;\mathbb {R}^{n\times n})$
, up to subsequences, for some $v\in L^2(\Omega ;\mathbb {R}^{n\times n})$![]() . On the other hand, by (3.19) we have that $\chi _{G_\varepsilon }$
. On the other hand, by (3.19) we have that $\chi _{G_\varepsilon }$![]() converges to $1$
converges to $1$![]() boundedly in measure. Since $\nabla u_\varepsilon \rightharpoonup \nabla u_0$
boundedly in measure. Since $\nabla u_\varepsilon \rightharpoonup \nabla u_0$![]() in $L^p(\Omega ;\mathbb {R}^{n\times n})$
in $L^p(\Omega ;\mathbb {R}^{n\times n})$![]() , we conclude that $\chi _{G_\varepsilon }\nabla u_\varepsilon \rightharpoonup \nabla u_0$
, we conclude that $\chi _{G_\varepsilon }\nabla u_\varepsilon \rightharpoonup \nabla u_0$![]() in $L^p(\Omega ;\mathbb {R}^{n\times n})$
in $L^p(\Omega ;\mathbb {R}^{n\times n})$![]() . Hence, $v=\nabla u_0$
. Hence, $v=\nabla u_0$![]() and (ii) is proved. By Sobolev embedding we have that $u_0\in \mathring {H}^1(\Omega ;\mathbb {R}^n)$
and (ii) is proved. By Sobolev embedding we have that $u_0\in \mathring {H}^1(\Omega ;\mathbb {R}^n)$![]() .
.
Since $SO(n)$![]() is a compact set, there exists $R_0\in SO(n)$
is a compact set, there exists $R_0\in SO(n)$![]() such that $R_\varepsilon \rightarrow R_0$
such that $R_\varepsilon \rightarrow R_0$![]() , up to subsequences. To prove that $R_0\in \mathcal {R}$
, up to subsequences. To prove that $R_0\in \mathcal {R}$![]() we argue as in the proof of lemma 3.6 and deduce
we argue as in the proof of lemma 3.6 and deduce

Note that in the last integral we used that $\hat{\pi} \equiv \pi$![]() on $\mathcal {O}$
on $\mathcal {O}$![]() .
.
Multiplying by $\varepsilon$![]() and then letting $\varepsilon \rightarrow 0$
and then letting $\varepsilon \rightarrow 0$![]() we infer that
we infer that
This implies that $R_0\in \mathcal {R}$![]() since by assumption the identity matrix is an optimal rotation.
since by assumption the identity matrix is an optimal rotation.
Uniqueness of $R_0$![]() is a straightforward consequence of (3.6). Uniqueness (up to an infinitesimal rigid motion) of $u_0$
is a straightforward consequence of (3.6). Uniqueness (up to an infinitesimal rigid motion) of $u_0$![]() follows by arguing as in [Reference Maor and Mora22, theorem 5.1], recalling that displacements have zero average in our setting.
follows by arguing as in [Reference Maor and Mora22, theorem 5.1], recalling that displacements have zero average in our setting.
The following proposition will be useful in both the liminf and the limsup inequalities to characterize the asymptotic behaviour of the rescaled pressure potential. Note that, besides the presence of $\hat{\pi}$![]() in place of $\pi$
in place of $\pi$![]() , the integral at the left-hand side of (3.20) differs from the rescaled pressure potential in the total energy whenever $R_\varepsilon$
, the integral at the left-hand side of (3.20) differs from the rescaled pressure potential in the total energy whenever $R_\varepsilon$![]() is not an optimal rotation.
is not an optimal rotation.
Proposition 3.9 Let $\hat{\pi}$![]() be a function as in lemma 3.4 and let $y_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
be a function as in lemma 3.4 and let $y_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() satisfy (3.3). Assume there exist $R_\varepsilon \in SO(n)$
satisfy (3.3). Assume there exist $R_\varepsilon \in SO(n)$![]() converging to $R_0\in \mathcal {R}$
converging to $R_0\in \mathcal {R}$![]() such that the corresponding displacements $u_\varepsilon,$
such that the corresponding displacements $u_\varepsilon,$![]() defined as in (3.4) , weakly converge in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
defined as in (3.4) , weakly converge in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() to $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
to $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$![]() . Then,
. Then,

Proof. We write

We start by considering $I_\varepsilon$![]() . Let $\Omega ^-_\varepsilon$
. Let $\Omega ^-_\varepsilon$![]() and $G_\varepsilon$
and $G_\varepsilon$![]() be defined as in (3.8) and (3.18). Since $(u_\varepsilon )$
be defined as in (3.8) and (3.18). Since $(u_\varepsilon )$![]() is bounded in $W^{1,p}(\Omega ;\mathbb {R}^n)$
is bounded in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() , property (3.19) still holds. Moreover, by (2.1)
, property (3.19) still holds. Moreover, by (2.1)

Since by (2.2) we have that for $k=1,\,\dots,\,n$![]()
we deduce that $G_\varepsilon \subset \Omega ^-_\varepsilon$![]() for $\varepsilon$
for $\varepsilon$![]() small enough. Therefore, using the nonnegativity of $\hat{\pi}$
small enough. Therefore, using the nonnegativity of $\hat{\pi}$![]() and (2.1) again, we obtain
and (2.1) again, we obtain

We first show that
Indeed, since $\hat{\pi} =\pi$![]() on $\mathcal {O}$
on $\mathcal {O}$![]() , we may write
, we may write

Using the Lipschitz continuity of $\hat {\pi }$![]() and the definition of $G_\varepsilon$
and the definition of $G_\varepsilon$![]() , the first integral at the right-hand side can be bounded as follows:
, the first integral at the right-hand side can be bounded as follows:

where the last term goes to zero, as $\varepsilon \rightarrow 0$![]() . Since $\chi _{G_\varepsilon }$
. Since $\chi _{G_\varepsilon }$![]() converges to $1$
converges to $1$![]() boundedly in measure, we have that $\chi _{G_\varepsilon }\mathrm {div}\, u_\varepsilon \rightharpoonup \mathrm {div}\, u_0$
boundedly in measure, we have that $\chi _{G_\varepsilon }\mathrm {div}\, u_\varepsilon \rightharpoonup \mathrm {div}\, u_0$![]() weakly in $L^p(\Omega )$
weakly in $L^p(\Omega )$![]() , hence the second term in (3.23) goes to zero, as well. This proves (3.22).
, hence the second term in (3.23) goes to zero, as well. This proves (3.22).
We now prove that both $J^2_\varepsilon$![]() and $J^3_\varepsilon$
and $J^3_\varepsilon$![]() converge to $0$
converge to $0$![]() , as $\varepsilon \rightarrow 0$
, as $\varepsilon \rightarrow 0$![]() . By (2.2) and (3.4), since $\hat{\pi}$
. By (2.2) and (3.4), since $\hat{\pi}$![]() is bounded, we obtain
is bounded, we obtain

Since $|\nabla u_\varepsilon |\le \varepsilon ^{-1/2}$![]() on $G_\varepsilon$
on $G_\varepsilon$![]() , we have that
, we have that

To bound $J^3_\varepsilon$![]() we use (3.3) and deduce
we use (3.3) and deduce
which vanishes by (3.19).
By combining the previous inequalities we conclude that
We now claim that
Assuming this is true, the thesis follows by (3.21), (3.24), (3.25) and the divergence theorem, since
To conclude we only need to prove (3.25). We can write the integrand in $II_\varepsilon$![]() as
as
and owing to the Lipschitz continuity of $\hat {\pi }$![]() we have
we have
Since $(u_\varepsilon )$![]() is bounded in $L^p(\Omega ;\mathbb {R}^n)$
is bounded in $L^p(\Omega ;\mathbb {R}^n)$![]() and $|\Omega \setminus \Omega _\varepsilon ^-|\leq |\Omega \setminus G_\varepsilon |\to 0$
and $|\Omega \setminus \Omega _\varepsilon ^-|\leq |\Omega \setminus G_\varepsilon |\to 0$![]() by (3.19), we deduce that
by (3.19), we deduce that
Hence, proving (3.25) is equivalent to show that
On the other hand, $u_\varepsilon \rightarrow u_0$![]() strongly in $L^1(\Omega ;\mathbb {R}^n)$
strongly in $L^1(\Omega ;\mathbb {R}^n)$![]() by compact embedding. Thus, by (3.27) and the generalized dominated convergence theorem, (3.28) is proved if we show that the integrand (3.26) converges a.e. to $\nabla \pi (R_0 x)\cdot R_0u_0(x)$
by compact embedding. Thus, by (3.27) and the generalized dominated convergence theorem, (3.28) is proved if we show that the integrand (3.26) converges a.e. to $\nabla \pi (R_0 x)\cdot R_0u_0(x)$![]() .
.
To this aim, we first note that, up to subsequences,
Indeed, the convergence is actually in $L^1(\mathcal {O};\mathbb {R}^n)$![]() . This can be easily proved by approximating $\nabla \hat{\pi}$
. This can be easily proved by approximating $\nabla \hat{\pi}$![]() with functions in $C^0(\overline {\mathcal {O}};\mathbb {R}^n)$
with functions in $C^0(\overline {\mathcal {O}};\mathbb {R}^n)$![]() . Now, by Rademacher theorem (we point out that we are working with a countable sequence of rotations $R_\varepsilon$
. Now, by Rademacher theorem (we point out that we are working with a countable sequence of rotations $R_\varepsilon$![]() ) for almost every $x\in \Omega$
) for almost every $x\in \Omega$![]() we have
we have
Since $u_\varepsilon \rightarrow u_0$![]() a.e., up to subsequences, and $\hat{\pi} \equiv \pi$
a.e., up to subsequences, and $\hat{\pi} \equiv \pi$![]() on $\mathcal {O}$
on $\mathcal {O}$![]() , we deduce the desired convergence. This concludes the proof.
, we deduce the desired convergence. This concludes the proof.
With the result of proposition 3.9 at hand, we are now in a position to state and prove the liminf and the limsup inequalities for the energy functionals $(1/{\varepsilon ^2})\mathcal {E}_\varepsilon$![]() .
.
Proposition 3.10 (Liminf inequality)
Assume (W1)–(W5), ($\pi$![]() 1) and ($\pi$
1) and ($\pi$![]() 2). For every $\varepsilon \in (0,\,1)$
2). For every $\varepsilon \in (0,\,1)$![]() let $y_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
let $y_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() be such that there exist $R_\varepsilon \in SO(n)$
be such that there exist $R_\varepsilon \in SO(n)$![]() converging to $R_0\in \mathcal {R}$
converging to $R_0\in \mathcal {R}$![]() and the corresponding displacements $u_\varepsilon$
and the corresponding displacements $u_\varepsilon$![]() , defined as in (3.4), weakly converge in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
, defined as in (3.4), weakly converge in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() to $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
to $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$![]() . Then
. Then
where $\mathcal {E}_0\colon \mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}\rightarrow \mathbb {R}$![]() is defined by
is defined by
The density $Q(x,\,\cdot )$![]() is the quadratic form given by
is the quadratic form given by
and $e(u_0)$![]() denotes the symmetric gradient of $u_0$
denotes the symmetric gradient of $u_0$![]() .
.
Remark 3.11 For any optimal rotation $R_0\in \mathcal {R}$![]() the functional $\mathcal {E}_0(\cdot,\, R_0)$
the functional $\mathcal {E}_0(\cdot,\, R_0)$![]() is invariant under perturbations by infinitesimal rigid motions. Indeed, if $u_0'(x)=u_0(x)+Ax$
is invariant under perturbations by infinitesimal rigid motions. Indeed, if $u_0'(x)=u_0(x)+Ax$![]() with $A\in \mathbb {R}^{n\times n}_{\rm skew}$
with $A\in \mathbb {R}^{n\times n}_{\rm skew}$![]() , then clearly $e(u_0')=e(u_0)$
, then clearly $e(u_0')=e(u_0)$![]() and by (2.10)
and by (2.10)
Proof of proposition 3.10. Without loss of generality we can assume
so that $y_\varepsilon \in Y^p$![]() and, up to subsequence, $\mathcal {E}_\varepsilon (y_\varepsilon )\leq C\varepsilon ^2$
and, up to subsequence, $\mathcal {E}_\varepsilon (y_\varepsilon )\leq C\varepsilon ^2$![]() . By lemma 3.6 and proposition 3.8 there exist a (possibly different) sequence $(R_\varepsilon ')\subset SO(n)$
. By lemma 3.6 and proposition 3.8 there exist a (possibly different) sequence $(R_\varepsilon ')\subset SO(n)$![]() such that, up to subsequences, $R'_\varepsilon \rightarrow R_0$
such that, up to subsequences, $R'_\varepsilon \rightarrow R_0$![]() , the corresponding displacements $u_\varepsilon '$
, the corresponding displacements $u_\varepsilon '$![]() satisfy (3.5) and, up to subsequences, weakly converge to $u_0+Ax$
satisfy (3.5) and, up to subsequences, weakly converge to $u_0+Ax$![]() for some $A\in \mathbb {R}^{n\times n}_{\rm skew}$
for some $A\in \mathbb {R}^{n\times n}_{\rm skew}$![]() . However, by remark 3.11 we can assume, without loss of generality, that $R_\varepsilon =R_\varepsilon '$
. However, by remark 3.11 we can assume, without loss of generality, that $R_\varepsilon =R_\varepsilon '$![]() and so, $u_\varepsilon =u_\varepsilon '$
and so, $u_\varepsilon =u_\varepsilon '$![]() and $A=0$
and $A=0$![]() .
.
Let $\widehat {\mathcal {E}}_\varepsilon$![]() be the auxiliary energy defined as in (3.1) with $\pi$
be the auxiliary energy defined as in (3.1) with $\pi$![]() replaced by the function $\hat{\pi}$
replaced by the function $\hat{\pi}$![]() given by lemma 3.4. By the properties of $\hat{\pi}$
given by lemma 3.4. By the properties of $\hat{\pi}$![]() we have
we have
Arguing as in [Reference Agostiniani, Dal Maso and DeSimone3, proof of theorem 2.4] one can prove that
Since condition (3.3) is satisfied by lemma 3.6, we can apply proposition 3.9 and we obtain
Finally, assumption (2.8) guarantees that the last term in (3.30) is nonnegative. This proves the desired inequality.
Proposition 3.12 (Limsup inequality)
Assume (W1)–(W5), ($\pi$![]() 1) and ( $\pi$
1) and ( $\pi$![]() 2). For every $(u_0,\,R_0)\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$
2). For every $(u_0,\,R_0)\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$![]() there exist $(u_\varepsilon,\,R_\varepsilon )\in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)\times SO(n)$
there exist $(u_\varepsilon,\,R_\varepsilon )\in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)\times SO(n)$![]() such that $u_\varepsilon \rightharpoonup u_0$
such that $u_\varepsilon \rightharpoonup u_0$![]() weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n),$
weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n),$![]() $R_\varepsilon \rightarrow R_0$
$R_\varepsilon \rightarrow R_0$![]() and, setting $y_\varepsilon (x):=R_\varepsilon (x+\varepsilon u_\varepsilon (x))$
and, setting $y_\varepsilon (x):=R_\varepsilon (x+\varepsilon u_\varepsilon (x))$![]() , there holds
, there holds
where $\mathcal {E}_0$![]() is the functional defined in (3.29).
is the functional defined in (3.29).
Proof. Let $(u_0,\,R_0)\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$![]() . By mollification there exists $(u_\varepsilon )\subset \mathring {W}^{1,\infty }(\Omega ;\mathbb {R}^n)$
. By mollification there exists $(u_\varepsilon )\subset \mathring {W}^{1,\infty }(\Omega ;\mathbb {R}^n)$![]() such that
such that
We define $R_\varepsilon :=R_0$![]() , so that $y_\varepsilon (x)=R_0(x+\varepsilon u_\varepsilon (x))$
, so that $y_\varepsilon (x)=R_0(x+\varepsilon u_\varepsilon (x))$![]() .
.
We first observe that $y_\varepsilon \in Y^p$![]() for $\varepsilon$
for $\varepsilon$![]() small enough. Indeed, by (2.3) it has zero-average and by (2.1) it satisfies
small enough. Indeed, by (2.3) it has zero-average and by (2.1) it satisfies

Since by (2.2) we have that for $k=1,\,\dots,\,n$![]()
for $\varepsilon$![]() small enough we obtain
small enough we obtain
and thus, $\det \nabla y_\varepsilon >0$![]() a.e. in $\Omega$
a.e. in $\Omega$![]() .
.
By (3.31) we have that for $\varepsilon$![]() small enough the set $y_\varepsilon (\Omega )$
small enough the set $y_\varepsilon (\Omega )$![]() is contained in the neighbourhood of $\mathcal {O}$
is contained in the neighbourhood of $\mathcal {O}$![]() where $\pi$
where $\pi$![]() and $\hat{\pi}$
and $\hat{\pi}$![]() coincide. Therefore, using also that $R_0\in \mathcal {R}$
coincide. Therefore, using also that $R_0\in \mathcal {R}$![]() , we can write
, we can write
Arguing as in [Reference Agostiniani, Dal Maso and DeSimone3, proof of theorem 2.4], one can show that
On the other hand, by (3.32) we have that
hence condition (3.3) is satisfied and we can apply proposition 3.9. By (3.33) we deduce
This concludes the proof.
Remark 3.13 If we include a.e. injectivity in the definition of the space $Y^p$![]() of admissible deformations (see remark 3.1), the limsup inequality can be proved by means of the same recovery sequence. Indeed, by [Reference Ciarlet4, theorem 5.5-1(b)] the deformations $y_\varepsilon$
of admissible deformations (see remark 3.1), the limsup inequality can be proved by means of the same recovery sequence. Indeed, by [Reference Ciarlet4, theorem 5.5-1(b)] the deformations $y_\varepsilon$![]() are a.e. injective owing to (3.31).
are a.e. injective owing to (3.31).
Combining together the previous propositions, we can prove the main result of this section. It ensures that almost minimizers of the nonlinear energy strongly converge to minimizers of the limiting energy.
Theorem 3.14 (Convergence of almost minimizers)
Assume (W1)–(W5), ($\pi$![]() 1) and ( $\pi$
1) and ( $\pi$![]() 2). If $(y_\varepsilon )$
2). If $(y_\varepsilon )$![]() is a sequence of almost minimizers for the energies $\mathcal {E}_\varepsilon$
is a sequence of almost minimizers for the energies $\mathcal {E}_\varepsilon$![]() , that is,
, that is,
then there exist $R_\varepsilon \in SO(n)$![]() such that, up to passing to a subsequence, we have
such that, up to passing to a subsequence, we have
• $u_\varepsilon \rightarrow u_0$
 strongly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
strongly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$ with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n),$
with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n),$
• $R_\varepsilon \rightarrow R_0$
 with $R_0\in \mathcal {R},$
with $R_0\in \mathcal {R},$
as $\varepsilon \to 0$![]() . Furthermore, the pair $(u_0,\,R_0)$
. Furthermore, the pair $(u_0,\,R_0)$![]() is a minimizer of $\mathcal {E}_0$
is a minimizer of $\mathcal {E}_0$![]() on $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$
on $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$![]() and
and
Proof. Let $(y_\varepsilon )$![]() be a sequence of almost minimizers. By corollary 3.7 we have that
be a sequence of almost minimizers. By corollary 3.7 we have that
hence by proposition 3.8 there exist $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$![]() and $R_0\in \mathcal {R}$
and $R_0\in \mathcal {R}$![]() such that, up to a subsequence,
such that, up to a subsequence,
We now show that $(u_0,\,R_0)$![]() is a minimizer of $\mathcal {E}_0$
is a minimizer of $\mathcal {E}_0$![]() . To this aim let $(v,\,S)\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$
. To this aim let $(v,\,S)\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$![]() and let $(v_\varepsilon,\,S_\varepsilon )$
and let $(v_\varepsilon,\,S_\varepsilon )$![]() be a recovery sequence for $(v,\,S)$
be a recovery sequence for $(v,\,S)$![]() , as in proposition 3.12. Let $z_\varepsilon (x):=S_\varepsilon (x+\varepsilon v_\varepsilon (x))$
, as in proposition 3.12. Let $z_\varepsilon (x):=S_\varepsilon (x+\varepsilon v_\varepsilon (x))$![]() . By proposition 3.10 we have
. By proposition 3.10 we have

This implies that $\mathcal {E}_0$![]() is minimized at $(u_0,\,R_0)$
is minimized at $(u_0,\,R_0)$![]() and, as a consequence, (3.35) holds.
and, as a consequence, (3.35) holds.
To conclude, it remains to prove that $u_\varepsilon \rightarrow u_0$![]() strongly in $W^{1,p}(\Omega ;\mathbb {R}^n)$
strongly in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() . We adapt the argument in [Reference Agostiniani, Dal Maso and DeSimone3, theorem 2.5] to our framework. We claim that the following properties hold:
. We adapt the argument in [Reference Agostiniani, Dal Maso and DeSimone3, theorem 2.5] to our framework. We claim that the following properties hold:
(a) $\chi _{G_\varepsilon }e(u_\varepsilon )\rightarrow e(u_0)$
 strongly in $L^2(\Omega ;\mathbb {R}^{n\times n}_{\rm sym})$
strongly in $L^2(\Omega ;\mathbb {R}^{n\times n}_{\rm sym})$ , where the set $G_\varepsilon$
, where the set $G_\varepsilon$ is defined as in (3.18);
is defined as in (3.18);(b) the sequence $(({1}/{\varepsilon ^p})\operatorname {dist}^p(\nabla y_\varepsilon ;SO(n)))$
 is equi-integrable;
is equi-integrable;(c) the sequence $(|\nabla u_\varepsilon |^p)$
 is equi-integrable.
is equi-integrable.
The thesis follows from (a) and (b), by using Vitali's convergence theorem together with Korn's second inequality, see [Reference Agostiniani, Dal Maso and DeSimone3, proof of theorem 2.5] for more details.
We now prove (a). By choosing $(v,\,S)=(u_0,\,R_0)$![]() in (3.36) we deduce
in (3.36) we deduce
By (3.30) and assumption (2.8) we have
Therefore, letting $\varepsilon \rightarrow 0$![]() and applying proposition 3.9 yield
and applying proposition 3.9 yield

On the other hand, by Taylor expansion of $W$![]() around $I$
around $I$![]() and by the weak convergence of $\chi _{G_\varepsilon }e(u_\varepsilon )$
and by the weak convergence of $\chi _{G_\varepsilon }e(u_\varepsilon )$![]() to $e(u_0)$
to $e(u_0)$![]() in $L^2(\Omega ;\mathbb {R}^{n\times n}_{\rm sym})$
in $L^2(\Omega ;\mathbb {R}^{n\times n}_{\rm sym})$![]() (see property (ii) in the proof of proposition 3.8) we obtain
(see property (ii) in the proof of proposition 3.8) we obtain

see, e.g. [Reference Agostiniani, Dal Maso and DeSimone3, Reference Dal Maso, Negri and Percivale8, Reference Maor and Mora22]. Combining the previous inequalities yields
Since $\chi _{G_\varepsilon }e(u_\varepsilon )\rightharpoonup e(u_0)$![]() weakly in $L^2(\Omega ;\mathbb {R}^{n\times n}_{\rm sym})$
weakly in $L^2(\Omega ;\mathbb {R}^{n\times n}_{\rm sym})$![]() and the quadratic form $Q(x,\,\cdot )$
and the quadratic form $Q(x,\,\cdot )$![]() is coercive on $\mathbb {R}^{n\times n}_{\rm sym}$
is coercive on $\mathbb {R}^{n\times n}_{\rm sym}$![]() by (W2), (W4) and (W5), equation (3.37) proves claim (a).
by (W2), (W4) and (W5), equation (3.37) proves claim (a).
To show claim (b) one can repeat verbatim the proof in [Reference Agostiniani, Dal Maso and DeSimone3, theorem 2.5].
We now prove claim (c). Given $\alpha >p$![]() and $\eta >0$
and $\eta >0$![]() , we deduce by (b) that there exists $M_\eta >0$
, we deduce by (b) that there exists $M_\eta >0$![]() such that, setting
such that, setting
where
we have that

Theorem 3.3 now ensures the existence of $\widetilde {R}_{\varepsilon,\eta }\in SO(n)$![]() and of $g_1^{\varepsilon,\eta },\, g_2^{\varepsilon,\eta }$
and of $g_1^{\varepsilon,\eta },\, g_2^{\varepsilon,\eta }$![]() such that
such that
Since $\nabla y_\varepsilon =R_\varepsilon +\varepsilon R_\varepsilon \nabla u_\varepsilon$![]() , we deduce that
, we deduce that
hence

where the last inequality follows from Hölder's inequality, (3.38), and (3.39).
On the other hand, by (3.40) we can write

Thus, by (3.38), (3.39) and (3.41) we have that for every measurable set $A\subset \Omega$![]()

Now, for every $\delta >0$![]() we can choose first $\eta =\eta (\delta )$
we can choose first $\eta =\eta (\delta )$![]() and then $\omega =\omega (\delta,\,\eta )$
and then $\omega =\omega (\delta,\,\eta )$![]() in such a way that the right-hand side above is less than $\delta$
in such a way that the right-hand side above is less than $\delta$![]() for every measurable set $A\subset \Omega$
for every measurable set $A\subset \Omega$![]() with $|A|<\omega$
with $|A|<\omega$![]() . This proves claim (c) and concludes the proof of the theorem.
. This proves claim (c) and concludes the proof of the theorem.
4. Pressure loads of arbitrary sign
Here we extend the results of the previous section to pressure loads whose intensity $\pi$![]() is not necessarily nonnegative (and still satisfies ($\pi$
is not necessarily nonnegative (and still satisfies ($\pi$![]() 1)). To deal with the negative part of $\pi$
1)). To deal with the negative part of $\pi$![]() we need to assume an additional bound from below for $W(\cdot,\,F)$
we need to assume an additional bound from below for $W(\cdot,\,F)$![]() in terms of $\det F$
in terms of $\det F$![]() :
:
(W6) $W(x,\,F)\ge c_2 g_q(|\det F-1|)$
 for a.e. $x\in \Omega$
for a.e. $x\in \Omega$ and for every $F\in \mathbb {R}^{n\times n}$
and for every $F\in \mathbb {R}^{n\times n}$ , for some $q\in [1,\,2]$
, for some $q\in [1,\,2]$ ,
,
where $g_q$![]() is defined as in (2.4) and $c_2>0$
is defined as in (2.4) and $c_2>0$![]() is a constant independent of $x$
is a constant independent of $x$![]() . According to the value of $q$
. According to the value of $q$![]() in (W6), we assume $\pi$
in (W6), we assume $\pi$![]() to satisfy the following condition:
to satisfy the following condition:
(π3) if $q=1$
 , $\pi ^-$
, $\pi ^-$ is bounded; if $q\in (1,\,2]$
is bounded; if $q\in (1,\,2]$ , $\pi ^-(y)\le C(1+|y|^{{p}/{q'}})$
, $\pi ^-(y)\le C(1+|y|^{{p}/{q'}})$ for every $y\in \mathbb {R}^n$
for every $y\in \mathbb {R}^n$ .
.
We note that the growth condition in ($\pi$![]() 3) is at most linear, since $p,\,q\in (1,\,2]$
3) is at most linear, since $p,\,q\in (1,\,2]$![]() implies $p/q'\in (0,\,1]$
implies $p/q'\in (0,\,1]$![]() .
.
In the current framework the energy $\mathcal {E}_\varepsilon$![]() is defined as in (3.1) with the set of admissible deformations $Y^p$
is defined as in (3.1) with the set of admissible deformations $Y^p$![]() replaced by
replaced by
Owing to ($\pi$![]() 3) the energy is well defined on $Y^p_q$
3) the energy is well defined on $Y^p_q$![]() : indeed, if $y\in Y^p_q$
: indeed, if $y\in Y^p_q$![]() , then the composition $\pi ^-\circ y$
, then the composition $\pi ^-\circ y$![]() belongs to $L^{q'}(\Omega )$
belongs to $L^{q'}(\Omega )$![]() and thus, $\pi (y)\det \nabla y$
and thus, $\pi (y)\det \nabla y$![]() is integrable. Clearly, all rigid motions $y_R$
is integrable. Clearly, all rigid motions $y_R$![]() with $R\in SO(n)$
with $R\in SO(n)$![]() are still admissible deformations. As observed in remark 3.1, also in this setting the a.e. injectivity condition can be included in the definition of $Y^p_q$
are still admissible deformations. As observed in remark 3.1, also in this setting the a.e. injectivity condition can be included in the definition of $Y^p_q$![]() without altering the results of this section.
without altering the results of this section.
As in the previous section we need a Lipschitz continuous function that extends $\pi$![]() outside a neighbourhood of $\overline {\mathcal {O}}$
outside a neighbourhood of $\overline {\mathcal {O}}$![]() , is below $\pi$
, is below $\pi$![]() everywhere, and satisfies the same growth condition ($\pi$
everywhere, and satisfies the same growth condition ($\pi$![]() 3) as $\pi$
3) as $\pi$![]() .
.
Lemma 4.1 Assume conditions ( $\pi$![]() 1) and ( $\pi$
1) and ( $\pi$![]() 3). Then, there exists a Lipschitz continuous function $\hat{\pi} \colon \mathbb {R}^n\rightarrow \mathbb {R}$
3). Then, there exists a Lipschitz continuous function $\hat{\pi} \colon \mathbb {R}^n\rightarrow \mathbb {R}$![]() such that $\hat{\pi}$
such that $\hat{\pi}$![]() coincides with $\pi$
coincides with $\pi$![]() in a neighbourhood of $\overline {\mathcal {O}},$
in a neighbourhood of $\overline {\mathcal {O}},$![]() $\hat{\pi} (y)\le \pi (y)$
$\hat{\pi} (y)\le \pi (y)$![]() for all $y\in \mathbb {R}^n,$
for all $y\in \mathbb {R}^n,$![]() and $\hat{\pi}$
and $\hat{\pi}$![]() has the following property: if $q=1,$
has the following property: if $q=1,$![]() $\hat{\pi}$
$\hat{\pi}$![]() is bounded; if $q\in (1,\,2],$
is bounded; if $q\in (1,\,2],$![]() $|\hat{\pi} (y)|\le C(1+|y|^{{p}/{q'}})$
$|\hat{\pi} (y)|\le C(1+|y|^{{p}/{q'}})$![]() for every $y\in \mathbb {R}^n$
for every $y\in \mathbb {R}^n$![]() .
.
Proof. We consider only the case $q\in (1,\,2]$![]() , being the case $q=1$
, being the case $q=1$![]() analogous and even simpler. Let $\overline {C}>0$
analogous and even simpler. Let $\overline {C}>0$![]() be a constant for which ($\pi$
be a constant for which ($\pi$![]() 3) is satisfied and let
3) is satisfied and let
Since $p/q'\in (0,\,1]$![]() , the function $h$
, the function $h$![]() is Lipschitz continuous in the whole of $\mathbb {R}^n$
is Lipschitz continuous in the whole of $\mathbb {R}^n$![]() and $\pi \ge -h$
and $\pi \ge -h$![]() by ($\pi$
by ($\pi$![]() 3). Hence we can apply lemma 3.4 to the function $\Pi :=\pi +h$
3). Hence we can apply lemma 3.4 to the function $\Pi :=\pi +h$![]() . This provides us with a function $\widehat \Pi$
. This provides us with a function $\widehat \Pi$![]() . It is now easy to check that the function $\hat {\pi }:=\widehat \Pi -h$
. It is now easy to check that the function $\hat {\pi }:=\widehat \Pi -h$![]() has all the required properties.
has all the required properties.
Under this new set of assumptions, the results of § 3 can be modified as follows.
Lemma 4.2 Assume (W1)–(W6), ($\pi$![]() 1) and ($\pi$
1) and ($\pi$![]() 3). Then there exists $\varepsilon _0\in (0,\,1)$
3). Then there exists $\varepsilon _0\in (0,\,1)$![]() such that, if $\mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$
such that, if $\mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$![]() for every $\varepsilon \in (0,\,\varepsilon _0),$
for every $\varepsilon \in (0,\,\varepsilon _0),$![]() then there holds
then there holds
Furthermore, there exist constant rotations $R_\varepsilon \in SO(n)$![]() such that the rescaled displacements $u_\varepsilon$
such that the rescaled displacements $u_\varepsilon$![]() defined by (3.4) satisfy
defined by (3.4) satisfy
and are uniformly bounded in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() .
.
If, moreover, $(R'_\varepsilon )\subset SO(n)$![]() is another sequence for which the rescaled displacements, defined as in (3.4), satisfy (4.3), then
is another sequence for which the rescaled displacements, defined as in (3.4), satisfy (4.3), then
for every $\varepsilon \in (0,\,\varepsilon _0)$![]() . Finally, one has
. Finally, one has
Proof. The choice of $\varepsilon _0$![]() will be made throughout the proof. We follow the lines of the proof of lemma 3.6. By lemma 4.1 inequality (3.7) still holds. By (W5) and theorem 3.2 there exists a sequence $(R_\varepsilon )\subset SO(n)$
will be made throughout the proof. We follow the lines of the proof of lemma 3.6. By lemma 4.1 inequality (3.7) still holds. By (W5) and theorem 3.2 there exists a sequence $(R_\varepsilon )\subset SO(n)$![]() such that
such that
and
We denote by $P_\varepsilon$![]() the last term in the above inequality. In the following, $c_2$
the last term in the above inequality. In the following, $c_2$![]() is the constant in condition (W6). If $q=1$
is the constant in condition (W6). If $q=1$![]() , the function $\hat{\pi}$
, the function $\hat{\pi}$![]() is bounded and thus, recalling the definition (3.8) of the sets $\Omega _\varepsilon ^\pm$
is bounded and thus, recalling the definition (3.8) of the sets $\Omega _\varepsilon ^\pm$![]() , we have
, we have

where we used Cauchy's inequality and (2.5). If instead $q\in (1,\,2]$![]() , the function $\hat{\pi}$
, the function $\hat{\pi}$![]() is Lipschitz continuous and satisfies a $p/q'$
is Lipschitz continuous and satisfies a $p/q'$![]() -growth condition with $p/q'\leq 1$
-growth condition with $p/q'\leq 1$![]() , so that we obtain
, so that we obtain

Using that $|\det \nabla y_\varepsilon -1|\leq 1$![]() on $\Omega _\varepsilon ^-$
on $\Omega _\varepsilon ^-$![]() and applying Hölder's inequality, from the previous equation we deduce
and applying Hölder's inequality, from the previous equation we deduce

where we used Young's inequality, (2.5) and the fact that $q'\geq 2$![]() . Combining (4.4) with the previous bounds on $P_\varepsilon$
. Combining (4.4) with the previous bounds on $P_\varepsilon$![]() , we obtain that
, we obtain that

in both cases $q=1$![]() and $q\in (1,\,2]$
and $q\in (1,\,2]$![]() .
.
Now, if $\varepsilon _0\le c_2/(4\overline {C})$![]() , where $\overline {C}$
, where $\overline {C}$![]() is a constant for which (4.5) is true, then by (W6) we deduce that
is a constant for which (4.5) is true, then by (W6) we deduce that
for every $\varepsilon \in (0,\,\varepsilon _0)$![]() . Combining (4.6) and (4.5) yields
. Combining (4.6) and (4.5) yields

Arguing as in (3.14)–(3.16) and using Poincaré–Wirtinger inequality we deduce that
Up to choosing $\varepsilon _0$![]() smaller, if needed, we obtain
smaller, if needed, we obtain
which implies $\|u_\varepsilon \|_{W^{1,p}}\le C$![]() .
.
Inequality (4.2) now follows easily from (4.6), while (4.3) is a consequence of (4.7) and (W5). The last two statements of the lemma can be proved arguing exactly as in the proofs of lemma 3.6 and corollary 3.7.
The proof of the following compactness result is completely analogous to that of proposition 3.8.
Proposition 4.3 (Compactness)
Assume (W1)–(W6), ($\pi$![]() 1) and ($\pi$
1) and ($\pi$![]() 3). If $\mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$
3). If $\mathcal {E}_\varepsilon (y_\varepsilon )\le C\varepsilon ^2$![]() for $\varepsilon \in (0,\,\varepsilon _0),$
for $\varepsilon \in (0,\,\varepsilon _0),$![]() then for any $R_\varepsilon,$
then for any $R_\varepsilon,$![]() $u_\varepsilon$
$u_\varepsilon$![]() given by lemma 4.2, we have that, up to subsequences,
given by lemma 4.2, we have that, up to subsequences,
• $u_\varepsilon \rightharpoonup u_0$
 weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$ with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$ ,
,• $R_\varepsilon \rightarrow R_0$
 with $R_0\in \mathcal {R}$
with $R_0\in \mathcal {R}$ ,
,
as $\varepsilon \rightarrow 0$![]() . Moreover, $R_0$
. Moreover, $R_0$![]() is independent of the choice of $R_\varepsilon$
is independent of the choice of $R_\varepsilon$![]() and $u_0$
and $u_0$![]() is independent up to infinitesimal rigid motions of the form $Ax$
is independent up to infinitesimal rigid motions of the form $Ax$![]() , with $A\in \mathbb {R}^{n\times n}_{\rm skew}$
, with $A\in \mathbb {R}^{n\times n}_{\rm skew}$![]() .
.
The next proposition is the analogue of proposition 3.9. However, in the present setting, owing to the assumptions (W6) and ($\pi$![]() 3), we can improve the result and show convergence on the whole of $\Omega$
3), we can improve the result and show convergence on the whole of $\Omega$![]() .
.
Proposition 4.4 Let $\hat{\pi}$![]() be a function as in lemma 4.1 and let $y_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
be a function as in lemma 4.1 and let $y_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() satisfy (4.2). Assume there exist $R_\varepsilon \in SO(n)$
satisfy (4.2). Assume there exist $R_\varepsilon \in SO(n)$![]() converging to $R_0\in \mathcal {R}$
converging to $R_0\in \mathcal {R}$![]() such that the corresponding displacements $u_\varepsilon,$
such that the corresponding displacements $u_\varepsilon,$![]() defined as in (3.4) , weakly converge in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
defined as in (3.4) , weakly converge in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() to $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
to $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$![]() . Then,
. Then,
Proof. We follow the lines of the proof of proposition 3.9. The only difference is in the analysis of the term $I_\varepsilon$![]() in (3.21), which now can be written as
in (3.21), which now can be written as

where the sets $\Omega _\varepsilon ^{\pm }$![]() and $G_\varepsilon$
and $G_\varepsilon$![]() are defined as in (3.8) and (3.18). Here we recall that $G_\varepsilon \subset \Omega ^-_\varepsilon$
are defined as in (3.8) and (3.18). Here we recall that $G_\varepsilon \subset \Omega ^-_\varepsilon$![]() for $\varepsilon$
for $\varepsilon$![]() small enough. The first integral $\tilde {J}^1_\varepsilon$
small enough. The first integral $\tilde {J}^1_\varepsilon$![]() can be handled exactly as in the proof of proposition 3.9. To conclude it is enough to show that the remaining terms are infinitesimal, as $\varepsilon \to 0$
can be handled exactly as in the proof of proposition 3.9. To conclude it is enough to show that the remaining terms are infinitesimal, as $\varepsilon \to 0$![]() .
.
By (2.2) and the Lipschitz continuity of $\hat{\pi}$![]() we obtain
we obtain

Since $|\nabla u_\varepsilon |\le \varepsilon ^{-1/2}$![]() on $G_\varepsilon$
on $G_\varepsilon$![]() , we have that
, we have that

Using (4.2), the boundedness of $(u_\varepsilon )$![]() in $W^{1,p}(\Omega ;\mathbb {R}^n)$
in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() , and recalling that $|\det \nabla y_\varepsilon {-}1|\leq 1$
, and recalling that $|\det \nabla y_\varepsilon {-}1|\leq 1$![]() on $\Omega _\varepsilon ^-$
on $\Omega _\varepsilon ^-$![]() , we deduce that
, we deduce that

where the last term goes to zero, as $\varepsilon \rightarrow 0$![]() , by (3.19).
, by (3.19).
To deal with $\tilde {J}^4_\varepsilon$![]() we consider the two cases $q=1$
we consider the two cases $q=1$![]() and $q\in (1,\,2]$
and $q\in (1,\,2]$![]() , separately. If $q=1$
, separately. If $q=1$![]() , the function $\hat{\pi}$
, the function $\hat{\pi}$![]() is bounded and so, we have
is bounded and so, we have
where in the last inequality we used (4.2).
If instead $q\in (1,\,2]$![]() , by using the $p/q'$
, by using the $p/q'$![]() -growth of $\hat{\pi}$
-growth of $\hat{\pi}$![]() we have
we have

By (4.2) and the boundedness of $(u_\varepsilon )$![]() in $W^{1,p}(\Omega ;\mathbb {R}^n)$
in $W^{1,p}(\Omega ;\mathbb {R}^n)$![]() we deduce
we deduce
It is easy to verify that ${2}/{q}+{p}/{q'}-1>0$![]() . Moreover, since $G_\varepsilon \subset \Omega _\varepsilon ^-$
. Moreover, since $G_\varepsilon \subset \Omega _\varepsilon ^-$![]() for $\varepsilon$
for $\varepsilon$![]() small enough, we have that $|\Omega _\varepsilon ^+|\to 0$
small enough, we have that $|\Omega _\varepsilon ^+|\to 0$![]() by (3.19). Therefore, we conclude that in both cases $\widetilde {J}^4_\varepsilon$
by (3.19). Therefore, we conclude that in both cases $\widetilde {J}^4_\varepsilon$![]() is infinitesimal, as $\varepsilon \rightarrow 0$
is infinitesimal, as $\varepsilon \rightarrow 0$![]() .
.
The next proposition collects both the liminf and the limsup inequalities, which thus provide a full $\Gamma$![]() -convergence result.
-convergence result.
Proposition 4.5 (Liminf and limsup inequalities)
Assume (W1)–(W6), ($\pi$![]() 1) and ($\pi$
1) and ($\pi$![]() 3). For every $\varepsilon \in (0,\,\varepsilon _0)$
3). For every $\varepsilon \in (0,\,\varepsilon _0)$![]() let $y_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
let $y_\varepsilon \in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() be such that there exist $R_\varepsilon \in SO(n)$
be such that there exist $R_\varepsilon \in SO(n)$![]() converging to $R_0\in \mathcal {R}$
converging to $R_0\in \mathcal {R}$![]() and the corresponding displacements $u_\varepsilon,$
and the corresponding displacements $u_\varepsilon,$![]() defined as in (3.4) , weakly converge in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
defined as in (3.4) , weakly converge in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() to $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
to $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$![]() . Then
. Then
where $\mathcal {E}_0$![]() is the functional defined in (3.29).
is the functional defined in (3.29).
On the other hand, for every $(u_0,\,R_0)\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$![]() there exist $(u_\varepsilon,\,R_\varepsilon )\in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)\times SO(n)$
there exist $(u_\varepsilon,\,R_\varepsilon )\in \mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)\times SO(n)$![]() such that $u_\varepsilon \rightharpoonup u_0$
such that $u_\varepsilon \rightharpoonup u_0$![]() weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n),$
weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n),$![]() $R_\varepsilon \rightarrow R_0$
$R_\varepsilon \rightarrow R_0$![]() and, setting $y_\varepsilon (x):=R_\varepsilon (x+\varepsilon u_\varepsilon (x))$
and, setting $y_\varepsilon (x):=R_\varepsilon (x+\varepsilon u_\varepsilon (x))$![]() , there holds
, there holds
Proof. The proof is the same of propositions 3.10 and 3.12, once we have at our disposal proposition 4.4. The only additional remark is that the deformations $y_\varepsilon$![]() in the recovery sequence are admissible since $\det \nabla y_\varepsilon \in L^\infty (\Omega )$
in the recovery sequence are admissible since $\det \nabla y_\varepsilon \in L^\infty (\Omega )$![]() by construction (see (4.1) for the definition of $Y^p_q$
by construction (see (4.1) for the definition of $Y^p_q$![]() ).
).
Combining the previous results and arguing as in theorem 3.14, one can infer the following convergence result for almost minimizers.
Theorem 4.6 (Convergence of almost minimizers)
Assume (W1)–(W6), ($\pi$![]() 1) and ($\pi$
1) and ($\pi$![]() 3). If $(y_\varepsilon )$
3). If $(y_\varepsilon )$![]() is a sequence of almost minimizers for the energies $\mathcal {E}_\varepsilon$
is a sequence of almost minimizers for the energies $\mathcal {E}_\varepsilon$![]() , that is,
, that is,
then there exist $R_\varepsilon \in SO(n)$![]() such that, up to passing to a subsequence, we have
such that, up to passing to a subsequence, we have
• $u_\varepsilon \rightarrow u_0$
 strongly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
strongly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$ with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$ ;
;• $R_\varepsilon \rightarrow R_0$
 with $R_0\in \mathcal {R}$
with $R_0\in \mathcal {R}$ .
.
Furthermore, the pair $(u_0,\,R_0)$![]() is a minimizer of $\mathcal {E}_0$
is a minimizer of $\mathcal {E}_0$![]() on $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$
on $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$![]() and
and
5. A refined $\Gamma$ -limit and a comparison with dead loads
-limit and a comparison with dead loads
In this section we make a comparison with the results obtained in [Reference Maor and Mora22], in the case of dead loads. In particular, we compute a refined version of the $\Gamma$![]() -limit of the rescaled energies $(1/{\varepsilon ^2})\mathcal {E}_\varepsilon$
-limit of the rescaled energies $(1/{\varepsilon ^2})\mathcal {E}_\varepsilon$![]() .
.
We assume (W1)–(W5) and ($\pi$![]() 1), together with either ($\pi$
1), together with either ($\pi$![]() 2) or ($\pi$
2) or ($\pi$![]() 3) and (W6). In addition, we require
3) and (W6). In addition, we require
(π4) $\pi$
 is of class $C^2$
is of class $C^2$ in the open set given by ($\pi$
in the open set given by ($\pi$ 1).
1).
Under this assumption any optimal rotation $R_0\in \mathcal {R}$![]() satisfies, in addition to (2.9), the following condition:
satisfies, in addition to (2.9), the following condition:
This is obtained by imposing that the second variation of the functional in (2.7) is positive semidefinite along the curve $t\mapsto R_0{\rm e}^{tA}$![]() . By the divergence theorem, condition (5.1) can be rewritten as
. By the divergence theorem, condition (5.1) can be rewritten as
In [Reference Maor and Mora22] the applied body force is assumed to be a dead load of the form
where $g\in L^2(\Omega ;\mathbb {R}^n)$![]() is given. In this setting the authors proved that the set of optimal rotations, which is defined as
is given. In this setting the authors proved that the set of optimal rotations, which is defined as
is a submanifold of $SO(n)$![]() (see [Reference Maor and Mora22, proposition 4.1]). Moreover, if $(y_\varepsilon )$
(see [Reference Maor and Mora22, proposition 4.1]). Moreover, if $(y_\varepsilon )$![]() is a sequence of deformations with total energy of order $\varepsilon ^2$
is a sequence of deformations with total energy of order $\varepsilon ^2$![]() , then any sequence of rotations $(R_\varepsilon )$
, then any sequence of rotations $(R_\varepsilon )$![]() provided by the rigidity estimate converges to an optimal rotation $R_0$
provided by the rigidity estimate converges to an optimal rotation $R_0$![]() (as in propositions 3.8 and 4.3) and, in addition, satisfies
(as in propositions 3.8 and 4.3) and, in addition, satisfies
where $\operatorname {dist}_{SO(n)}$![]() is the intrinsic distance in $SO(n)$
is the intrinsic distance in $SO(n)$![]() , that is,
, that is,
Finally, the $\Gamma$![]() -limit of the rescaled energies can be expressed as
-limit of the rescaled energies can be expressed as
where $u_0$![]() is the limit displacement, $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$
is the limit displacement, $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$![]() is the limit of
is the limit of
(which exists up to subsequences), and $\mathcal {P}_{\!g}$![]() is the projection operator on $\mathcal {R}_g$
is the projection operator on $\mathcal {R}_g$![]() (see [Reference Maor and Mora22, § 5]). We note that the last term in (5.5) is the second variation of the functional in (5.3) at $R_0$
(see [Reference Maor and Mora22, § 5]). We note that the last term in (5.5) is the second variation of the functional in (5.3) at $R_0$![]() computed in the direction $A_0$
computed in the direction $A_0$![]() . In [Reference Maor and Mora22] it is then proved that $A_0=0$
. In [Reference Maor and Mora22] it is then proved that $A_0=0$![]() for sequences $(y_\varepsilon )$
for sequences $(y_\varepsilon )$![]() of almost minimizers, so that the last term in (5.5) is identically equal to $0$
of almost minimizers, so that the last term in (5.5) is identically equal to $0$![]() on minimizers.
on minimizers.
In our setting of a pressure live load, a first difference with [Reference Maor and Mora22] is that the set of optimal rotations may not be a manifold, as the following example shows.
Example 5.1 Let $n=2$![]() and let $\Omega$
and let $\Omega$![]() be the set given in polar coordinates by
be the set given in polar coordinates by
(see figure 1). We note that (2.3) is satisfied.
FIG. 1. Set $\Omega$![]() in example 5.1.
in example 5.1.
Let $\varphi \in C^3([0,\,\pi /2])$![]() be a nonnegative function satisfying $\varphi (0)=0$
be a nonnegative function satisfying $\varphi (0)=0$![]() and attaining its maximum at $\alpha =\pi /2$
and attaining its maximum at $\alpha =\pi /2$![]() . Suppose, in addition, that the first, second and third derivatives of $\varphi$
. Suppose, in addition, that the first, second and third derivatives of $\varphi$![]() vanish at $\alpha =0$
vanish at $\alpha =0$![]() and at $\alpha =\pi /2$
and at $\alpha =\pi /2$![]() . Let $\psi \in C^2([1,\,+\infty ))$
. Let $\psi \in C^2([1,\,+\infty ))$![]() be a bounded function with bounded first and second derivatives satisfying $\psi (1)=\psi '(1)=\psi ''(1)=0$
be a bounded function with bounded first and second derivatives satisfying $\psi (1)=\psi '(1)=\psi ''(1)=0$![]() and $\int _{1}^{2}\rho \psi (\rho )\,\mathrm {d}\rho =1$
and $\int _{1}^{2}\rho \psi (\rho )\,\mathrm {d}\rho =1$![]() . A simple choice of $\psi$
. A simple choice of $\psi$![]() could be $\psi (\rho )=({20}/{9})(\rho -1)^3$
could be $\psi (\rho )=({20}/{9})(\rho -1)^3$![]() for $\rho \in [1,\,2]$
for $\rho \in [1,\,2]$![]() , suitably extended to $[2,\,+\infty )$
, suitably extended to $[2,\,+\infty )$![]() .
.
We consider the following pressure function:

Elementary computations show that $\pi$![]() is Lipschitz continuous and of class $C^2(\mathbb {R}^n)$
is Lipschitz continuous and of class $C^2(\mathbb {R}^n)$![]() , so that ($\pi$
, so that ($\pi$![]() 1) and ($\pi$
1) and ($\pi$![]() 4) are satisfied; furthermore, $\pi$
4) are satisfied; furthermore, $\pi$![]() is bounded, so also ($\pi$
is bounded, so also ($\pi$![]() 3) holds true.
3) holds true.
We recall that $SO(2)$![]() can be identified with the unit sphere $\mathbb {S}^1$
can be identified with the unit sphere $\mathbb {S}^1$![]() via the map
via the map
so that we can write
For $\alpha \in [0,\,\pi /2)$![]() we can compute
we can compute

Similarly, we obtain

Since $\varphi$![]() is nonnegative and maximized at $\pi /2$
is nonnegative and maximized at $\pi /2$![]() , the set of optimal rotations corresponds to the zero-level set of the function $\phi$
, the set of optimal rotations corresponds to the zero-level set of the function $\phi$![]() . In particular, in $[0,\,\pi /2)$
. In particular, in $[0,\,\pi /2)$![]() this is given by the zero-level set of $\varphi$
this is given by the zero-level set of $\varphi$![]() , which can be any closed set at positive distance from $\pi /2$
, which can be any closed set at positive distance from $\pi /2$![]() .
.
This example shows that, in general, we cannot expect $\mathcal {R}$![]() to be a manifold.
to be a manifold.
Since $\mathcal {R}$![]() is not in general a manifold, the projection operator on $\mathcal {R}$
is not in general a manifold, the projection operator on $\mathcal {R}$![]() is not well defined. However, in the limiting process we can keep track of the distance of the approximating rotations $R_\varepsilon$
is not well defined. However, in the limiting process we can keep track of the distance of the approximating rotations $R_\varepsilon$![]() from $\mathcal {R}$
from $\mathcal {R}$![]() through a suitable sequence of skew-symmetric matrices $A_\varepsilon$
through a suitable sequence of skew-symmetric matrices $A_\varepsilon$![]() . In contrast with (5.4), the scaling of this distance may be larger than $\sqrt \varepsilon$
. In contrast with (5.4), the scaling of this distance may be larger than $\sqrt \varepsilon$![]() (actually, larger than $\sqrt [k]{\varepsilon }$
(actually, larger than $\sqrt [k]{\varepsilon }$![]() for any given $k>2$
for any given $k>2$![]() ), see example 5.3. To recover compactness of $(A_\varepsilon )$
), see example 5.3. To recover compactness of $(A_\varepsilon )$![]() we rescale it by $|A_\varepsilon |\vee \sqrt {\varepsilon }$
we rescale it by $|A_\varepsilon |\vee \sqrt {\varepsilon }$![]() and we denote by $A_0$
and we denote by $A_0$![]() its limit. The $\Gamma$
its limit. The $\Gamma$![]() -limit of the rescaled energies can be then expressed as
-limit of the rescaled energies can be then expressed as
where $\mathcal {F}\colon \mathcal {R}\times \mathbb {R}^{n\times n}_{\rm skew}\rightarrow [0,\,+\infty )$![]() is the second variation of the functional in (2.7). This additional term measures the cost due to the fluctuations of the approximating rotations from the set $\mathcal {R}$
is the second variation of the functional in (2.7). This additional term measures the cost due to the fluctuations of the approximating rotations from the set $\mathcal {R}$![]() . Arguing as in (5.1) and (5.2), the functional $\mathcal {F}$
. Arguing as in (5.1) and (5.2), the functional $\mathcal {F}$![]() takes the form
takes the form
For sequences $(y_\varepsilon )$![]() of almost minimizers the limit $A_0$
of almost minimizers the limit $A_0$![]() may be different from $0$
may be different from $0$![]() ; however, we have $\mathcal {F}(R_0,\,A_0)=0$
; however, we have $\mathcal {F}(R_0,\,A_0)=0$![]() . More precisely, we have the following result.
. More precisely, we have the following result.
Theorem 5.2 Under the assumptions of propositions 3.8 or 4.3 , we have in addition that there exist $A_\varepsilon \in \mathbb {R}^{n\times n}_{\rm skew}$![]() such that $R_\varepsilon =S_\varepsilon {\rm e}^{A_\varepsilon }$
such that $R_\varepsilon =S_\varepsilon {\rm e}^{A_\varepsilon }$![]() for some $S_\varepsilon \in \mathcal {R}$
for some $S_\varepsilon \in \mathcal {R}$![]() and, up to subsequences, $A_\varepsilon \rightarrow 0$
and, up to subsequences, $A_\varepsilon \rightarrow 0$![]() and ${A_\varepsilon }/({|A_\varepsilon |\vee \sqrt {\varepsilon }})\rightarrow A_0$
and ${A_\varepsilon }/({|A_\varepsilon |\vee \sqrt {\varepsilon }})\rightarrow A_0$![]() for some $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$
for some $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$![]() with $|A_0|\le 1$
with $|A_0|\le 1$![]() .
.
If also ($\pi$![]() 4) is in force, then
4) is in force, then
with respect to the following convergences: $u_\varepsilon \rightharpoonup u_0$![]() weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
weakly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$![]() , $R_\varepsilon \rightarrow R_0$
, $R_\varepsilon \rightarrow R_0$![]() , $A_\varepsilon \rightarrow 0$
, $A_\varepsilon \rightarrow 0$![]() , and ${A_\varepsilon }/({|A_\varepsilon |\vee \sqrt {\varepsilon }})\rightarrow A_0$
, and ${A_\varepsilon }/({|A_\varepsilon |\vee \sqrt {\varepsilon }})\rightarrow A_0$![]() .
.
Finally, if $(y_\varepsilon )$![]() is a sequence of almost minimizers for the energies $\mathcal {E}_\varepsilon$
is a sequence of almost minimizers for the energies $\mathcal {E}_\varepsilon$![]() , that is, (3.34) holds, then there exist $R_\varepsilon \in SO(n)$
, that is, (3.34) holds, then there exist $R_\varepsilon \in SO(n)$![]() and $A_\varepsilon \in \mathbb {R}^{n\times n}_{\rm skew}$
and $A_\varepsilon \in \mathbb {R}^{n\times n}_{\rm skew}$![]() as above such that, up to a subsequence, we have
as above such that, up to a subsequence, we have
• $u_\varepsilon \rightarrow u_0$
 strongly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$
strongly in $\mathring {W}^{1,p}(\Omega ;\mathbb {R}^n)$ with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$
with $u_0\in \mathring {H}^{1}(\Omega ;\mathbb {R}^n)$ ,
,• $R_\varepsilon \rightarrow R_0$
 with $R_0\in \mathcal {R}$
with $R_0\in \mathcal {R}$ ,
,• $A_\varepsilon \rightarrow 0$
 and ${A_\varepsilon }/({|A_\varepsilon |\vee \sqrt {\varepsilon }})\rightarrow A_0$
and ${A_\varepsilon }/({|A_\varepsilon |\vee \sqrt {\varepsilon }})\rightarrow A_0$ with $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$
with $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$ , $|A_0|\le 1$
, $|A_0|\le 1$ .
.
Furthermore, the triplet $(u_0,\,R_0,\,A_0)$![]() is a minimizer of $\mathcal {E}_0+(1/2)\mathcal {F}$
is a minimizer of $\mathcal {E}_0+(1/2)\mathcal {F}$![]() on $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}\times \mathbb {R}^{n\times n}_{\rm skew}$
on $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}\times \mathbb {R}^{n\times n}_{\rm skew}$![]() , $\mathcal {F}(R_0,\,A_0)=0$
, $\mathcal {F}(R_0,\,A_0)=0$![]() , and
, and

Proof. As for the compactness statement, since $\mathcal {R}$![]() is a closed set, there exist $S_\varepsilon \in \mathcal {R}$
is a closed set, there exist $S_\varepsilon \in \mathcal {R}$![]() and $A_\varepsilon \in \mathbb {R}^{n\times n}_{\rm skew}$
and $A_\varepsilon \in \mathbb {R}^{n\times n}_{\rm skew}$![]() such that $R_\varepsilon =S_\varepsilon {\rm e}^{A_\varepsilon }$
such that $R_\varepsilon =S_\varepsilon {\rm e}^{A_\varepsilon }$![]() and
and
Since, up to subsequences, $R_\varepsilon \rightarrow R_0\in \mathcal {R}$![]() , we have that $A_\varepsilon \rightarrow 0$
, we have that $A_\varepsilon \rightarrow 0$![]() . The remaining properties follow from the fact the sequence $({A_\varepsilon }/({|A_\varepsilon |\vee \sqrt {\varepsilon }}))$
. The remaining properties follow from the fact the sequence $({A_\varepsilon }/({|A_\varepsilon |\vee \sqrt {\varepsilon }}))$![]() is bounded by $1$
is bounded by $1$![]() .
.
We now give a sketch of the proof of the liminf inequality. By proposition 3.10 or 4.5 we have that
In fact, the last term above was always neglected in the previous computations, since it is nonnegative by (2.8). Using that $S_\varepsilon \in \mathcal {R}$![]() , a Taylor expansion of $\pi$
, a Taylor expansion of $\pi$![]() and of the exponential map yields
and of the exponential map yields

where $t_\varepsilon (x)\in [0,\,1]$![]() , $H_\varepsilon$
, $H_\varepsilon$![]() is a uniformly bounded matrix, and
is a uniformly bounded matrix, and
We now note that the first integral in (5.7) is equal to $0$![]() by (2.9); thus, by multiplying and dividing by $\alpha _\varepsilon :=(|A_\varepsilon |\vee \sqrt {\varepsilon })^2$
by (2.9); thus, by multiplying and dividing by $\alpha _\varepsilon :=(|A_\varepsilon |\vee \sqrt {\varepsilon })^2$![]() we obtain
we obtain

We observe that the left-hand side is nonnegative, hence the term within square brackets is nonnegative, as well. Since $\sqrt {\alpha _\varepsilon }=|A_\varepsilon |\vee \sqrt {\varepsilon }\ge \sqrt {\varepsilon }$![]() , $|A_\varepsilon |\rightarrow 0$
, $|A_\varepsilon |\rightarrow 0$![]() , $S_\varepsilon \rightarrow R_0$
, $S_\varepsilon \rightarrow R_0$![]() , ${A_\varepsilon }/{\sqrt {\alpha _\varepsilon }}\rightarrow A_0$
, ${A_\varepsilon }/{\sqrt {\alpha _\varepsilon }}\rightarrow A_0$![]() , letting $\varepsilon \rightarrow 0$
, letting $\varepsilon \rightarrow 0$![]() and using the dominated convergence theorem yield
and using the dominated convergence theorem yield

The liminf inequality follows now from the divergence theorem.
For the construction of the recovery sequence we proceed as in proposition 3.12 or 4.5, but choosing $R_\varepsilon :=R_0{\rm e}^{A_\varepsilon }$![]() with $A_\varepsilon :=\sqrt {\varepsilon }A_0$
with $A_\varepsilon :=\sqrt {\varepsilon }A_0$![]() . Since $R_0$
. Since $R_0$![]() is in $\mathcal {R}$
is in $\mathcal {R}$![]() , we can write
, we can write

The first two integrals at the right-hand side can be bounded by $\mathcal {E}_0(u_0,\,R_0)$![]() , arguing as in proposition 3.12 or 4.5. Repeating the same computations as for the liminf inequality, one can show that the last integral converges to $(1/2)\mathcal {F}(R_0,\,A_0)$
, arguing as in proposition 3.12 or 4.5. Repeating the same computations as for the liminf inequality, one can show that the last integral converges to $(1/2)\mathcal {F}(R_0,\,A_0)$![]() .
.
Convergence of almost minimizers and of infima can be proved exactly as in theorems 3.14 and 4.6. Finally, we observe that the functional $\mathcal {F}$![]() is always nonnegative by (5.2) and $\mathcal {F}(R,\,0)=0$
is always nonnegative by (5.2) and $\mathcal {F}(R,\,0)=0$![]() for every $R\in \mathcal {R}$
for every $R\in \mathcal {R}$![]() . Hence, by minimality we deduce that $\mathcal {F}(R_0,\,A_0)=0$
. Hence, by minimality we deduce that $\mathcal {F}(R_0,\,A_0)=0$![]() . This concludes the proof.
. This concludes the proof.
We conclude the paper with an example showing that, given any sequence $\lambda _\varepsilon \to 0$![]() such that
such that
for some $k>2$![]() , there may exist sequences of almost minimizers of $\mathcal {E}_\varepsilon$
, there may exist sequences of almost minimizers of $\mathcal {E}_\varepsilon$![]() for which any approximating sequence of rotations has a distance from $\mathcal {R}$
for which any approximating sequence of rotations has a distance from $\mathcal {R}$![]() of order $\lambda _\varepsilon$
of order $\lambda _\varepsilon$![]() . This provides a further difference with the case of dead loads [Reference Maor and Mora22].
. This provides a further difference with the case of dead loads [Reference Maor and Mora22].
Example 5.3 We start by considering a sequence $(\lambda _\varepsilon )$![]() satisfying (5.9) with $k=3$
satisfying (5.9) with $k=3$![]() . We assume the pressure intensity $\pi$
. We assume the pressure intensity $\pi$![]() to be of class $C^3$
to be of class $C^3$![]() . Moreover, we assume that the set of optimal rotations $\mathcal {R}$
. Moreover, we assume that the set of optimal rotations $\mathcal {R}$![]() is finite and that for every $R_0\in \mathcal {R}$
is finite and that for every $R_0\in \mathcal {R}$![]() there exists $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$
there exists $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$![]() such that
such that
These properties are satisfied, for instance, in example 5.1 if the function $\varphi$![]() is strictly increasing. Indeed, in this case the function $\phi$
is strictly increasing. Indeed, in this case the function $\phi$![]() in (5.6) attains its minimum only at $\alpha =0$
in (5.6) attains its minimum only at $\alpha =0$![]() and $\alpha =\pi$
and $\alpha =\pi$![]() and thus, $\mathcal {R}=\{\pm I\}$
and thus, $\mathcal {R}=\{\pm I\}$![]() . Moreover, in this example $\nabla \pi (x)=\nabla \pi (-x)=0$
. Moreover, in this example $\nabla \pi (x)=\nabla \pi (-x)=0$![]() for every $x\in \partial \Omega$
for every $x\in \partial \Omega$![]() , so that $\mathcal {F}(R_0,\,A_0)=0$
, so that $\mathcal {F}(R_0,\,A_0)=0$![]() for every $R_0\in \mathcal {R}$
for every $R_0\in \mathcal {R}$![]() and every $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$
and every $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$![]() . Finally, $\pi$
. Finally, $\pi$![]() is of class $C^3$
is of class $C^3$![]() if we assume, in addition, $\varphi \in C^4([0,\,\pi /2])$
if we assume, in addition, $\varphi \in C^4([0,\,\pi /2])$![]() with $\varphi ^{\rm (iv)}(0)=\varphi ^{\rm (iv)}(\pi /2)=0$
with $\varphi ^{\rm (iv)}(0)=\varphi ^{\rm (iv)}(\pi /2)=0$![]() and $\psi \in C^3([1,\,+\infty ))$
and $\psi \in C^3([1,\,+\infty ))$![]() with bounded third derivative and $\psi '''(1)=0$
with bounded third derivative and $\psi '''(1)=0$![]() .
.
Now let $(u_0,\, R_0)$![]() be a minimizer of $\mathcal {E}_0$
be a minimizer of $\mathcal {E}_0$![]() on $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$
on $\mathring {H}^{1}(\Omega ;\mathbb {R}^n)\times \mathcal {R}$![]() and let $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$
and let $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$![]() satisfy (5.10). Let $R_\varepsilon :=R_0 {\rm e}^{\lambda _\varepsilon A_0}$
satisfy (5.10). Let $R_\varepsilon :=R_0 {\rm e}^{\lambda _\varepsilon A_0}$![]() and let $(u_\varepsilon )$
and let $(u_\varepsilon )$![]() be an approximating sequence for $u_0$
be an approximating sequence for $u_0$![]() as in (3.31). We claim that the deformations $y_\varepsilon (x):=R_\varepsilon (x+\varepsilon u_\varepsilon (x))$
as in (3.31). We claim that the deformations $y_\varepsilon (x):=R_\varepsilon (x+\varepsilon u_\varepsilon (x))$![]() are a sequence of almost minimizers of $\mathcal {E}_\varepsilon$
are a sequence of almost minimizers of $\mathcal {E}_\varepsilon$![]() . Indeed, arguing as in the proof of the limsup inequality in theorem 5.2, we have by (5.8) that
. Indeed, arguing as in the proof of the limsup inequality in theorem 5.2, we have by (5.8) that

Therefore, the claim is proved if we show that the last term above vanishes, as $\varepsilon \rightarrow 0$![]() . To prove it we argue as in the proof of the liminf inequality in theorem 5.2, now expanding up to the third order. By (2.9) and (5.10) we obtain
. To prove it we argue as in the proof of the liminf inequality in theorem 5.2, now expanding up to the third order. By (2.9) and (5.10) we obtain
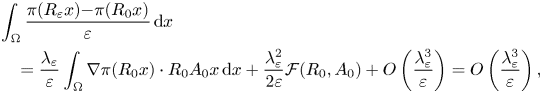
which proves the claim owing to (5.9) with $k=3$![]() .
.
We note that the intrinsic distance of $R_\varepsilon =R_0 {\rm e}^{\lambda _\varepsilon A_0}$![]() from $\mathcal {R}$
from $\mathcal {R}$![]() in $SO(n)$
in $SO(n)$![]() is of order $\lambda _\varepsilon$
is of order $\lambda _\varepsilon$![]() . Indeed, since $\mathcal {R}$
. Indeed, since $\mathcal {R}$![]() is finite by assumption, we have that $\,\mathrm {d}_{SO(n)}(R_\varepsilon ;\mathcal {R})=\,\mathrm {d}_{SO(n)}(R_\varepsilon,\,R_0)$
is finite by assumption, we have that $\,\mathrm {d}_{SO(n)}(R_\varepsilon ;\mathcal {R})=\,\mathrm {d}_{SO(n)}(R_\varepsilon,\,R_0)$![]() for $\varepsilon$
for $\varepsilon$![]() small enough. By definition we clearly have $\,\mathrm {d}_{SO(n)}(R_\varepsilon,\,R_0)\leq \lambda _\varepsilon$
small enough. By definition we clearly have $\,\mathrm {d}_{SO(n)}(R_\varepsilon,\,R_0)\leq \lambda _\varepsilon$![]() . On the other hand, since the intrinsic distance in $SO(n)$
. On the other hand, since the intrinsic distance in $SO(n)$![]() is equivalent to the Euclidean distance, we obtain
is equivalent to the Euclidean distance, we obtain
We now prove that for the sequence $(y_\varepsilon )$![]() constructed above, any sequence $(R_\varepsilon ')$
constructed above, any sequence $(R_\varepsilon ')$![]() of approximating rotations satisfies
of approximating rotations satisfies
By lemma 3.6 or lemma 4.2 we deduce that $|R_\varepsilon -R'_\varepsilon |\le C\varepsilon$![]() , hence $R_\varepsilon '\rightarrow R_0$
, hence $R_\varepsilon '\rightarrow R_0$![]() . Since $\mathcal {R}$
. Since $\mathcal {R}$![]() is finite, we have that $\,\mathrm {d}_{SO(n)}(R_\varepsilon ';\mathcal {R})=\,\mathrm {d}_{SO(n)}(R_\varepsilon ',\,R_0)$
is finite, we have that $\,\mathrm {d}_{SO(n)}(R_\varepsilon ';\mathcal {R})=\,\mathrm {d}_{SO(n)}(R_\varepsilon ',\,R_0)$![]() for $\varepsilon$
for $\varepsilon$![]() small enough. Let $A_\varepsilon '\in \mathbb {R}^{n\times n}_{\rm skew}$
small enough. Let $A_\varepsilon '\in \mathbb {R}^{n\times n}_{\rm skew}$![]() be such that $R_\varepsilon '=R_0 {\rm e}^{A_\varepsilon '}$
be such that $R_\varepsilon '=R_0 {\rm e}^{A_\varepsilon '}$![]() and $\,\mathrm {d}_{SO(n)}(R_\varepsilon ',\,R_0)=|A_\varepsilon '|$
and $\,\mathrm {d}_{SO(n)}(R_\varepsilon ',\,R_0)=|A_\varepsilon '|$![]() . Assume by contradiction that (5.12) does not hold, that is, $|A'_\varepsilon |/\lambda _\varepsilon \to 0$
. Assume by contradiction that (5.12) does not hold, that is, $|A'_\varepsilon |/\lambda _\varepsilon \to 0$![]() , as $\varepsilon \to 0$
, as $\varepsilon \to 0$![]() . Then we have
. Then we have
Dividing by $\lambda _\varepsilon$![]() and sending $\varepsilon \to 0$
and sending $\varepsilon \to 0$![]() , we obtain a contradiction, since the left-hand side vanishes by (5.9) and the right-hand side converges to $|A_0|=1$
, we obtain a contradiction, since the left-hand side vanishes by (5.9) and the right-hand side converges to $|A_0|=1$![]() .
.
Using again that $|R_\varepsilon -R'_\varepsilon |\le C\varepsilon$![]() , it is easy to see that in fact the intrinsic distance of $R_\varepsilon '$
, it is easy to see that in fact the intrinsic distance of $R_\varepsilon '$![]() from $\mathcal {R}$
from $\mathcal {R}$![]() is of order $\lambda _\varepsilon$
is of order $\lambda _\varepsilon$![]() .
.
If, instead, the sequence $(\lambda _\varepsilon )$![]() satisfies (5.9) for some $k\geq 4$
satisfies (5.9) for some $k\geq 4$![]() , the previous arguments can be adapted with small changes as follows. We assume, in addition, that $\pi$
, the previous arguments can be adapted with small changes as follows. We assume, in addition, that $\pi$![]() is of class $C^k$
is of class $C^k$![]() and that for every $R_0\in \mathcal {R}$
and that for every $R_0\in \mathcal {R}$![]() there exists $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$
there exists $A_0\in \mathbb {R}^{n\times n}_{\rm skew}$![]() such that
such that
where ${\mathcal {V}}_j(R_0,\,A_0)$![]() is the $j$
is the $j$![]() -th variation of the functional in (2.7) at $R_0$
-th variation of the functional in (2.7) at $R_0$![]() computed in the direction $A_0$
computed in the direction $A_0$![]() . This is fulfilled by the pressure load in example 5.1, if $\varphi$
. This is fulfilled by the pressure load in example 5.1, if $\varphi$![]() and $\psi$
and $\psi$![]() have enough regularity and satisfy suitable boundary conditions. By expanding up to order $k$
have enough regularity and satisfy suitable boundary conditions. By expanding up to order $k$![]() in (5.11), condition (5.13) guarantees that the sequence $(y_\varepsilon )$
in (5.11), condition (5.13) guarantees that the sequence $(y_\varepsilon )$![]() , constructed as above, is still a sequence of almost minimizers. The bounds on the intrinsic distance from $\mathcal {R}$
, constructed as above, is still a sequence of almost minimizers. The bounds on the intrinsic distance from $\mathcal {R}$![]() can be proved as before.
can be proved as before.
Remark 5.4 If condition (5.10) is not satisfied, that is, for every $R_0\in \mathcal {R}$![]() one has
one has
the phenomenon described in the previous example cannot arise. More precisely, one can show that the intrinsic distance of the approximating rotations from $\mathcal {R}$![]() is at most of order $\sqrt {\varepsilon }$
is at most of order $\sqrt {\varepsilon }$![]() , as in (5.4). The argument is the same as in [Reference Maor and Mora22, theorem 5.1], combined with (5.7).
, as in (5.4). The argument is the same as in [Reference Maor and Mora22, theorem 5.1], combined with (5.7).
Acknowledgments
The authors acknowledge support by the Italian Ministry of University and Research through the National Research Project PRIN 2017 ‘Variational Methods for Stationary and Evolution Problems with Singularities and Interfaces’. The authors are members of GNAMPA-INdAM.