Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-20T06:15:38.731Z Has data issue: false hasContentIssue false
  • English
  • Français

The one-sided dyadic Hardy—Littlewood maximal operator

Published online by Cambridge University Press:  03 October 2014

María Lorente  and
Francisco J. Martín-Reyes
María Lorente
Affiliation:
Departamento de Análisis Matemático, Facultad de Ciências, Universidad de Málaga, 29071 Málaga, Spain, ([email protected]; [email protected])
Francisco J. Martín-Reyes
Affiliation:
Departamento de Análisis Matemático, Facultad de Ciências, Universidad de Málaga, 29071 Málaga, Spain, ([email protected]; [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

The main aim of this paper is to introduce an appropriate dyadic one-sided maximal operator 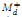, smaller than the one-sided Hardy–Littlewood maximal operator M+ but such that it controls M+ in a similar way to how the usual dyadic maximal operator controls the Hardy-Littlewood maximal operator. We characterize the weighted inequalities for this dyadic one-sided maximal operator.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 144 , Issue 5 , October 2014 , pp. 991 - 1006
Copyright
Copyright © Royal Society of Edinburgh 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Aimar, H. and Crescimbeni, R.. On one-sided BMO and Lipschitz functions. Annali Scuola Norm.. Sup. Pisa IV 27 (1998), 437–456.Google Scholar
2Aimar, H., Forzani, L. and Martín-Reyes, F. J.. On weighted inequalities for one-sided singular integrals. Proc. Am. Math. Soc. 125 (1997), 2057–2064.Google Scholar
3Andersen, K. F.. Weighted inequalities for maximal functions associated with general measures. Trans. Am. Math. Soc. 326 (1991), 907–920.CrossRefGoogle Scholar
4Bernardis, A. L., Lorente, M., Martín-Reyes, F. J., Martinez, M. T., de la Torre, A. and Torrea, J. L.. Differential transforms in weighted spaces. J. Fourier Analysis Applic. 12 (2006), 83–103.Google Scholar
5Fefferman, C. and Stein, E.. Some maximal inequalities. Am. J. Math. 93 (1971), 107–115.Google Scholar
6García-Cuerva, J. and de Francia, J. L. Rubio. Weighted norm inequalities and related topics, Mathematical Studies, vol. 116 (Amsterdam: North-Holland, 1985).Google Scholar
7Hunt, R. A., Kurtz, D. S. and Neugebauer, C. J.. A note on the equivalence of Ap and Sawyer's condition for equal weights. In Harmonic Analysis, Proc. Conf. in honor of Antoni Zygmund, vol. II, Wadsworth Mathematics Series, pp. 156–158 (London: Chapman and Hall, 1982).Google Scholar
8Lorente, M. and Riveros, M. S.. Weighted inequalities for commutators of one-sided singular integrals. Commentat. Math. Univ. Carolinae 43 (2002), 83–101.Google Scholar
9Lorente, M., Riveros, M. S. and de la Torre, A.. Weighted estimates for singular integral operators satisfying Hormander's conditions of Young type. J. Fourier Analysis Applic. 11(2005), 497–509.Google Scholar
10Martín-Reyes, F. J.. New proofs of weighted inequalities for the one-sided Hardy-Littlewood maximal functions. Proc. Am. Math. Soc. 117 (1993), 691–698.Google Scholar
11Martín-Reyes, F. J. and de la Torre, A.. One sided BMO spaces. J. Lond. Math. Soc. (2) 49 (1994), 529–542.Google Scholar
12Martín-Reyes, F. J., Ortega, P. and de la Torre, A.. Weighted inequalities for one-sided maximal functions. Trans. Am. Math. Soc. 319 (1990), 517–534.Google Scholar
13Martín-Reyes, F. J., Ortega, P. and de la Torre, A.. Weights for one-sided operators. In Recent developments in real and harmonic analysis, Applied and Numerical Harmonic Analysis, pp. 97–132, (Birkhäuser, 2010).CrossRefGoogle Scholar
14Muckenhoupt, B.. Weighted norm inequalities for the Hardy maximal function. Trans. Am. Math. Soc. 165 (1972), 207–226.Google Scholar
15Ombrosi, S.. Weak weighted inequalities for a dyadic one-sided maximal function in ℝn. Proc. Am. Math. Soc. 133 (2005), 1769–1775.Google Scholar
16Sawyer, E.. Two weight norm inequalities for certain maximal and integral operators. In Harmonic analysis, Lecture Notes in Mathematics, vol. 908, pp. 102–127 (Springer, 1982).Google Scholar
17Sawyer, E.. Weighted inequalities for the one-sided Hardy–Littlewood maximal functions. Trans. Am. Math. Soc. 297 (1986), 53–61.Google Scholar