Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-28T02:35:28.307Z Has data issue: false hasContentIssue false
  • English
  • Français

On the domains of minimal and maximal operators for regularisable singular differential expressions

Published online by Cambridge University Press:  14 November 2011

D. Race
D. Race
Affiliation:
Department of Mathematical and Computing Sciences, University of Surrey, Guildford, Surrey GU2 5XH, England, U.K.
Get access Rights & Permissions [Opens in a new window]

Synopsis

Certain classical differential expressions which are singular at a finite end-point (or at an interior point) can be represented as regular, scalar quasi-differential expressions, the best-known examples being the Boyd Equation and Laplace Tidal Wave Equation. We show here that in all such cases the domains of the minimal and maximal operators in the appropriate weighted Hilbert space 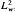, for the regularised expression, coincide with the corresponding domains for the expression in its original, singular form.

This is contrasted with a known property of the corresponding expression domains. Whereas for an expression M, the operator domains contain only functions y for which both y and My lie in the appropriate Hilbert space, the expression domain comprises a much larger set of functions with no such restrictions beyond those necessary for My to exist as a function. In the second-order case, the expression domain of the regularisation of a singular expression is known to be a strict subset of the original expression domain, contrasting with the results proved here for the operator domains.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 121 , Issue 3-4 , 1992 , pp. 191 - 202
Copyright
Copyright © Royal Society of Edinburgh 1992

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Atkinson, F. V., Everitt, W. N. and Zettl, A.. Regularization of a Sturm-Liouville problem with an interior singularity using quasi-derivatives. Diff. and Int. Equations 1 (1988), 213–221.Google Scholar
2Boyd, J. P.. Sturm-Liouville problems with an interior pole. J. Math. Phys. 22 (1981), 1575–1590.CrossRefGoogle Scholar
3Edmunds, D. E. and Evans, W. D.. Spectral Theory and Differential Operators (Oxford: Oxford University Press, 1987).Google Scholar
4Everitt, W. N.. On the transformation theory of ordinary second-order linear symmetric differential expressions. Czech. Math. J. 32 (1982), 275–306.CrossRefGoogle Scholar
5Everitt, W. N., Gunson, J. and Zettl, A.. Some comments on Sturm-Liouville eigenvalue problems with interior singularities. J. App. Math and Phys. (ZAMP) 38 (1987), 813–838.CrossRefGoogle Scholar
6Everitt, W. N. and Race, D.. Some remarks on linear ordinary quasi-differential expressions. Proc. London Math. Soc. 54 (1987), 300–320.CrossRefGoogle Scholar
7Everitt, W. N. and Race, D.. The regular representation of singular second-order differential expressions using quasi-derivatives. Proc. London Math. Soc, to appear.Google Scholar
8Everitt, W. N. and Race, D.. The regularization of singular second-order differential expressions using Frentzen-type quasi-derivatives (preprint).Google Scholar
9Everitt, W. N. and Zettl, A.. Products of differential expressions without smoothness assumptions. Quaestiones Math. 3 (1978), 67–82.CrossRefGoogle Scholar
10Frentzen, H.. Equivalence, adjoints and symmetry of quasi-differential expressions with matrix- valued coefficients and polynomials in them. Proc. Roy. Soc. Edinburgh Sect. A. 92 (1982), 123–146.CrossRefGoogle Scholar
11Homer, M. S.. Boundary value problems for the Laplace tidal wave equation. Proc. Roy. Soc. London Ser. A 428 (1990), 157–180.Google Scholar
12Naimark, M. A.. Linear differential operators Part II (New York: Ungar, 1968).Google Scholar
13Zettl, A.. Formally self-adjoint quasi-differential operators. Rocky Mountain J. Math. 5 (1975), 453–474.CrossRefGoogle Scholar