Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-22T21:07:42.913Z Has data issue: false hasContentIssue false
  • English
  • Français

On higher differentiability of solutions of parabolic systems with discontinuous coefficients and (p, q)-growth

Part of: Partial differential equations Parabolic equations and systems Miscellaneous topics - Partial differential equations

Published online by Cambridge University Press:  26 January 2019

Flavia Giannetti ,
Antonia Passarelli di Napoli  and
Christoph Scheven
Flavia Giannetti
Affiliation:
Dipartimento di Matematica e Applicazioni ‘R. Caccioppoli’, Università di Napoli ‘Federico II’, via Cintia - 80126, Napoli, Italy ([email protected]; [email protected])
Antonia Passarelli di Napoli
Affiliation:
Dipartimento di Matematica e Applicazioni ‘R. Caccioppoli’, Università di Napoli ‘Federico II’, via Cintia - 80126, Napoli, Italy ([email protected]; [email protected])
Christoph Scheven
Affiliation:
Faculty for Mathematics, University Duisburg-Essen, D-45117 Essen, Germany ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

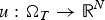We consider weak solutions $u:\Omega _T\to {\open R}^N$ to parabolic systems of the type

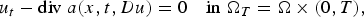$$u_t-{\rm div}\;a(x,t,Du) = 0\quad {\rm in}\;\Omega _T = \Omega \times (0,T),$$
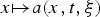where the function a(x, t, ξ) satisfies (p, q)-growth conditions. We give an a priori estimate for weak solutions in the case of possibly discontinuous coefficients. More precisely, the partial maps $x\mapsto a(x,t,\xi )$ under consideration may not be continuous, but may only possess a Sobolev-type regularity. In a certain sense, our assumption means that the weak derivatives 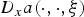$D_xa(\cdot ,\cdot ,\xi )$ are contained in the class 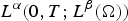$L^\alpha (0,T;L^\beta (\Omega ))$, where the integrability exponents 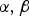$\alpha ,\beta $ are coupled by
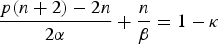$$\displaystyle{{p(n + 2)-2n} \over {2\alpha }} + \displaystyle{n \over \beta } = 1-\kappa $$
for some κ ∈ (0,1). For the gap between the two growth exponents we assume
$$2 \les p < q \les p + \displaystyle{{2\kappa } \over {n + 2}}.$$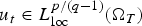
Under further assumptions on the integrability of the spatial gradient, we prove a result on higher differentiability in space as well as the existence of a weak time derivative $u_t\in L^{p/(q-1)}_{{\rm loc}}(\Omega _T)$. We use the corresponding a priori estimate to deduce the existence of solutions of Cauchy–Dirichlet problems with the mentioned higher differentiability property.

Keywords

Parabolic systemshigher differentiabilitydiscontinuous coefficients

MSC classification

Primary: 35B65: Smoothness and regularity of solutions
Secondary: 35K40: Second-order parabolic systems 35K92: Quasilinear parabolic equations with $p$-Laplacian 35R05: Partial differential equations with discontinuous coefficients or data
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 1 , February 2020 , pp. 419 - 451
Copyright
Copyright © Royal Society of Edinburgh 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Acerbi, E. and Mingione, G.. Gradient estimates for a class of parabolic systems. Duke Math. J. 136 (2007), 285–320.CrossRefGoogle Scholar
2Bögelein, V., Duzaar, F. and Mingione, G.. The boundary regularity of non-linear parabolic systems. II. Ann. Inst. H. Poincaré Anal. Non Linéaire 27 (2010), 145–200.CrossRefGoogle Scholar
3Bögelein, V., Foss, M. and Mingione, G.. Regularity in parabolic systems with continuous coefficients. Math. Z. 270 (2012), 903–938.CrossRefGoogle Scholar
4Bögelein, V., Duzaar, F. and Marcellini, P.. Parabolic equations with p,q-growth. J. Math. Pures Appl. 100 (2013a), 535–563.CrossRefGoogle Scholar
5Bögelein, V., Duzaar, F. and Marcellini, P.. Parabolic systems with p,q-growth: a variational approach. Arch. Rational Mech. Anal. 210 (2013b), 219–267.CrossRefGoogle Scholar
6Bögelein, V., Duzaar, F. and Marcellini, P.. Existence of evolutionary variational solutions via the calculus of variations. J. Differ. Equ. 256 (2014), 3912–3942.CrossRefGoogle Scholar
7Carozza, M., Kristensen, J. and Passarelli di Napoli, A.. Higher differentiability of minimizers of convex variational integrals. Ann. Inst. H. Poincaré Anal. Non Linéaire 28 (2011), 395–411.CrossRefGoogle Scholar
8Carozza, M., Kristensen, J. and Passarelli di Napoli, A.. Regularity of minimizers of autonomous convex variational integrals. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 13 (2014), 1065–1089.Google Scholar
9Cupini, G., Giannetti, F., Giova, R. and Passarelli di Napoli, A.. Higher integrability for minimizers of asymptotically convex integrals with discontinuous coefficients. Nonlinear Anal. 154 (2017), 7–24. (doi: 10.1016/j.na.2016.02.017).CrossRefGoogle Scholar
10Cupini, G., Marcellini, P. and Mascolo, E.. Existence and regularity for elliptic equations under p,q-growth. Adv. Differ. Equ. 19 (2014), 693–724.Google Scholar
11DeVore, R. A. and Sharpley, R. C.. Maximal functions measuring smoothness. Mem. Am. Math. Soc. 47 (1984), no. 293.Google Scholar
12DiBenedetto, E.. Degenerate parabolic equations (New York: Universitext, Springer-Verlag, 1993).CrossRefGoogle Scholar
13Duzaar, F. and Mingione, G.. Second order parabolic systems, optimal regularity, and singular sets of solutions. Ann. Inst. Henri Poincaré, Anal. Non Linéaire 22 (2005), 705–751.CrossRefGoogle Scholar
14Duzaar, F., Mingione, G. and Steffen, K.. Parabolic systems with polynomial growth and regularity. Mem. Am. Math. Soc. 214 (2011), no. 1005.Google Scholar
15Eleuteri, M., Marcellini, P. and Mascolo, E., Lipschitz estimates for systems with ellipticity conditions at infinity. Ann. Mat. Pura. Appl. 14 (2016), 1575–1603 (doi: 10.1007/s10231-015-0529-4).CrossRefGoogle Scholar
16Esposito, L., Leonetti, F. and Mingione, G.. Regularity for minimizers of functionals with p-q growth. NoDEA Nonlinear Differ. Equ. Appl. 6 (1999a), 133–148.CrossRefGoogle Scholar
17Esposito, L., Leonetti, F. and Mingione, G., Higher integrability for minimizers of integral functionals with (p, q) growth. J. Differ. Equ. 157 (1999b), 414–438.CrossRefGoogle Scholar
18Esposito, L., Leonetti, F. and Mingione, G.. Regularity results for minimizers of irregular integrals with (p, q) growth. Forum Math. 14 (2002), 245–272.CrossRefGoogle Scholar
19Giannetti, F. and Passarelli di Napoli, A.. Higher differentiability of minimizers of variational integrals with variable exponents. Math. Zeitschrift 280 (2015), 873–892.CrossRefGoogle Scholar
20Giannetti, F., Passarelli di Napoli, A. and Scheven, C.. Higher differentiability of solutions of parabolic systems with discontinuous coefficients. J. London Math. Soc. (2) 94 (2016), 1–20.CrossRefGoogle Scholar
21Giaquinta, M. and Modica, G.. Partial regularity of minimizers of quasiconvex integrals. Ann. Inst. H. Poincaré Anal. Non Linéaire 3 (1986), 185–208.CrossRefGoogle Scholar
22Giova, R.. Higher differentiability for n-harmonic systems with Sobolev coefficients. J. Diff. Equ. 259 (2015), 5667–5687.CrossRefGoogle Scholar
23Giusti, E.. Direct methods in the calculus of variations (Singapore: World Scientific, 2003).CrossRefGoogle Scholar
24Hajlasz, P.. Sobolev spaces on an arbitrary metric space. Potential Anal. 5 (1996), 403–415.Google Scholar
25Lichnewsky, A. and Temam, R.. Pseudosolutions of the time-dependent minimal surface problem. J. Differ. Equ. 30 (1978), 340–364.CrossRefGoogle Scholar
26Lions, J.. Quelques méthodes de résolution des problèmes aux limites non linéaires (Paris: Gauthier-Villars, 1969).Google Scholar
27Marcellini, P.. Regularity of minimizers of integrals of the calculus of variations with nonstandard growth conditions. Arch. Ration. Mech. Anal. 105 (1989), 267–284.CrossRefGoogle Scholar
28Marcellini, P.. Regularity and existence of solutions of elliptic equations with p, q-growth conditions. J. Differ. Equ. 90 (1991), 1–30.CrossRefGoogle Scholar
29Passarelli di Napoli, A.. Higher differentiability of minimizers of variational integrals with Sobolev coefficients. Adv. Calc. Var. 7 (2014a), 59–89.CrossRefGoogle Scholar
30Passarelli di Napoli, A.. Higher differentiability of solutions of elliptic systems with Sobolev coefficients: the case p = n = 2. Potential Anal. 41 (2014b), 715–735.CrossRefGoogle Scholar
31Scheven, C., Regularity for subquadratic parabolic systems: higher integrability and dimension estimates. Proc. R. Soc. Edinburgh 140A (2010a), 1269–1308.CrossRefGoogle Scholar
32Scheven, C.. Non-linear Calderón-Zygmund theory for parabolic systems with subquadratic growth. J. Evol. Equ. 10 (2010b), 597–622.CrossRefGoogle Scholar
33Showalter, R., Monotone operators in banach space and nonlinear partial differential equations (Providence, RI: Am. Math. Soc., 1997).Google Scholar
34Singer, T., Existence of weak solutions of parabolic systems with p, q-growth. Manuscripta Math. 151 (2016), 87–112.CrossRefGoogle Scholar
35You, J.. Regularity of solutions of certain parabolic system with nonstandard growth condition. Acta Math. Sinica 14 (1998), 145–160.Google Scholar