We consider the Cauchy problem for a general class of parabolic partial differential equations in the Euclidean space ℝN. We show that given a weighted Lp-space 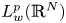$L_w^p({\mathbb {R}}^N)$ with 1 ⩽ p < ∞ and a fast growing weight w, there is a Schauder basis 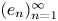$(e_n)_{n=1}^\infty$ in 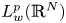$L_w^p({\mathbb {R}}^N)$ with the following property: given an arbitrary positive integer m there exists nm > 0 such that, if the initial data f belongs to the closed linear span of en with n ⩾ nm, then the decay rate of the solution of the problem is at least t−m for large times t.

The result generalizes the recent study of the authors concerning the classical linear heat equation. We present variants of the result having different methods of proofs and also consider finite polynomial decay rates instead of unlimited m.