Published online by Cambridge University Press: 15 January 2019
In this note, we prove the Payne-type conjecture about the behaviour of the nodal set of least energy sign-changing solutions for the equation 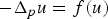 $-\Delta _p u = f(u)$ in bounded Steiner symmetric domains
$-\Delta _p u = f(u)$ in bounded Steiner symmetric domains  $ \Omega \subset {{\open R}^N} $ under the zero Dirichlet boundary conditions. The nonlinearity f is assumed to be either superlinear or resonant. In the latter case, least energy sign-changing solutions are second eigenfunctions of the zero Dirichlet p-Laplacian in Ω. We show that the nodal set of any least energy sign-changing solution intersects the boundary of Ω. The proof is based on a moving polarization argument.
$ \Omega \subset {{\open R}^N} $ under the zero Dirichlet boundary conditions. The nonlinearity f is assumed to be either superlinear or resonant. In the latter case, least energy sign-changing solutions are second eigenfunctions of the zero Dirichlet p-Laplacian in Ω. We show that the nodal set of any least energy sign-changing solution intersects the boundary of Ω. The proof is based on a moving polarization argument.