Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-23T17:40:29.742Z Has data issue: false hasContentIssue false
  • English
  • Français

Nonlinear fractional Laplacian problems with nonlocal ‘gradient terms’

Part of: Partial differential equations Miscellaneous topics - Partial differential equations Integral, integro-differential, and pseudodifferential operators Elliptic equations and systems

Published online by Cambridge University Press:  04 February 2020

Boumediene Abdellaoui  and
Antonio J. Fernández
Boumediene Abdellaoui
Affiliation:
Laboratoire d'Analyse Nonlinéaire et Mathématiques Appliquées, Département de Mathématiques, Université Abou Bakr Belkaïd, Tlemcen13000, Algeria ([email protected])
Antonio J. Fernández
Affiliation:
Univ. Polytechnique Hauts-de-France, EA 4015 - LAMAV - FR CNRS 2956, F-59313Valenciennes, France Laboratoire de Mathématiques (UMR 6623), Université de Bourgogne-Franche-Comté, 16 route de Gray, 25030Besançon Cedex, France ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

Let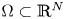$\Omega \subset \mathbb{R}^{N} $, N ≽ 2, be a smooth bounded domain. For s ∈ (1/2, 1), we consider a problem of the form

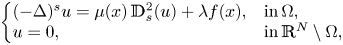$$\left\{\begin{array}{@{}ll} (-\Delta)^s u = \mu(x)\, \mathbb{D}_s^{2}(u) + \lambda f(x), & {\rm in}\,\Omega, \\ u= 0, & {\rm in}\,\mathbb{R}^{N} \setminus \Omega,\end{array}\right.$$
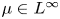where λ > 0 is a real parameter, f belongs to a suitable Lebesgue space, $\mu \in L^{\infty}$ and $\mathbb {D}_s^2$ is a nonlocal ‘gradient square’ term given by
$$\mathbb{D}_s^2 (u) = \frac{a_{N,s}}{2} \int_{\mathbb{R}^{N}} \frac{|u(x)-u(y)|^2}{|x-y|^{N+2s}}\,{\rm d}y.$$
 Depending on the real parameter λ > 0, we derive existence and non-existence results. The proof of our existence result relies on sharp Calderón–Zygmund type regularity results for the fractional Poisson equation with low integrability data. We also obtain existence results for related problems involving different nonlocal diffusion terms.

Keywords

Fractional LaplacianNonlocal gradientCalderón–ZygmundRegularityFractional Poisson equation

MSC classification

Primary: 35B65: Smoothness and regularity of solutions
Secondary: 35J62: Quasilinear elliptic equations 35R09: Integro-partial differential equations 47G20: Integro-differential operators
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 5 , October 2020 , pp. 2682 - 2718
Check for updates
Copyright
Copyright © The Author(s) 2020. Published by The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Abdellaoui, B., Attar, A. and Bentifour, R.. On the Fractional p-Laplacian equations with weight and general datum. Adv. Nonlinear Anal. 8 (2019), 144–174.CrossRefGoogle Scholar
2Abdellaoui, B. and Bentifour, R.. Caffarelli–Kohn–Nirenberg type inequalities of fractional order with applications. J. Funct. Anal. 272 (2017), 3998–4029.CrossRefGoogle Scholar
3Abdellaoui, B., Dall'Aglio, A. and Peral, I.. Some remarks on elliptic problems with critical growth in the gradient. J. Differ. Equ. 222 (2006), 21–62.CrossRefGoogle Scholar
4Abdellaoui, B. and Peral, I.. Towards a deterministic KPZ equation with fractional diffusion: The stationary problem. Nonlinearity 31 (2018), 1260.CrossRefGoogle Scholar
5Adams, R. A.. Sobolev spaces (New York: Academic Press, 1975).Google Scholar
6Applebaum, D., Lévy processes and stochastic calculus. Cambridge Studies in Advanced Mathematics, vol. 93 (Cambridge: Cambridge University Press, 2004).CrossRefGoogle Scholar
7Arcoya, D., De Coster, C., Jeanjean, L. and Tanaka, K.. Continuum of solutions for an elliptic problem with critical growth in the gradient. J. Funct. Anal. 268 (2015), 2298–2335.CrossRefGoogle Scholar
8Barrios, B., Figalli, A. and Ros-Oton, X.. Free boundary regularity in the parabolic fractional obstacle problem. Commun. Pure Appl. Math. 71 (2018), 2129–2159.Google Scholar
9Barrios, B., Figalli, A. and Ros-Oton, X.. Global regularity for the free boundary in the obstacle problem for the fractional Laplacian. Am. J. Math. 140 (2018), 415–447.Google Scholar
10Boccardo, L., Murat, F. and Puel, J.-P., Existence de solutions faibles pour des équations elliptiques quasi-linéaires à croissance quadratique. In Nonlinear partial differential equations and their applications. Collège de France Seminar, Vol. IV (Paris, 1981/1982). Res. Notes in Math., vol. 84, pp. 19–73 (Boston, MA, London: Pitman, 1983).Google Scholar
11Bourgain, J., Brezis, H. and Mironescu, P., Another look at Sobolev spaces. In Optimal control and partial differential equations. pp. 439–455. (Amsterdam: IOS, 2001).Google Scholar
12Brezis, H. and Mironescu, P.. Gagliardo–Nirenberg inequalities and non-inequalities: The full story. Ann. Inst. H. Poincaré Anal. Non Linéaire 35 (2018), 1355–1376.CrossRefGoogle Scholar
13Caffarelli, L. and Dávila, G.. Interior regularity for fractional systems. Ann. Inst. H. Poincaré Anal. Non Linéaire 36 (2018), 165–180.Google Scholar
14Caffarelli, L. and Figalli, A.. Regularity of solutions to the parabolic fractional obstacle problem. J. Reine Angew. Math. 680 (2013), 191–233.Google Scholar
15Caffarelli, L. and Vasseur, A.. Drift diffusion equations with fractional diffusion and the quasi-geostrophic equation. Ann. Math. 171 (2010), 1903–1930.CrossRefGoogle Scholar
16Chen, H. and Véron, L.. Semilinear fractional elliptic equations involving measures. J. Differ. Equ. 257 (2014), 1457–1486.Google Scholar
17Chen, H. and Véron, L.. Semilinear fractional elliptic equations with gradient nonlinearity involving measures. J. Funct. Anal. 266 (2014), 5467–5492.Google Scholar
18Cont, R. and Tankov, P., Financial modelling with jump processes. Chapman & Hall/CRC Financial Mathematics Series (Boca Raton, FL: Chapman & Hall/CRC, 2004).Google Scholar
19Da Lio, F. and Rivière, T.. Sub-criticality of non-local Schrödinger systems with antisymmetric potentials and applications to half-harmonic maps. Adv. Math. 227 (2011), 1300–1348.CrossRefGoogle Scholar
20Da Lio, F. and Schikorra, A.. n/p-Harmonic maps: Regularity for the sphere case. Adv. Calc. Var. 7 (2014), 1–26.CrossRefGoogle Scholar
21De Coster, C. and Fernández, A. J.. Existence and multiplicity for elliptic p-Laplacian problems with critical growth in the gradient. Calc. Var. Partial Differ. Equ. 57 (2018), 89.CrossRefGoogle Scholar
22Di Nezza, E., Palatucci, G. and Valdinoci, E.. Hitchhiker's guide to the fractional Sobolev spaces. Bull. Sci. Math. 136 (2012), 521–573.Google Scholar
23Dipierro, S., Figalli, A. and Valdinoci, E.. Strongly nonlocal dislocation dynamics in crystals. Commun. Partial Differ. Equ. 39 (2014), 2351–2387.Google Scholar
24Dipierro, S., Palatucci, G. and Valdinoci, E.. Dislocation dynamics in crystals: A macroscopic theory in a fractional Laplace setting. Commun. Math. Phys. 333 (2015), 1061–1105.Google Scholar
25Ferone, V. and Murat, F.. Nonlinear problems having natural growth in the gradient: An existence result when the source terms are small. Nonlinear Anal. Ser. A: Theory Methods) 42 (2000), 1309–1326.CrossRefGoogle Scholar
26Ferrari, F. and Verbitsky, I. E.. Radial fractional Laplace operators and Hessian inequalities. J. Differ. Equ. 253 (2012), 244–272.CrossRefGoogle Scholar
27Frank, R. L. and Seiringer, R.. Non-linear ground state representations and sharp Hardy inequalities. J. Funct. Anal. 255 (2008), 3407–3430.CrossRefGoogle Scholar
28Grenon, N., Murat, F. and Porretta, A.. Existence and a priori estimate for elliptic problems with subquadratic gradient dependent terms. C. R. Math. Acad. Sci. Paris 342 (2006), 23–28.Google Scholar
29Jeanjean, L. and Sirakov, B.. Existence and multiplicity for elliptic problems with quadratic growth in the gradient. Commun. Partial Differ. Equ. 38 (2013), 244–264.CrossRefGoogle Scholar
30Kardar, M., Parisi, G. and Zhang, Y.-C.. Dynamic scaling of growing interfaces. Phys. Rev. Lett. 56 (1986), 889–892.CrossRefGoogle ScholarPubMed
31Kuusi, T., Mingione, G. and Sire, Y.. Nonlocal equations with measure data. Commun. Math. Phys. 337 (2015), 1317–1368.CrossRefGoogle Scholar
32Kuusi, T., Mingione, G. and Sire, Y.. Nonlocal self-improving properties. Anal. PDE 8 (2015), 57–114.CrossRefGoogle Scholar
33Laskin, N.. Fractional quantum mechanics and lévy path integrals. Phys. Lett. A 268 (2000), 298–305.CrossRefGoogle Scholar
34Leonori, T., Peral, I., Primo, A. and Soria, F.. Basic estimates for solutions of a class of nonlocal elliptic and parabolic equations. Discrete Contin. Dyn. Syst. 35 (2015), 6031–6068.CrossRefGoogle Scholar
35Millot, V. and Sire, Y.. On a fractional Ginzburg–Landau equation and 1/2-harmonic maps into spheres. Arch. Ration. Mech. Anal. 215 (2015), 125–210.CrossRefGoogle Scholar
36Phuc, N. C.. Morrey global bounds and quasilinear Riccati type equations below the natural exponent. J. Math. Pure Appl. 102 (2014), 99–123.Google Scholar
37Ponce, A. C., Elliptic PDEs, measures and capacities. From the Poisson equations to nonlinear Thomas-Fermi problems. EMS Tracts in Mathematics, vol. 23 (Zürich: European Mathematical Society (EMS), 2016).CrossRefGoogle Scholar
38Schikorra, A.. Integro-differential harmonic maps into spheres. Commun. Partial Differ. Equ. 40 (2015), 506–539.CrossRefGoogle Scholar
39Shieh, T.-T. and Spector, D.. On a new class of fractional partial differential equations. Adv. Calc. Var. 8 (2015), 321–336.CrossRefGoogle Scholar
40Sirakov, B.. Solvability of uniformly elliptic fully nonlinear PDE. Arch. Ration. Mech. Anal. 195 (2010), 579–607.CrossRefGoogle Scholar
41Stein, E. M., Singular integrals and differentiability properties of functions. Princeton Mathematical Series, No. 30 (Princeton, NJ: Princeton University Press, 1970).Google Scholar
42Toland, J. F.. The Peierls–Nabarro and Benjamin–Ono equations. J. Funct. Anal. 145 (1997), 136–150.Google Scholar