Hostname: page-component-669899f699-rg895 Total loading time: 0 Render date: 2025-04-29T21:57:50.010Z Has data issue: false hasContentIssue false
  • English
  • Français

Local regularity for nonlocal double phase equations in the Heisenberg group

Part of: Partial differential equations Integral, integro-differential, and pseudodifferential operators Representations of solutions

Published online by Cambridge University Press:  25 November 2024

Yuzhou Fang ,
Chao Zhang Open the ORCID record for Chao Zhang [Opens in a new window]  and
Junli Zhang
Yuzhou Fang
Affiliation:
School of Mathematics, Harbin Institute of Technology, 150001 Harbin, China ([email protected])
Chao Zhang
Affiliation:
School of Mathematics and Institute for Advanced Study in Mathematics, Harbin Institute of Technology, 150001 Harbin, China ([email protected])
Junli Zhang
Affiliation:
School of Mathematics and Data Science, Shaanxi University of Science and Technology, 710021 Xi’an, China ([email protected]) (corresponding author)
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove interior boundedness and Hölder continuity for the weak solutions of nonlocal double phase equations in the Heisenberg group 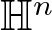$\mathbb{H}^n$. This solves a problem raised by Palatucci and Piccinini et al. in 2022 and 2023 for the nonlinear integro-differential problems in Heisenberg setting. Our proof of the a priori estimates bases on De Giorgi–Nash–Moser theory, where the important ingredients are Caccioppoli-type inequality and Logarithmic estimate. To achieve this goal, we establish a new and crucial Sobolev–Poincaré type inequality in local domain, which may be of independent interest and potential applications.

Keywords

energy inequalitiesHeisenberg groupnonlocal double phase equationregularitySobolev–Poincaré type inequality

MSC classification

Primary: 35D30: Weak solutions 35B45: A priori estimates 35B65: Smoothness and regularity of solutions 47G20: Integro-differential operators
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , First View , pp. 1 - 37
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Adimurthi, A. and Mallick, A.. Hardy type inequality on fractional order Sobolev spaces on the Heisenberg group. Ann. Sc. Norm. Super. Pisa Cl. Sci. 18 (2018), 917–949.Google Scholar
Branson, T. P., Fontana, L. and Morpurgo, C.. Moser-Trudinger and Beckner-Onofri’s Inequalities on the CR sphere. Ann. of Math. 177 (2013), 1–52.CrossRefGoogle Scholar
Byun, S. S., Kim, H. and Ok, J.. Local Hölder continuity for fractional nonlocal equations with general growth. Math. Ann. 387 (2023), .CrossRefGoogle Scholar
Byun, S. S., Ok, J. and Song, K.. Hölder regularity for weak solutions to nonlocal double phase problems. J. Math. Pures Appl. 168 (2022), 110–142.CrossRefGoogle Scholar
Chaker, J., Kim, M. and Weidner, M.. Harnack inequality for nonlocal problems with non-standard growth. Math. Ann. 386 (2022), 533–550.CrossRefGoogle Scholar
Ciatti, P., Cowling, M. G. and Ricci, F.. Hardy and uncertainty inequalities on stratified Lie groups. Adv. Math. 277 (2015), 365–387.CrossRefGoogle Scholar
Cinti, E. and Tan, J.. A nonlinear Liouville theorem for fractional equations in the Heisenberg group. J. Math. Anal. Appl. 433 (2016), 434–454.CrossRefGoogle Scholar
Colombo, M. and Mingione, G.. Bounded minimisers of double phase variational integrals. Arch. Ration. Mech. Anal. 218 (2015), 219–274.CrossRefGoogle Scholar
Colombo, M. and Mingione, G.. Regularity for double phase variational problems. Arch. Ration. Mech. Anal. 215 (2015), 443–496.CrossRefGoogle Scholar
Cupini, G., Marcellini, P. and Mascolo, E.. Local boundedness of minimizers with limit growth conditions. J. Optim. Theory Appl. 166 (2015), 1–22.CrossRefGoogle Scholar
Cygan, J.. Subadditicity of homogeneous norms on certain nilpotent Lie groups. Proc. Am. Math. Soc. 83 (1981), 69–70.CrossRefGoogle Scholar
De Filippis, C. and Palatucci, G.. Hölder regularity for nonlocal double phase equations. J. Differential Equations. 267 (2019), 547–586.CrossRefGoogle Scholar
Di Castro, A., Kuusi, T. and Palatucci, G.. Nonlocal Harnack inequalities. J. Funct. Anal. 267 (2014), 1807–1836.CrossRefGoogle Scholar
Di Castro, A., Kuusi, T. and Palatucci, G.. Local behavior of fractional p-minimizers. Ann. Inst. H. Poincaré Anal. Non LinéAire. 33 (2016), 1279–1299.CrossRefGoogle Scholar
Di Nezza, E., Palatucci, G. and Valdinoci, E.. Hitchhiker’s guide to the fractional Sobolev spaces. Bull. Sci. Math. 136 (2012), 521–573.CrossRefGoogle Scholar
Fang, Y. and Zhang, C.. Harnack inequality for the nonlocal equations with general growth. Proc. Roy. Soc. Edinburgh Sect. A. 153 (2023), 1479–1502.CrossRefGoogle Scholar
Fang, Y. and Zhang, C.. On weak and viscosity solutions of nonlocal double phase equations. Int. Math. Res. Not. IMRN. 5 (2023), 3746–3789.CrossRefGoogle Scholar
Ferrari, F. and Franchi, B.. Harnack inequality for fractional Laplacians in Carnot groups. Math. Z. 279 (2015), 435–458.CrossRefGoogle Scholar
Ferrari, F., Miranda, M.Jr., Pallara, D., Pinamonti, A. and Sire, Y.. Fractional Laplacians, perimeters and heat semigroups in Carnot groups. Discrete Cont. Dyn. Syst. Ser. S. 11 (2018), 477–491.Google Scholar
Frank, R., Gonzalez, M., Monticelli, D. and Tan, J.. An extension problem for the CR fractional Laplacian. Adv. Math. 270 (2015), 97–137.CrossRefGoogle Scholar
Garofalo, N. and Tralli, G.. Feeling the heat in a group of Heisenberg type. Adv. Math. 381 (2021), .CrossRefGoogle Scholar
Garofalo, N. and Tralli, G.. A class of nonlocal hypoelliptic operators and their extensions. Indiana Univ. Math. J. 70 (2022), 1717–1744.CrossRefGoogle Scholar
Giacomoni, J., Kumar, D. and Sreenadh, K.. Global regularity results for non-homogeneous growth fractional problems. J. Geom. Anal. 32 (2021), .Google Scholar
Giacomoni, J., Kumar, D. and Sreenadh, K.. Hölder regularity results for parabolic nonlocal double phase problems. arXiv:2112.04287v1.Google Scholar
Giusti, E.. Direct Methods in the Calculus of Variations (World Scientific Publishing Co. Inc., River Edge, NJ, 2003).CrossRefGoogle Scholar
Iannizzotto, A., Mosconi, S. and Squassina, M.. Global Hölder regularity for the fractional p-Laplacian. Rev. Mat. Iberoam. 32 (2016), 1353–1392.CrossRefGoogle Scholar
Kassymov, A. and Suragan, D.. Lyapunov-type inequalities for the fractional p-sub-Laplacian. Adv. Oper. Theory. 5 (2020), 435–452.CrossRefGoogle Scholar
Kassymov, A. and Surgan, D.. Some functional inequalities for the fractional p-sub-Laplacian. arXiv:1804.01415.Google Scholar
Korvenpää, J., Kuusi, T. and Palatucci, G.. The obstacle problem for nonlinear integro-differential operators. Calc. Var. Partial Differential Equations. 55 (2016), .CrossRefGoogle Scholar
Korvenpää, J., Kuusi, T. and Palatucci, G.. Fractional superharmonic functions and the Perron method for nonlinear integro-differential equations. Math. Ann. 369 (2017), 1443–1489.CrossRefGoogle Scholar
Manfredini, M., Palatucci, G., Piccinini, M. and Polidoro, S.. Hölder continuity and boundedness estimates for nonlinear fractional equations in the Heisenberg group. J. Geom. Anal. 33 (2023), .CrossRefGoogle Scholar
Palatucci, G. and Piccinini, M.. Nonlocal Harnack inequalities in the Heisenberg group. Calc. Var. Partial Differential Equations. 61 (2022), .CrossRefGoogle Scholar
Palatucci, G. and Piccinini, M.. Nonlinear fractional equations in the Heisenberg group. Bruno Pini Mathematical Analysis Seminar. 14 (2023), 163–200.Google Scholar
Piccinini, M.. The obstacle problem and the Perron Method for nonlinear fractional equations in the Heisenberg group. Nonlinear Anal. 222 (2022), .CrossRefGoogle Scholar
Prasad, H. and Tewary, V.. boundedness of variational solutions to nonlocal double phase parabolic equations. J. Differential Equations. 351 (2023), 243–276.CrossRefGoogle Scholar
Roncal, L. and Thangavelu, S.. Hardy’s Inequality for fractional powers of the sublaplacian on the Heisenberg group. Adv. Math. 302 (2016), 106–158.CrossRefGoogle Scholar
Scott, J. and Mengesha, T.. Self-improving inequalities for bounded weak solutions to nonlocal double phase equations. Commun. Pure Appl. Anal. 21 (2022), 183–212.CrossRefGoogle Scholar