Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Hoffmann-Ostenhof, Maria
and
Hoffmann-Ostenhof, Thomas
1984.
On embedded eigenvalues for the one-dimensional Schrödinger equation.
Journal of Mathematical Physics,
Vol. 25,
Issue. 8,
p.
2490.
Amrein, Werner O.
1984.
Bornes inférieurs pour des fonctions propres de l'opérateur de Schrödinger.
Journées équations aux dérivées partielles,
p.
1.
Herbst, Ira W.
1986.
Lower bounds in cones for solutions to the schrödinger equation.
Journal d'Analyse Mathématique,
Vol. 47,
Issue. 1,
p.
151.
Hoffmann-Ostenhof, M.
1990.
On the asymptotic decay of L2-solutions of one-body Schrödinger equations in unbounded domains.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 115,
Issue. 1-2,
p.
65.
Cruz-Sampedro, J.
1999.
Unique continuation at infinity of solutions to Schrödinger equations with complex-valued potentials.
Proceedings of the Edinburgh Mathematical Society,
Vol. 42,
Issue. 1,
p.
143.
Kuchment, Peter
and
Vainberg, Boris
2000.
On absence of embedded eigenvalues for schrÖdinger operators with perturbed periodic potentials.
Communications in Partial Differential Equations,
Vol. 25,
Issue. 9-10,
p.
1809.
Kenig, C E
Ponce, G
and
Vega, L
2012.
Lower bounds for non-trivial travelling wave solutions of equations of KdV type.
Nonlinearity,
Vol. 25,
Issue. 5,
p.
1235.
Davey, Blair
2014.
Some Quantitative Unique Continuation Results for Eigenfunctions of the Magnetic Schrödinger Operator.
Communications in Partial Differential Equations,
Vol. 39,
Issue. 5,
p.
876.
Lin, Ching-Lung
and
Wang, Jenn-Nan
2014.
Quantitative uniqueness estimates for the general second order elliptic equations.
Journal of Functional Analysis,
Vol. 266,
Issue. 8,
p.
5108.
Herbst, I.
and
Skibsted, E.
2015.
Decay of eigenfunctions of elliptic PDE's, I.
Advances in Mathematics,
Vol. 270,
Issue. ,
p.
138.
Herbst, I.
and
Skibsted, E.
2017.
Decay of eigenfunctions of elliptic PDE's, II.
Advances in Mathematics,
Vol. 306,
Issue. ,
p.
177.
El Aïdi, Mohammed
2017.
On a unique continuation for Aharonov–Bohm magnetic Schrödinger equation.
Forum Mathematicum,
Vol. 29,
Issue. 6,
p.
1349.
Aïdi, Mohammed El
2020.
On the decay at infinity of solutions of fractional Schrödinger equations.
Complex Variables and Elliptic Equations,
Vol. 65,
Issue. 2,
p.
141.
Liu, Wencai
2022.
Topics on Fermi varieties of discrete periodic Schrödinger operators.
Journal of Mathematical Physics,
Vol. 63,
Issue. 2,
Liu, Wencai
2022.
Irreducibility of the Fermi variety for discrete periodic Schrödinger operators and embedded eigenvalues.
Geometric and Functional Analysis,
Vol. 32,
Issue. 1,
p.
1.
Cassano, Biagio
2022.
Sharp exponential decay for solutions of the stationary perturbed Dirac equation.
Communications in Contemporary Mathematics,
Vol. 24,
Issue. 02,
Kuchment, Peter
2023.
Analytic and algebraic properties of dispersion relations (Bloch varieties) and Fermi surfaces. What is known and unknown.
Journal of Mathematical Physics,
Vol. 64,
Issue. 11,


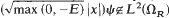 for some γ>0 or ψ has compact support. Related results are given for potentials tending to infinity for |x|→∞.
for some γ>0 or ψ has compact support. Related results are given for potentials tending to infinity for |x|→∞.