Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Anderson, Douglas R.
and
Onitsuka, Masakazu
2020.
Hyers–Ulam Stability for Quantum Equations of Euler Type.
Discrete Dynamics in Nature and Society,
Vol. 2020,
Issue. ,
p.
1.
Anderson, Douglas R.
and
Onitsuka, Masakazu
2021.
Ulam stability for nonautonomous quantum equations.
Journal of Inequalities and Applications,
Vol. 2021,
Issue. 1,
Dragičević, Davor
2021.
On the Hyers–Ulam stability of certain nonautonomous and nonlinear difference equations.
Aequationes mathematicae,
Vol. 95,
Issue. 5,
p.
829.
Dragičević, Davor
2021.
Hyers–Ulam Stability for a Class of Perturbed Hill’s Equations.
Results in Mathematics,
Vol. 76,
Issue. 3,
Backes, Lucas
and
Dragičević, Davor
2021.
Shadowing for infinite dimensional dynamics and exponential trichotomies.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 151,
Issue. 3,
p.
863.
Dragičević, Davor
and
Pituk, Mihály
2021.
Shadowing for nonautonomous difference equations with infinite delay.
Applied Mathematics Letters,
Vol. 120,
Issue. ,
p.
107284.
Anderson, Douglas R.
and
Onitsuka, Masakazu
2021.
Nonlinear Analysis, Differential Equations, and Applications.
Vol. 173,
Issue. ,
p.
17.
Backes, Lucas
and
Dragičević, Davor
2021.
A general approach to nonautonomous shadowing for nonlinear dynamics.
Bulletin des Sciences Mathématiques,
Vol. 170,
Issue. ,
p.
102996.
Barreira, Luis
and
Valls, Claudia
2022.
Stability of delay equations.
Electronic Journal of Qualitative Theory of Differential
Equations,
p.
1.
Anderson, Douglas R.
and
Onitsuka, Masakazu
2022.
Hyers–Ulam Stability for Differential Systems with $$2\times 2$$ Constant Coefficient Matrix.
Results in Mathematics,
Vol. 77,
Issue. 3,
Backes, Lucas
Dragičević, Davor
and
Singh, Lokesh
2022.
Shadowing for nonautonomous and nonlinear dynamics with impulses.
Monatshefte für Mathematik,
Vol. 198,
Issue. 3,
p.
485.
Backes, Lucas
Dragičević, Davor
Pituk, Mihály
and
Singh, Lokesh
2022.
Weighted shadowing for delay differential equations.
Archiv der Mathematik,
Vol. 119,
Issue. 5,
p.
539.
Dragičević, Davor
2024.
Generalized Dichotomies and Hyers–Ulam Stability.
Results in Mathematics,
Vol. 79,
Issue. 1,
Backes, Lucas
and
Dragičević, Davor
2024.
Stability of nonautonomous systems on Fréchet spaces.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 118,
Issue. 3,
Backes, Lucas
Dragičević, Davor
Onitsuka, Masakazu
and
Pituk, Mihály
2024.
Conditional Lipschitz Shadowing for Ordinary Differential Equations.
Journal of Dynamics and Differential Equations,
Vol. 36,
Issue. 4,
p.
3535.
Anderson, Douglas R.
Onitsuka, Masakazu
and
O'Regan, Donal
2024.
Best Ulam constants for two‐dimensional nonautonomous linear differential systems.
Mathematische Nachrichten,
Vol. 297,
Issue. 9,
p.
3470.
Dragičević, Davor
Zhang, Weinian
and
Zhou, Linfeng
2024.
Measurable weighted shadowing for random dynamical systems on Banach spaces.
Journal of Differential Equations,
Vol. 392,
Issue. ,
p.
364.
Backes, Lucas
Dragičević, Davor
and
Tang, Xiao
2024.
Parameterized shadowing for nonautonomous dynamics.
Journal of Mathematical Analysis and Applications,
Vol. 529,
Issue. 1,
p.
127584.
Buică, Adriana
and
Tőtős, György
2024.
Characterization of Ulam-Hyers stability of linear differential equations with periodic coefficients.
Journal of Mathematical Analysis and Applications,
Vol. 530,
Issue. 1,
p.
127739.
Dragičević, Davor
and
Peček, Nevena Jurčević
2024.
HYERS–ULAM–RASSIAS STABILITY FOR NONAUTONOMOUS DYNAMICS.
Rocky Mountain Journal of Mathematics,
Vol. 54,
Issue. 1,
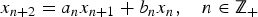 $$x_{n + 2} = a_nx_{n + 1} + b_nx_n,\quad n\in {\open Z}_+ $$
$$x_{n + 2} = a_nx_{n + 1} + b_nx_n,\quad n\in {\open Z}_+ $$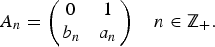 $$A_n = \left( {\matrix{ 0 & 1 \cr {b_n} & {a_n} \cr } } \right)\quad n\in {\open Z}_+ .$$
$$A_n = \left( {\matrix{ 0 & 1 \cr {b_n} & {a_n} \cr } } \right)\quad n\in {\open Z}_+ .$$ $${x}^{\prime \prime}(t) = a(t){x}^{\prime}(t) + b(t)x(t),\quad t\in {\open R},$$
$${x}^{\prime \prime}(t) = a(t){x}^{\prime}(t) + b(t)x(t),\quad t\in {\open R},$$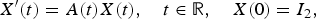 $${X}^{\prime}(t) = A(t)X(t),\quad t\in {\open R},\quad X(0) = I_2,$$
$${X}^{\prime}(t) = A(t)X(t),\quad t\in {\open R},\quad X(0) = I_2,$$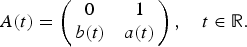 $$A(t) = \left( {\matrix{ 0 & 1 \cr {b(t)} & {a(t)} \cr } } \right),\quad t\in {\open R}.$$
$$A(t) = \left( {\matrix{ 0 & 1 \cr {b(t)} & {a(t)} \cr } } \right),\quad t\in {\open R}.$$