Hostname: page-component-77c89778f8-vsgnj Total loading time: 0 Render date: 2024-07-16T18:24:52.290Z Has data issue: false hasContentIssue false
  • English
  • Français

Ground state solutions of Hamiltonian elliptic systems in dimension two

Part of: Equations of mathematical physics and other areas of application Elliptic equations and systems

Published online by Cambridge University Press:  12 February 2019

Djairo G. de Figueiredo ,
João Marcos do Ó  and
Jianjun Zhang
Djairo G. de Figueiredo
Affiliation:
IMECC, Universidade Estadual de Campinas, C.P. 6065, 13081–970, Campinas, SP, Brazil ([email protected])
João Marcos do Ó
Affiliation:
Federal University of Paraíba, 58051–900, João Pessoa-PB, Brazil ([email protected])
Jianjun Zhang
Affiliation:
College Math. and Statistics, Chongqing Jiaotong University, Chongqing400074PR China ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

The aim of this paper is to study Hamiltonian elliptic system of the form 0.1

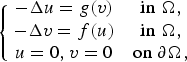$$\left\{ {\matrix{ {-\Delta u = g(v)} & {{\rm in}\;\Omega,} \cr {-\Delta v = f(u)} & {{\rm in}\;\Omega,} \cr {u = 0,v = 0} & {{\rm on}\;\partial \Omega,} \cr } } \right.$$
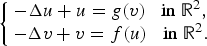where Ω ⊂ ℝ2 is a bounded domain. In the second place, we present existence results for the following stationary Schrödinger systems defined in the whole plane 0.2
$$\left\{ {\matrix{ {-\Delta u + u = g(v)\;\;\;{\rm in}\;{\open R}^2,} \cr {-\Delta v + v = f(u)\;\;\;{\rm in}\;{\open R}^2.} \cr } } \right.$$
We assume that the nonlinearities f, g have critical growth in the sense of Trudinger–Moser. By using a suitable variational framework based on the generalized Nehari manifold method, we obtain the existence of ground state solutions of both systems (0.1) and (0.2).

Keywords

Hamiltonian elliptic systemTrudinger–Moser inequalityground stategeneralized Nehari manifold

MSC classification

Primary: 35Q55: NLS-like equations (nonlinear Schrödinger)
Secondary: 35J50: Variational methods for elliptic systems 35J60: Nonlinear elliptic equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 4 , August 2020 , pp. 1737 - 1768
Check for updates
Copyright
Copyright © Royal Society of Edinburgh 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Adachi, S. and Tanaka, K.. Trudinger type inequalities in ℝN and their best exponents. Proc. Am. Math. Soc. 128 (2000), 2051–2057.CrossRefGoogle Scholar
2Albuquerque, F., do Ó, J. M. and Medeiros, E.. On a class of Hamiltonian elliptic systems involving unbounded or decaying potentials in dimension two. Math. Nachr. 289 (2016), 1568–1584.CrossRefGoogle Scholar
3Alves, C. O., Souto, M. and Montenegro, M.. Existence of a ground state solution for a nonlinear scalar field equation with critical growth. Calc. Var. Partial Differ. Equ. 43 (2012), 537–554.CrossRefGoogle Scholar
4Benci, V. and Rabinowitz, P.. Critical point theorems for indefinite functionals. Invent. Math. 52 (1979), 241–273.CrossRefGoogle Scholar
5Bonheure, D., dos Santos, E. and Ramos, M.. Ground state and non-ground state solutions of some strongly coupled elliptic systems. Trans. Am. Math. Soc. 364 (2012), 447–491.CrossRefGoogle Scholar
6Bonheure, D., dos Santos, E. and Tavares, H.. Hamiltonian elliptic systems: a guide to variational frameworks. Port. Math. 71 (2014), 301–395.CrossRefGoogle Scholar
7Brezis, H. and Nirenberg, L.. Positive solutions of nonlinear elliptic equations involving critical Sobolev exponents. Comm. Pure Appl. Math. 36 (1983), 437–477.CrossRefGoogle Scholar
8Cao, D. M.. Nontrivial solution of semilinear elliptic equation with critical exponent in ℝ2. Comm. Partial Differ. Equ. 17 (1992), 407–435.CrossRefGoogle Scholar
9Cassani, D. and Tarsi, C.. Existence of solitary waves for supercritical Schrödinger systems in dimension two. Calc. Var. Partial Differ. Equ. 54 (2015), 1673–1704.CrossRefGoogle Scholar
10Clement, P. H., de Fegueiredo, D. and Mitedieri, E.. Positive solutions of semilinear elliptic systems. Comm. Partial Differ. Equ. 17 (1992), 923–940.CrossRefGoogle Scholar
11de Figueiredo, D. and Felmer, P.. On superquadratic elliptic systems. Trans. Am. Math. Soc. 343 (1994), 99–116.CrossRefGoogle Scholar
12de Figueiredo, D. and Yang, J.. Decay, symmetry and existence of solutions of semilinear elliptic systems. Nonlinear Anal. 33 (1998), 211–234.CrossRefGoogle Scholar
13de Figueiredo, D., Miyagaki, O. H. and Ruf, B.. Elliptic equations in ℝ2 with nonlinearities in the critical growth range. Calc. Var. PDE 3 (1995), 139–153.CrossRefGoogle Scholar
14de Figueiredo, D., do Ó, J. M. and Ruf, B.. On an inequality by N. Trudinger and J. Moser and related elliptic equations. Comm. Pure Appl. Math. 55 (2002), 135–152.CrossRefGoogle Scholar
15de Figueiredo, D., do Ó, J. M. and Ruf, B.. Critical and subcritical elliptic systems in dimension two. Indiana Univ. Math. J. 53 (2004), 1037–1054.CrossRefGoogle Scholar
16de Figueiredo, D., do Ó, J. M. and Ruf, B.. An Orlicz-space approach to superlinear elliptic systems. J. Funct. Anal. 224 (2005), 471–496.CrossRefGoogle Scholar
17de Figueiredo, D., do Ó, J. M. and Ruf, B.. Elliptic equations and systems with critical Trudinger-Moser nonlinearities. Discrete Contin. Dyn. Syst. 30 (2011), 455–476.CrossRefGoogle Scholar
18de Souza, M. and do Ó, J. M.. Hamiltonian elliptic systems in ℝ2 with subcritical and critical exponential growth. Ann. Mat. 195 (2016), 935–956.CrossRefGoogle Scholar
19do Ó, J. M.. N-Laplacian equations in R N with critical growth. Abstr. Appl. Anal. 2 (1997), 301–315.CrossRefGoogle Scholar
20do Ó, J. M. and Souto, M. S.. On a class of nonlinear Schrödinger equations in ℝ2 involving critical growth. J. Differ. Equ. 174 (2001), 289–311.CrossRefGoogle Scholar
21Ekeland, I.. On the variational principle. J. Math. Anal. Appl. 47 (1974), 324–353.CrossRefGoogle Scholar
22Esteban, M.. Nonsymmetric ground states of symmetric variational problems. Comm. Pure Appl. Math. 44 (1991), 259–274.CrossRefGoogle Scholar
23Hulshof, J. and van derVorst, R.. Differential systems with strongly indefinite variational structure. J. Funct. Anal. 114 (1993), 32–58.CrossRefGoogle Scholar
24Kavian, O.. Introduction a la Theorie des Points Critiques et Applications aux Problemes Elliptiques (Paris: Springer Verlag, 1993).Google Scholar
25Kryszewski, W. and Szulkin, A.. An infinite dimensional Morse theory with applications. Trans. Am. Math. Soc. 349 (1997), 3181–3234.CrossRefGoogle Scholar
26Lam, N. and Lu, G.. Existence and multiplicity of solutions to equations of N-Laplacian type with critical exponential growth in ℝ N. J. Funct. Anal. 262 (2012), 1132–1165.CrossRefGoogle Scholar
27Lam, N. and Lu, G.. Elliptic equations and systems with subcritical and critical exponential growth without the Ambrosetti-Rabinowitz condition. J. Geom. Anal. 24 (2014), 118–143.CrossRefGoogle Scholar
28Li, G. and Yang, J.. Asymptotically linear elliptic systems. Comm. Partial Differ. Equ. 29 (2004), 925–954.CrossRefGoogle Scholar
29Lions, P.-L.. The concentration-compactness principle in the calculus of variations, the locally compact case, parts I and II. Ann. Inst. H. Poincaré 1 (1984), 109–145.CrossRefGoogle Scholar
30Lions, P.-L.. The concentration-compactness principle in the calculus of variations. The limit case. I. Rev. Mat. Iberoamericana 1 (1985), 145–201.CrossRefGoogle Scholar
31Masmoudi, N. and Sani, F.. Trudinger-Moser inequalities with the exact growth condition in ℝN and applications. Comm. Partial Differ. Equ. 49 (2015), 1408–1440.CrossRefGoogle Scholar
32Moser, J.. A sharp form of an inequality by N. Trudinger. Indiana Univ. Math. J. 20 (1971), 1077–1092.CrossRefGoogle Scholar
33Pankov, A.. Periodic nonlinear Schrödinger equation with application to photonic crystals. Milan J. Math. 73 (2005), 259–287.CrossRefGoogle Scholar
34Pohozaev, S. I.. The Sobolev embedding in the case pl = n, Proceedings of the technical scientific conference on advances of scientific research 1964–1965. Mathematics section, Moscov. Energet. Inst. (1965), 158–170.Google Scholar
35Ruf, B.. A sharp Trudinger-Moser type inequality for unbounded domains in ℝ2. J. Funct. Anal. 219 (2005), 340–367.CrossRefGoogle Scholar
36Ruf, B.. Sani, F.. Ground states for elliptic equations in ℝ2 with exponential critical growth. Geometric properties for parabolic and elliptic PDE's, pp. 251–267, Springer INdAM Ser., 2, Springer, Milan, 2013.Google Scholar
37Sirakov, B.. On the existence of solutions of Hamiltonian elliptic systems in ℝN. Adv. Diff. Equ. 100-12 (2000), 1445–1464.Google Scholar
38Sirakov, B. and Soares, S.. Soliton solutions to systems of coupled Schrodinger equations of Hamiltonian type. Trans. Am. Math. Soc. 362 (2010), 5729–5744.CrossRefGoogle Scholar
39Szulkin, A. and Weth, T.. Ground state solutions for some indefinite variational problems. J. Funct. Anal. 257 (2009), 3802–3822.CrossRefGoogle Scholar
40Szulkin, A. and Weth, T.. The method of Nehari manifold, In Handbook of nonconvex analysis and applications (ed Gao, D. Y. and Motreanu, D.) pp. 597–632 (Boston, MA: International Press, 2010).Google Scholar
41Trudinger, N.. On the embedding into Orlicz spaces and some applications. J. Math. Mech. 17 (1967), 473–484.Google Scholar
42Wang, J., Xu, J. and Zhang, F.. Existence of semiclassical groundstate solutions for semilinear elliptic systems. Proc. R. Soc. Edinb. Sect. A 142 (2012), 867–895.CrossRefGoogle Scholar