Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Harris, B. J.
1987.
On the asymptotic properties of linear differential equations.
Mathematika,
Vol. 34,
Issue. 2,
p.
187.


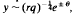 ,
, 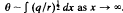 . These involve the coefficients and their derivatives up to some order
. These involve the coefficients and their derivatives up to some order 