No CrossRef data available.
Published online by Cambridge University Press: 18 November 2024
In this article, we explore the bifurcation problem of limit cycles near the double eight figure loop (compound cycle with a 2-polycycle connecting two homoclinic loops). A general theory is established to find the lower bound of the maximal number of limit cycles (isolated periodic orbits) near the double eight figure loop. The Liénard system, a well-known nonlinear dynamical model, appears in a natural way in physics, chemistry, engineering, and so on, where periodic phenomena play a relevant role. As an application, we investigate an 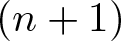 $(n+1)$th-order generalized Liénard system and prove the system has at least
$(n+1)$th-order generalized Liénard system and prove the system has at least 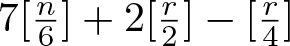 $7[\frac{n}{6}]+2[\frac{r}{2}]-[\frac{r}{4}]$ limit cycles near the double eight figure loop for any
$7[\frac{n}{6}]+2[\frac{r}{2}]-[\frac{r}{4}]$ limit cycles near the double eight figure loop for any 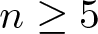 $n\geq5$ and
$n\geq5$ and 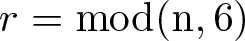 $r=\rm mod(n,6)$, and their distribution is also gained.
$r=\rm mod(n,6)$, and their distribution is also gained.