Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-19T03:49:50.446Z Has data issue: false hasContentIssue false
  • English
  • Français

The form of blow-up for nonlinear parabolic equations

Published online by Cambridge University Press:  14 November 2011

A. A. Lacey
A. A. Lacey
Affiliation:
Department of Mathematics, Heriot-Watt University, Riccarton, Edinburgh EH14 4AS
Get access Rights & Permissions [Opens in a new window]

Synopsis

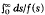Semilinear parabolic equations of the form u1 = ∇2u + δf(u), where f is positive and is finite, are known to exhibit the phenomenon of blow-up, i.e. for sufficiently large S, u becomes infinite after a finite time t*. We consider one-dimensional problems in the semi-infinite region x>0 and find the time to blow-up (t*). Also, the limiting behaviour of u as t→t*- and x→∞ is determined; in particular, it is seen that u blows up at infinity, i.e. for any given finite x, u is bounded as t→t*. The results are extended to problems with convection.

The modified equation xu, = uxx +f(u) is discussed. This shows the possibility of blow-up at x =0 even if u(0, f) = 0. The manner of blow-up is estimated.

Finally, bounds on the time to blow-up for problems in finite regions are obtained by comparing u with upper and lower solutions.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 98 , Issue 1-2 , 1984 , pp. 183 - 202
Copyright
Copyright © Royal Society of Edinburgh 1984

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Ayeni, R. O.. On the thermal runaway of variable viscosity flows between concentric cylinders. Z. Angew. Math. Phys. 33 (1982), 408–413.CrossRefGoogle Scholar
2Fujita, H.. On the nonlinear equations Δu+eu = 0 and ut =Δu +eu. Bull. Amer. Math. Soc. 75 (1969), 132–135.CrossRefGoogle Scholar
3Kapila, A. K.. Reactive-diffusive system with Arrhenius kinetics: dynamics of ignition. SIAM J. Appl. Math. 39 (1980), 21–36.CrossRefGoogle Scholar
4Kassoy, D. R. and Poland, J.. The thermal explosion confined by a constant temperature boundary: I The induction period solution. SIAM J. Appl. Math. 39 (1980). 412–430.CrossRefGoogle Scholar
5Kassoy, D. R. and Poland, J.. The thermal explosion confined by a constant temperature boundary: II The extremely rapid transient. SIAM J. Appl. Math. 41 (1981), 231–246.CrossRefGoogle Scholar
6Lacey, A. A.. The spatial dependence of supercritical reacting systems. IMA J. Appl. Math. 27 (1981). 71–84.CrossRefGoogle Scholar
7Lacey, A. A.. Mathematical analysis of thermal runaway for spatially inhomogeneous reactions.SIAMJ. Appl. Math. 43 (1983), 1350–1366.CrossRefGoogle Scholar
8Levine, H. A.. Nonexistence of global weak solutions to some properly and improperly posed problems of mathematical physics: the method of unbounded Fourier coefficients. Math. Ann. 214 (1975), 205–220.CrossRefGoogle Scholar
9Ockendon, H.. Channel flow with temperature-dependent viscosity and internal viscous dissipation. J. Fluid Mech. 93 (1979), 737–746.CrossRefGoogle Scholar
10Pao, C. V.. Nonexistence of global solutions and bifurcation analysis of a boundary-value problemof parabolic type. Proc. Amer. Math. Soc. 65 (1977), 245–251.CrossRefGoogle Scholar
11Weissler, F. B.. Single point blow-up for a semilinear initial value problem. To appear.Google Scholar