This paper is concerned with the following semilinear elliptic equations of the form

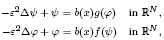
where ε is a small positive parameter, and where f and g denote superlinear and subcritical nonlinearity. Suppose that b(x) has at least one maximum. We prove that the system has a ground-state solution (ψε, φε) for all sufficiently small ε > 0. Moreover, we show that (ψε, φε) converges to the ground-state solution of the associated limit problem and concentrates to a maxima point of b(x) in certain sense, as ε → 0. Furthermore, we obtain sufficient conditions for nonexistence of ground-state solutions.