Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gilbert, D. J.
and
Harris, B. J.
2000.
Bounds for the points of spectral concentration of Sturm–Liouville problems.
Mathematika,
Vol. 47,
Issue. 1-2,
p.
327.
Gilbert, D.J.
and
Harris, B.J.
2001.
On the Recovery of a Differential Equation from Its Spectral Functions.
Journal of Mathematical Analysis and Applications,
Vol. 262,
Issue. 1,
p.
355.
Riehl, S. M.
2003.
The form of the spectral functions associated with Dirac equations.
Mathematika,
Vol. 50,
Issue. 1-2,
p.
153.
Gilbert, D.J.
Harris, B.J.
and
Riehl, S.M.
2004.
The spectral function for Sturm–Liouville problems where the potential is of Wigner–von Neumann type or slowly decaying.
Journal of Differential Equations,
Vol. 201,
Issue. 1,
p.
139.


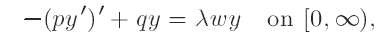
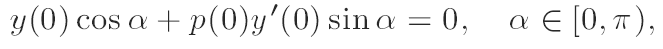
 its symmetric derivative. We show that for almost all μ ∈ R, if
its symmetric derivative. We show that for almost all μ ∈ R, if 
