Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bing, Xiao
Qifeng, Wu
Wenjun, Yuan
and
Domoshnitsky, Alexander I.
2011.
Composite Holomorphic Functions and Normal Families.
Abstract and Applied Analysis,
Vol. 2011,
Issue. 1,
Yuan, Wenjun
Xiao, Bing
and
Wu, Qifeng
2011.
Composite meromorphic functions and normal families.
Archiv der Mathematik,
Vol. 96,
Issue. 5,
p.
435.
Battaglia, Erika
and
Bonfiglioli, Andrea
2014.
Normal families of functions for subelliptic operators and the theorems of Montel and Koebe.
Journal of Mathematical Analysis and Applications,
Vol. 409,
Issue. 1,
p.
1.
Grahl, Jürgen
and
Nevo, Shahar
2014.
Exceptional functions wandering on the sphere and normal families.
Israel Journal of Mathematics,
Vol. 202,
Issue. 1,
p.
21.
Charak, Kuldeep Singh
and
Singh, Virender
2016.
Sharing of a set of meromorphic functions and Montel’s theorem.
Archiv der Mathematik,
Vol. 106,
Issue. 3,
p.
257.
Chen, Shirong
and
Xu, Yan
2021.
Normality relationships between two families concerning shared values.
Monatshefte für Mathematik,
Vol. 196,
Issue. 1,
p.
1.
Singh, Virender
and
Lal, Banarsi
2022.
Normality and Product of Spherical Derivatives.
Acta Mathematica Vietnamica,
Vol. 47,
Issue. 2,
p.
571.
Lal, Banarsi
and
Singh, Virender
2023.
On $$\varphi $$-normal meromorphic functions.
Rendiconti del Circolo Matematico di Palermo Series 2,
Vol. 72,
Issue. 2,
p.
1277.
Singh, Virender
and
Lal, Banarsi
2023.
A generalization of Lappan’s five point theorem.
Arabian Journal of Mathematics,
Vol. 12,
Issue. 3,
p.
697.
Lal, Banarsi
and
Singh, Virender
2024.
Sharing on a punctured domain and normality.
São Paulo Journal of Mathematical Sciences,
Vol. 18,
Issue. 2,
p.
1807.
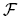 is a family of meromorphic functions in a domain D ⊂ ℂ, and a(z), b(z) and c(z) are distinct meromorphic functions in D and if, for all f ∈
is a family of meromorphic functions in a domain D ⊂ ℂ, and a(z), b(z) and c(z) are distinct meromorphic functions in D and if, for all f ∈ 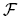 and all z ∈ D, f(z) ≠ a(z), f(z) ≠ b(z) and f(z) ≠ c(z), then
and all z ∈ D, f(z) ≠ a(z), f(z) ≠ b(z) and f(z) ≠ c(z), then 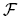 is normal in D. Secondly, letting R(w) be a rational function of degree greater than or equal to 3 and
is normal in D. Secondly, letting R(w) be a rational function of degree greater than or equal to 3 and 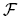 be a family of functions meromorphic in a domain D ⊂ ℂ, if there exists a non-constant meromorphic function α(z) in D such that, for all f ∈
be a family of functions meromorphic in a domain D ⊂ ℂ, if there exists a non-constant meromorphic function α(z) in D such that, for all f ∈ 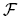 and z ∈ D, R(f(z)) ≠ α(z), then
and z ∈ D, R(f(z)) ≠ α(z), then 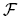 is normal in D.
is normal in D.