We study curvature structures of compact hypersurfaces in the unit sphere Sn+1(1) with two distinct principal curvatures. First of all, we prove that the Riemannian product 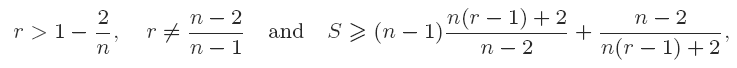is the only compact hypersurface in Sn+1(1) with two distinct principal curvatures, one of which is simple and satisfies where n(n − 1)r is the scalar curvature of hypersurfaces and c2 = (n − 2)/nr. This generalized the result of Cheng, where the scalar curvature is constant is assumed. Secondly, we prove that the Riemannian product 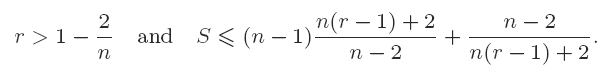is the only compact hypersurface with non-zero mean curvature in Sn+1(1) with two distinct principal curvatures, one of which is simple and satisfies This gives a partial answer for the problem proposed by Cheng.