No CrossRef data available.
Published online by Cambridge University Press: 11 January 2024
We investigate the existence and branching patterns of wave trains in the mass-in-mass (MiM) lattice, which is a variant of the Fermi–Pasta–Ulam (FPU) lattice. In contrast to FPU lattice, we have to solve coupled advance-delay differential equations, which are reduced to a finite-dimensional bifurcation equation with an inherited Hamiltonian structure by applying a Lyapunov–Schmidt reduction and invariant theory. We establish a link between the MiM lattice and the monatomic FPU lattice. That is, the monochromatic and bichromatic wave trains persist near $\mu =0$ in the nonresonance case and in the resonance case $p:q$
in the nonresonance case and in the resonance case $p:q$ where $q$
where $q$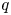 is not an integer multiple of $p$
is not an integer multiple of $p$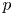 . Furthermore, we obtain the multiplicity of bichromatic wave trains in $p:q$
. Furthermore, we obtain the multiplicity of bichromatic wave trains in $p:q$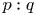 resonance where $q$
resonance where $q$ is an integer multiple of $p$
is an integer multiple of $p$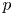 , based on the singular theorem.
, based on the singular theorem.